2022 Live Review 3 | AP Physics C: Mechanics | Work, Energy, and Power
TLDRIn this educational video, Angela Genswold, a physics teacher from Diamond Bar High School, California, delves into the concepts of work, energy, and power within the realm of Physics C Mechanics. She explains that work is a transfer of energy into or out of a system, emphasizing the importance of defining the system for analysis. Genswold covers the conservation of mechanical energy, the mathematical definition of work as an integral of force and displacement, and the concept of power as the rate of work done. She also guides viewers through multiple-choice questions and free-response problems to illustrate the application of these principles, concluding with the significance of work in energy transfer and system analysis.
Takeaways
- 📚 The session focuses on the concepts of work, energy, and power within the context of Physics C Mechanics.
- 🔍 Work is defined as the transfer of energy into or out of a system, and it's crucial to first define the system for analysis.
- 🔄 Mechanical energy conservation states that if no external forces do work on a system, the sum of kinetic and potential energies remains constant.
- ⚙️ The mathematical definition of work involves integrating the dot product of force and displacement vectors.
- 📉 Power is the rate at which work is done, and it can be calculated as the integral of power with respect to time for variable forces.
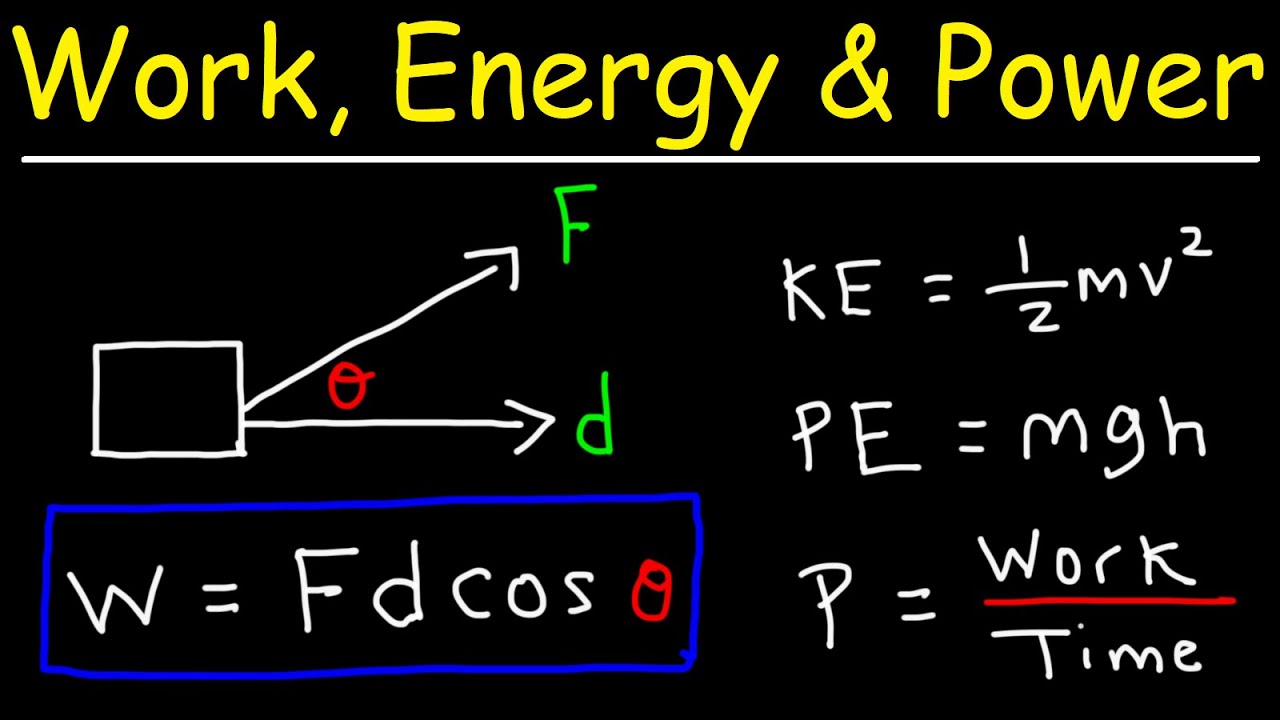
- 📐 For a constant force, work can be calculated using the formula W = Fd cos(θ), where θ is the angle between the force and displacement.
- 🧩 The script includes multiple choice questions to illustrate the calculation of work done by various forces and the change in kinetic energy.
- 📉 It explains how to use graphical integration to determine work from a power-time graph, which represents the area under the curve.
- 🔢 The session covers the calculation of kinetic energy change using both constant force scenarios and situations involving friction.
- 🔍 The importance of defining the system is emphasized for analyzing energy transformations and work done by external forces.
- 📚 The transcript concludes with a review of energy concepts, including potential and kinetic energy, and their transformations in various scenarios.
Q & A
What is the primary focus of the video script?
-The primary focus of the video script is to discuss the concepts of work, energy, and power within the context of physics, specifically for a course on AP Physics C Mechanics.
What is the definition of work given in the script?
-Work is defined as the transfer of energy into or out of a system, and it is calculated as the integral of the dot product of the force and the displacement vector.
What is the condition for mechanical energy conservation in a system?
-Mechanical energy, which is the sum of kinetic and potential energies, is conserved in a system when there are no external forces doing work on it.
How is power related to work?
-Power is the rate at which work is done. It can be calculated as the integral of power with respect to time or, for a constant force, as the product of the force, displacement, and cosine of the angle between them.
What is the significance of defining a system in physics problems?
-Defining a system is crucial because it helps to determine which forces are internal and which are external to the system. This distinction is important for analyzing energy transfers and work done within the context of the problem.
How is the work done by a force on a particle calculated when moving from x=2 meters to x=1 meter with a force given by kx^3?
-The work done by the force is calculated by integrating the force (kx^3) over the displacement from x=2 meters to x=1 meter, taking into account the direction of the force and displacement. The work in this case is found to be 15 joules.
What is the relationship between the work done by a motor and the area under its power-time graph?
-The work done by the motor during a specific time interval is equal to the area under the power-time graph for that interval, as work is the integral of power with respect to time.
How can the change in kinetic energy of a block be determined when an external force is applied?
-The change in kinetic energy can be determined by calculating the work done by the external force using the formula Fd cos(theta), where F is the force, d is the displacement, and theta is the angle between the force and displacement vectors.
What is the role of friction in the context of energy conservation?
-Friction is a force that dissipates energy from a system, typically converting mechanical energy into thermal energy or internal energy, thus reducing the mechanical energy of the system.
How can the coefficient of friction between a box and a floor be calculated if the initial and final kinetic energies are known?
-The coefficient of friction can be calculated using the work-energy principle, where the work done by friction (negative of the product of the coefficient of friction, normal force, and distance) equals the change in kinetic energy of the system.
What is the change in potential energy when a spring is stretched from its equilibrium position by a certain amount?
-The change in potential energy when a spring is stretched is equal to the integral of the force (given by the spring's force equation) with respect to the displacement from the equilibrium position to the final position.
How does the graph of mechanical energy of a cart-spring-earth system as a function of time differ from the graph of the cart system alone?
-The cart-spring-earth system includes both potential and kinetic energy transformations, while the cart system alone only includes kinetic energy since it cannot have gravitational potential energy without another object or height difference.
What is the procedure to determine the acceleration due to gravity g using a block, a spring, and a frictionless table?
-The procedure involves measuring the spring compression and the horizontal distance the block travels while airborne after being released. These measurements are used in an equation derived from energy conservation principles to calculate the acceleration due to gravity.
How can the acceleration of the compartment in an amusement park ride be described when it is brought to rest by a braking force?
-The acceleration can be described using a differential equation based on Newton's second law, where the braking force (negative kv) is the only horizontal force acting on the compartment. The solution to this differential equation gives the velocity as a function of time.
What is the initial acceleration of the compartment in the amusement park ride when brought to rest by a braking force?
-The initial acceleration of the compartment is the braking force divided by the mass of the compartment, which is kvd/m at time zero, where vd is the velocity of the compartment at the start of braking.
What are the intercepts, asymptotes, maxima, or minima of the graph representing the magnitude of acceleration of the compartment as a function of time?
-The graph has an initial intercept at kvd/m, representing the maximum acceleration at time zero. There is an asymptote at zero acceleration, indicating that the compartment comes to rest over time due to the braking force.
Outlines
📚 Introduction to Work, Energy, and Power Concepts
The video begins with an introduction to the topic of energy, focusing on work, energy, and power within the context of physics. Angela Genswold, a teacher from Diamond Bar High School, California, explains that work is the transfer of energy into or out of a system. She emphasizes the importance of defining the system for analysis. The concept of mechanical energy conservation is introduced, stating that if no external forces do work on the system, the sum of kinetic and potential energies remains constant. The mathematical definition of work as the integral of the dot product of force and displacement vector is provided. Power is defined as the rate at which work is done, with formulas for constant force scenarios also given. The segment ends with multiple-choice questions to illustrate the concepts discussed.
🔍 Work and Energy Calculations with Multiple Choice Questions
This paragraph delves into specific problems involving calculations of work and energy. The first problem involves calculating the work done by a force given by the equation kx^3 as a particle moves along the x-axis. The work is determined to be negative, reflecting the force and displacement being in opposite directions. The integration of the force equation from x=2 to x=1 yields the work done as 15 joules. The second problem involves a pump motor's power output graph, where the area under the graph represents the work done over the interval from 0 to 8 seconds, calculated to be 42 kilojoules. The third problem discusses the change in kinetic energy of a block moved by a force at an angle on a frictionless surface, using the work-energy principle to find an increase in kinetic energy. The final problem examines the change in kinetic energy of a box pushed across a rough floor, using an energy bar chart to account for work done and energy lost to friction, concluding with 60 joules allocated to kinetic energy.
📘 Coefficient of Friction and Spring Potential Energy
The focus shifts to determining the coefficient of friction between a box and a floor using principles of energy conservation and work done by friction. By equating the work done by friction to the change in kinetic energy, the coefficient of friction is calculated using the formula mv^2/2mg. The next problem involves a non-linear spring, where the force required to stretch the spring is given by 40x - 6x^2. The change in potential energy when the spring is stretched two meters from its equilibrium position is found by integrating the force function over the displacement, resulting in 64 joules. These problems highlight the application of work-energy principles in different physical scenarios.
🌐 Energy Conservation in a Spring-Cart System
This section discusses an example involving a cart compressing a spring, situated on a table above the ground, and the energy transformations that occur when the cart is released. The cart-spring-earth system is analyzed at different time points, illustrating the conservation of mechanical energy. The spring does work on the system, transferring energy into it, and the cart's potential energy is transformed into kinetic energy and vice versa. The problem also includes a question about which graph represents the mechanical energy of the cart-earth system over time, with the correct answer being graph B. The analysis includes a comparison between the cart-spring system and the cart-earth system, explaining why the total mechanical energy remains constant in each case.
🧩 Determining Acceleration Due to Gravity Using a Spring-Block System
The paragraph outlines an experiment to determine the acceleration due to gravity (g) using a block, a spring, and a frictionless table. The procedure involves measuring the spring compression (l) and the horizontal distance (x) the block travels while airborne after being released. The relationship between l^2 and x is derived from energy conservation principles, leading to a linear graph where the slope can be used to calculate g. The experiment's steps are detailed, including measuring the necessary quantities and ensuring accuracy through multiple trials. The paragraph concludes with a discussion of how to graph the data and use it to find g.
🎢 Analysis of a Passenger Compartment on an Amusement Park Ride
The video script presents a scenario where a passenger compartment on an amusement park ride moves from rest at point A to point E along a track. The compartment experiences free fall and circular motion, and the forces acting on it at point C are identified as the gravitational force and the normal force from the track. An expression for the centripetal force at point C is derived using geometric relationships. The speed of the compartment at point D is calculated using conservation of energy principles, and the scenario where the compartment is brought to rest by friction is analyzed to find the coefficient of friction. Additionally, a case with no friction but with a braking force proportional to velocity is considered, leading to a differential equation for velocity as a function of time.
🛑 Conclusion on Work, Energy, and System Analysis
The final paragraph wraps up the video by reiterating the importance of work as a transfer of energy and the necessity of defining the system for analysis. It summarizes the mathematical definition of work and the conditions for energy conservation. Power is again defined as the rate of work done, and the formula for work done by a constant force is reiterated. The video concludes with an invitation to tune in for the next session on linear momentum and collisions, emphasizing the interconnectedness of these concepts in physics.
Mindmap
Keywords
💡Work
💡Energy Conservation
💡Power
💡Kinetic Energy
💡Potential Energy
💡Force
💡Displacement
💡Mechanical Energy
💡Friction
💡Spring Constant
💡Centripetal Force
Highlights
Introduction to the concept of work as a transfer of energy into or out of a system, emphasizing the importance of defining the system for analysis.
Explanation of mechanical energy conservation when no external forces act on a system, highlighting the sum of kinetic and potential energies.
Mathematical definition of work through the integral of the dot product of force and displacement vector.
Power defined as the rate at which work is done, with a formula for constant force scenarios.
Warm-up multiple-choice questions to apply concepts of work, energy, and power.
Solution to a problem involving work done by a force given by kx^3, integrating to find the change in energy.
Graphical method to determine work done over a time interval by calculating areas under a power-time graph.
Calculation of kinetic energy change using work done by an external force and the fd*cosine(theta) formula.
Use of an energy bar chart to visualize the change in kinetic energy with work done and energy dissipated by friction.
Determination of the coefficient of friction using the work-energy principle and force diagram analysis.
Calculation of potential energy change in a non-linear spring using integral calculus and force-displacement relationship.
Analysis of mechanical energy graphs for a cart-spring system to understand energy transformations over time.
Explanation of how to create graphs of mechanical energy for different defined systems to analyze energy changes.
Procedure for an experiment to determine gravitational acceleration g using a spring, block, and table setup.
Method to graph data from an experiment to find g, using the relationship between spring compression and horizontal distance.
Consideration of non-constant quantities during a collision in an amusement park ride physics problem.
Derivation of expressions for centripetal force and speed of a passenger compartment in a circular motion scenario.
Calculation of the coefficient of friction needed to stop a compartment using kinetic energy and work done by friction.
Development of a differential equation for velocity as a function of time with a braking force.
Integration to solve the differential equation for velocity, leading to an expression for velocity over time.
Graphing of acceleration as a function of time for a compartment with braking force, noting intercepts and asymptotes.
Summary of key concepts: work as energy transfer, system definition, energy conservation, and power as work rate.
Transcripts
Browse More Related Video
Work, Energy, and Power - Basic Introduction
AP Physics 1 review of Energy and Work | Physics | Khan Academy

AP Physics 1: Work, Energy and Power Review

College Physics 1: Lecture 28 - Work and Energy

AP Physics C: Work, Energy, and Power Review (Mechanics)

8.01x - Lect 11 - Work, Kinetic & Potential Energy, Gravitation, Conservative Forces
5.0 / 5 (0 votes)
Thanks for rating: