Second fundamental theorem and chain rule | MIT 18.01SC Single Variable Calculus, Fall 2010

MIT OpenCourseWare
7 Jan 201105:04
EducationalLearning
32 Likes 10 Comments

TLDRIn this recitation video, the professor guides students through the application of the fundamental theorem of calculus and the chain rule to find the derivative of a composite function involving an integral. The problem involves differentiating the integral from 0 to x squared of the cosine function, which is not directly solvable by the fundamental theorem alone due to the presence of x squared. The solution process is clearly explained, demonstrating how to handle more complex integral expressions by combining these two mathematical concepts, ultimately arriving at the answer, 2x times cosine of x squared.

Takeaways
  • 📚 The problem involves finding the derivative of an integral, specifically ∫ from 0 to x squared of cosine t dt.
  • 🤔 The challenge is that the integral is of a function of x (x squared), not just x, which complicates the application of the fundamental theorem of calculus.
  • 🌟 The solution strategy combines the fundamental theorem of calculus with the chain rule to tackle the problem.
  • 📈 Define F(x) as the integral from 0 to x of cosine t dt, which allows us to use the fundamental theorem to find F'(x) = cosine x.
  • 🔄 To find the derivative of F(x squared), consider F(x) as the outer function and x squared as the inner function, applying the chain rule.
  • 📝 The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function, multiplied by the derivative of the inner function.
  • 🧮 The derivative of ∫ from 0 to x squared of cosine t dt is F'(x squared) = F'(x) * 2x, where F'(x) is the derivative of F at x.
  • 🥂 Evaluating F'(x) at x squared gives us cosine of x squared, so the final answer is 2x * cosine of x squared.
  • 🎯 This method is generalizable to any function in place of x squared, making it a powerful tool for solving similar calculus problems.
  • 🌐 The lesson emphasizes the importance of understanding the fundamental theorem of calculus and the chain rule for solving a wide range of derivative problems.
Q & A

  • What is the main problem presented in the video?

    -The main problem is to find the derivative of the integral from 0 to x squared of cosine t dt.

  • What theorem is mentioned as a basis for solving simpler integral problems?

    -The fundamental theorem of calculus is mentioned as the basis for solving simpler integral problems.

  • How does the presence of x squared complicate the problem?

    -The presence of x squared complicates the problem because it is not a simple function like x, which would make the problem easy to solve using the fundamental theorem of calculus.

  • Which two mathematical rules are combined to solve the given problem?

    -The fundamental theorem of calculus and the chain rule are combined to solve the given problem.

  • How is the chain rule applied in this context?

    -The chain rule is applied by considering the composition of functions, where the outside function is F(x) and the inside function is x squared, leading to the derivative of F evaluated at x squared times the derivative of x squared.

  • What is the final answer to the problem?

    -The final answer to the problem is 2x times cosine of x squared.

  • What does the professor emphasize about the process used to solve the problem?

    -The professor emphasizes that the process can be generalized to solve problems where any function of x is integrated from 0 to x.

  • How does the problem illustrate the application of the fundamental theorem of calculus?

    -The problem illustrates the application of the fundamental theorem of calculus by showing how it can be used in conjunction with the chain rule to find the derivative of a more complex integral expression.

  • What alternative function could be used in place of x squared according to the professor?

    -According to the professor, alternative functions such as natural log x or a complex polynomial could be used in place of x squared.

  • How does the video aim to enhance the understanding of calculus concepts?

    -The video aims to enhance the understanding of calculus concepts by demonstrating the step-by-step process of solving a more complex problem using fundamental theorem of calculus and the chain rule.

  • What is the significance of the 2x term in the final answer?

    -The 2x term in the final answer represents the derivative of x squared, which is used in the chain rule to find the derivative of the composite function F(x squared).

Outlines
00:00
📚 Introduction to the Calculus Problem

The professor begins the recitation by introducing a calculus problem involving the derivative of a definite integral. The problem is to find the derivative of the integral from 0 to x squared of the cosine function with respect to t. The professor encourages students to think about the problem before proceeding to solve it. The main theme of this paragraph is the presentation of the problem and the initial approach to solving it using the fundamental theorem of calculus and the chain rule.

🤔 Problem Analysis and Breakdown

In this segment, the professor analyzes the problem and highlights the challenge of having a function of x (in this case, x squared) under the integral sign. The professor explains that the straightforward application of the fundamental theorem of calculus would be possible if the function was just x. The key point here is understanding the need to combine the fundamental theorem with the chain rule to tackle the problem effectively.

🧠 Combining Fundamental Theorem and Chain Rule

The professor proceeds to solve the problem by defining a function F(x) as the integral from 0 to x of cosine t dt. The explanation includes the application of the chain rule, where the derivative of F evaluated at x squared is multiplied by the derivative of x squared. The professor emphasizes the generalizability of this method, stating that it can be applied to any function of x under the integral sign. The summary of this paragraph focuses on the step-by-step process of solving the problem using calculus concepts and the broader implications for solving similar problems.

🎓 Conclusion and Generalization

The professor concludes the problem-solving segment by reiterating the process used to solve the problem, which involved the fundamental theorem of calculus and the chain rule. The professor emphasizes the importance of understanding how to apply this method to any function of x under the integral sign, thereby providing students with a powerful tool for solving a wide range of calculus problems. The main takeaway from this paragraph is the broader applicability of the method demonstrated and the encouragement for students to apply this knowledge to other problems.

Mindmap
Keywords
💡Recitation
Recitation in the context of the video refers to a type of academic session where a professor or teacher goes over problems or concepts with students, often in a step-by-step manner. This is a method of teaching that allows for interactive learning and immediate feedback. In the video, the professor uses recitation to guide students through the process of solving a calculus problem, demonstrating how to apply specific theorems and rules.
💡Integral
An integral is a fundamental concept in calculus that represents the accumulation of a quantity, such as the area under a curve, over a given interval. Integrals are used to calculate the total change or accumulation of a variable and are closely related to the concept of a function's rate of change, or derivative. In the video, the professor is working with an integral expression, which involves integrating a function with respect to 't' from 0 to 'x squared'.
💡Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus is a key theorem that connects the concepts of differentiation and integration, the two main operations in calculus. It states that if a function can be integrated, then it can also be differentiated, and vice versa. This theorem is crucial for solving problems that involve both integration and differentiation, as it allows us to find the derivative of an integral. In the video, the professor refers to the Fundamental Theorem of Calculus as a basis for solving the given problem, indicating that it simplifies the process when the function being integrated is a simple one, like 'x'.
💡Chain Rule
The Chain Rule is a mathematical principle used in calculus to find the derivative of a composite function, which is a function made up of other functions. It states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function. This rule is essential for solving problems where a function is composed of multiple functions or where a function is evaluated at another function's result. In the context of the video, the Chain Rule is used to find the derivative of the integral of 'cosine t' from 0 to 'x squared'. The professor explains that the Chain Rule allows us to break down the problem into simpler parts by treating 'F of x' as the outer function and 'x squared' as the inner function.
💡Derivative
A derivative in calculus is a measure of how a function changes as its input changes. It gives us the rate of change or the slope of the function at any point. Derivatives are used to analyze the behavior of functions, such as their maxima, minima, and inflection points, as well as to model real-world phenomena like motion and growth. In the video, the concept of the derivative is central to the problem-solving process. The professor is looking to find the derivative of an integral expression, which involves understanding the relationship between the integral (area under a curve) and the derivative (slope of the curve).
💡Cosine Function
The cosine function is a trigonometric function that describes periodic phenomena in mathematics. It is commonly used to model wave-like behavior and is defined as the ratio of the adjacent side to the hypotenuse in a right-angled triangle, where the angle varies. In the provided script, the cosine function is the function being integrated with respect to 't'. The professor discusses how the derivative of the integral of the cosine function would be if the function inside the integral were a simple one like 'x', and then extends this concept to handle more complex functions like 'x squared'.
💡x squared
In mathematics, 'x squared' refers to the square of the variable 'x', which is denoted as 'x^2'. This operation is an example of exponentiation, where a number is raised to the power of 2. In the context of the video, 'x squared' is the upper limit of the integral, which complicates the problem compared to a simpler function like 'x'.
💡Function Composition
Function composition is a mathematical operation where a function is applied to the result of another function. It is denoted by f(g(x)), where 'f' is the outer function and 'g' is the inner function. This concept is crucial when dealing with composite functions, where one function is nested within another. In the video, the professor discusses the concept of function composition when explaining how to find the derivative of 'F of x squared'. The professor describes 'F' as the outer function and 'x squared' as the inner function, emphasizing the need to apply the Chain Rule to differentiate the composite function.
💡Rate of Change
The rate of change is a mathematical term that describes how quickly a quantity changes with respect to another quantity. In calculus, this concept is often used to describe the derivative of a function, which gives the rate of change of the function's value at a specific point. In the context of the video, the rate of change is central to the problem, as the professor is looking to find the derivative of an integral expression. The derivative provides information about the rate of change of the integral with respect to 'x'.
💡Trigonometry
Trigonometry is a branch of mathematics that deals with the relationships between the angles and sides of triangles, particularly right-angled triangles. It involves the use of trigonometric functions such as sine, cosine, and tangent, which are used to model periodic phenomena and solve problems involving angles and lengths. In the video, trigonometry is relevant because the function being integrated is the cosine function, a fundamental trigonometric function. The professor's discussion of the integral of the cosine function and the subsequent derivative calculation are rooted in trigonometric principles.
💡Slope
The slope of a curve at a particular point is a measure of how steep the curve is at that point. It is a concept from geometry and calculus that describes the rate at which the y-value of a function changes as the x-value changes. The slope is the tangent of the angle made by the curve with the x-axis at that point. In the video, the concept of slope is implicitly discussed when the professor talks about derivatives. Since the derivative of a function at a point gives the slope of the tangent line to the curve at that point, understanding slopes is crucial for visualizing and calculating the behavior of functions.
Highlights

Introduction to the calculus problem involving the derivative of an integral.

The problem involves a function inside the integral (x^2) instead of just x.

The solution process combines the fundamental theorem of calculus with the chain rule.

Defining F(x) as the integral from 0 to x of cosine t dt.

The derivative of F(x) is cosine x, based on the fundamental theorem of calculus.

The problem requires finding the derivative of F(x^2), not just F(x).

The chain rule is applied to find the derivative of the composite function F(x^2).

The derivative of the outside function F is evaluated at x^2.

The derivative of the inside function x^2 is 2x.

The final answer is 2x times cosine of x squared.

The method can be generalized to solve problems with any function of x inside the integral.

The process involves defining F(x) according to the fundamental theorem of calculus.

Identifying F'(x) is crucial for applying the chain rule.

Evaluating F at the given function inside the integral is a key step.

The derivative of the inside function is multiplied with F' evaluated at the inside function.

The solution emphasizes the broader applicability of the method beyond the specific problem.

The problem-solving approach is demonstrated to be flexible and adaptable for various functions.

Transcripts

Browse More Related Video

Derivatives of Integrals (w/ Chain Rule)

Finding derivative with fundamental theorem of calculus: x is on both bounds | Khan Academy

Definite Integral by Substitution | MIT 18.01SC Single Variable Calculus, Fall 2010

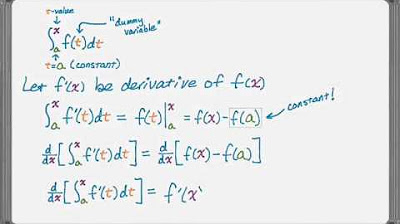
Second Fundamental Theorem of Calculus 2nd FTC

Worked example: Finding derivative with fundamental theorem of calculus | Khan Academy

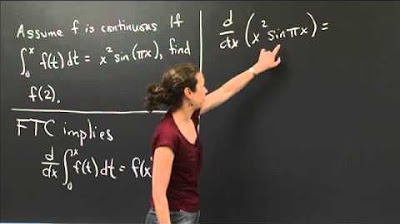
Applying the Second Fundamental Theorem | MIT 18.01SC Single Variable Calculus, Fall 2010

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus TechniquesIntegral DerivativesChain RuleFundamental TheoremVariable FunctionsMathematical AnalysisEducational ContentOnline LectureAdvanced MathCosine Function