Proof: d/dx(e^x) = e^x | Taking derivatives | Differential Calculus | Khan Academy

Khan Academy
27 Apr 200804:40
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the fascinating mathematical concept that the derivative of e to the power of x (e^x) is always equal to e^x itself. This unique property of e^x is highlighted as mind-boggling, with the slope of the function at any point being equal to the function's value at that point. The script also touches on the method of proving this property using the definition of e and the chain rule, emphasizing its significance in calculus and its profound implications for understanding mathematical functions.

Takeaways
  • 📚 The derivative of e to the x is equal to e to the x, showcasing a unique property of the exponential function.
  • 🤔 The slope of the function e to the x at any point is equal to the value of the function at that point, which is a mind-boggling concept.
  • 🔄 The second and all higher-order derivatives of e to the x are also equal to e to the x, indicating a consistent behavior across different orders of differentiation.
  • 📈 The proof of the derivative of e to the x can be approached by using the definition of e and the limit as n approaches infinity.
  • 🌟 The natural logarithm function, ln(x), has been proven to have a derivative of 1/x, which is a fundamental result in calculus.
  • 🔗 The chain rule is applied to demonstrate the derivative of e to the x, involving the inner and outer functions and their derivatives.
  • 🎨 The process of proving the derivative of e to the x can be visualized through different methods, such as direct calculation or using the chain rule.
  • 🧠 Understanding the derivative of e to the x is not only a mathematical exercise but also an opportunity to appreciate the beauty and intricacies of calculus.
  • 🌐 The significance of the derivative of e to the x extends beyond mathematics, as it can lead to profound insights and further explorations in various fields.
  • 🚀 The concept of the derivative of e to the x being equal to itself serves as a foundation for more advanced and dramatic mathematical results in the future.
Q & A

  • What is the main concept discussed in the transcript?

    -The main concept discussed in the transcript is the unique property of the derivative of the exponential function e^x, which is equal to the function itself.

  • What does the speaker find amazing about calculus or math?

    -The speaker finds it amazing that the derivative of e^x at any point is equal to e, and that all higher-order derivatives of e^x are also equal to e^x.

  • How does the speaker describe the relationship between the slope of the function e^x and its value at a point?

    -The speaker describes that the slope of the function e^x at any point is equal to the value of the function at that point, meaning the derivative equals the function itself.

  • What is the definition of e used in the proof?

    -The definition of e used in the proof is the limit as n approaches infinity of (1 + 1/n)^n.

  • How is the derivative of ln(x) related to the derivative of e^x?

    -The derivative of ln(x) is equal to 1/x, which is used in the proof to show that the derivative of e^x is e^x.

  • What mathematical concept is used to derive the derivative of e^x?

    -The chain rule is used to derive the derivative of e^x.

  • What does the chain rule state?

    -The chain rule states that the derivative of a composite function f(g(x)) is the derivative of the outer function evaluated at the inner function times the derivative of the inner function.

  • How does the speaker prove that the derivative of e^x is e^x using the chain rule?

    -The speaker takes the derivative of ln(e^x), applies the chain rule, and simplifies the expression to show that the derivative of e^x with respect to x is equal to 1/e^x, which then is multiplied by e^x to show that the derivative is e^x.

  • What is the significance of the property of e^x in calculus?

    -The significance of the property of e^x in calculus is that it demonstrates a unique function whose rate of change is proportional to its own value, which is foundational in understanding exponential growth and decay in various fields.

  • What does the speaker suggest about the importance of this mathematical property?

    -The speaker suggests that the importance of this mathematical property is so profound that it might warrant a national holiday for people to ponder its implications.

  • What can be inferred about the speaker's perspective on the subject?

    -The speaker's perspective on the subject is one of deep fascination and appreciation for the elegance and fundamental nature of the mathematical concepts being discussed.

Outlines
00:00
📚 Derivative of e to the x

The paragraph discusses the unique property of the derivative of e to the power of x (e^x), which is equal to itself. It emphasizes the significance of this mathematical concept, as it implies that the slope of the function at any point is equal to the value of the function at that point. The explanation includes a brief mention of how the number e is defined and how the derivative of natural logarithm (ln x) and log base e (log_e x) were previously established to lead to the current proof. The paragraph also introduces the concept of the chain rule and demonstrates its application to further prove the derivative of e^x. The speaker expresses amazement at the mathematical properties and suggests the profound impact of these concepts.

Mindmap
Keywords
💡Derivative
The derivative is a fundamental concept in calculus that represents the rate of change or the slope of a function at a particular point. In the context of the video, the derivative of an exponential function with base 'e' is shown to be the function itself, which is a remarkable property. This is used to demonstrate the unique behavior of the exponential function in relation to its own rate of change.
💡e to the x
The term 'e to the x' refers to the exponential function with base 'e', where 'e' is a mathematical constant approximately equal to 2.71828. In the video, it is demonstrated that the derivative of 'e to the x' with respect to 'x' is equal to 'e to the x' itself, highlighting the unique nature of the exponential function in calculus.
💡Slope
Slope is a measure of the steepness of a line, representing the rate of change of the 'y' value with respect to the 'x' value. In the video, the slope of the 'e to the x' function at any point is shown to be equal to the value of the function at that point, which is a distinctive property of exponential functions.
💡Logarithm
A logarithm is the inverse operation to exponentiation, where the logarithm of a number is the exponent to which another fixed value, the base, must be raised to produce that number. In the video, the logarithm is used to derive the derivative of 'e to the x' and to establish the relationship between the exponential and logarithmic functions.
💡Chain Rule
The Chain Rule is a fundamental rule in calculus that allows the differentiation of composite functions. It states that the derivative of a function composed of two or more functions is the product of the derivative of the outer function evaluated at the inner function, and the derivative of the inner function. In the video, the Chain Rule is used to prove that the derivative of 'e to the x' is 'e to the x'.
💡Limit
A limit in mathematics is a value that a function or sequence approaches as the input (or index) approaches some point. In the video, the limit is used to define the constant 'e' as the limit of (1 + 1/n)^n as 'n' approaches infinity, which is a key step in establishing the properties of 'e' and its related functions.
💡ln(x)
The notation 'ln(x)' represents the natural logarithm of 'x', which is the logarithm to the base 'e'. In the video, the derivative of 'ln(x)' is shown to be 1/x, which is an important relationship used in the proof of the derivative of 'e to the x'.
💡Rate of Change
The rate of change is a measure of how quickly a quantity changes with respect to another quantity. In the context of the video, the rate of change or derivative of 'e to the x' is shown to be constant and equal to 'e to the x', which is a unique and fascinating property of exponential growth.
💡Exponential Growth
Exponential growth refers to a process where a quantity increases by a constant proportion rather than a constant amount. In the video, the function 'e to the x' exemplifies exponential growth, as its rate of increase is proportional to its current value, leading to very rapid growth over time.
💡Unique Property
A unique property is a characteristic or feature that is distinct to a particular object, function, or concept. In the video, the unique property of the 'e to the x' function is that its derivative is equal to itself, which is not true for most other functions and is a key point of discussion.
💡National Holiday
The term 'national holiday' is used in the video metaphorically to suggest the significance of the mathematical concept being discussed. It implies that the discovery and understanding of the unique properties of 'e to the x' and its derivative are so profound that they deserve a day of celebration and reflection, similar to how national holidays commemorate important cultural or historical events.
Highlights

The derivative of e to the x is equal to e to the x, which is an amazing property of the exponential function.

The slope of the function e to the x at any point is equal to the value of the function at that point, not the x-value.

The second derivative, third derivative, and all higher order derivatives of e to the x are also equal to e to the x, which is mind-boggling.

The number e is defined such that the derivative of e to the x equals the function itself.

The limit definition of e is used, which is the limit as n approaches infinity of 1 over 1 plus n to the end.

The derivative of the natural logarithm ln of x is equal to 1/x, which is a fundamental result in calculus.

The chain rule is applied to prove the derivative of e to the x, involving the derivative of the inner function and the outer function.

The derivative of ln of e to the x is shown to be trivial and equal to 1, using the properties of logarithms.

An alternative proof using the chain rule confirms that the derivative of e to the x is indeed e to the x.

The process of differentiating e to the x involves multiplying both sides of an equation by e to the x, leading to the conclusion.

The mathematical property of e to the x being its own derivative is so fascinating that it might warrant a national holiday for reflection.

The proof of the derivative of e to the x will lead to more dramatic results in future mathematical explorations.

The transcript discusses the profound implications of the mathematical properties of e and its derivatives.

The use of limits and logarithms in proving the derivative of e to the x showcases the interconnectedness of mathematical concepts.

The chain rule is a powerful tool in calculus, as demonstrated by its application in the proof of the derivative of e to the x.

The transcript highlights the beauty of mathematics and the excitement of discovering and proving fundamental properties.

Transcripts

Browse More Related Video

Business Calculus - Math 1329 - Section 4.3 - Derivatives of Exponential and Logarithmic Functions

Lesson 13 - Derivatives and Integrals Exponential Functions (Calculus 1 Tutor)

Maclaurin series of e_ | Series | AP Calculus BC | Khan Academy

Proofs of derivatives of ln(x) and e^x | Taking derivatives | Differential Calculus | Khan Academy

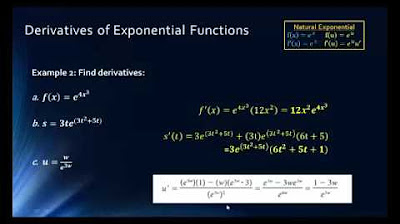
Math1325 Lecture 11 2 - Derivatives of Exponential

2.2 - Derivatives of Exponential Functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusDerivativesMathematical Constantse to the power of xLogarithmsChain RuleInfinite DerivativesEducational ContentMathematical WondersCalculus Fundamentals