Lesson 13 - Derivatives and Integrals Exponential Functions (Calculus 1 Tutor)
TLDRIn this instructional video, the focus is on understanding the derivative and integral of exponential functions. The lecturer clarifies that the derivative of e^x with respect to x is simply e^x, highlighting its unique property as an 'indestructible' function. The antiderivative of e^x is also discussed, which includes a constant, C. The video further illustrates how to apply the chain rule to find the derivative of a composite function, such as f(x) = e^(√x), emphasizing the importance of breaking down the function into simpler components for easier computation.
Takeaways
- 📝 The course section focuses on derivatives and integrals of exponential functions, advancing from basic functions covered earlier.
- 💡 Exponential functions are highlighted as a more complex topic following the basics, with logarithms also mentioned as a future subject.
- 🔮 Emphasizes that while exponential functions may seem more complicated, they are governed by different, straightforward rules.
- 🎶 Introduces the derivative of the exponential function (e^x), humorously dubbed as the 'indestructible function' because it remains unchanged upon differentiation.
- 📈 Clarifies a common misconception: the derivative of (e^x) is not (x × e^{x-1}), highlighting the unique property of (e^x).
- 📚 Advises against applying the formula for the derivative of (x^n) directly to (e^x), emphasizing the difference between a number raised to (x) and (x) raised to a number.
- ➡️ The integral (antiderivative) of (e^x) is also (e^x + C), showcasing the symmetry of differentiation and integration for exponential functions.
- 📌 Encourages students to practice with exercises, suggesting that while the rules are simple, applying them can be less straightforward.
- 📗 Offers an example problem involving (e) raised to the square root of (x), to illustrate the application of the chain rule in derivatives.
- 📃 Breaks down the process of using the chain rule for (e^{\sqrt{x}}), converting the square root into a power of (1/2) and demonstrating step-by-step differentiation.
Q & A
What is the main topic of this section of the course?
-The main topic of this section is the derivative and integral of the exponential function.
What have been discussed in the previous parts of the course?
-In the previous parts of the course, the discussion was focused on how to take the derivative of basic functions and the integral of basic functions.
What is the derivative of the exponential function with respect to X, where the base is e?
-The derivative of the exponential function with respect to X, where the base is e, is e to the X power.
Why is the exponential function referred to as indestructible?
-The exponential function is referred to as indestructible because it cannot be eliminated or altered when taking derivatives of exponential functions, no matter how many times they are differentiated.
What is the antiderivative of e to the X power with respect to X?
-The antiderivative of e to the X power with respect to X is e to the X power plus a constant C.
How does the chain rule apply to the function f(X) = e to the square root of X?
-The chain rule is applied by first rewriting the function as e to the power of X to the 1/2, and then taking the derivative as e to the X to the 1/2 power times the derivative of the exponent (1/2 X to the negative 1/2).
What is the significance of the constant C in the antiderivative of the exponential function?
-The constant C represents an arbitrary constant that is added to the antiderivative to account for the infinite number of solutions that can exist for the integral of a function.
What is the rule for finding the derivative of a function that is a power of X, such as X squared?
-The rule for finding the derivative of a function that is a power of X, such as X squared, is to take the exponent out in front, multiply it by the base X, and then subtract 1 from the exponent.
What is the relationship between the derivative and the integral of the exponential function?
-The relationship between the derivative and the integral of the exponential function is that the derivative of the exponential function is the function itself, and the integral of the exponential function is also the function plus a constant.
Why is it important to remember the rules for derivatives and integrals of exponential functions?
-It is important to remember the rules for derivatives and integrals of exponential functions because they are fundamental to calculus and are used in solving a wide variety of mathematical problems, especially in advanced topics.
What is the role of the exponent when taking the derivative of a function that is a power of X?
-When taking the derivative of a function that is a power of X, the role of the exponent is to determine the new coefficient in the derivative. The exponent is multiplied by the base X, and then 1 is subtracted from the exponent to find the derivative.
Outlines
📚 Introduction to Derivatives and Integrals of Exponential Functions
This paragraph introduces the topic of derivatives and integrals of exponential functions. The speaker begins by welcoming the audience to the section and provides a brief recap of previous lessons on basic functions and their derivatives. The focus then shifts to more advanced concepts, specifically the derivative and integral of exponential functions. The speaker emphasizes that while the rules may differ, the process is not necessarily more difficult, just different. The main point highlighted is that the derivative of an exponential function with base 'e' (Euler's number) is simply the function itself, demonstrating its unique 'indestructible' property. The paragraph concludes with a teaser for upcoming topics, such as logarithms and other advanced functions.
Mindmap
Keywords
💡derivative
💡integral
💡exponential function
💡chain rule
💡e (Euler's number)
💡function
💡constant
💡rules
💡properties
💡antiderivative
💡compound interest
Highlights
The course section focuses on the derivative and integral of the exponential function.
Basic functions' derivatives and integrals were discussed in previous sections.
The exponential function is represented as e^x, where e is approximately 2.71828.
The derivative of e^x with respect to x is e^x, showcasing its unique property.
The term 'indestructible function' is used to describe the exponential function due to its unchanging nature under differentiation.
The integral of e^x is simply e^x plus a constant (C).
The derivative of a number raised to the power of x is that number multiplied by the exponent (x).
The derivative of x^2 is obtained by taking the exponent out and halving the base, then subtracting 1.
The derivative of a function composed of an exponential with a variable inside, such as f(x) = e^(√x), requires the use of the chain rule.
When applying the chain rule, rewrite the function in terms of simpler exponential forms, like e^(x^(1/2)) for e^(√x).
The derivative of the exponent (1/2 in this case) is calculated by multiplying the outer function's derivative by the inner function's derivative.
The derivative of e^(1/2x) is e^(1/2x) multiplied by (1/2)x^(-1/2).
The process of finding derivatives and integrals of exponential functions involves applying specific rules and properties.
The simplicity of the derivative of the exponential function makes it a fundamental concept in calculus.
Understanding the properties of exponential functions is crucial for solving more complex calculus problems.
The course aims to demystify the calculus of exponential functions and empower students with the necessary tools.
The exponential function's unique properties are emphasized as key takeaways for students to remember.
Transcripts
Browse More Related Video

Solving for the constant in the general anti-derivative
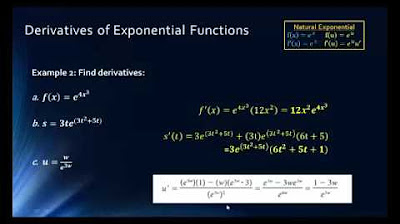
Math1325 Lecture 11 2 - Derivatives of Exponential
2.2 - Derivatives of Exponential Functions

Calculus - The basic rules for derivatives

Derivatives of Integrals (w/ Chain Rule)

Proof: d/dx(e^x) = e^x | Taking derivatives | Differential Calculus | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: