_-substitution: rational function | AP Calculus AB | Khan Academy

Khan Academy
28 Dec 201204:04
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the process of solving the indefinite integral of 4x^3 over x^4 plus 7 dx using u-substitution. It highlights the importance of recognizing the relationship between the integrand and its derivative, leading to setting u as x^4 plus 7. The script then explains the substitution process, where du equals 4x^3 dx, and the integral is rewritten as the natural log of the absolute value of u, with the final result being the natural log of the absolute value of x^4 plus 7, plus a constant C.

Takeaways
  • 📚 The problem involves taking the indefinite integral of a function involving x^4 + 7.
  • 🤔 The challenge is to recognize the relationship between the given expression and its derivative, 4x^3.
  • 👉 U-substitution is identified as the appropriate method to tackle this integral.
  • 🎯 The key is to set u equal to the expression whose derivative is readily available, which in this case is x^4 + 7.
  • 🔄 By setting u = x^4 + 7, we find that du = 4x^3 dx, which simplifies the original integral.
  • 📈 The rewritten integral is now ∫(4x^3 dx) / (x^4 + 7), which simplifies to ∫du/u.
  • 📝 The integral of 1/u du is the natural logarithm of the absolute value of u, plus a constant.
  • 🌟 The natural logarithm is used to handle the integration of a function that is the reciprocal of another function.
  • 🔙 Un-substituting u back into the integral gives us the final result in terms of x.
  • 🎓 The final answer is the natural logarithm of the absolute value of (x^4 + 7), plus a constant (denoted as C).
  • 📊 This process demonstrates the power of u-substitution in simplifying complex integrals and the importance of recognizing when to apply it.
Q & A

  • What is the integral being discussed in the transcript?

    -The integral being discussed is the indefinite integral of 4x^3 over x^4 plus 7 dx.

  • Why is u-substitution suggested as the tool of choice for this integral?

    -U-substitution is suggested because the expression x^4 + 7 and its derivative 4x^3 are both present in the integral, which provides a clear indication that substituting u will simplify the calculation.

  • What is the value of u in the context of this integral?

    -In this context, u is set to be equal to x^4 + 7, as it represents the expression whose derivative is readily available.

  • What is the value of du in terms of x?

    -du is equal to the derivative of u with respect to x, which is 4x^3 dx, since the derivative of x^4 is 4x^3 and the derivative of 7 is 0.

  • How can the integral be rewritten using u and du?

    -The integral can be rewritten as the indefinite integral of 1 over u du, since we have established that du is 4x^3 dx and u is x^4 + 7.

  • What is the result of the integral after applying u-substitution?

    -After applying u-substitution, the result of the integral is the natural log of the absolute value of u, plus a constant C.

  • How can the result in terms of u be converted back to x?

    -By substituting back u with x^4 + 7, the result becomes the natural log of the absolute value of x^4 + 7, plus the constant C.

  • Why is the absolute value used in the result of the integral?

    -The absolute value is used to ensure that the result is defined for all values of u, including negative values, even though in this specific case it's not necessary as u represents an expression that is always positive when x is real.

  • What is the significance of the constant C in the result of the indefinite integral?

    -The constant C represents the arbitrary constant that is always present in the result of an indefinite integral, indicating that there are infinitely many antiderivatives that differ by a constant.

  • How does this method help in solving more complex integrals?

    -This method demonstrates the use of u-substitution, a powerful technique that can simplify the process of integrating complex functions by transforming them into more manageable forms, making the calculation of the integral easier.

  • What is the final result of the integral after substituting back x for u?

    -The final result of the integral is the natural log of the absolute value of (x^4 + 7) plus a constant C.

Outlines
00:00
📚 Introduction to Indefinite Integral

This paragraph introduces the concept of taking the indefinite integral of a given function, specifically 4x^3/x^4 + 7 dx. The speaker acknowledges the complexity of the integral and suggests that the key to solving it lies in recognizing the expression and its derivative. The paragraph emphasizes the importance of identifying the derivative of the given expression (4x^3) and considering u-substitution as a potential solution. The speaker prompts the audience to think about what 'u' should be set to and outlines the steps to follow in order to perform the u-substitution.

Mindmap
Keywords
💡indefinite integral
The indefinite integral is a fundamental concept in calculus that represents the reverse process of differentiation. It is used to find the original function whose derivative is given. In the context of the video, the indefinite integral of the expression 4x^3 over x^4 plus 7 dx is the main goal, which is tackled using u-substitution.
💡expression
In mathematics, an expression is a combination of numbers, variables, and operations (such as addition, subtraction, multiplication, and division). In the video, the expression x^4 + 7 is key to solving the integral, as it is both the function to be integrated and its derivative is present in the integrand.
💡derivative
The derivative is a mathematical concept that gives the rate of change of a function with respect to its variable. In the video, the derivative of x^4 plus 7 is 4x^3, which is crucial for identifying the u-substitution method to solve the integral.
💡u-substitution
U-substitution is a technique used in calculus to evaluate integrals by substituting a variable that simplifies the integrand. In this video, setting u equal to x^4 plus 7 allows the integral to be rewritten in terms of du, making the problem easier to solve.
💡differential form
Differential form refers to the representation of a function and its derivative as an equation involving differentials (dx, du, etc.). In the video, the expression is converted into differential form (du = 4x^3 dx) to facilitate the u-substitution process.
💡natural log
The natural log, or logarithm with base e, is a mathematical function that calculates the power to which e must be raised to obtain a given value. In the video, after the u-substitution, the integral simplifies to the natural log of the absolute value of u, which is then evaluated.
💡absolute value
The absolute value of a number is its distance from zero on the number line, regardless of direction. In the video, the absolute value is used in the natural log function to ensure that the result is defined for all values of u, including negative ones.
💡constant of integration
The constant of integration, often denoted by C, is an additional term that appears when solving indefinite integrals. It accounts for the fact that the derivative of a constant is zero, and thus there can be multiple antiderivative functions. In the video, the constant C is mentioned as part of the final answer after un-substituting u back into the integral.
💡manipulate
In the context of the video, manipulate refers to the process of rearranging or transforming mathematical expressions to simplify them or to solve for certain variables. This is particularly relevant when converting between differential forms and equivalent expressions involving dx.
💡equivalent statements
Equivalent statements in mathematics are expressions or equations that convey the same information or relationship, even if they are written differently. In the video, the equivalence between du = 4x^3 dx and du/dX = 4x^3 is emphasized to facilitate the u-substitution process.
Highlights

The problem involves taking the indefinite integral of a function involving x^4 and a constant.

The expression x^4 + 7 and its derivative 4x^3 are key insights for integration.

u-substitution is identified as a potential method to solve the integral.

The choice for u is the inside expression of the integral, x^4 + 7.

The derivative of u with respect to x is calculated as 4x^3.

The integral can be rewritten in terms of u and du for easier computation.

The integral in terms of u simplifies to 1/u du.

The indefinite integral of 1/u du is the natural log of the absolute value of u.

The absolute value is used to ensure the solution is defined for negative values of u.

The integration constant C is mentioned as part of the final answer.

The process of u-substitution is explained in a step-by-step manner.

The integral is transformed back to the original variable x after substitution.

The final answer involves the natural log of x^4 + 7, demonstrating the power of substitution.

The video promises a follow-up to confirm the validity of the solution.

The method can be applied to a variety of similar integral problems.

The explanation is clear and methodical, making it accessible for learners.

Transcripts

Browse More Related Video

_-substitution: logarithmic function | AP Calculus AB | Khan Academy

(2^ln x)/x Antiderivative Example

_-substitution: multiplying by a constant | AP Calculus AB | Khan Academy

_-substitution: definite integrals | AP Calculus AB | Khan Academy

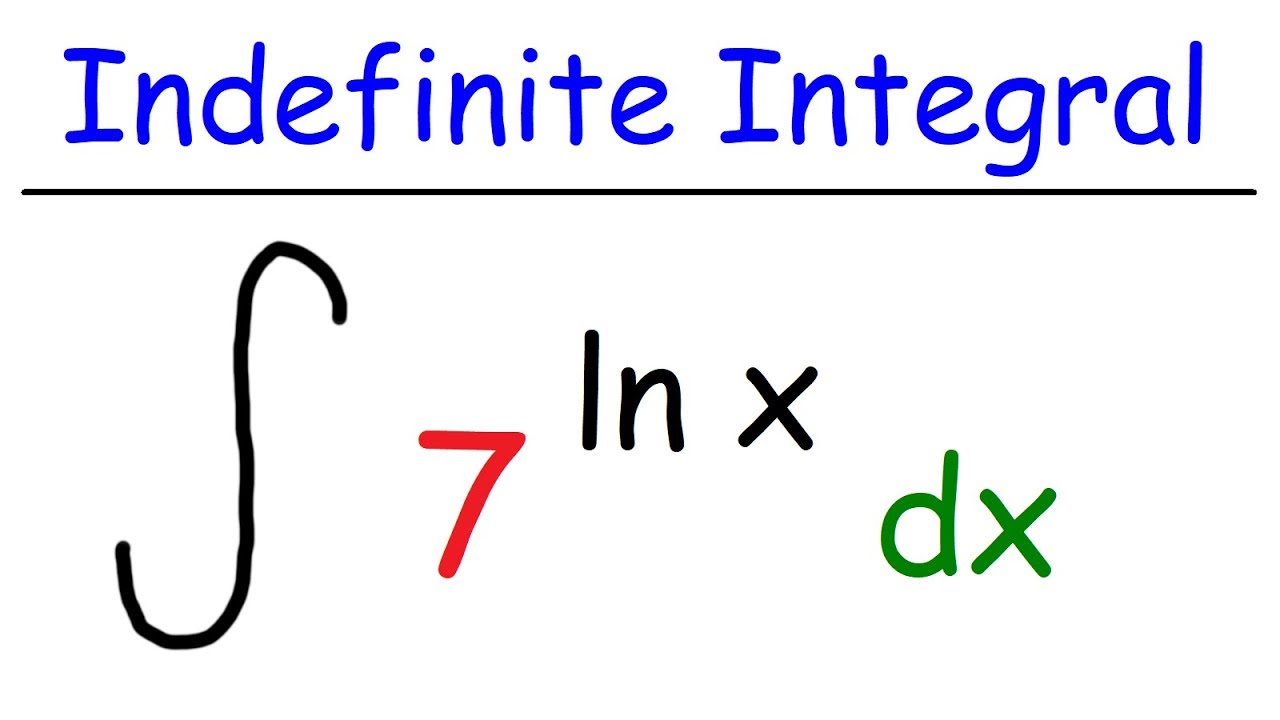
Indefinite Integral of 7^lnx

Definite integral involving natural log | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsCalculusIntegrationu-SubstitutionDifferential EquationsProblem SolvingEducational ContentMath TutorialIntegral TechniquesMathematics Education