Indefinite integrals of sin(x), cos(x), and e_ | AP Calculus AB | Khan Academy

Khan Academy
25 Jan 201304:04
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the concept of antiderivatives, emphasizing that they are not limited to functions of x but can apply to any variable. It explains the process of finding antiderivatives for sine and cosine functions, using the relationship between their derivatives. The script then corrects a mistake regarding the variable of integration and proceeds to find the antiderivatives of e to the power of a and 1/a, highlighting the importance of including a constant factor in the final answer.

Takeaways
  • 📚 The script focuses on the concept of taking antiderivatives of basic functions.
  • 🔄 It emphasizes that antiderivatives can be taken with respect to different variables, not just 'x'.
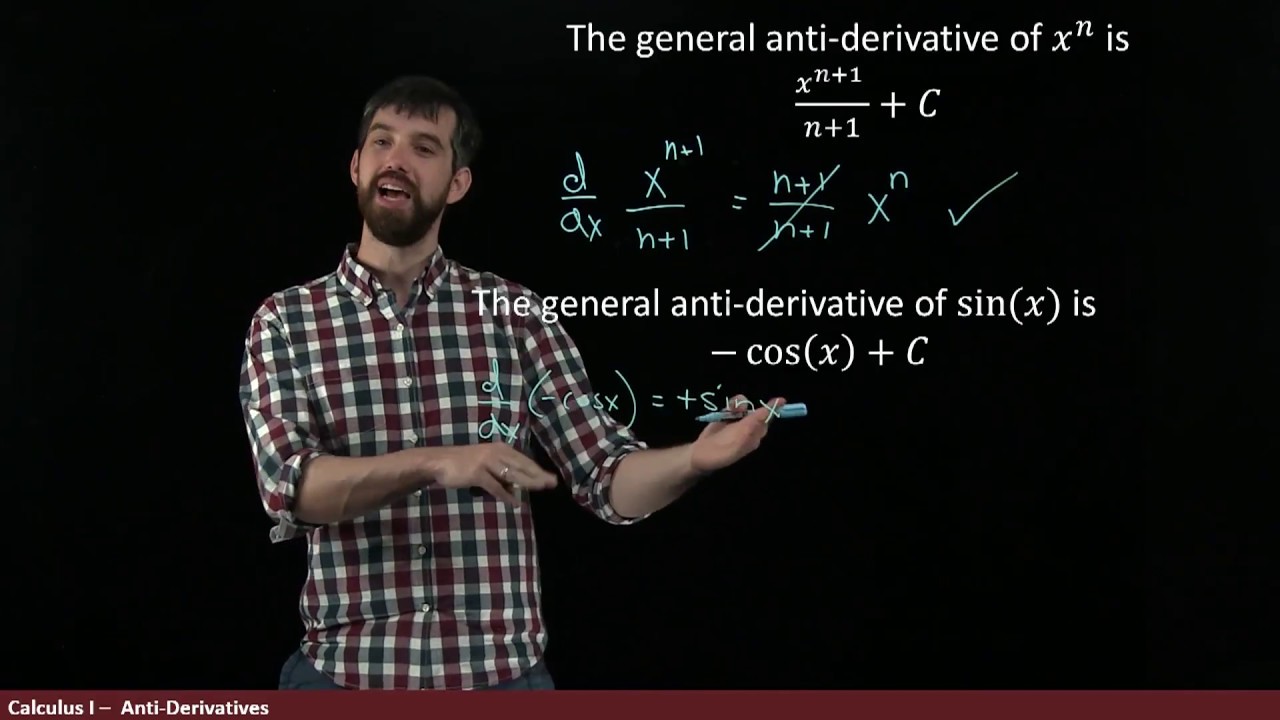
  • 🌀 The antiderivative of sine (sin) is negative cosine (-cos), and vice versa, the antiderivative of cosine is sine.
  • ∫ The indefinite integral of a function is the antiderivative, and it is represented with a 'plus constant'.
  • 📐 When dealing with integrals, the variable of integration is treated as a constant with respect to the functions being integrated.
  • 💡 The antiderivative of an exponential function e^x is the same exponential function, e^x.
  • 📈 The antiderivative of 1/a with respect to 'a' is the natural logarithm of the absolute value of 'a'.
  • 🎓 The importance of remembering to include a constant when stating the antiderivative of a function.
  • 🔍 The process of finding antiderivatives involves understanding the relationship between derivatives and antiderivatives.
  • 📊 The script provides examples to illustrate the process of finding antiderivatives for different types of functions, including trigonometric and exponential functions.
Q & A

  • What is the main topic discussed in the transcript?

    -The main topic discussed in the transcript is the process of finding antiderivatives of various basic functions, including trigonometric and exponential functions, with respect to different variables.

  • Why does the speaker mention that it doesn't always have to be functions of x?

    -The speaker mentions this to clarify that antiderivatives can be found with respect to different variables, not just x. In the given example, the function is with respect to t, highlighting the versatility in integration.

  • What is the antiderivative of sine of t?

    -The antiderivative of sine of t is negative cosine of t, which can be expressed as -cos(t).

  • How does the speaker arrive at the antiderivative of cosine of t?

    -The speaker uses the relationship between the derivative of cosine with respect to t (which is negative sine of t) to determine that the antiderivative of cosine of t is sine of t, expressed as sin(t).

  • What mistake does the speaker point out in the script?

    -The speaker points out that there should be 'da' instead of 'dt' in the expression for integration with respect to 'a', as 'dt' would imply integration with respect to 't'.

  • What is the antiderivative of e to the a?

    -The antiderivative of e to the a is e to the a, which can be written as e^a, since the derivative of e^x with respect to x is e^x, and this property extends to any variable 'a'.

  • What is the antiderivative of 1/a with respect to 'a'?

    -The antiderivative of 1/a with respect to 'a' is the natural logarithm of the absolute value of 'a', expressed as ln(|a|).

  • Why does the speaker emphasize the importance of including a constant in the antiderivative?

    -The speaker emphasizes including a constant because it's a fundamental part of the general form of an antiderivative. The constant accounts for the infinite number of possible antiderivatives due to the arbitrary constant of integration.

  • How does the speaker use the concept of derivatives to find antiderivatives?

    -The speaker uses the concept of derivatives by reversing the process. They find the derivative of the given function and then use the relationship between the derivative and the antiderivative to find the original function, which is the antiderivative.

  • What is the significance of the phrase 'most general antiderivative'?

    -The phrase 'most general antiderivative' refers to the fact that there are infinitely many antiderivatives for a given function, differing only by a constant. The 'most general' form includes this arbitrary constant to account for all possible antiderivatives.

Outlines
00:00
📚 Understanding Antiderivatives and Functions of Variables

The paragraph discusses the concept of taking antiderivatives of basic functions, emphasizing that it's not limited to functions of x but can also be functions of other variables like t. It clarifies the notation used in the process, highlighting the importance of using the correct variable for integration. The explanation proceeds with examples, focusing on the antiderivatives of sine and cosine functions, and how they relate to their derivatives. The paragraph also introduces the idea of indefinite integrals and how they relate to antiderivatives, using the context of definite integrals to illustrate the point.

Mindmap
Keywords
💡antiderivative
The antiderivative, also known as the indefinite integral, is a fundamental concept in calculus that represents the reverse process of differentiation. It is used to find the original function from which a given derivative can be obtained. In the video, the process of finding antiderivatives of various functions, such as sine and cosine, is discussed to reinforce understanding of basic calculus operations.
💡functions
Functions are mathematical relationships that describe how one variable changes in relation to another. In the context of the video, functions are the central objects of study, with a focus on how their derivatives and antiderivatives can be calculated. The video specifically mentions functions of 'x' and 't', emphasizing that the process of taking derivatives and antiderivatives applies to any variable.
💡sine and cosine
Sine and cosine are two of the six trigonometric functions, which are used to model periodic phenomena. In the video, the antiderivative of sine and cosine functions is discussed, highlighting the relationship between these functions and their derivatives. For instance, the derivative of cosine results in negative sine, and vice versa, which is key to finding their antiderivatives.
💡definite integrals
Definite integrals are used in calculus to calculate the area under a curve within a specified interval. The video script mentions that the notation for antiderivatives changes when dealing with definite integrals, which is an important distinction for understanding more advanced calculus concepts.
💡notation
Notation in mathematics refers to the symbols and expressions used to write mathematical concepts in a standardized way. The video emphasizes the importance of correct notation when working with antiderivatives, such as using 'dx' instead of 'da', which is crucial for accurate mathematical communication.
💡exponential functions
Exponential functions are mathematical functions of the form f(x) = a^x, where 'a' is a constant. In the video, the antiderivative of an exponential function with respect to 'a' is discussed, noting that the antiderivative of e^x (where e is the base of the natural logarithm) is e^x itself, which is a unique property of exponential functions.
💡constants
Constants are values that do not change. In the context of the video, it is mentioned that when finding antiderivatives, one must always include a constant, as the derivative of a constant is zero, and thus the antiderivative must account for this.
💡natural logarithm
The natural logarithm is the logarithm to the base e, where e is a mathematical constant approximately equal to 2.71828. In the video, it is mentioned that the antiderivative of 1/a is the natural logarithm of the absolute value of 'a', which is a key result in calculus involving logarithmic functions.
💡derivative
A derivative is a concept in calculus that measures how a function changes as its input changes. The video script discusses the relationship between derivatives and antiderivatives, emphasizing that understanding derivatives is crucial for finding antiderivatives of functions.
💡variable
In mathematics, a variable is a symbol that represents a value that can change. The video script uses variables like 'x' and 't' to illustrate how functions and their derivatives and antiderivatives can be expressed, highlighting the importance of variables in understanding calculus concepts.
Highlights

The session focuses on taking antiderivatives of basic functions to build comfort with the process.

It's clarified that antiderivatives can be taken with respect to variables other than x, such as t.

The antiderivative of sine and cosine functions are discussed, emphasizing the relationship between their derivatives.

The derivative of cosine with respect to t is shown to be equal to negative sine of t.

The antiderivative of sine of t is identified as negative cosine of t.

The antiderivative of cosine of t is explained as sine of t, highlighting the inverse relationship between derivatives and antiderivatives.

An error in the example is corrected, shifting the focus to the correct variable a, instead of t.

The indefinite integral of e to the power of a is explored, emphasizing the unique properties of exponential functions.

The antiderivative of e to the a is shown to be e to the a, plus a constant, due to the nature of exponential functions.

The natural log of the absolute value of a is identified as the antiderivative of 1/a.

The importance of remembering to include a constant when finding antiderivatives is emphasized.

The process of taking antiderivatives is shown to be systematic and builds on the understanding of derivatives.

The session aims to make the concept of antiderivatives clear and accessible through examples and explanations.

The relationship between derivatives and antiderivatives is a central theme, with examples illustrating this connection.

The session provides a comprehensive overview of taking antiderivatives of trigonometric and exponential functions.

The practical application of antiderivatives in solving definite integrals is alluded to, setting the stage for further exploration.

The session concludes with the successful identification of antiderivatives for the given expressions, reinforcing the learned concepts.

Transcripts

Browse More Related Video

Calculus AB/BC – 7.2 Verifying Solutions for Differential Equations

What's an anti-derivative?

Antiderivatives!

BusCalc 13.2 Integration by Substitution

Evaluating Indefinite Integrals

Double Integrals

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusAntiderivativesIntegralsTrigonometricFunctionsExponentialsMathematicsEducationalTutorialMath