_-substitution: defining _ (more examples) | AP Calculus AB | Khan Academy

Khan Academy
8 Sept 201704:34
EducationalLearning
32 Likes 10 Comments

TLDRThe video script focuses on the application of u-substitution in solving integrals, specifically highlighting the process of identifying suitable functions and their derivatives for substitution. It demonstrates the technique with two examples: the integral of the natural log of x to the 10th power and the integral of tangent of x. The script illustrates how to transform the integrals into a form where u-substitution can be applied, simplifies the expressions, and emphasizes the importance of back-substitution to find the final result.

Takeaways
  • 📚 The video focuses on practicing the method of u-substitution for solving integrals.
  • 🔍 U-substitution is applicable when there's a function and its derivative involved in the integral.
  • 🌟 Recognizing the derivative of the function inside the integral is crucial for choosing the right u.
  • 📈 An example given is the integral of natural log of x to the 10th power over x, where u is set to the natural log of x.
  • 🛠 The derivative of the chosen u (in the first example, natural log of x) is used to express du/dx.
  • 🧩 The integral simplifies to u to the power of 10 times du after applying u-substitution.
  • 🔄 Back-substitution is used to evaluate the indefinite integral by replacing u with its original function.
  • 📊 Another example is the integral of tangent of x, which is rewritten in terms of sine and cosine to apply u-substitution.
  • 👉 The strategy involves multiplying by negative one to align the derivative with the form required for u-substitution.
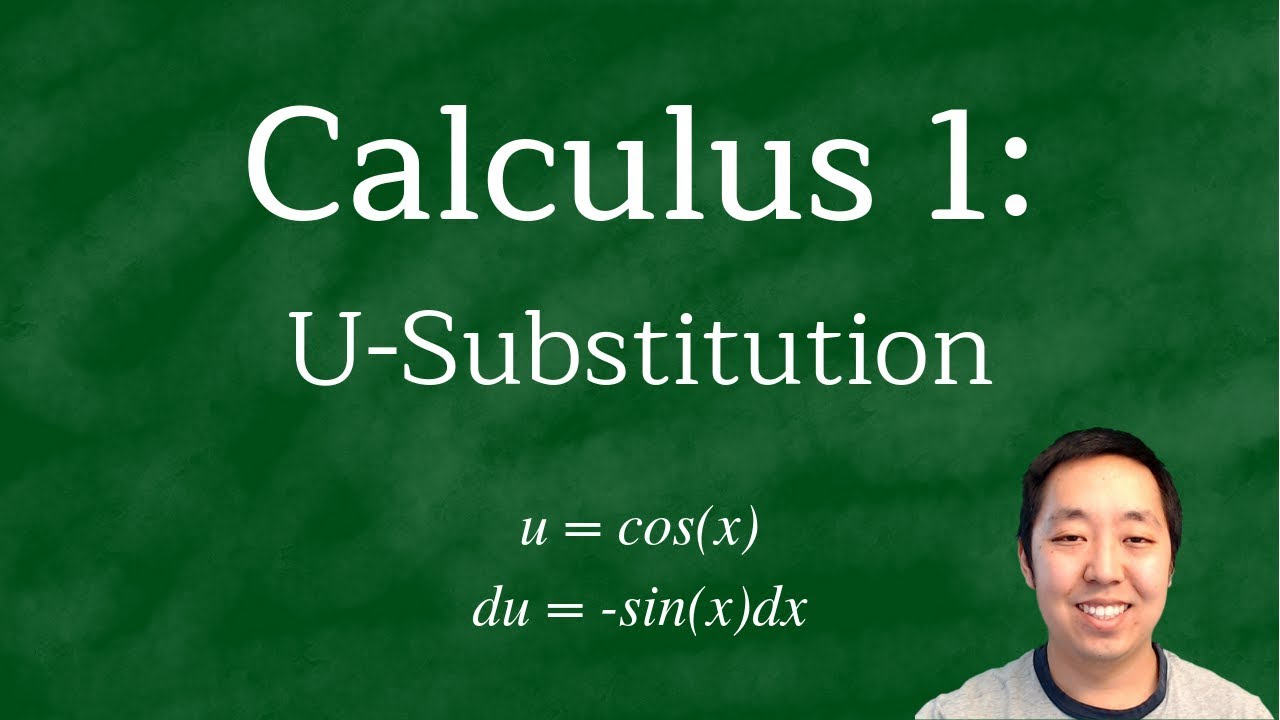
  • 📱 In the second example, u is set to cosine of x, and du/dx is negative sine of x, simplifying the integral.
  • 🎯 The final step is to evaluate the simpler integral and then perform back-substitution to find the original integral's value.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is practicing the method of u-substitution in integration.

  • What is the first integral example given in the video?

    -The first integral example is the indefinite integral of the natural log of x to the 10th power over x dx.

  • How is u-substitution applied in the first example?

    -In the first example, u-substitution is applied by recognizing that the natural log of x to the 10th power has a derivative of one over x, leading to the substitution u = natural log of x and du = 1/x dx.

  • What is the significance of identifying the derivative in u-substitution?

    -Identifying the derivative is crucial in u-substitution because it allows us to express the integrand in terms of u and du, simplifying the integral.

  • What is the second integral example discussed in the video?

    -The second integral example is the integral of tangent of x dx.

  • How is the tangent function rewritten to apply u-substitution in the second example?

    -The tangent function is rewritten in terms of sine and cosine as the integral of sine of x over cosine of x dx.

  • What is the key insight for choosing u in the second example?

    -The key insight is recognizing that the derivative of cosine of x is negative sine of x, which leads to the substitution u = cosine of x and du = -sine of x dx.

  • How does the process of u-substitution simplify the integral of tangent of x dx?

    -By substituting u with cosine of x and du with -sine of x dx, the integral simplifies to the negative indefinite integral of one over u, making it easier to evaluate.

  • What is the final step in u-substitution after evaluating the integral?

    -The final step is back-substitution, where the original variable (in this case, x) replaces the u variable to find the antiderivative.

  • Why is u-substitution a useful technique in integration?

    -U-substitution is a useful technique because it can transform complicated integrands into simpler forms that are easier to integrate, thus facilitating the solution of integrals.

  • What is the role of the derivative in the selection of u in u-substitution?

    -The derivative plays a critical role in the selection of u because u is typically chosen to be a function whose derivative is present in the integrand, allowing for simplification through substitution.

Outlines
00:00
📚 Introduction to U-Substitution

This paragraph introduces the concept of u-substitution, a technique used in calculus for solving integrals. The instructor explains the conditions under which u-substitution can be applied, which is when there is a function and its derivative present in the integral. The example given is the indefinite integral of the natural log of x to the 10th power over x. The instructor demonstrates how to identify the function (natural log of x) and its derivative (1/x), and then proceeds to make the substitution where u is set as the natural log of x and du/dx equals 1/x. The integral is then simplified to u to the 10th power times du, which is easier to evaluate. The process of back-substitution is also mentioned, where the original variable (x) is substituted back in for u to solve the integral.

Mindmap
Keywords
💡u-substitution
u-substitution is a method used in calculus to evaluate integrals by transforming them into a simpler form. It is applicable when the integrand can be expressed as a function of a new variable, u, and its derivative. In the video, the instructor demonstrates u-substitution by identifying the function and its derivative in the integral, such as using u = natural log of x for the integral of natural log of x to the 10th power over x dx.
💡indefinite integral
An indefinite integral represents a family of functions that differ by a constant, known as the constant of integration. It is the reverse process of differentiation and is used to find the original function whose derivative is given. In the context of the video, the instructor aims to find the indefinite integral of various functions using u-substitution.
💡natural log
The natural log, or logarithm with base e, is a fundamental mathematical function that represents the power to which e must be raised to obtain a given value. It is denoted as ln(x) and is used in various fields such as mathematics, physics, and engineering. In the video, the natural log is used in the integral of ln(x)^10 over x dx, where the instructor applies u-substitution with u = ln(x).
💡derivative
A derivative represents the rate of change of a function with respect to its independent variable. It is a fundamental concept in calculus and is used to analyze the behavior of functions, such as their slope, critical points, and maxima/minima. In the video, the derivative is crucial for identifying when to apply u-substitution, as it helps to find the function whose derivative is present in the integrand.
💡tangent
The tangent function, often abbreviated as tan, is a trigonometric function that relates the angles of a right triangle to the ratios of its opposite and adjacent sides. It is used in various applications, including geometry, physics, and engineering. In the video, the tangent function is discussed in the context of integrals and is rewritten in terms of sine and cosine to apply u-substitution.
💡sine and cosine
Sine and cosine are two of the six trigonometric functions that relate the angles of a right triangle to the ratios of its sides. They are widely used in mathematics, physics, engineering, and other fields. In the video, sine and cosine are used to rewrite the tangent function and to find a suitable u for u-substitution in the integral of tan(x) dx.
💡integration by parts
Integration by parts is a technique used in calculus to evaluate integrals of products of functions. It is based on the product rule for differentiation and is used when u-substitution is not immediately applicable. Although not explicitly mentioned in the video, the process of rewriting the tangent function in terms of sine and cosine could be seen as a form of integration by parts.
💡antiderivative
An antiderivative is a function whose derivative is equal to the given function being integrated. It represents a family of functions that differ by a constant. In the video, finding the antiderivative is the goal of the u-substitution process, as it allows the evaluation of the indefinite integral.
💡back-substitution
Back-substitution is the process of replacing the u variable in the result of the u-substitution with the original function used to define u. This step is necessary to obtain the final answer to the integral in terms of the original variable. In the video, the instructor emphasizes the importance of back-substitution after finding the antiderivative using u-substitution.
💡constant of integration
The constant of integration is an arbitrary constant, usually denoted as C, that is added to the antiderivative to account for the fact that there can be infinitely many functions with the same derivative. It is a fundamental concept in calculus and is present in the context of indefinite integrals. In the video, the constant of integration would be included in the final answer of the integrals discussed.
💡integration properties
Integration properties are a set of rules that help simplify the process of integration. They include properties such as linearity, which states that the integral of a sum is equal to the sum of the integrals, and properties related to constants and derivatives. In the video, the instructor uses integration properties to manipulate the integral before applying u-substitution, such as multiplying by -1 to match the derivative with the integrand.
Highlights

The video discusses the application of u-substitution in integration.

The indefinite integral of natural log of x to the 10th power over x is used as an example.

The derivative of natural log of x is identified as one over x.

The integral is rewritten to clearly show the function and its derivative.

The substitution u equals natural log of x is suggested based on the derivative.

The du dx relationship is established as one over x.

The integral simplifies to u to the 10th power times u to the 10th power du.

Back-substitution is mentioned as a method to evaluate the indefinite integral.

The integral of tangent of x is discussed as another example.

Tangent of x is rewritten in terms of sine and cosine.

The derivative of cosine of x is identified as negative sine of x.

A method for engineering the integral by multiplying by negative one is introduced.

The substitution u equals cosine of x is proposed based on the derivative.

The du relationship is established as negative sine of x dx.

The integral simplifies to the negative indefinite integral of one over u.

The process of back-substitution is mentioned for the final evaluation of the integral.

The video provides a clear and methodical approach to using u-substitution in integration.

Transcripts

Browse More Related Video

_-substitution: definite integrals | AP Calculus AB | Khan Academy

Back Substitution - When a u-sub doesn't match cleanly!

Calculus 1: U-Substitution Examples

Definite Integrals from Graphs (Part 2 of 3) - U-Substitution

Lesson 11 - Integration By Substitution (Calculus 1 Tutor)

Integration Using The Substitution Rule

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus TechniquesIntegral Methodsu-SubstitutionNatural LogarithmTrigonometric IntegralsMathematics EducationAdvanced MathOnline TutorialEducational ContentMath Problem Solving