INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 11

TIKLE'S ACADEMY OF MATHS
20 Jun 202019:05
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the concept of integral calculus, focusing on solving a specific problem involving a function. It emphasizes the importance of understanding the definition of integrals and functions, and the process of converting given integrals into a form that can be easily solved. The video also highlights the need to check for errors and ensure that the solution matches the definition of the integral. The่ฎฒๅธˆ encourages viewers to practice and review previous videos for a better grasp of the subject.

Takeaways
  • ๐Ÿ“š The video is a continuation of a mathematics lecture series focusing on integral calculus, specifically delving into the unit on integral calculus and its applications.
  • ๐Ÿ”ข The lecture introduces a problem-solving approach within the context of integral calculus, emphasizing the importance of understanding the definition of integral functions and their applications.
  • ๐Ÿ“ˆ The script discusses the concept of converting given integrals into a specific form, which involves shifting from the question's standpoint to the integral's standpoint, and applying the definition of the integral function to find its value.
  • ๐Ÿงฎ The process of solving integrals involves converting the given integral into a form that can be easily integrated, which may require special manipulations and understanding of logarithmic and exponential functions.
  • ๐Ÿ”„ The script highlights the importance of checking each step when solving problems, as even small mistakes can lead to incorrect results, emphasizing the need for precision in mathematical calculations.
  • ๐ŸŒ The video also touches on the concept of limits in integral calculus, explaining how to handle and change limits when solving integrals, which is crucial for obtaining accurate results.
  • ๐Ÿ“Š The lecture provides a detailed explanation of the formula for the integral function, including the concept of factorial and how it applies to the calculation of integrals.
  • ๐ŸŽ“ The script underscores the educational value of the video, encouraging viewers to review previous videos in the series for a comprehensive understanding of the topic.
  • ๐Ÿค The video encourages interaction, inviting viewers to like, share, and comment on the video if they found it helpful or have any questions, and to subscribe for future content.
  • ๐Ÿ”‘ The key to solving the problem presented in the video lies in the application of the definition of integral functions and the manipulation of mathematical expressions to reach the solution.
  • ๐Ÿš€ The video concludes with a reminder of the importance of practice and continuous learning, promising to cover more nuanced topics in upcoming videos to enhance the viewers' understanding of integral calculus.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is to solve a problem related to the integral calculus unit and to advance in the album collection of integral calculus.

  • What does the video mention about previous problems and topics?

    -The video mentions that many problems and other top mathematical topics were covered in previous sessions, and all the problems and topics can be accessed through the description of the video.

  • What is the warning call about in the context of integral calculus?

    -The warning call is about the knowledge of integral calculus and the function, emphasizing the importance of understanding the integral calculus unit and its application in problem-solving.

  • How does the video describe the process of solving the given problem?

    -The video describes the process of solving the problem by converting the given integral into a specific form, applying the definition of the integral function, and calculating its value using the techniques used in previous processes.

  • What is the significance of the integral's definition in solving the problem?

    -The integral's definition is significant as it provides the formula to convert the given integral into a form that can be solved, allowing the calculation of its value.

  • How does the video handle the concept of limits in integral calculus?

    -The video discusses the concept of limits by showing how to convert the given integral into a form that includes the lower and upper limits, and then how to adjust these limits according to the properties of the integral function.

  • What is the role of the special 'l' in the integral calculus as mentioned in the video?

    -The special 'l' in the integral calculus, as mentioned in the video, seems to be a placeholder or a variable used in the process of converting and solving the integral. The exact role is not clearly defined in the provided transcript.

  • How does the video address the concept of factorization in the context of the problem?

    -The video suggests that factorization is a key step in solving the problem, as it involves breaking down complex expressions into simpler ones, which can then be integrated or calculated more easily.

  • What is the final result of the integral problem solved in the video?

    -The final result of the integral problem is not explicitly mentioned in the transcript, but it involves a detailed process of converting the integral, applying definitions, and calculating values while considering the limits and other mathematical properties.

  • What advice does the video give for students studying integral calculus?

    -The video advises students to thoroughly understand the concepts and definitions of integral calculus, to practice solving problems using these concepts, and to review previous videos for a comprehensive understanding of the subject.

  • How does the video conclude?

    -The video concludes by summarizing the problem-solving process, emphasizing the importance of understanding integral calculus, and encouraging students to continue learning and practicing to improve their skills.

Outlines
00:00
๐Ÿ“˜ Introduction to Integral Calculus

The paragraph introduces the viewer to a new topic in the realm of Integral Calculus. It emphasizes the importance of understanding the concept of integral calculus and its applications. The speaker mentions that they will be solving a problem related to a function called 'Capil' and discusses the relevance of previous lessons in the series. The paragraph sets the stage for a detailed exploration of integral calculus, including the definition and application of integral functions.

05:02
๐Ÿ”ข Working with Limits and Functions

This paragraph delves into the specifics of working with limits and functions within the context of integral calculus. The speaker explains the process of converting a given integral into a form that can be easily manipulated. They discuss the concept of limits, the definition of integral functions, and the importance of accurately performing calculations. The paragraph also touches on the technique of solving problems and the need to ensure that replacements are correctly made to avoid errors.

10:04
๐Ÿ“š Advanced Concepts and Problem Solving

The speaker continues with advanced concepts in integral calculus, focusing on solving complex problems. They discuss the manipulation of integral expressions, including the use of different expansions and the application of limits. The paragraph highlights the importance of understanding the properties of integration and the impact of changing limits on the final result. The speaker also emphasizes the need for precision and the potential for mistakes in the calculation process.

15:06
๐ŸŽ“ Finalizing the Calculation and Review

In the final paragraph, the speaker concludes the lecture by summarizing the integral calculus concepts discussed. They emphasize the importance of matching the calculated integral with the definition of the integral function. The speaker also provides guidance on how to approach complex problems, including the use of factorial functions and the handling of errors. The paragraph ends with a reminder to review previous lessons and an invitation to follow along with upcoming videos for further exploration of integral calculus.

Mindmap
Keywords
๐Ÿ’กIntegral Calculus
Integral Calculus is a branch of mathematics that deals with the study of integrals, which are used to find the area under a curve, the volume of solid shapes, and other similar quantities. In the context of the video, Integral Calculus is the main subject being discussed, with the speaker focusing on solving integral-related problems and explaining concepts such as antiderivatives and the fundamental theorem of calculus.
๐Ÿ’กFunctions
In mathematics, a function is a relation that pairs each element from a set (called the domain) with exactly one element of another set (known as the range). Functions are fundamental to the study of calculus, as they represent the input-output relationships and can be differentiated or integrated. In the video, the speaker is likely to discuss functions in the context of integral calculus, solving problems that involve finding antiderivatives and working with integrals of functions.
๐Ÿ’กLimits
Limits are a fundamental concept in calculus that describe the behavior of a function as the input (or sometimes the output) approaches a particular value. Limits are used to define continuity, derivatives, and integrals, and are essential for understanding the properties of functions and their graphs. In the video, the speaker might explain how to compute limits, particularly in the context of evaluating integrals.
๐Ÿ’กAntiderivatives
An antiderivative, also known as an indefinite integral, is a function whose derivative is a given function. In the context of the video, finding antiderivatives is a key part of solving integral calculus problems, as it allows for the calculation of definite integrals which can represent areas, volumes, and other quantities.
๐Ÿ’กPower Functions
Power functions are a class of functions that have the form f(x) = x^n, where n is a real number. These functions are important in calculus because they exhibit various behaviors depending on the exponent, and their derivatives and integrals can be easily computed. In the video, power functions might be discussed in the context of integration and differentiation exercises.
๐Ÿ’กIntegration
Integration is a fundamental process in calculus that involves finding the area under a curve or the accumulated quantity associated with a function over an interval. It is the inverse process of differentiation and is used to solve a wide range of problems in mathematics, physics, and engineering. The video likely focuses on the techniques and applications of integration within the context of integral calculus.
๐Ÿ’กDifferential
A differential is a measure of how a function changes as its input changes. In calculus, differentials are used to approximate the change in a function's value and to define derivatives. They are also used in integral calculus to find the exact values of integrals. The video might explain how to work with differentials when solving integral calculus problems.
๐Ÿ’กFactorial
The factorial of a non-negative integer n, denoted by n!, is the product of all positive integers less than or equal to n. Factorials are used in combinatorics, probability, and many other areas of mathematics. In the context of the video, factorials might be discussed when dealing with certain types of integrals or functions that involve counting orๆŽ’ๅˆ—/็ป„ๅˆ.
๐Ÿ’กLimits of Integration
The limits of integration are the boundaries that define the interval over which an integral is calculated. These limits are crucial in determining the definite integral, which gives the exact area under the curve of a function. In the video, the speaker might explain how to set and evaluate the limits of integration for specific problems.
๐Ÿ’กExponential Functions
Exponential functions are mathematical functions of the form f(x) = a^x, where a is a constant and x is the independent variable. These functions are important in many areas of mathematics and science, as they model growth and decay processes. In the context of the video, exponential functions might be discussed in relation to their integrals and the techniques used to evaluate them.
๐Ÿ’กPolynomial Functions
Polynomial functions are expressions that consist of variables and coefficients, involving only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. These functions are fundamental in algebra and calculus, and their integrals are typically studied in introductory calculus courses. The video likely includes polynomial functions as part of the integral calculus lessons.
Highlights

The video welcomes viewers to a mathematics YouTube channel, focusing on integral calculus.

The lesson will advance the understanding of integral calculus and solve a problem involving a young function.

Previous videos have covered many problems and topics in mathematics, including other top subjects.

All previous problems and topics are accessible via a link in the video description for easy viewing.

The video emphasizes the importance of understanding the concept of integral calculus and functions.

A warning call is made about knowledge and functions in the integral calculus unit.

The problem to be solved involves a well-defined integral in question form, showcasing a specific mathematical expression.

The technique used to solve the problem will be the same as previously used in all processes.

The function's second definition is used, which is rooted in the integrals of products to infinity minus a point.

The integral is converted into a form that can be easily solved, involving logarithms and exponents.

The process of converting the given integral into a solvable form is demonstrated step by step.

The video explains how to handle the integral's limit, emphasizing the importance of getting the limit right.

The integral's lower and upper limits are discussed, along with the implications of changing them.

The video provides a clear example of how to apply the definition of knowledge and functions to the integral calculus.

The solution to the integral problem is shown, involving the use of integral properties and limit changes.

The final solution of the integral is presented, demonstrating the application of integral calculus concepts.

The video concludes with a reminder to check the solution against the definition of integral calculus for accuracy.

The importance of practicing and reviewing previous videos is stressed for better understanding and application.

Transcripts

Browse More Related Video

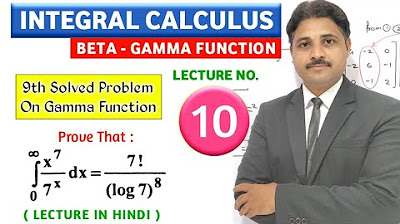
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 10

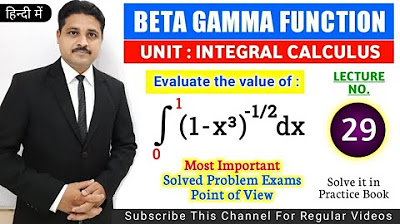
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 29 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 15

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 14

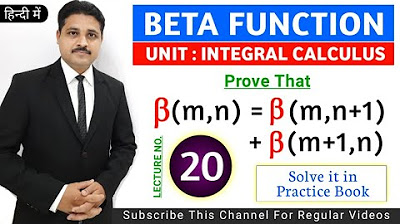
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 20 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

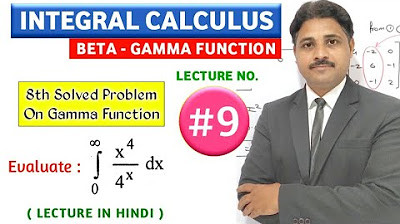
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 9

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
IntegralCalculusMathematicsLectureKapilSharmaAdvancedFunctionsEducationalContentProblemSolvingYouTubeChannelMathTutorialsSeriesCollectionAcademicLearning