Integration of Vector Functions (Calculus 3)
TLDRThis video script from Houston Math Prep delves into the integration of vector-valued functions, emphasizing the component-wise approach for anti-derivatives. It illustrates indefinite integrals with constants of integration for each component and suggests alternative notations for clarity. The script also covers definite integrals, showcasing examples with bounds, and discusses finding position vectors from given acceleration functions and initial conditions, guiding viewers through the integration process to solve for velocity and position functions.
Takeaways
- 📚 The process of integrating vector-valued functions is done component-wise, treating each component of the vector as a separate integral.
- 🔍 When integrating vector-valued functions without bounds, the result is a vector with constants of integration for each component.
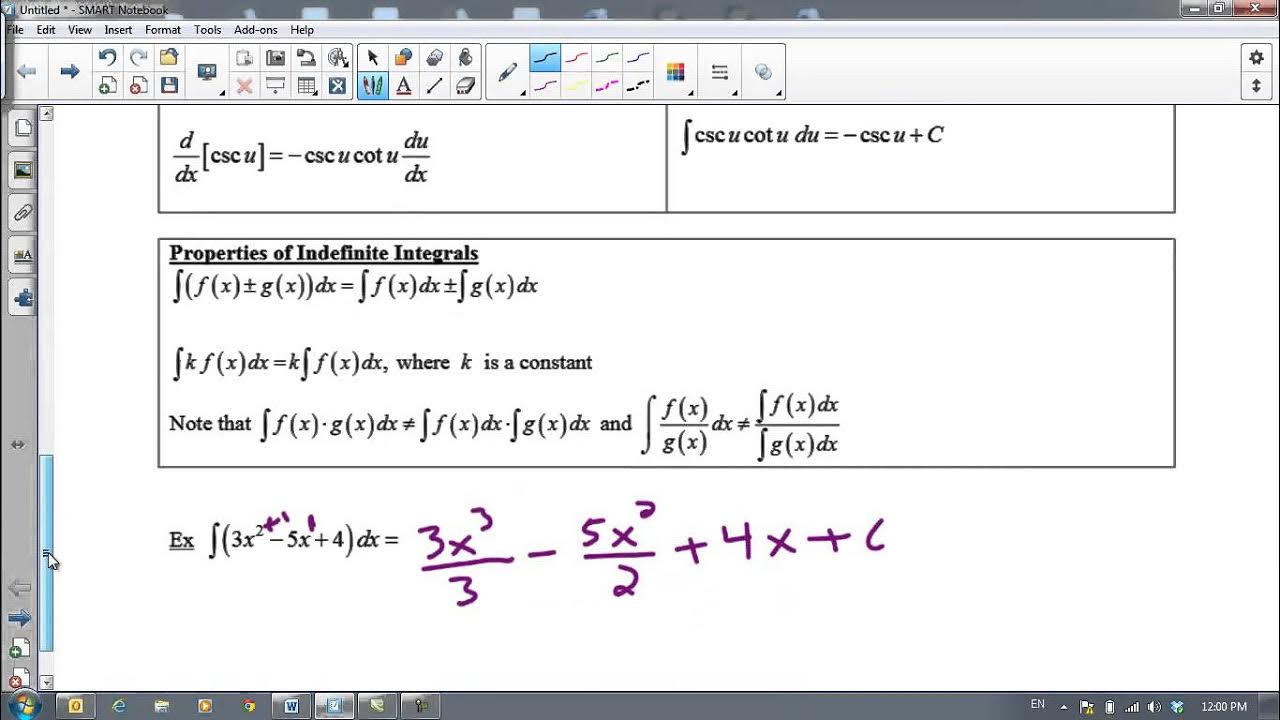
- 📝 An example given in the script demonstrates integrating a vector-valued function \( \langle 4t, t^3, e^{2t} \rangle \) and handling the constants of integration in different ways.
- 👉 The script suggests an alternative method of writing the solution by creating a vector of constants, simplifying the notation for the indefinite integral.
- 🧩 The importance of remembering integration rules for antiderivatives of functions like \( 1/t^2 + 1 \), \( 1/(t+1) \), and \( 1/\sqrt{t+1} \) is highlighted.
- 📉 The script covers the use of integration by parts for products and the application of substitution methods for integrals involving functions like \( t\cos(t) \).
- 📌 Definite integrals of vector-valued functions require evaluating the antiderivative at the bounds of integration and performing vector subtraction.
- 🚀 The script explains how to find the position vector given an acceleration function and initial conditions, involving double integration and solving for constants.
- 🔑 The process of solving for constants in the velocity and position functions by using initial conditions is demonstrated with a specific example.
- 📐 The final position function is derived by integrating the velocity function twice and applying the given initial conditions for both velocity and position at time zero.
Q & A
What is the process for integrating a vector-valued function?
-To integrate a vector-valued function, you integrate each component of the vector separately, treating them as individual functions. The result is also a vector, with each component being the integral of the corresponding part of the original function.
How are constants of integration handled in the indefinite integral of a vector-valued function?
-In the indefinite integral of a vector-valued function, a constant of integration is included for each component function that is being integrated, resulting in a vector where each component has its own constant.
Can you provide an example of integrating a vector-valued function with components 4t, t^3, and e^(2t)?
-The integral of the vector-valued function with components 4t, t^3, and e^(2t) would be 2t^2, (1/4)t^4, and (1/2)e^(2t) respectively, each with its own constant of integration.
What is an alternative way to express the constants of integration in the integral of a vector-valued function?
-An alternative way to express the constants of integration is to create a vector that contains all the constants by themselves, separate from the integrated components.
How can the integral of a vector-valued function be simplified in notation?
-The integral can be simplified by writing the integrated components followed by a shorthand notation indicating a vector of constants with as many components as there are in the original vector function.
What is the process for integrating a vector-valued function with components 1/t^2 + 1, 1/(t+1), and 1/√(t+1)?
-Each component is integrated using the appropriate rule: the first is an inverse tangent function, the second is a natural logarithm (with absolute value if considering negative t values), and the third is a power rule after a substitution, resulting in 2√(t+1).
How do you find the position vector given an acceleration function and initial conditions?
-First, integrate the acceleration function to find the velocity function, using the initial velocity condition to solve for the constants of integration. Then, integrate the velocity function to find the position function, using the initial position condition to solve for the remaining constants.
In the context of the script, what is the antiderivative of e^t with respect to t?
-The antiderivative of e^t with respect to t is simply e^t, as the derivative of e^t is itself.
What is the antiderivative of t+1 with respect to t, and how do you find the constants of integration for this component?
-The antiderivative of t+1 with respect to t is (1/2)t^2 + t. The constants of integration for this component are found by using the initial velocity condition at t=0.
How do you handle the definite integral of a vector-valued function with bounds?
-For a definite integral of a vector-valued function, integrate each component with respect to t, and then evaluate the result at the upper and lower bounds, subtracting the lower bound result from the upper bound result for each component.
Can you provide an example of evaluating a definite integral of a vector-valued function from 0 to π/2 of the components 2t, sin(t), and t*cos(t)?
-After integrating, the components become t^2, -cos(t), and (t*sin(t) + cos(t)). Evaluating from 0 to π/2 and subtracting, the result is (π^2/4, 1, π/2 - 1).
Outlines
📚 Introduction to Vector-Valued Function Integration
This paragraph introduces the concept of integrating vector-valued functions. The process involves integrating each component of the vector separately, using standard integration techniques such as the power rule. The result is a vector of anti-derivatives, which may include constants of integration for indefinite integrals. An example is provided, integrating the vector-valued function \( \langle 4t, t^3, e^{2t} \rangle \), resulting in a vector with components \( \langle 2t^2 + C_1, \frac{1}{4}t^4 + C_2, \frac{1}{2}e^{2t} + C_3 \rangle \). An alternative representation using a constant vector is also discussed.
🔍 Definite Integration of Vector-Valued Functions
The second paragraph delves into the process of computing definite integrals for vector-valued functions. It explains that after integrating each component, one must evaluate the result at the upper and lower bounds and subtract to obtain the final vector with constant components. An example integral from 0 to \( \frac{\pi}{2} \) of the function \( \langle 2t, \sin(t), t\cos(t) \rangle \) is worked out, demonstrating the use of integration techniques such as integration by parts and the application of bounds to find the definite integral result.
🚀 Finding Position Vector from Acceleration Function
This paragraph discusses a practical application of integration in physics: finding the position vector given an acceleration function and initial conditions. The process requires two integrations: first to find the velocity function from the acceleration, using the initial velocity condition to solve for constants, and then integrating again to find the position function. The initial position condition is used to solve for the remaining constants in the position function, resulting in a specific solution that satisfies both the acceleration function and the given conditions.
🧩 Solving for Position Function with Given Conditions
The final paragraph continues the process of finding the position function by integrating the velocity function twice. It emphasizes the importance of using given initial conditions to solve for constants in the general solution. The specific solution for the position function is presented, which incorporates the constants solved from the initial conditions, resulting in a detailed expression for the position vector as a function of time that satisfies the provided conditions.
Mindmap
Keywords
💡Integration
💡Vector-Valued Functions
💡Anti-Derivative
💡Constant of Integration
💡Power Rule
💡Indefinite Integral
💡Definite Integral
💡Acceleration Function
💡Velocity Function
💡Position Function
💡Integration by Parts
Highlights
Integration of vector-valued functions can be done component-wise, treating each component separately.
Indefinite integrals of vector-valued functions result in a constant of integration for each component function.
A basic example demonstrates the integration process of a vector-valued function and suggests alternative solution writing methods.
Differentiation between constants of integration for each component is necessary as they may not be the same.
An alternative method for handling constants involves creating a vector of constants.
Integration rules must be remembered for anti-derivatives of vector-valued functions.
Examples of anti-derivatives using inverse tangent and log rules are provided.
Integration by parts is a common technique for products in anti-derivatives.
Definite integrals of vector-valued functions involve plugging in bounds and subtracting results.
Position vectors can be found from given acceleration functions and initial conditions.
Velocity functions are derived from acceleration functions using integration.
Initial conditions are used to solve for constants in the velocity function.
Position functions are obtained by integrating the velocity function.
Constants in the position function are solved using initial position conditions.
The process of finding position functions from acceleration involves two integrations and condition application.
A specific example walks through the steps of finding a position function given an acceleration function and initial velocity and position.
The final position function is presented with all constants solved, satisfying the given conditions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: