The Geometric Meaning of Differential Equations // Slope Fields, Integral Curves & Isoclines
TLDRThis video script explores the geometric interpretation of differential equations, drawing parallels between analytic and geometric concepts. The explanation begins with the fundamental equation y' = f(x, y), highlighting its geometric meaning as a slope field. The video introduces the method of isoclines for plotting and the concept of integral curves, which represent solutions to the differential equations. The script effectively connects the dots between the abstract analytic form of a differential equation and its concrete geometric representation, emphasizing how initial conditions and solutions are visualized and understood in both domains.
Takeaways
- 📐 The geometric meaning of a differential equation is to represent the slope of a function at any given point (x, y).
- 🔄 Differential equations have both analytic and geometric interpretations, which run parallel to each other.
- 🌐 The concept of a slope field or direction field is introduced to visually represent the differential equation geometrically.
- 📈 In a slope field, line segments are drawn at each point to indicate the slope as given by the differential equation.
- 🏁 Isoclines are lines of constant slope derived from the differential equation, where f(x, y) equals a constant.
- 🖌️ The method of isoclines is a way to plot differential equations by drawing lines with the same slope as the right-hand side of the equation.
- 💻 Computers can be used to plot slope fields more accurately and efficiently than by hand.
- 🔵 Integral curves are the graphical representation of solutions to a differential equation, where the curve's slope matches the slope field at every point.
- 🎯 An initial condition in the context of differential equations specifies a particular point through which the integral curve must pass.
- 🧩 Solving an initial value problem (IVP) involves finding an integral curve that satisfies the differential equation and passes through the given initial condition.
- 🔗 The connection between the analytic and geometric viewpoints is established by the fact that the integral curve is tangent to the slope field at every point.
Q & A
What is the geometric meaning of the derivative in the context of a differential equation?
-The geometric meaning of the derivative in a differential equation is the slope of the function at any given point (x, y). It represents the rate of change of the dependent variable (y) with respect to the independent variable (x) at that particular point on the curve.
What is the relationship between the analytic and geometric concepts in differential equations?
-The analytic concepts involve solving the differential equation by finding a function that satisfies the equation, while the geometric concepts involve visualizing the equation as a slope field where each point on the plot has a corresponding slope given by the differential equation.
What is a slope field or direction field in the context of differential equations?
-A slope field, or direction field, is a visual representation of a differential equation where small line segments are drawn at each point to indicate the slope of the function at that point, according to the differential equation's formula.
How is the method of isoclines used to plot a differential equation?
-The method of isoclines involves setting the right-hand side of the differential equation to constants, which results in horizontal lines representing places where the slope is constant. These lines, called isoclines, help in plotting a slope field for the differential equation.
What is an integral curve in the context of differential equations?
-An integral curve is the graph of a function that represents a solution to the differential equation. It is a curve that is tangent to the slope field at every point, meaning that the slope of the curve at any given point matches the slope specified by the differential equation at that point.
How does an initial condition relate to the geometric and analytic viewpoints of a differential equation?
-Analytically, an initial condition specifies the value of y at a certain x value (x_0, y_0). Geometrically, it corresponds to a specific point on the slope field where the solution curve (integral curve) must pass through.
What does it mean for a solution to an initial value problem to be tangent to the slope field?
-For a solution to an initial value problem to be tangent to the slope field means that the integral curve, which represents the solution, has the same slope as the slope field at every point along the curve. This tangency ensures that the solution accurately follows the rate of change as described by the differential equation.
How can a computer tool be used to plot a slope field for a differential equation?
-A computer tool can be used to plot a slope field by inputting the differential equation and parameters. The tool calculates the slope at each point on the plot and draws the corresponding line segments to visually represent the slope field, often allowing for the display of integral curves and the movement of a point to illustrate how solutions are traced.
What happens when the differential equation's right-hand side does not depend on x?
-When the right-hand side of the differential equation does not depend on x, the slope field will have horizontal lines as isoclines, because the slope (y) is constant across all x values for a given y value.
What is the significance of the red dot in the computer-plotted slope field?
-The red dot in the computer-plotted slope field represents an initial condition. It signifies the starting point (x value, y value) for a solution curve, and the computer plots the integral curve that follows the slopes indicated by the slope field from that point.
How does the slope field help in understanding differential equations?
-The slope field provides a geometric interpretation of the differential equation, allowing us to visualize the slopes at various points on the plot. This helps in gaining insights into the behavior of solutions and how they evolve according to the differential equation's rates of change.
Outlines
📚 Introduction to Differential Equations and Geometric Interpretation
This paragraph introduces the geometric meaning of a differential equation, specifically focusing on the derivative of y with respect to x. It draws parallels between geometric and analytic concepts previously discussed in the differential equations playlist. The video aims to demonstrate how these concepts relate to each other, starting with the observation that the derivative represents the slope of the function y = f(x). The speaker uses the example of the exponential growth equation (y' = y) to illustrate how a solution can be found by plugging it back into the equation. The concept of a slope field or direction field is introduced, which visually represents the slopes at different points on the graph. The method of isoclines is explained as a way to plot differential equations geometrically, where isoclines are lines of constant slope as dictated by the differential equation.
🖥️ Visualizing Differential Equations with a Slope Field and Computer Plotting
In this paragraph, the speaker continues the discussion on slope fields and how they can be visualized using computer tools. The video demonstrates how to plot a slope field for the differential equation y' = y, and how to use a computer to generate a more precise version of the manual plot. The concept of an integral curve is introduced, which is a curve that follows the slopes indicated by the slope field. The speaker uses a red dot to represent an initial condition and shows how moving this dot around results in different integral curves, each tangent to the slope field at every point. The video also explores a more complex differential equation (y * x) and how it can be visualized using the slope field. The paragraph concludes with a review of the key concepts learned, emphasizing the connection between the analytic and geometric viewpoints in understanding differential equations and their solutions.
Mindmap
Keywords
💡Differential Equation
💡Derivative
💡Slope Field
💡Direction Field
💡Isocline
💡Integral Curve
💡Initial Condition
💡Analytic Solution
💡Geometric Interpretation
💡Method of Isoclines
💡Computer Tool
Highlights
The geometric meaning of a differential equation is explored, drawing parallels between geometric and analytic concepts.
The derivative's geometric interpretation as the slope of a function is fundamental in understanding differential equations.
The concept of a slope field or direction field is introduced to visually represent the slopes indicated by a differential equation.
The method of isoclines is described as a way to plot differential equations using lines of constant slope.
The term 'isocline' is defined as lines where the right-hand side of the differential equation is equal to a constant, representing constant slope.
A computer tool is used to plot a better version of the slope field, allowing for more precision and visual clarity.
The concept of an integral curve is introduced, which is a curve that aligns with the slopes of the slope field at every point.
An initial condition is represented by a specific point on the slope field, guiding where solutions should pass through.
A solution to a differential equation, analytically, is a function that satisfies the equation, while geometrically, it is represented by an integral curve.
The integral curve is tangent to the slope field at every point, indicating that the slopes of the curve match the slopes prescribed by the differential equation.
An initial value problem in differential equations is solved by finding an integral curve that passes through the specified initial condition point.
The video connects the geometric and analytic perspectives, showing that they are parallel and complementary ways to understand differential equations.
The video provides a comprehensive overview of the geometric interpretation of first-order differential equations and their solutions.
The use of computer tools for visualizing mathematical concepts is demonstrated, enhancing understanding through visual representation.
The video concludes by emphasizing the value of understanding both the geometric and analytic sides of differential equations for a complete comprehension.
Transcripts
Browse More Related Video

Lec 1 | MIT 18.03 Differential Equations, Spring 2006
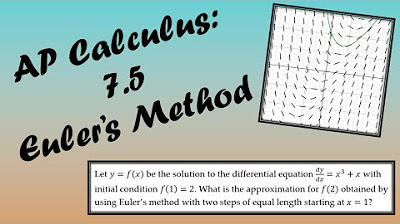
AP Calculus BC Lesson 7.5

Creating a slope field | First order differential equations | Khan Academy
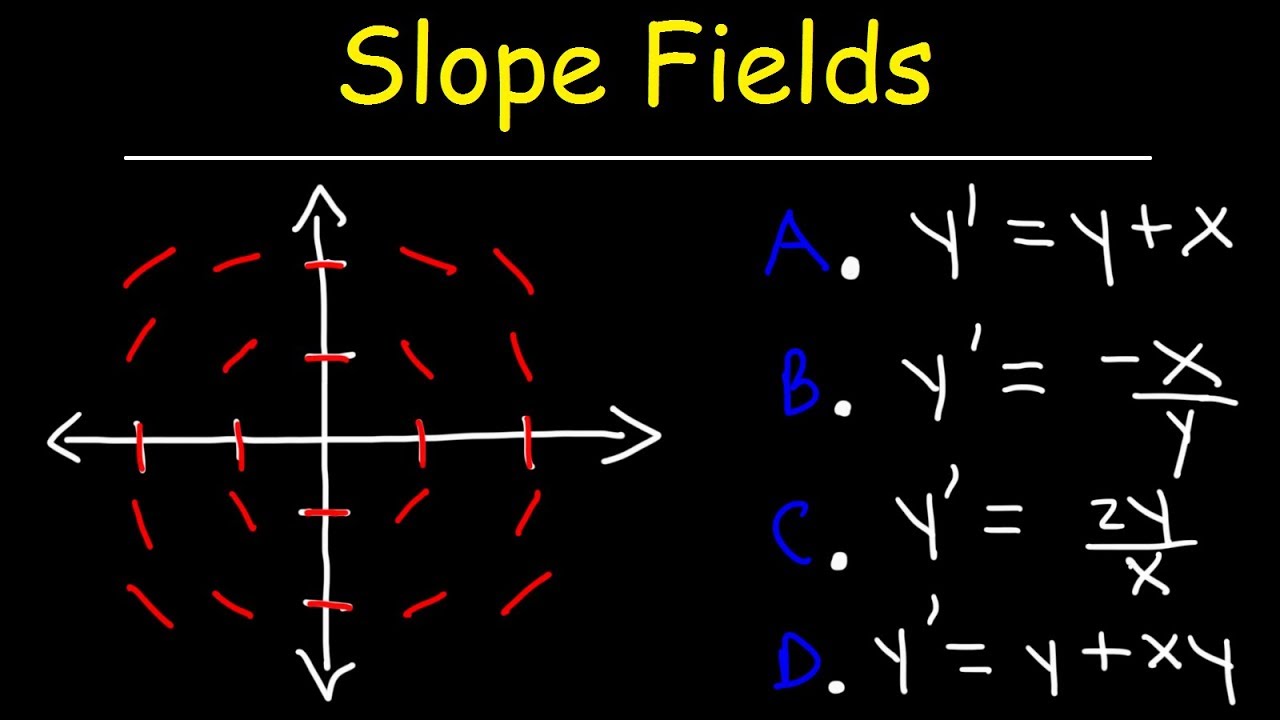
Slope Fields | Calculus

Differential equation introduction | First order differential equations | Khan Academy
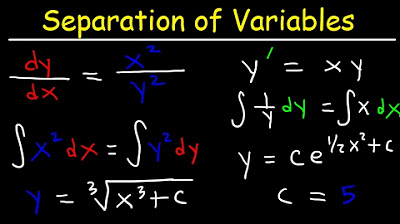
Separable First Order Differential Equations - Basic Introduction
5.0 / 5 (0 votes)
Thanks for rating: