Energy Systems Clarified
TLDRThis script explores the relationship between work and energy within a system, using the example of a block sliding up an incline attached to a spring. It discusses how different forces (gravity, normal force, spring force, kinetic friction) affect the work done on the block and the system's energy. By expanding the system to include the Earth, the spring, and the incline, the discussion highlights the conservation of energy principle and the distinction between work done by conservative and non-conservative forces. The lesson emphasizes the importance of correctly identifying the system's components for accurate work-energy analysis.
Takeaways
- 🔍 Identifying the objects in a system is crucial for understanding how work and energy are related.
- 📐 The initial and final points chosen for analysis affect the understanding of energy and work relationships.
- 🎢 In a simple system of a block sliding up an incline, the forces acting on the block include gravity, normal force, spring force, and kinetic friction.
- ⚙️ The normal force does not do work on the block because it is perpendicular to the displacement.
- 🔄 The work-energy theorem states that the change in energy of a system equals the net work done on the system.
- 🌍 When the earth is included in the system, gravitational forces become internal and cancel out, allowing for the consideration of gravitational potential energy.
- 🔄 Adding the spring to the system makes the spring force internal, introducing elastic potential energy into the work-energy equation.
- 🏗️ Including the incline in the system makes frictional forces internal, affecting the system's internal energy.
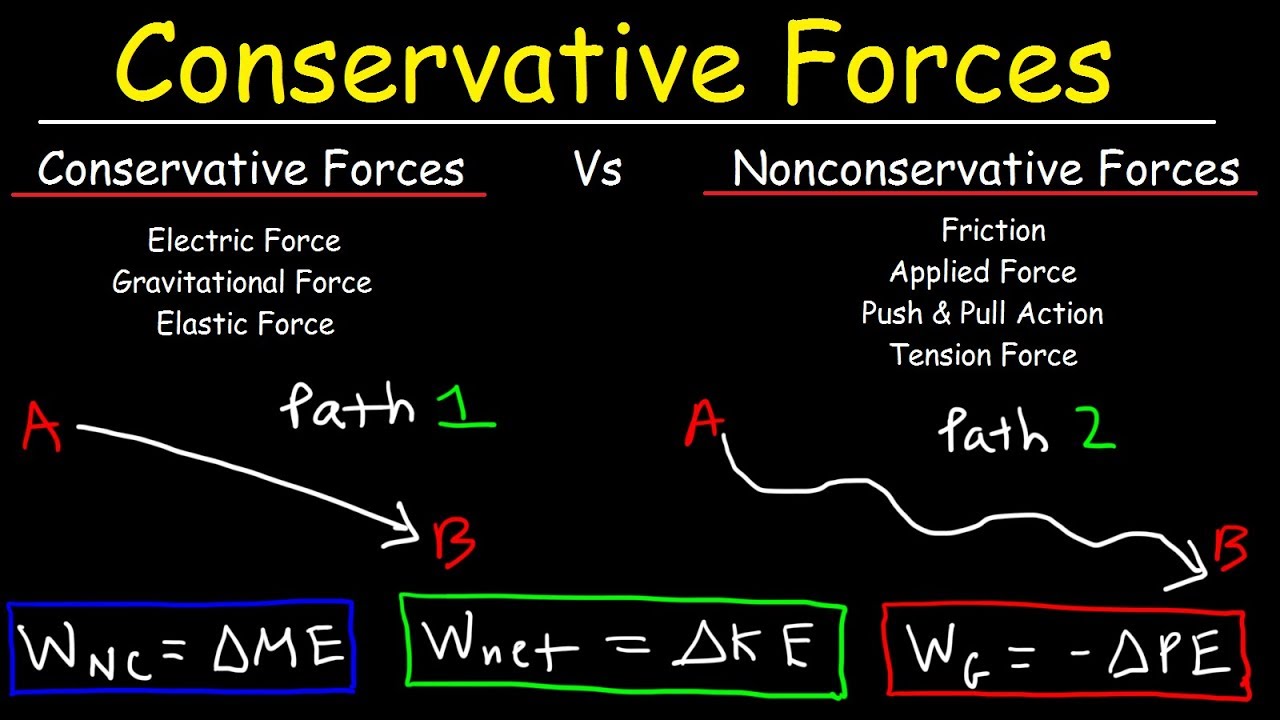
- 💢 The work done by non-conservative forces (like friction) equals the change in mechanical energy of the system.
- 🔄 Work done by conservative forces equals the negative change in their associated potential energy.
- 🌐 In a closed system where all objects are included, the total energy is conserved, and the sum of energy changes equals zero.
Q & A
Why is it important to identify the objects that are part of a system in physics?
-Identifying the objects within a system is crucial because it determines how work and energy are related within that system. The choice of system boundaries affects the analysis of energy and work interactions.
What is the initial setup in the example of the block sliding up an incline?
-The initial setup involves a block attached to a spring that starts moving up an incline. The initial point is defined after the block has started moving, and the final point is before the block stops and before it reaches the spring's equilibrium position on the incline.
What is the significance of choosing the horizontal zero line at the bottom of the incline?
-Placing the horizontal zero line at the bottom of the incline ensures that the block is always above the zero line, which helps in understanding how the block's kinetic energy and position are related throughout the motion.
Which forces act on the block as it slides up the incline?
-The forces acting on the block include gravity pulling it downward, the normal force acting perpendicular to the surface, the spring force acting parallel to the incline, and kinetic friction acting down and parallel to the incline.
Why does the normal force not do work on the block?
-The normal force does not do work on the block because its direction is perpendicular to the block's displacement. Work is only done by forces whose direction is along the path of displacement.
What is the work-energy theorem and how is it applied in this scenario?
-The work-energy theorem states that the change in energy of a system is equal to the net work done on the system. In this scenario, it is expressed as the final kinetic energy minus the initial kinetic energy equals the net work done by all external forces acting on the block.
How does adding the Earth to the system change the work-energy analysis?
-When the Earth is included in the system, the force of gravity becomes an internal force, and instead of considering the work done by gravity, the analysis can focus on the initial and final gravitational potential energies of the system.
What happens when the spring is also included in the system?
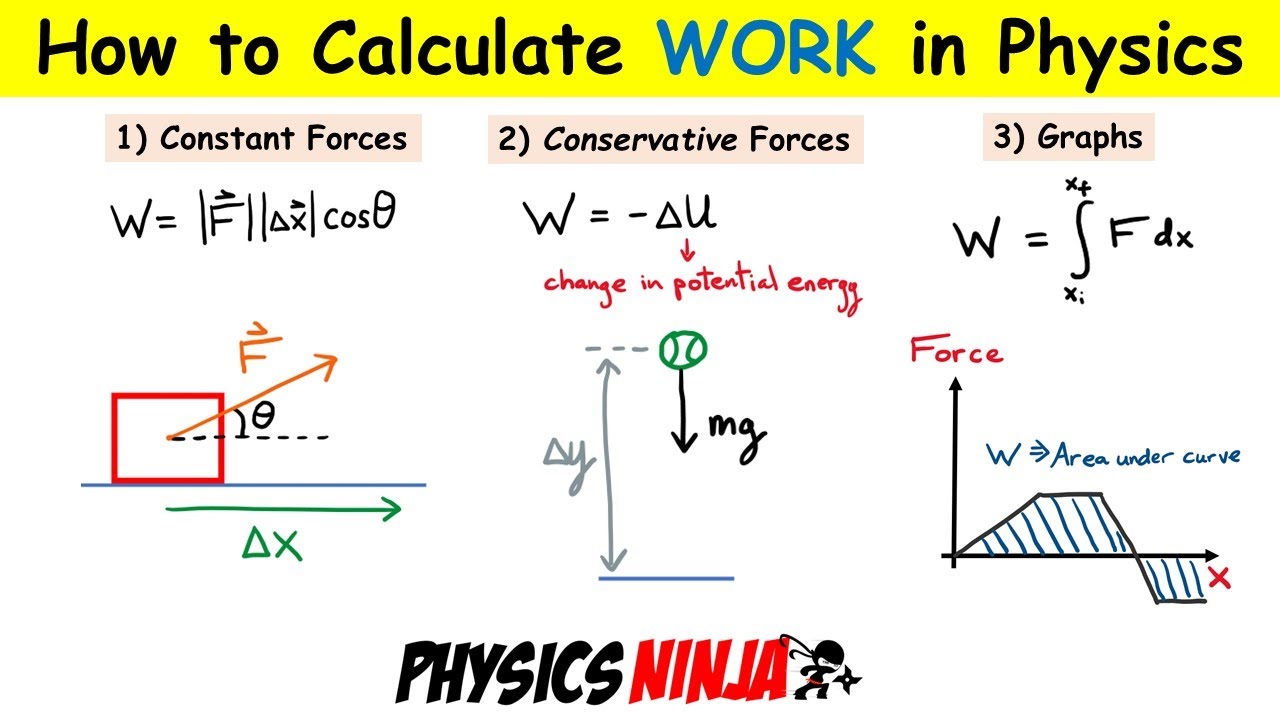
-Including the spring in the system makes the spring force an internal force. The system now accounts for initial and final elastic potential energies, and the work done by the spring force is related to the change in elastic potential energy.
What is the significance of the work done by non-conservative forces in the context of the system?
-The work done by non-conservative forces, such as friction, is equal to the change in mechanical energy of the system. This reflects the principle that non-conservative forces dissipate or add energy to the system in the form of heat or other non-mechanical forms.
How does the inclusion of the incline affect the work-energy equation?
-When the incline is included, the force of friction becomes an internal force. The equation now accounts for initial and final internal energy, and when all energies are considered, the sum of the changes in energies equals zero, demonstrating the conservation of energy principle.
What can we conclude from the changes in energy and work in this comprehensive system?
-We conclude that energy is conserved within the system when everything is included. The work done by conservative forces is related to potential energy changes, and the work done by non-conservative forces is equal to the change in the system's mechanical energy.
Outlines
🔍 Understanding Work and Energy in a System
This paragraph introduces the concept of identifying objects within a system to understand the relationship between work and energy. It uses the example of a block sliding up an incline attached to a spring to illustrate how work done by external forces results in changes in the system's energy. The discussion includes the definition of initial and final points for the block's motion and the forces acting on the block, such as gravity, normal force, spring force, and kinetic friction. It emphasizes that no energies are zero at the chosen points to better understand the energy-work relationship.
🌐 Expanding the System: Earth and Spring Included
The second paragraph expands on the initial concept by including the Earth and the spring in the system. It explains how the work done by conservative forces like gravity and the spring force can be related to potential energies. The paragraph clarifies that when the Earth is part of the system, the force of gravity becomes an internal force and contributes to the system's gravitational potential energy. Similarly, including the spring means the system now has elastic potential energy. The work-energy theorem is revisited, and the concept of mechanical energy is introduced, stating that work done by non-conservative forces equals the change in mechanical energy of the system.
🔄 Conservation of Energy with a Complete System
The final paragraph discusses the addition of the incline to the system, making it complete with the block, Earth, spring, and incline. It explains that with the complete system, the force of friction becomes an internal force and contributes to the system's internal energy. The discussion concludes with the law of conservation of energy, stating that when all relevant objects are included in the system, the total change in energy is zero, as energy is neither created nor destroyed, only transformed. The paragraph reinforces the importance of correctly identifying the system's boundaries when analyzing work and energy relationships.
Mindmap
Keywords
💡System
💡Work
💡Energy
💡Kinetic Energy
💡Gravitational Potential Energy
💡Elastic Potential Energy
💡Conservation of Energy
💡Work-Energy Theorem
💡Forces
💡Friction
💡Newton's Third Law
Highlights
Identifying objects within a system is crucial for understanding the relationship between work and energy.
An example of a simple system is a block attached to a spring sliding up an incline.
The initial and final points for energy analysis are set to understand energy relationships.
The horizontal zero line is set at the bottom of the incline for consistent reference.
A force does not do work on an object if it is perpendicular to the displacement.
The change in energy of a system equals the net energy transferred into or out of the system.
All forces acting on the block are external to the block system when only the block is considered.
Gravitational potential energy is not present when the system consists of only one object, like the block.
The work-energy theorem states that net work equals change in kinetic energy.
Adding the Earth to the system makes the force of gravity an internal force, canceling out in energy equations.
Including the spring in the system makes the spring force an internal force, introducing elastic potential energy.
The work done by non-conservative forces equals the change in mechanical energy of the system.
Adding the incline to the system makes the force of friction an internal force, affecting internal energy.
Energy conservation is observed when the system includes all relevant objects, as energy changes forms but is not created or destroyed.
Work done by conservative forces equals the negative change in their associated potential energy.
The original work-energy equation can be derived back to a system consisting of only the block.
Identifying the system's objects affects the work and energy equations used in analysis.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: