Momentum - Open and Closed Systems - IB Physics
TLDRThe video script discusses the conservation of momentum, addressing common misconceptions through examples like pushing a cart and the role of friction in preventing the person's movement. It explains how the Earth's immense momentum makes its changes unnoticeable in such interactions. The concepts of 'system', 'closed system', and 'open system' are introduced, with examples illustrating when to apply each for problem-solving in physics. The video emphasizes the practicality of using open systems to simplify calculations when external forces significantly affect the system's momentum.
Takeaways
- 🌌 Momentum conservation is often misunderstood when one object seems to change velocity while another doesn't, but it's still conserved due to unseen forces like friction.
- 💡 Newton's Third Law states that for every action, there is an equal and opposite reaction, which applies to forces between objects and results in momentum conservation.
- 🌍 The Earth's immense mass makes its changes in momentum due to small interactions nearly immeasurable, complicating the application of momentum conservation in certain scenarios.
- 🚀 When calculating momentum, the Earth is typically not considered part of the system if the force of gravity and normal force balance out, simplifying the conservation of momentum calculations.
- 🔄 Systems can be defined as closed or open based on whether they experience a net force from outside objects, affecting how momentum is calculated.
- 🔒 A closed system has no net external forces acting on it, allowing for the conservation of momentum within the system, making calculations straightforward.
- 🔓 An open system has a net force acting on it from an external source, leading to a change in the system's total momentum, which must be accounted for in calculations.
- 📐 When solving problems, choose a system type (closed or open) based on the ease of finding the momentum of the objects and the presence of external forces.
- 🎯 In scenarios with air resistance or other complex forces, it's often more practical to treat the system as open and not include the difficult-to-calculate particles in the system.
- 📊 Examples of closed and open systems demonstrate how to calculate momentum changes, either by maintaining a constant total momentum (closed) or by adding the external impulse (open).
- 🛠️ Understanding the concept of systems and the difference between closed and open systems is crucial for solving momentum problems in physics.
Q & A
What is an example of a situation where momentum does not appear to be conserved?
-An example is when a person pushes a cart, and it seems like only the cart experiences a change in momentum because the person, due to friction, does not change their velocity.
How is the Earth involved in the conservation of momentum when a person pushes a cart?
-The Earth is involved because it experiences a force of friction from the person in the opposite direction of the push. This results in the Earth also experiencing an impulse, maintaining the conservation of momentum.
Why is it difficult to measure the change in Earth's momentum during such interactions?
-It is difficult because Earth's momentum is already so large (approximately 1.9 times 10^29 Newton seconds) that the small impulse from a person pushing a cart would barely affect its velocity, making the change too small to measure.
Under what conditions is Earth not considered part of the momentum problem?
-Earth is not part of the momentum problem when the force of gravity is balanced out by the normal force, meaning the total impulse on the object from Earth is zero.
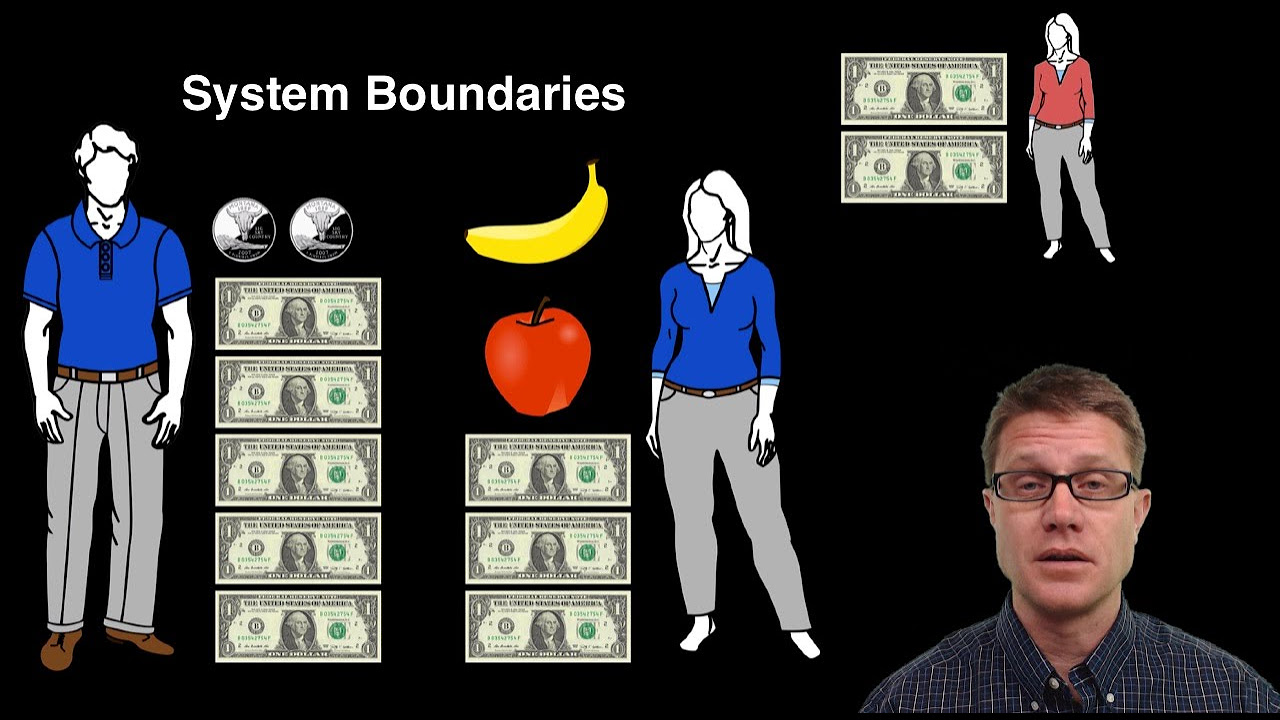
What is the definition of a 'system' in the context of physics?
-A 'system' is a group of objects that are being examined together, and it can be defined subjectively based on what the examiner wants to study.
What is the difference between a closed and an open system?
-A closed system is a group of objects that do not experience a net force from any object outside the system, conserving momentum. An open system experiences a nonzero net force from an object outside the system, leading to a change in total momentum.
How can you determine whether to use a closed or open system approach in problem-solving?
-Use a closed system approach if it's easy to find the momentum of each object within the system. Use an open system approach if it's not easy to find the total momentum of each object, and simply add the impulse from the outside object to the total momentum of the system.
What is the total momentum of the cart-1 and cart-2 system before they interact?
-The total momentum of the cart-1 and cart-2 system before they interact is 4 Newton seconds.
After the interaction, what is the final velocity of the 3 kg sphere in the closed system example?
-After the interaction, the final velocity of the 3 kg sphere is 3 meters per second to the right.
In the open system example with the sphere rolling down a tilted surface, what is the total momentum of the 3 kg sphere after 2 seconds?
-The total momentum of the 3 kg sphere after 2 seconds is 38.4 Newton seconds.
How does the concept of systems help in understanding physics?
-The concept of systems helps in understanding physics by providing a framework to analyze and solve problems involving the interaction of objects, considering the conservation of momentum, and simplifying calculations by defining the scope of the analysis.
Outlines
🚗 Conservation of Momentum and Newton's Third Law
This paragraph discusses the conservation of momentum in scenarios where it may seem that momentum is not conserved. The example given involves a person pushing a cart, where it appears only the cart's momentum changes due to the person not moving. However, the video explains that the Earth also experiences an impulse due to the person's force, via friction, making the Earth part of the system. The Earth's immense momentum means that the change in its velocity due to such small interactions is immeasurable. The paragraph emphasizes that while momentum is conserved, the Earth's involvement in the momentum problem complicates calculations due to its large mass.
🌍 Understanding Systems: Closed and Open
The second paragraph introduces the concept of 'systems' in physics, defining it as a group of objects and explaining that the choice of what objects constitute a system is subjective. It differentiates between closed and open systems. A closed system is one where objects do not experience a net force from outside the system, thus conserving momentum. An open system, on the other hand, experiences a net force from an external object, leading to a change in total momentum. The paragraph uses examples such as two carts bumping into each other (closed system) and a cart hitting a wall (open system) to illustrate these concepts. It also addresses the practicality of using open systems when calculating momentum is too complex, such as when air resistance is involved.
🔄 Applying Closed and Open Systems to Problem Solving
The final paragraph provides a practical guide for applying the concepts of closed and open systems to problem-solving in physics. It suggests using a closed system approach when it's easy to find the momentum of objects within the system and they do not experience external forces. Conversely, an open system should be used when determining the total momentum of each object is challenging, by including only the easily identifiable objects and accounting for external forces. The paragraph offers examples of both closed and open systems, such as two spheres colliding (closed) and a sphere rolling down a ramp under the influence of gravity (open), to demonstrate how to calculate momentum in these scenarios.
Mindmap
Keywords
💡Momentum
💡Conservation of Momentum
💡Newton's Third Law
💡Friction
💡Impulse
💡Closed System
💡Open System
💡Earth's Momentum
💡System
💡Force
💡Impossibly Large Positive Momentum
Highlights
Momentum conservation is often misunderstood due to everyday examples like pushing a cart.
Even when it appears that momentum is not conserved, such as pushing a cart on a table, the principle still holds true.
Friction plays a crucial role in preventing the person from moving when pushing the cart, illustrating the interconnected forces in a system.
The Earth experiences an equal and opposite impulse to the cart when a force is applied, due to the universal application of Newton's Laws.
The Earth's immense momentum means that small changes due to everyday interactions are virtually immeasurable.
When solving momentum problems, the Earth is typically not considered part of the system if gravity and normal forces balance out.
In scenarios where gravity and normal forces are balanced, the Earth does not contribute to the total impulse, simplifying calculations.
The concept of 'system' in physics is introduced as a group of objects that can be defined subjectively based on the problem at hand.
A closed system is defined as a group of objects not experiencing a net force from outside the system, where momentum is conserved.
An open system involves a net force from an external object, leading to a change in the total momentum of the system.
The choice between using a closed or open system in problem-solving depends on the ease of calculating the momentum of the interacting objects.
In situations where calculating the total momentum of each object is difficult, an open system approach is often more practical.
Air resistance, when significant, is typically treated as an open system, with the air particles' momentum considered external to the system.
Examples of both closed and open systems are provided, illustrating the calculation of momentum in different physical scenarios.
The concept of systems is fundamental to understanding physics, laying the groundwork for more complex problem-solving in the future.
The video series aims to clarify common misconceptions about momentum and provide a solid understanding of its conservation.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: