Open vs Closed Systems and Total Mechanical Energy & Momentum (AP Physics 1)
TLDRThis video script delves into the concepts of open and closed systems, focusing on how external forces and the conservation of energy and momentum affect these systems. It explains the role of forces like gravity and springs in altering mechanical energy and distinguishes between conservative and non-conservative forces. The video uses examples of blocks, springs, and Earth to illustrate how work done by external forces can either increase or decrease the total mechanical energy of a system.
Takeaways
- 📚 An open system is one where external forces act on it, leading to the conservation of energy and momentum within a closed boundary.
- 🚫 In a closed system, no external forces are present, and both energy and momentum are conserved, meaning they remain constant throughout any process.
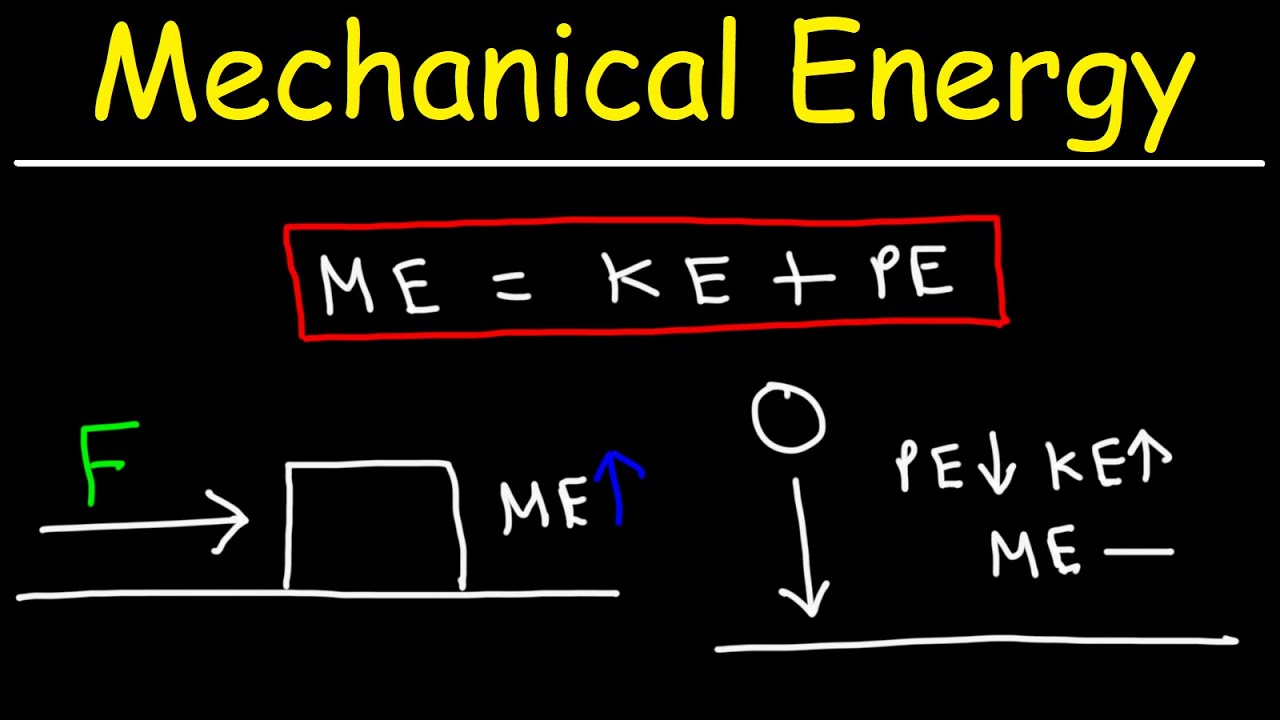
- 🔄 The conservation of mechanical energy (the sum of kinetic and potential energies) is dependent on the system being closed and no external forces acting upon it.
- 🔧 When external forces do work on a system, the mechanical energy changes by the amount of work done, which can be positive or negative depending on the direction of the force relative to motion.
- 🎯 To determine if a system is open or closed, define the system by identifying what is included within the boundary and what forces are acting on it from outside.
- 📈 The work done by external forces can be assessed by considering the direction of the force relative to the motion of the object within the system.
- 🌐 In a multi-object system, the definition of the system and the inclusion or exclusion of forces (like strings or springs) can change the conservation status from open to closed.
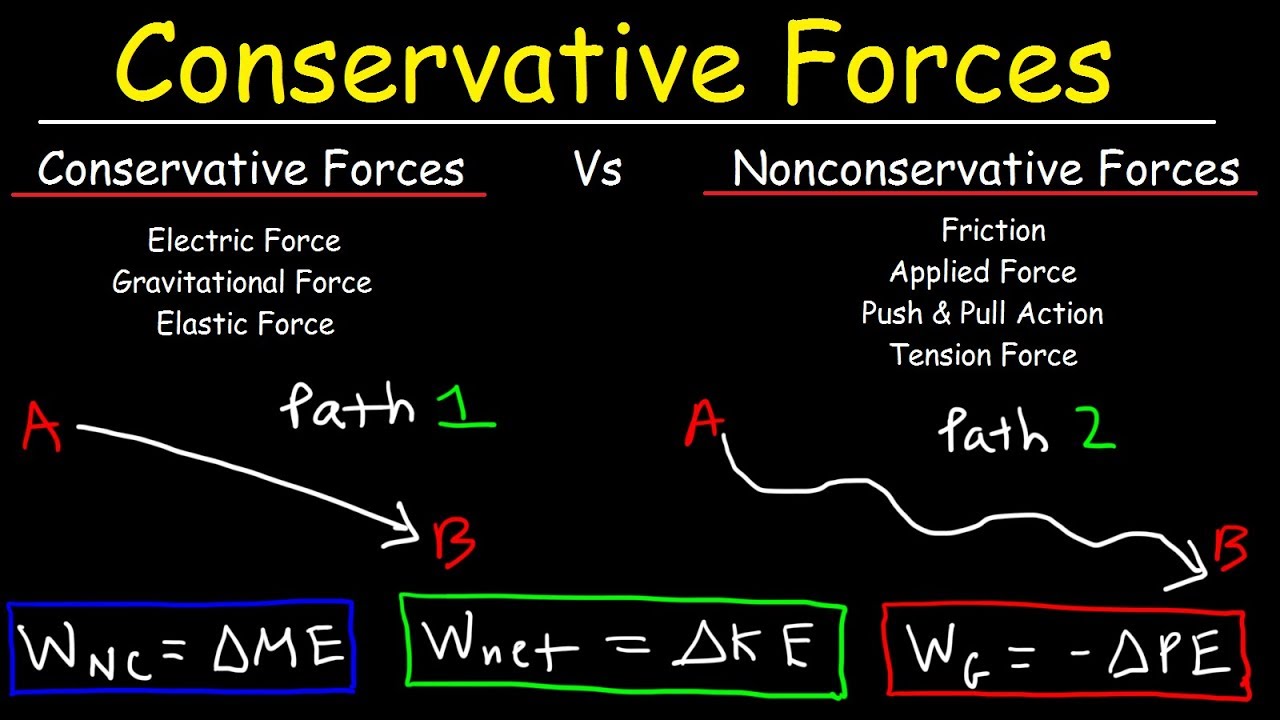
- 🔄 Conservative forces, like gravity and spring forces, depend only on the initial and final positions and store potential energy, whereas non-conservative forces, like friction, dissipate energy and depend on the path of motion.
- 📉 Friction is a non-conservative force that always reduces the mechanical energy of a system unless the surface it acts upon is considered part of the system.
- 🔎 The script provides examples of block-spring systems to illustrate how to identify open and closed systems and how to determine changes in mechanical energy based on the work done by external forces.
Q & A
What is the main difference between an open and a closed system?
-A closed system has no external forces acting on it, conserving energy and momentum, while an open system has external forces that can do work on the system, leading to changes in energy and momentum.
How does the presence of external forces affect the mechanical energy in an open system?
-In an open system, external forces can do positive or negative work, depending on the direction of the force relative to the motion. This results in an increase or decrease in the system's mechanical energy.
What is the role of friction in a system?
-Friction is a non-conservative force that dissipates energy from the system, typically reducing its mechanical energy, unless the surface causing friction is considered part of the system.
How does the direction of work done by a force affect the mechanical energy of a system?
-If the work done by a force is in the same direction as the motion, it is positive work and increases the mechanical energy. If it is in the opposite direction, it is negative work and decreases the mechanical energy.
What is the significance of defining the system correctly when analyzing mechanical energy?
-Defining the system correctly is crucial for determining which forces are internal and which are external. This affects whether the mechanical energy is conserved or changes due to the work done by external forces.
How does the concept of conservation of energy apply to a closed system?
-In a closed system, the total mechanical energy remains constant because there are no external forces doing work on the system. This is a direct application of the conservation of energy principle.
What are conservative forces and how do they differ from non-conservative forces?
-Conservative forces, like gravity and spring force, depend only on the initial and final positions and store potential energy. Non-conservative forces, like friction, dissipate energy and depend on the path taken, not just the initial and final positions.
How does the mechanical energy of a system change when only conservative forces are acting within it?
-When only conservative forces are acting within a system, the mechanical energy remains constant. This is because the work done by conservative forces is path-independent and the total mechanical energy is conserved.
What is the relationship between the direction of motion and the work done by external forces in an open system?
-The work done by external forces in an open system is related to the direction of motion in that forces acting in the same direction as the motion do positive work, while forces acting in the opposite direction do negative work, affecting the mechanical energy accordingly.
How does the inclusion of a spring force in a system affect the mechanical energy calculation?
-The inclusion of a spring force in a system must be considered based on whether the spring is inside or outside the defined system. If it is inside, it does not affect the mechanical energy as it is a conservative force within the system. If it is outside, it can do work on the system, changing the mechanical energy.
What is the key to solving problems involving the mechanical energy of open and closed systems?
-The key is to correctly identify the system's boundaries, determine which forces are internal and which are external, and understand how these forces affect the mechanical energy through work done in the direction of motion.
Outlines
📘 Understanding Open and Closed Systems
This paragraph introduces the concepts of open and closed systems. A closed system is one where no external forces act upon it, conserving energy and momentum. In contrast, an open system experiences outside forces that can do work, leading to changes in energy. The speaker clarifies the difference between the two by discussing how external forces affect the mechanical energy within a system, emphasizing that mechanical energy will not change in a closed system but can increase or decrease in an open system depending on the work done by external forces.
🌐 Conservative and Non-Conservative Forces
The speaker delves into the distinction between conservative and non-conservative forces. Conservative forces, such as gravity, depend only on the initial and final positions, storing potential energy regardless of the path taken. Non-conservative forces, like friction, dissipate energy and depend on the path of motion. The speaker explains that friction always reduces the mechanical energy of a system unless the surface causing friction is considered part of the system. This paragraph establishes a foundational understanding of how different types of forces impact the energy within a system.
🔄 Analyzing the Work Done in Various Systems
The speaker applies the concepts of open and closed systems to analyze three different scenarios involving a block, a spring, and sometimes the Earth. In each case, the speaker determines whether the system is open or closed based on the forces acting upon it. The analysis focuses on whether the net force does positive or negative work, which in turn affects the mechanical energy of the system. The speaker concludes that in all scenarios, the systems are open, and the mechanical energy changes accordingly, either increasing or decreasing based on the work done by external forces.
🎉 Reflecting on the Learning Process
In the final paragraph, the speaker expresses gratitude towards the viewers for their support and engagement. They reflect on the importance of their educational mission, sharing their motivation to help students understand complex concepts. The speaker also mentions their commitment to producing content, even at the expense of personal rest, to ensure that learners have access to valuable educational resources. This paragraph concludes the video on a personal note, highlighting the speaker's dedication to their audience.
Mindmap
Keywords
💡Open System
💡Closed System
💡Mechanical Energy
💡Conservation of Energy
💡Work
💡Kinetic Energy
💡Potential Energy
💡Force
💡Conservative Force
💡Non-Conservative Force
💡Momentum
Highlights
An open system is one where external forces act on it, causing energy and momentum to change.
A closed system has no external forces acting on it, conserving energy and momentum.
In an open system, energy changes by the amount of work done by external forces.
Work done by external forces can be positive or negative depending on the direction of the force relative to motion.
A single object system, like a block, can have its energy and momentum changed by external forces.
In a closed system, like a block with a spring, the mechanical energy remains constant as there are no external forces.
Friction is a non-conservative force that dissipates energy, reducing the mechanical energy of a system unless included in the system.
Conservative forces, like gravity and spring forces, depend only on initial and final positions, storing potential energy.
The mechanical energy of a system changes if there are forces acting on it that are not part of the system.
For a system of a block and a spring, the mechanical energy decreases due to the negative work done by gravity.
In a system of a block, spring, and Earth, the mechanical energy increases due to the positive work done by the spring force.
Defining the system correctly is crucial for determining whether it is open or closed and how energy changes.
Mechanical energy is the sum of kinetic and potential energies in a system.
In a closed system with only internal forces, like block-spring-earth, mechanical energy remains constant.
Understanding the interaction of forces and the definition of the system is key to solving problems involving mechanical energy.
The direction of forces relative to the motion determines whether work is positive or negative, affecting the mechanical energy.
The concept of open and closed systems is essential for grasping the conservation of energy and momentum in physics.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: