Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (42 of 92) k=? of a Diatomic Molecule

Michel van Biezen
1 Apr 201804:59
EducationalLearning
32 Likes 10 Comments

TLDRThis video delves into the spring constant of a diatomic molecule, using carbon monoxide as a case study. It explains how the molecule's vibrational frequency, observed through infrared radiation, can be related to its reduced mass and the spring constant. By calculating the energy absorbed or emitted in electron volts and applying quantum mechanics principles, the video demonstrates how the molecule's oscillatory behavior can be quantified, revealing that carbon monoxide vibrates at an astonishing 65 trillion times per second. Ultimately, it shows that the spring constant for such a molecule is approximately 2,000 Newtons per meter, highlighting the quantum jumps in energy during molecular absorption and emission processes.

Takeaways
  • 🌟 The spring constant of a diatomic molecule is explored using carbon monoxide (CO) as an example.
  • 📈 Carbon monoxide absorbs or emits radiation with an energy frequency of 0.27 electron volts, which is in the infrared range.
  • 🔵 The energy of a photon (E) is related to Planck's constant (h) and the frequency (ν) of the photon: E = hν.
  • 🎆 The frequency (ν) can be expressed as ν = Ω / 2π, where Ω is the angular frequency.
  • 📊 The angular frequency (Ω) for CO is calculated using the observed energy (0.27 eV) and Planck's constant (h-bar).
  • 🧬 The vibrational frequency of a CO molecule is found to be 65 trillion vibrations per second.
  • 🔗 The reduced mass (μ) of the CO molecule is used to calculate the spring constant (K) as K = Ω^2 * μ.
  • 📈 The calculated spring constant for CO is 1926 Newtons per meter, which is close to the observed value.
  • 🌐 The units for the spring constant are derived from the combination of mass, length, and force units (kg m/s² becomes N/m).
  • 🚀 Diatomic molecules like CO vibrate at high frequencies, with each vibration corresponding to a quantum energy level.
  • 🌈 The energy difference (ΔE) between levels is quantified by h-bar times the angular frequency (h-barΩ), which is equal to 0.27 eV.
Q & A

  • What is the subject of the video?

    -The video explores the spring constant of a diatomic molecule, specifically using carbon monoxide as an example.

  • What type of molecule is used as an example in the video?

    -Carbon monoxide, which consists of a single carbon atom and a single oxygen atom, is used as the example diatomic molecule.

  • What is the energy frequency of the radiation absorbed or emitted by a carbon monoxide molecule?

    -The energy frequency of the radiation absorbed or emitted by a carbon monoxide molecule is 0.27 electron volts, which is in the infrared range below the visible light range.

  • How is the energy of a photon related to its frequency?

    -The energy of a photon is related to its frequency by the equation E = h * f, where E is the energy, h is Planck's constant, and f is the frequency of the photon.

  • What is the relationship between the angular frequency (Omega) and the reduced mass (μ) in the context of a diatomic molecule?

    -The angular frequency (Omega) is related to the reduced mass (μ) through the equation Omega = sqrt(K/μ), where K is the spring constant and Omega is the angular frequency of the molecule's oscillation.

  • How many oscillations per second does a carbon monoxide molecule vibrate at?

    -A carbon monoxide molecule vibrates at 65 trillion oscillations per second, which is its base vibration frequency.

  • What is the spring constant equivalent for a diatomic molecule like carbon monoxide?

    -The spring constant equivalent for a diatomic molecule like carbon monoxide is measured to be 1926 Newtons per meter.

  • How can the spring constant be calculated from the observed vibrational frequency of a molecule?

    -The spring constant can be calculated using the equation K = Omega^2 * μ, where Omega is the angular frequency of the molecule's oscillation and μ is the reduced mass.

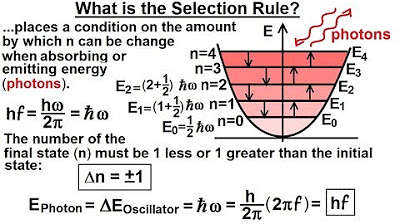
  • What is the significance of the energy difference (ΔE) when a molecule absorbs or emits a photon?

    -The energy difference (ΔE) represents the amount of energy the molecule absorbs or emits as it jumps from one energy level to another. This energy change is quantized, meaning it occurs in discrete amounts equal to h-bar times the angular frequency (h-bar * Omega).

  • How does the concept of quantum jumps relate to the energy absorption and emission of a diatomic molecule?

    -Quantum jumps describe the discrete changes in energy levels of a quantum mechanical oscillator, such as a diatomic molecule. The molecule can only absorb or emit energy in amounts equal to the energy of the photons associated with the difference in energy levels.

  • What is the practical implication of understanding the spring constant and vibrational frequency of diatomic molecules?

    -Understanding the spring constant and vibrational frequency of diatomic molecules allows for the prediction and analysis of their behavior, including how they interact with electromagnetic radiation and their potential energy states, which is crucial in fields such as spectroscopy and molecular physics.

Outlines
00:00
🌟 Introduction to Diatomic Molecule's Spring Constant

This paragraph introduces the concept of the spring constant of a diatomic molecule, specifically focusing on carbon monoxide (CO). It explains that the energy frequency of radiation absorbed or emitted by CO lies in the infrared range, below visible light. The energy of a photon is described as being equal to Planck's constant times the frequency of the photon, which is then related to the spring constant through the vibrational frequency and reduced mass of the molecule. The paragraph concludes by calculating the spring constant for CO to be approximately 1926 Newtons per meter, which is close to the observed value.

Mindmap
Keywords
💡diatomic molecule
A diatomic molecule is a chemical species that consists of two atoms bonded together. In the context of the video, carbon monoxide (CO) is used as an example of a diatomic molecule, which is made up of one carbon atom and one oxygen atom. This concept is central to the video's theme as it explores the vibrational properties and energy transitions of such molecules, particularly focusing on how they absorb and emit radiation.
💡spring constant
The spring constant, often denoted as k, is a measure of the stiffness of a spring. In the context of the video, it is used as an analogy to describe the force constant of a diatomic molecule like carbon monoxide when it vibrates. The spring constant is related to the vibrational frequency of the molecule and can be calculated using the observed emission or absorption of energy by the molecule.
💡Planck's constant
Planck's constant is a fundamental constant in quantum mechanics, denoted by the symbol h or h-bar (reduced Planck's constant). It relates the energy of a photon to its frequency. In the video, Planck's constant is used to connect the energy of the absorbed or emitted photons with the vibrational frequency of the carbon monoxide molecule, allowing the calculation of the molecule's angular frequency and ultimately its spring constant.
💡angular frequency
Angular frequency, denoted by the symbol ω (Omega), is a measure of how quickly an object vibrates or oscillates, expressed in radians per second. In the video, the angular frequency of the carbon monoxide molecule's vibration is calculated using the energy of the absorbed or emitted photon and Planck's constant. This concept is crucial for understanding the molecule's vibrational characteristics and its quantized energy levels.
💡reduced mass
The reduced mass, often denoted as μ, is a concept used in the two-body problem in physics to describe the mass parameter that affects the motion of two objects bound by a force, such as a spring. In the context of the video, the reduced mass of the carbon monoxide molecule is used in the equation for the angular frequency to determine the molecule's vibrational characteristics. It is calculated based on the masses of the two atoms in the molecule.
💡quantum jumps
Quantum jumps, or quantum leaps, refer to the discrete changes in energy levels that a quantum system can undergo. In the video, this concept is applied to the vibrational energy levels of the carbon monoxide molecule, explaining that the molecule can only absorb or emit energy in discrete amounts corresponding to the energy difference between its quantized vibrational levels.
💡infrared range
The infrared range is a portion of the electromagnetic spectrum that lies just beyond the red end of visible light. It is characterized by longer wavelengths and lower frequencies compared to visible light. In the video, the energy frequency of radiation absorbed or emitted by the carbon monoxide molecule is found to be in the infrared range, which is below the visible light range.
💡vibrational frequency
Vibrational frequency refers to the rate at which a molecule vibrates or oscillates. In the context of the video, the vibrational frequency of the carbon monoxide molecule is determined by analyzing the energy of the photons it absorbs or emits. This frequency is a key characteristic of the molecule's vibrational motion and is used to calculate other properties such as the spring constant.
💡energy levels
Energy levels are the quantized, discrete states of energy that a quantum system can occupy. In the video, the energy levels of the carbon monoxide molecule are discussed in relation to its vibrational motion. The molecule can only transition between these energy levels by absorbing or emitting photons, each corresponding to a specific energy difference.
💡oscillator
An oscillator is a system that vibrates with a consistent frequency and amplitude. In the context of the video, the carbon monoxide molecule is treated as a quantum mechanical oscillator, which allows for the application of classical oscillator concepts to understand its vibrational behavior and the quantized nature of its energy levels.
💡photon
A photon is a quantum of electromagnetic radiation, carrying energy and momentum. In the video, photons are used to explain how the carbon monoxide molecule interacts with the electromagnetic spectrum, specifically through the absorption and emission of photons in the infrared range.
Highlights

Exploration of the spring constant of a diatomic molecule, specifically carbon monoxide.

Carbon monoxide's energy frequency of radiation is in the infrared range, below visible light.

The energy absorbed or emitted by carbon monoxide is 0.27 electron volts.

The energy of a photon is calculated using Planck's constant times the frequency of the photon.

The basic frequency of a molecule is denoted as Omega sub nought or F sub nought.

The angular frequency (Omega) is calculated as HF divided by H bar.

The angular frequency of a carbon dioxide molecule is 4.1 x 10^14 oscillations per second.

The actual oscillations per second are found by dividing the angular frequency by 2 pi.

Carbon monoxide molecules vibrate at 65 trillion vibrations per second as their base vibration.

The spring constant for a diatomic molecule like carbon monoxide is derived from the reduced mass equation.

The spring constant is equivalent to 1926 Newtons per meter for carbon monoxide.

The equation for the spring constant is the square root of K over m M (reduced mass).

The reduced mass for carbon monoxide was calculated in a previous video.

The energy difference (delta energy) between levels is given by h-bar Omega.

The energy absorbed or emitted by the molecule is quantized, jumping from one level to another.

The molecule absorbs or emits photons equal to the energy difference between levels.

The relationship between the absorption and emission of energy by a diatomic molecule is explained through quantum mechanics.

A diatomic molecule like carbon monoxide acts as a quantum mechanical oscillator.

The spring constant of a typical diatomic molecule is in the order of 2,000 Newtons per meter.

The vibrational frequency of a typical diatomic molecule is in the order of many trillions of vibrations per second.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (51 of 92) Oscillator Amplitude - Diatomic

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (39 of 92) What is the Quantum Oscillator?

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (46 of 92) Quantum Nature of Oscillator 2

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (40 of 92) What is Reduced Mass?

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (50 of 92) What is Oscillator Amplitude?

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (44 of 92) What is the Selective Rule?

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Quantum MechanicsCarbon MonoxideVibrational AnalysisSpring ConstantPlanck's ConstantInfrared SpectrumMolecular OscillationPhoton EnergyQuantum JumpsPhysics Education