Lec 2 | MIT 18.01 Single Variable Calculus, Fall 2007
TLDRIn this lecture, the professor revisits the concept of derivatives, initially defined as the slope of a tangent line, and then introduces them as a measure of rate of change. Examples from physics, such as current and speed, are used to illustrate the concept of instantaneous rate of change. The lecture also covers different types of discontinuities and their implications. The professor emphasizes the importance of understanding limits and continuity, explaining left-hand and right-hand limits, and the theorem that differentiability implies continuity. The discussion aims to deepen students' understanding of calculus concepts and their applications in real-world scenarios.
Takeaways
- 📚 The lecture begins with a reminder of the definition of a derivative as the slope of a tangent line and the computation of derivatives for basic functions.
- 🔍 The professor emphasizes the importance of understanding different interpretations of a derivative, particularly the rate of change, which is crucial for applying calculus in real-world scenarios.
- 📈 The concept of rate of change is introduced, explaining the difference between average rate of change (delta y / delta x) and instantaneous rate of change (denoted as dy/dx).
- 🚀 Examples from physics are given to illustrate the concept of derivatives, such as current (dq/dt) and speed (ds/dt), with a detailed example of the 'pumpkin drop' to explain instantaneous speed.
- 🏢 The 'pumpkin drop' example demonstrates calculating average and instantaneous speed, highlighting the significance of the instantaneous speed upon impact.
- ✈️ The application of derivatives extends beyond physics, with examples given from other fields such as temperature gradients in weather prediction and the sensitivity of GPS measurements.
- 🛰️ The GPS example explains how errors in measured distances (delta h) affect the calculated horizontal distance (delta L), and the importance of this in accurately landing airplanes.
- 📉 The lecture transitions into a more rigorous discussion of limits and continuity, which are foundational for understanding derivatives and differentiability.
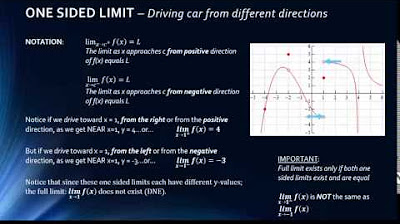
- 🔑 The professor introduces the concept of left-hand and right-hand limits, explaining their significance in determining the continuity of a function at a given point.
- 🚫 Discontinuities are categorized into types such as jump discontinuities, removable discontinuities, and infinite discontinuities, each with distinct characteristics and implications.
- 🔄 The final part of the lecture focuses on the theorem that differentiability implies continuity, with a proof provided to show that if a function is differentiable at a point, it is also continuous at that point.
Q & A
What is the main topic discussed in the second lecture?
-The main topic discussed in the second lecture is the derivative, focusing on its interpretation as the rate of change and providing various examples from physics and other fields to illustrate this concept.
What is the geometric interpretation of a derivative mentioned in the lecture?
-The geometric interpretation of a derivative mentioned in the lecture is the slope of the tangent line to the curve of a function at a given point.
What are the two computations of derivatives provided in the lecture?
-The two computations of derivatives provided in the lecture are the derivative of 1/x, which is -1/x^2, and the derivative of x to the nth power for n = 1, 2, etc., which turns out to be nx^(n-1).
What is the physical interpretation of dq/dt?
-In physics, dq/dt represents the current, where 'q' usually denotes the charge.
How is the rate of change of distance related to speed?
-The rate of change of distance with respect to time is known as speed. It is a tangible example used to illustrate the concept of the derivative as an instantaneous rate.
Can you explain the example of the pumpkin drop used in the lecture?
-The pumpkin drop example is used to illustrate the concept of instantaneous speed. It describes an event where objects are dropped from the top of a building, and the instantaneous speed upon impact is calculated using the derivative of the height function with respect to time.
What is the significance of the temperature gradient (dT/dx) in weather prediction?
-The temperature gradient (dT/dx) is significant in weather prediction because it represents the temperature difference that causes air flows and leads to changes in weather patterns.
Why is the concept of sensitivity in measurements important in GPS?
-The concept of sensitivity in measurements is important in GPS because it helps estimate the horizontal distance (L) from the measured distance (h) using radio signals. This sensitivity, or the derivative of L with respect to h, is crucial for accurate positioning, such as landing airplanes.
What is the equation for height given in the lecture and how is it derived?
-The equation for height given in the lecture is h = 80 - 5t^2, where h is in meters and t is time in seconds. It is derived from the physics formula for the distance traveled under constant acceleration due to gravity, which is part of the curriculum in a physics course like 8.01 at MIT.
What is the difference between an easy limit and a derivative limit?
-An easy limit can be evaluated by simply plugging in the value of x into the function, whereas a derivative limit involves a 0/0 indeterminate form that requires algebraic manipulation to evaluate, often involving the concept of limits from the left and right sides of a point.
What is the definition of continuity at a point x_0?
-A function f is continuous at x_0 if the limit of f(x) as x tends to x_0 exists and is equal to f(x_0). This means that the left and right limits must exist, be equal, and match the value of the function at x_0.
What are the different types of discontinuities mentioned in the lecture?
-The different types of discontinuities mentioned are jump discontinuities, removable discontinuities, infinite discontinuities, and other ugly discontinuities like oscillating functions with no left or right limit as x approaches a certain value.
Why is it incorrect to say that the limit as x goes to 0 of 1/x is infinity?
-It is incorrect because the limit as x goes to 0 of 1/x actually has different values depending on the direction from which x approaches 0. From the right (x > 0), the limit is positive infinity, and from the left (x < 0), the limit is negative infinity. Therefore, stating simply 'infinity' without specifying is misleading and incorrect.
How does the theorem 'differentiable implies continuous' relate to the proof provided?
-The theorem 'differentiable implies continuous' states that if a function has a derivative at a point x_0, then it is also continuous at x_0. The proof provided demonstrates this by showing that the limit of (f(x) - f(x_0)) as x approaches x_0 is zero, which is achieved by multiplying and dividing the expression by (x - x_0) and using the fact that the derivative exists at x_0.
What is the significance of the proof that a differentiable function is continuous?
-The proof is significant because it establishes a fundamental property of differentiable functions. It shows that continuity is a necessary condition for differentiability, meaning that a function cannot be differentiable at a point if it is not continuous at that point. This is important for understanding the relationship between differentiability and continuity in calculus.
Outlines
📚 Introduction to Derivatives and MIT OpenCourseWare
The lecture begins with an introduction to MIT OpenCourseWare and a call to support its mission to provide free, high-quality educational resources. The professor then recaps the previous lecture, where the derivative was defined as the slope of a tangent line, and basic derivative computations were discussed, including the derivatives of 1/x and x to the power of n. The focus shifts to exploring different interpretations of derivatives, emphasizing the importance of understanding the concept in various contexts, especially the rate of change, which is introduced as a key aspect of derivatives.
🔄 Derivative as Rate of Change and Physical Examples
The professor delves into the concept of derivatives as rates of change, illustrating this with the average rate of change formula delta y / delta x. This concept is clarified with examples from physics, such as current (dq/dt) and speed (ds/dt). A detailed example of instantaneous speed is given using the 'pumpkin drop' event at MIT, where the acceleration due to gravity is applied to calculate the height over time and the instantaneous speed at impact, highlighting the practical applications of derivatives in real-world scenarios.
🌡️ Derivatives in Non-Temporal Contexts and Sensitivity
The discussion expands to include derivatives in contexts beyond time and physics, such as temperature gradients (dT/dx) in weather prediction and the sensitivity of GPS measurements. The 'Flat Earth Model' of GPS is used to explain how horizontal distance (L) is deduced from the measured distance (h), and the importance of the derivative in estimating errors (delta L/delta h) is emphasized, showcasing the broad applicability of derivatives in various scientific and engineering disciplines.
🤔 Clarifying Derivatives and the Role of Limits
The lecture continues with a student's question about the equation for height, which the professor attributes to physics principles covered in another course. The focus then moves to the systematic approach of limits and continuity, which are foundational for deriving derivative formulas. The professor introduces 'easy limits' and contrasts them with the complexities involved in finding derivatives, where limits often involve indeterminate forms that require algebraic manipulation for resolution.
📉 Understanding Left-Hand and Right-Hand Limits
The concept of left-hand and right-hand limits is introduced to clarify the process of evaluating limits from different directions. A visual representation on a number line is used to distinguish between the two. The professor provides an example of a function defined differently on either side of a point, demonstrating how to calculate left and right limits and how they can differ, which is crucial for understanding continuity and discontinuity in functions.
🔗 Continuity and Its Relation to Limits
Continuity is defined as a function being equal to its limit at a given point, requiring both the existence of the limit and the function being defined at that point. The professor emphasizes the importance of evaluating left and right limits separately to ensure they are the same, which is a key aspect of a function's continuity. The difference between evaluating limits and plugging in the value directly is highlighted, setting the stage for a deeper exploration of discontinuous functions.
🚫 Exploring Types of Discontinuities
The lecture presents a 'zoo of discontinuities,' categorizing different types of non-continuous functions. Jump discontinuities, where left and right limits exist but are unequal, are explained, along with removable discontinuities, where the limits exist and are equal, but the function may be undefined at a point. Examples such as sin x / x and 1 - cos x / x are given to illustrate removable discontinuities, which can be 'fixed' by redefining the function at the problematic point.
∞ Infinite Discontinuities and Their Characteristics
Infinite discontinuities are introduced, where the function's values approach infinity or negative infinity as x approaches a certain point. The hyperbola y = 1 / x is used to demonstrate this, with the left and right limits approaching positive and negative infinity, respectively. The professor corrects common misconceptions about stating limits at infinity and emphasizes the importance of specifying the direction from which the limit is approached.
📉 Derivative Functions and Their Relationship to the Original Function
The professor discusses the relationship between a function and its derivative, using the example of y = 1 / x and its derivative y = -1 / x^2. It is highlighted that the derivative function does not necessarily resemble the original function, and intuitions about their behavior being similar are incorrect. The concept of odd and even functions and their derivatives is briefly touched upon, reinforcing the idea that the derivative can have different characteristics from the original function.
🛑 Other Discontinuities and the Theorem of Differentiability Implying Continuity
The lecture concludes with a mention of 'other ugly discontinuities,' such as y = sin(1 / x), which oscillate infinitely as x approaches zero, resulting in no left or right limits. The professor then introduces a fundamental theorem stating that differentiability implies continuity, providing a concise proof that leverages the existence of the derivative to show that the function must be continuous at a given point. The proof is explained in a way that clarifies common misconceptions about multiplying by zero in limits.
Mindmap
Keywords
💡Derivative
💡Rate of Change
💡Tangent Line
💡Limit
💡Continuity
💡Discontinuity
💡Instantaneous Speed
💡Average Speed
💡Temperature Gradient
💡Sensitivity of Measurements
Highlights
Introduction to the concept of a derivative as the slope of a tangent line.
Computational example of the derivative of 1/x being -1/x^2.
Derivative computation for x to the nth power, resulting in nx^(n-1).
Emphasis on the importance of understanding different interpretations of a derivative.
Derivative as a rate of change, with delta y/delta x representing the average rate of change.
Introduction of dy/dx as the notation for the instantaneous rate of change.
Physical interpretation of derivatives with examples like current (dq/dt) and speed (ds/dt).
Illustration of instantaneous speed using the MIT pumpkin drop event.
Calculation of the average and instantaneous speed of the pumpkin drop.
Differentiation between average and instantaneous speed and their relevance.
Examples of derivatives in contexts other than time, like temperature gradients.
Discussion on the sensitivity of measurements, with a simplified GPS model example.
Introduction to the concept of left-hand and right-hand limits.
Explanation of continuity and discontinuity in functions.
Types of discontinuities: jump, removable, and infinite discontinuities.
The theorem that differentiability implies continuity and its proof.
Clarification on the proper notation and understanding of limits, especially when dealing with infinity.
Differentiation between the derivative function and the original function in terms of their graphs.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: