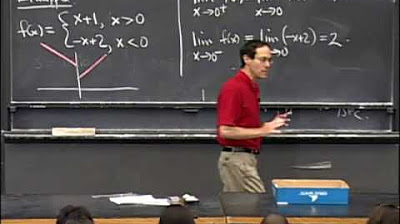Math 1325 - Lecture 9.1 - Intro to Calculus & Limits
TLDRIn this introductory lecture for Math 1325 Calculus for Business and Social Science, Professor Michael Bailey from Brookhaven College, Dallas, Texas, presents the two foundational concepts of calculus: instantaneous change and accumulated change. He explains that derivatives are used to measure instantaneous change, while integrals are employed for accumulated change. The lecture delves into the concept of limits, illustrating how they can be used to determine the value of a function as it approaches a certain point, with a focus on the notation and calculation of one-sided and full limits. Bailey also highlights the importance of continuity in functions and provides a method for calculating limits for continuous and discontinuous rational functions, including piecewise functions. The lecture concludes with examples that demonstrate how to apply these concepts in practice, offering students a solid foundation for further study in calculus.
Takeaways
- 📈 The two main concepts of calculus are instantaneous change (using derivatives) and accumulated change (using integrals).
- 📊 In algebra, the rate of change is the slope, calculated as the change in Y values over the change in X values.
- 🚗 The concept of a limit illustrates the value of Y as X approaches a certain value, using the notation lim (X→C) f(X) = L.
- 🔍 One-sided limits consider the value as X approaches a specific point from the left or right, denoted as lim (X→C⁺) and lim (X→C⁻).
- 🚫 If one-sided limits have different values, the full limit as X approaches a point does not exist.
- 🔢 Limits have several properties, such as the limit of a constant being the constant itself, and the limit of a sum or product being the sum or product of the limits.
- 🔁 A function is considered continuous if its graph can be drawn without lifting the pen from the paper.
- ✅ For continuous functions, the limit as X approaches a value C is the same as the function's value at C, i.e., f(C).
- 🔍 When calculating limits for rational functions, check that the denominator does not equal zero at the point of interest.
- 📉 If the denominator equals zero and the numerator does not, the limit does not exist (DNE).
- 📚 For piecewise functions, calculate one-sided limits and check if they exist and are equal to determine the full limit.
- 🔑 The lecture concludes with an emphasis on understanding the concept of limits and their role in calculus for precise calculations of change.
Q & A
What are the two fundamental concepts of calculus discussed in the lecture?
-The two fundamental concepts of calculus discussed are instantaneous change and accumulated change. Instantaneous change is about how things change at a precise moment, while accumulated change is about the result of change over time, such as more revenues coming in or more fluid being pumped into a tank.
What are derivatives and integrals used for in calculus?
-Derivatives are used to measure instantaneous change, while integrals are used to measure accumulated change.
What is the formula for the rate of change in algebra?
-In algebra, the rate of change is equal to the slope, which is calculated as the change in Y values over the change in X values, represented by the formula (y2 - y1) / (x2 - x1).
How is the limit defined in calculus?
-A limit in calculus is defined as the value of Y as you approach a value of X. It is notated as lim (X → C) f(X) = L, which is read as 'the limit as X approaches C of f(X) equals L'.
What is the concept of one-sided limits?
-One-sided limits refer to the value that a function approaches as X gets closer to a certain value from either the positive or negative direction. If the one-sided limits from both directions are not equal, the full limit does not exist.
What are the properties of limits?
-The properties of limits include: the limit of a constant is equal to the constant, the limit of X as X approaches C is equal to C, the limit of a sum or difference of functions is the sum or difference of their limits, the limit of a product is the product of the limits, the limit of a quotient is the quotient of the limits (provided the denominator's limit is not zero), and the limit of the nth root of a function is the nth root of the limit of that function.
How is a continuous function defined?
-A function is considered continuous if its graph can be drawn without lifting the pen from the paper, meaning there are no breaks, jumps, or holes in the graph.
What is the rule for calculating limits of rational functions?
-For rational functions, if the denominator does not equal zero when a certain value is plugged in, you can directly plug in the value into the numerator to find the limit. However, if the denominator equals zero and the numerator also equals zero at that value, further simplification or factoring may be required to determine the limit.
How do you calculate limits for piecewise functions?
-For piecewise functions, you calculate the one-sided limits as X approaches the interval mark from both the positive and negative directions. If both one-sided limits exist and are equal, then the full limit exists. If they are not equal, the full limit does not exist.
What does it mean if the one-sided limits of a function at a certain point are different?
-If the one-sided limits of a function at a certain point are different, it indicates that the function does not have a well-defined limit at that point. The full limit as X approaches that point does not exist.
Why is it important to check the domain of a rational function when calculating limits?
-Checking the domain of a rational function is important because you cannot divide by zero. If the value you are plugging into the function makes the denominator zero, the function is undefined at that point, and the limit may not exist or require further analysis.
Can you provide an example of a limit calculation using the concept of instantaneous change?
-An example of a limit calculation using instantaneous change could be finding the rate of change of the number of Internet users on a specific date, such as March 5th, 1993. While the average rate of change over a period might be known, calculus would be used to find the precise instantaneous change at that particular point in time.
Outlines
📚 Introduction to Calculus Concepts
Professor Michael Bailey introduces the course, Math 1325 Calculus for Business and Social Science, at Brookhaven College. He explains two fundamental concepts of calculus: instantaneous change, which is about how things change at a precise moment, and accumulated change, which is about the result of change over time. These concepts are represented by derivatives and integrals, respectively. The lecture also touches on the rate of change from algebra, which is related to the slope of a line. The professor uses the example of the growth of Internet users to illustrate the concept of average rate of change. The idea of a limit is introduced as a method to understand the value of a function as it approaches a certain point, which is crucial for calculating instantaneous rates of change.
🚗 Understanding Limits and One-Sided Limits
The lecture continues with a deeper exploration of limits, emphasizing the difference between the limit value as X approaches a specific point and the function's value at that point. One-sided limits are introduced, showing how the direction from which X approaches the point can affect the limit's value. The concept of a function being continuous is discussed, with examples of how to calculate limits when the function is continuous at a point. Properties of limits are listed, and the importance of understanding these properties for calculating limits is highlighted. The lecture also clarifies the notation and calculation methods for limits, including the limit of a constant, a variable, a sum or difference, a product, a quotient, and the nth root of a function.
🔢 Calculating Limits for Continuous and Discontinuous Functions
The third paragraph delves into the calculation of limits for continuous functions, explaining that if a function is continuous at a point, the limit as X approaches that point is the same as the function's value at that point. The professor provides methods for calculating limits for polynomial, rational, and piecewise functions. Special attention is given to rational functions, where the domain must be considered to ensure the denominator is not zero. The lecture also addresses how to handle cases where the function is discontinuous, such as when there are holes or jumps in the graph. Techniques for factoring and reducing fractions are discussed to find limits in certain scenarios, and the conditions under which a limit does not exist are explained.
🧩 Limits of Piecewise Functions and Conclusion
The final paragraph focuses on calculating limits for piecewise functions, which have different equations based on the value of X. The process involves checking one-sided limits as X approaches a specific point within the function's domain. The full limit exists only if both one-sided limits exist and are equal. The professor illustrates this with an example, showing how to calculate the limit as X approaches 1 from both the positive and negative directions using the appropriate piecewise function equation for each approach. The lecture concludes with the finding that if the one-sided limits are not equal, the full limit does not exist.
Mindmap
Keywords
💡Calculus
💡Instantaneous Change
💡Accumulated Change
💡Derivatives
💡Integrals
💡Limit
💡Continuous Function
💡One-Sided Limits
💡Polynomial Functions
💡Rational Functions
💡Piecewise Functions
Highlights
The two fundamental concepts of calculus are instantaneous change and accumulated change
Derivatives are used to measure instantaneous change, while integrals measure accumulated change
The rate of change in algebra is equal to the slope of a line
A limit illustrates the value of y as you approach a certain value of x
One-sided limits measure the value as you approach a point from the left or right direction
If one-sided limits have different values, the full limit does not exist
Limits have several properties including sum, difference, product, quotient, and nth root
A function is continuous if you can draw it without lifting your pencil from the paper
For a continuous function, the limit as x approaches a value is equal to the function value at that point
Most trigonometric, rational, and polynomial functions are continuous
To calculate limits for rational functions, check if the denominator equals zero at the point of interest
If the denominator equals zero, check if the numerator also equals zero for a potential limit
Factoring can help simplify rational functions and find limits
For piecewise functions, calculate one-sided limits from both directions and check if they are equal
A limit exists for a piecewise function only if both one-sided limits exist and are equal
This lecture covered key concepts of limits, continuity, and calculating limits for various types of functions
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: