Physics 37.1 Gauss's Law Understood (2 of 29) What is Electric Flux?
TLDRThis lecture delves into the concept of electric flux, defined as the product of the electric field strength, area, and the cosine of the angle between them. It illustrates the principle using examples with varying field strengths and areas, emphasizing the dependency of flux on these factors. The lecture clarifies that when the loop is perpendicular to the field, maximum flux is observed, dropping to zero when parallel, highlighting the importance of orientation in flux calculation.
Takeaways
- 📚 Electric flux is a concept that describes the amount of electric field passing through a given area.
- 🔍 The initial definition of electric flux is given when the area of the loop is perpendicular to the electric field.
- 📐 The formula for electric flux in this perpendicular case is the product of the electric field's magnitude and the area's magnitude.
- 📈 If the electric field is not perpendicular, the formula includes the cosine of the angle between the perpendicular to the loop and the direction of the electric field.
- 🌐 Examples are provided to illustrate how varying the strength of the electric field and the size of the area affects the electric flux.
- 🔢 A stronger electric field (2000 Newtons per Coulomb) with a larger area (5 square meters) results in a higher electric flux (10,000 Newtons per Coulomb times meter square).
- 🔎 A smaller area with the same strong electric field results in less flux (200 Newtons per Coulomb times square), showing the impact of area size.
- 🌀 Even a weak electric field (50 Newtons per Coulomb) can produce significant flux if the area is large enough (4000 Newtons per Coulomb times meter square).
- 🔄 When the area is small and the electric field is weak, the resulting flux is minimal (10 Newtons per Coulomb times meter square).
- 🧭 Rotating the loop so that it is perpendicular to the electric field direction results in zero flux due to the cosine of 90 degrees being zero.
- 🔑 The key to understanding electric flux is recognizing it as a measure of how much of the electric field passes through a given loop.
Q & A
What is the initial definition of electric flux discussed in the lecture?
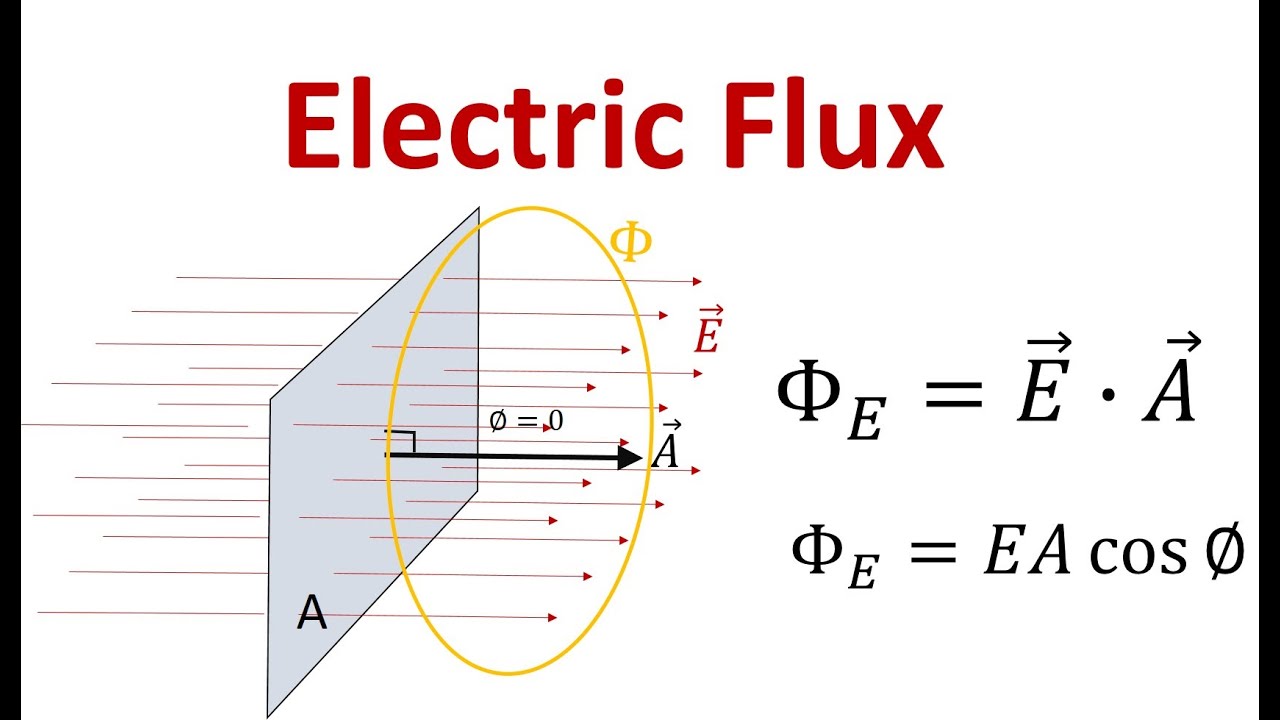
-The initial definition of electric flux is when a loop of area 'A' is placed perpendicular to an electric field 'E', the electric flux 'Φ' through the loop is defined as the product of the magnitude of the electric field and the magnitude of the area.
How is electric flux calculated when the electric field is not perpendicular to the loop?
-When the electric field is not perpendicular to the loop, the electric flux is calculated as the product of the electric field strength, the area of the loop, and the cosine of the angle between the perpendicular to the loop and the direction of the electric field.
What is the formula for calculating electric flux through a loop?
-The formula for calculating electric flux through a loop is Φ = E * A * cos(θ), where Φ is the electric flux, E is the electric field strength, A is the area of the loop, and θ is the angle between the electric field and the normal to the loop.
What is the electric field strength of the first example given in the lecture?
-The electric field strength of the first example is 2000 Newtons per Coulomb, and it is pointed in the x direction.
What happens to the electric flux if the area of the loop is very small with a strong electric field?
-If the area of the loop is very small with a strong electric field, only a few field lines will go through the area, resulting in a smaller electric flux through the loop.
How does the size of the loop affect the electric flux through it?
-The size of the loop affects the electric flux through it by determining how many electric field lines pass through the area. A larger loop will have more field lines passing through, resulting in a greater electric flux.
What is the electric field strength of the weaker example given in the lecture?
-The electric field strength of the weaker example is 50 Newtons per Coulomb, also pointing in the x direction.
What is the significance of the cosine term in the electric flux formula?
-The cosine term in the electric flux formula accounts for the angle between the electric field and the normal to the loop. It ensures that the flux is zero when the loop is parallel to the electric field and maximized when the loop is perpendicular to the field.
What happens to the electric flux if the loop is turned 90 degrees so that it is perpendicular to the electric field?
-If the loop is turned 90 degrees so that it is perpendicular to the electric field, the cosine of 90 degrees is zero, and there will be no flux going through the loop, as the electric field lines cannot penetrate the loop in this orientation.
How does the strength of the electric field and the size of the loop together determine the electric flux?
-The strength of the electric field and the size of the loop together determine the electric flux by affecting how many field lines pass through the area of the loop. A stronger field or a larger loop will result in a greater electric flux, assuming the loop is oriented to maximize the angle with the field.
Can the electric flux be zero even if there is an electric field?
-Yes, the electric flux can be zero if the loop is oriented such that it is parallel to the electric field, as the cosine of the angle (90 degrees) would be zero, resulting in no flux through the loop.
Outlines
🌐 Understanding Electric Flux Basics
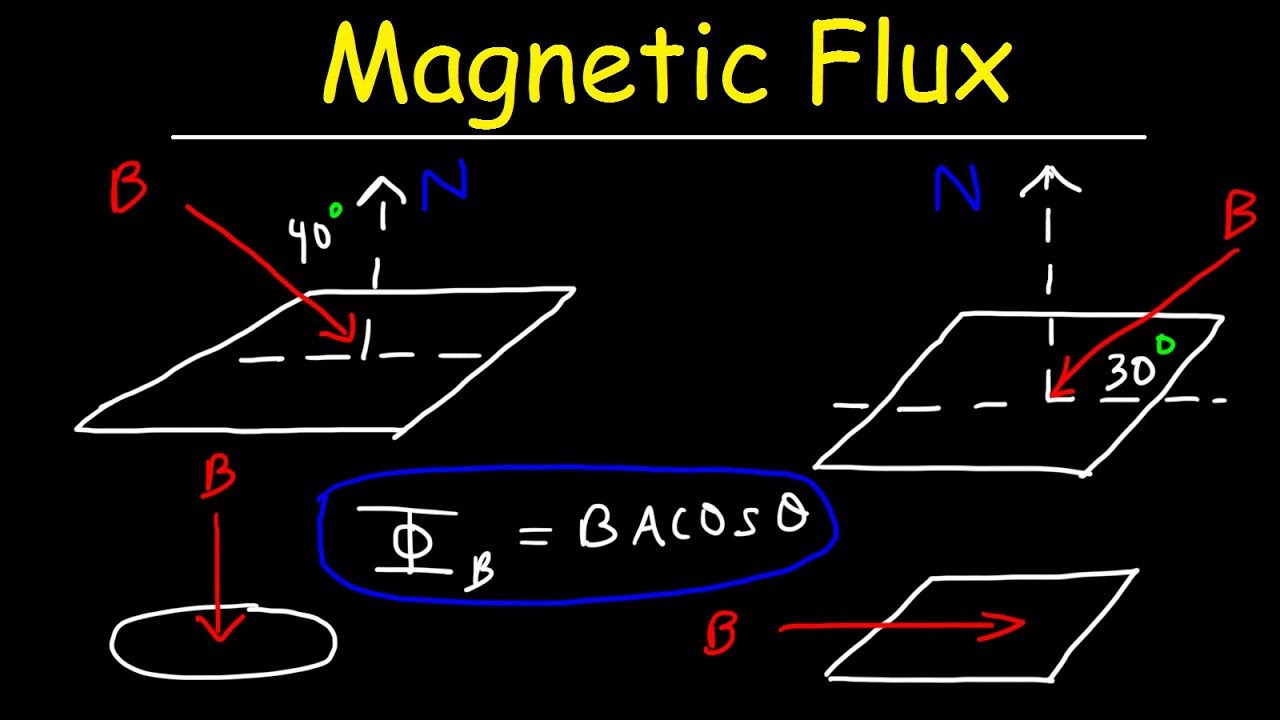
This paragraph introduces the concept of electric flux, explaining its definition and calculation. When a loop of area 'a' is perpendicular to an electric field, the electric flux is the product of the electric field's magnitude and the area's size. If the field and loop are not perpendicular, the calculation involves the cosine of the angle between them. The paragraph uses examples with varying field strengths and areas to illustrate how flux is affected by these factors, emphasizing that flux is maximized when the loop is perpendicular to the field and minimized or zero when parallel.
Mindmap
Keywords
💡Electric Flux
💡Electric Field
💡Area (A)
💡Perpendicular
💡Cosine (cos)
💡Newton per Coulomb (N/C)
💡Square Meter (m^2)
💡Field Lines
💡Angle
💡Loop
Highlights
Electric flux is defined as the product of the magnitude of the electric field and the area of a loop, assuming they are perpendicular to each other.
If the electric field and the loop are not perpendicular, the electric flux is calculated as the product of the electric field strength, area, and cosine of the angle between them.
A stronger electric field of 2000 Newtons per Coulomb was used in the first example.
A weaker electric field of 50 Newtons per Coulomb was also considered.
The size of the area affects the electric flux through the loop.
A larger area results in more electric flux even with the same electric field strength.
A smaller area with the same electric field results in less electric flux.
A large area with a small electric field can still have a significant amount of electric flux.
A small area with a small electric field results in minimal electric flux.
When the loop is turned 90 degrees, the cosine of the angle becomes zero, resulting in no electric flux.
The electric flux is zero when the loop is perpendicular to the electric field.
The concept of electric flux is associated with how much of the electric field goes through the loop.
Understanding electric flux helps in visualizing how electric field lines interact with a loop.
The examples provided illustrate the relationship between electric field strength, area size, and electric flux.
The lecture emphasizes the importance of perpendicularity between the electric field and the loop for calculating electric flux.
Cosine of the angle is a crucial factor in determining the electric flux when the field and loop are not perpendicular.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: