AP Calculus AB - 1.11 Defining Continuity at a Point

Daniel Bortnick

4 Oct 202019:35

EducationalLearning

32 Likes 10 Comments

TLDRIn this video, Mr. Bortnick explains the concept of continuity in calculus, focusing on defining continuity at a point. He breaks down the formal definition into three essential conditions that must all be met for a function to be considered continuous. The lesson includes practical examples of piecewise functions, illustrating how to determine if a function is continuous at specific points and identifying different types of discontinuities, such as holes, jumps, and step discontinuities. The video is designed to prepare students for AP Calculus exams, emphasizing the importance of understanding and applying the formal definition of continuity.

Takeaways

📘 The lesson is about defining continuity at a point in calculus, focusing on the formal definition of continuity.
📝 The informal definition of continuity is the ability to draw a function without lifting the pencil, but this is not mathematically rigorous.
🔍 For a function to be continuous at a point x=c, three conditions must be met: the function value f(c) must exist, the limit as x approaches c from both sides must exist, and the function value must equal the limit.
📉 The concept of limits from the left and right is crucial for determining continuity; they must converge to the same value as the function at the point x=c.
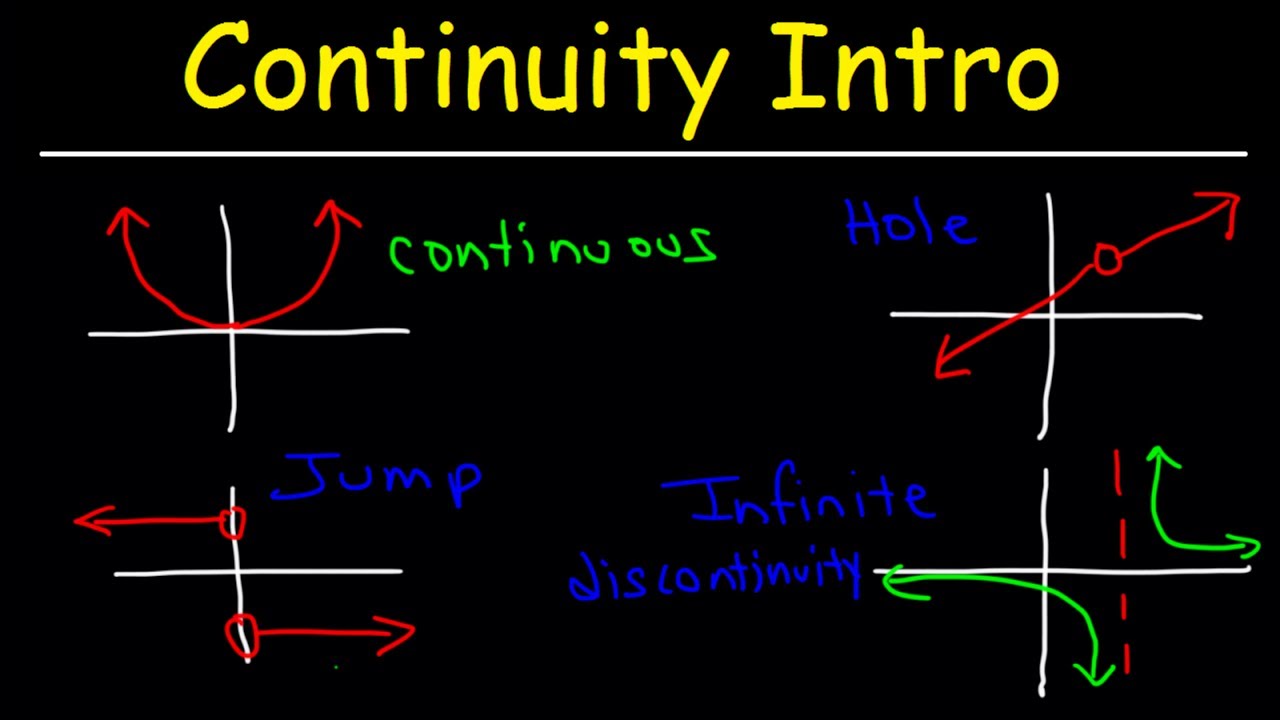
🚫 If any of the three conditions for continuity are not met, the function is considered discontinuous at that point.
🔑 The types of discontinuities include holes, vertical asymptotes, steps, or jumps, depending on which condition is violated.
📚 The script provides an example of a piecewise function and analyzes its continuity at specific points using the formal definition.
📐 The example demonstrates how to find the function value, the limit from the left, and the limit from the right to determine continuity.
🔢 A step-by-step process is shown to identify the type of discontinuity if the function is not continuous, such as a hole or a jump.
✍️ The importance of understanding and applying the formal definition of continuity is emphasized for success in AP Calculus exams.
📝 The lesson concludes with a problem to determine the value of k that would make a given piecewise function continuous at a specific point, illustrating the application of the continuity definition.

Q & A

What is the informal definition of continuity in calculus?
-The informal definition of continuity is the idea of being able to draw the function without lifting your pencil. If you can draw it without lifting your pencil, then it's considered continuous; if you have to lift the pencil, it's discontinuous.
What are the three conditions that must be met for a function to be considered continuous at a point?
-For a function to be continuous at a point x=c, the following three conditions must be met: 1) The function value f(c) must exist. 2) The limit as x approaches c of f(x) must exist. 3) The limit as x approaches c of f(x) must be equal to f(c).
What does it mean if the limit from the left and the limit from the right do not converge to the same y-value?
-If the limit from the left and the limit from the right do not converge to the same y-value, it indicates that the overall limit does not exist, and thus the function is not continuous at that point.
What is the definition of a piecewise function?
-A piecewise function is a function that is defined by different expressions over different domains or intervals of its independent variable. It consists of multiple 'pieces' of different functions.
How can you determine if a piecewise function is continuous at a specific point?
-To determine if a piecewise function is continuous at a specific point, you must check if the function value exists at that point, if the limit from both the left and the right exists and converges to the same value, and if the limit is equal to the function value at that point.
What type of discontinuity occurs if the function value and the limit as x approaches a point are not equal?
-If the function value and the limit as x approaches a point are not equal, the function has a 'hole' discontinuity at that point.
What is the significance of the value of 'k' in the context of making a function continuous?
-In the context of making a function continuous, the value of 'k' is determined by setting the limit from the left equal to the limit from the right at the point of interest, ensuring that the function value at that point equals the limit.
What is a step or jump discontinuity?
-A step or jump discontinuity occurs when the limit exists but the function value at the point of discontinuity is not equal to the limit, resulting in a 'step' or 'jump' in the graph of the function.
How can you find the value of 'k' that makes a function continuous at a specific point?
-To find the value of 'k' that makes a function continuous at a specific point, you set the function value at that point equal to the limit as x approaches that point from both the left and the right, and solve for 'k'.
What is the importance of understanding the formal definition of continuity in calculus?
-Understanding the formal definition of continuity is crucial for accurately determining the continuity of functions, which is a fundamental concept in calculus. It is also a common topic on exams like the AP Calculus exam.

Outlines

00:00

📚 Introduction to Continuity in Calculus

Mr. Bortnick introduces the concept of continuity in calculus, focusing on defining continuity at a point. He contrasts the informal 'no pencil lift' definition with the formal definition, which requires three conditions: the existence of the function at a point, the limit from both sides existing and converging to the same value, and the function value being equal to the limit. The lesson emphasizes the importance of understanding these conditions to determine if a function is continuous at a given point, and how the violation of any condition leads to different types of discontinuities.

05:01

🔍 Analyzing Continuity at Specific Points

The script continues with an in-depth analysis of a piecewise function's continuity at x = -1. It demonstrates how to apply the formal definition of continuity by checking if the function value exists, if the limit from both sides exists and is equal, and if the function value matches the limit. The conclusion is that the function is discontinuous at x = -1 due to the limit from the left and right not being equal, thus violating the second condition of continuity.

10:02

🔄 Continuity Check at x = 2 and Identifying Discontinuities

The video script proceeds to examine the continuity of the same piecewise function at x = 2. It shows that the function value and the limit from both sides exist and are equal, thus satisfying all conditions for continuity. The function is declared continuous at this point. The script then identifies a step or jump discontinuity at x = 4, where the limits from the left and right do not match, indicating a break in the second condition of continuity.

15:03

🔍 Continuity Analysis at x = -1 and Solving for a Continuous Function

The script revisits the analysis of continuity at x = -1 for a different piecewise function, confirming the existence of the function value and the limit. However, a discrepancy between the function value and the limit indicates a discontinuity due to the third condition being violated, which is identified as a hole. The final part of the script involves determining the value of 'k' that would make a given piecewise function continuous at x = -2. By setting the left and right limits equal to each other at this point, the value of 'k' is solved to be 6, ensuring the function's continuity.

Mindmap

Keywords

💡Continuity

Continuity in calculus refers to the property of a function that allows its graph to be drawn without lifting the pen from the paper, meaning there are no breaks, jumps, or holes at any point. In the video, the concept of continuity is explored through a formal definition involving three conditions that must be met for a function to be considered continuous at a point. The script uses the formal definition to analyze piecewise functions and determine their points of continuity and discontinuity.

💡Limit

A limit is a fundamental concept in calculus that describes the value that a function approaches as the input (x-value) approaches a certain point. The script discusses the importance of limits in determining continuity, stating that for a function to be continuous at a point, the limit as x approaches that point must exist and be equal to the function's value at that point.

💡Formal Definition

The formal definition in the context of the video is the precise mathematical criteria used to determine if a function is continuous at a point. It involves checking if the function's value at a point exists, if the limit exists as x approaches that point, and if the function's value equals the limit. The script emphasizes the importance of this definition in contrast to the informal notion of continuity.

💡Piecewise Function

A piecewise function is a function that is defined by multiple sub-functions, each applicable to a specific interval of the domain. In the video, the script uses piecewise functions as examples to illustrate the process of determining continuity at various points, highlighting how different parts of the function are evaluated based on the value of x.

💡Discontinuity

Discontinuity refers to points where a function is not continuous. The script explains that if any of the three conditions for continuity are not met, the function is discontinuous at that point. Types of discontinuities, such as holes, vertical asymptotes, steps, or jumps, are mentioned in relation to which condition is violated.

💡Domain

The domain of a function is the set of all possible input values (x-values) for which the function is defined. In the context of piecewise functions discussed in the script, the domain is broken into intervals, each corresponding to a different rule or sub-function that defines the function's behavior.

💡Function Value

The function value, or f(c), is the output of a function for a specific input value c. In the video, the script checks if the function value exists at a certain point c as part of the formal definition of continuity, ensuring there is a defined y-value or point on the graph at x=c.

💡Limit from the Left and Right

The concept of limits from the left and right refers to the behavior of a function as x approaches a certain point, but from values less than (left) or greater than (right) that point. The script uses these concepts to determine if the limit exists at a point, which is necessary for the function to be continuous there.

💡Hole Discontinuity

A hole discontinuity occurs at a point on the graph of a function where the function is defined at that point, and the limit exists, but the function value does not equal the limit. The script identifies this type of discontinuity in the context of the formal definition of continuity, where the third condition is not met.

💡Jump Discontinuity

A jump discontinuity is a type of discontinuity where the left and right limits exist but are not equal, resulting in a 'jump' in the function's value. The script describes this as a situation where the function has different values approaching the point from the left and right, breaking the second rule of continuity.

💡AP Exam

The AP Exam, or Advanced Placement Exam, is a series of standardized tests in the United States that high school students can take to earn college credit. The script mentions the AP Exam as it is tailored to students preparing for the AP Calculus exam, emphasizing the importance of understanding the formal definition of continuity for exam success.

Highlights

Introduction to the formal definition of continuity in calculus.

Explanation of the informal definition of continuity as drawing a function without lifting the pencil.

Three conditions required for a function to be considered continuous at a point.

Condition 1: The function value f(c) must exist.

Condition 2: The limit as x approaches c of f(x) must exist from both sides.

Condition 3: The function value at c must equal the limit as x approaches c.

Different types of discontinuities based on which condition is broken.

Analysis of a piecewise function for continuity at x = -1.

Finding f(-1) for the piecewise function and confirming its existence.

Checking the limit from the left and right of x = -1 and identifying discontinuity.

Conclusion that the function is discontinuous at x = -1 due to non-equal limits.

Analysis for continuity at x = 2, including checking the function value and limits.

Confirmation of continuity at x = 2 as all three conditions are met.

Identification of a step or jump discontinuity at x = 4 in the piecewise function.

Determination of a hole discontinuity at x = -1 for another piecewise function.

Problem-solving approach to find the value of k that makes a function continuous.

Setting up an equation to find k by equating the left and right limits at x = -2.

Solution for k to make the function continuous, with k = 6.

Encouragement for students to practice and check their answers before mastery checks.

Transcripts

Browse More Related Video

Calculus AB/BC – 1.11 Defining Continuity at a Point

Continuity!

Continuity

AP Calculus AB - 1.10 Exploring Types of Discontinuities

Continuity Basic Introduction, Point, Infinite, & Jump Discontinuity, Removable & Nonremovable

AP Calculus AB: Lesson 2.5 Differentiability