AP Calculus AB - 1.10 Exploring Types of Discontinuities

Daniel Bortnick
4 Oct 202019:59
EducationalLearning
32 Likes 10 Comments

TLDRIn this AP Calculus AB video, Mr. Bortnick explores the concept of discontinuities in functions, providing an informal definition of continuity as the ability to draw a function without lifting the pencil. He categorizes functions as either continuous or discontinuous and delves into types of discontinuities, including holes, vertical asymptotes, and jumps. Through examples and factoring, he teaches how to identify and categorize discontinuities, emphasizing the importance of the denominator in causing discontinuities. The lesson also covers trigonometric functions and the behavior of polynomials, aiming to help students master the topic.

Takeaways
  • 📝 Today's topic is exploring types of discontinuities in functions.
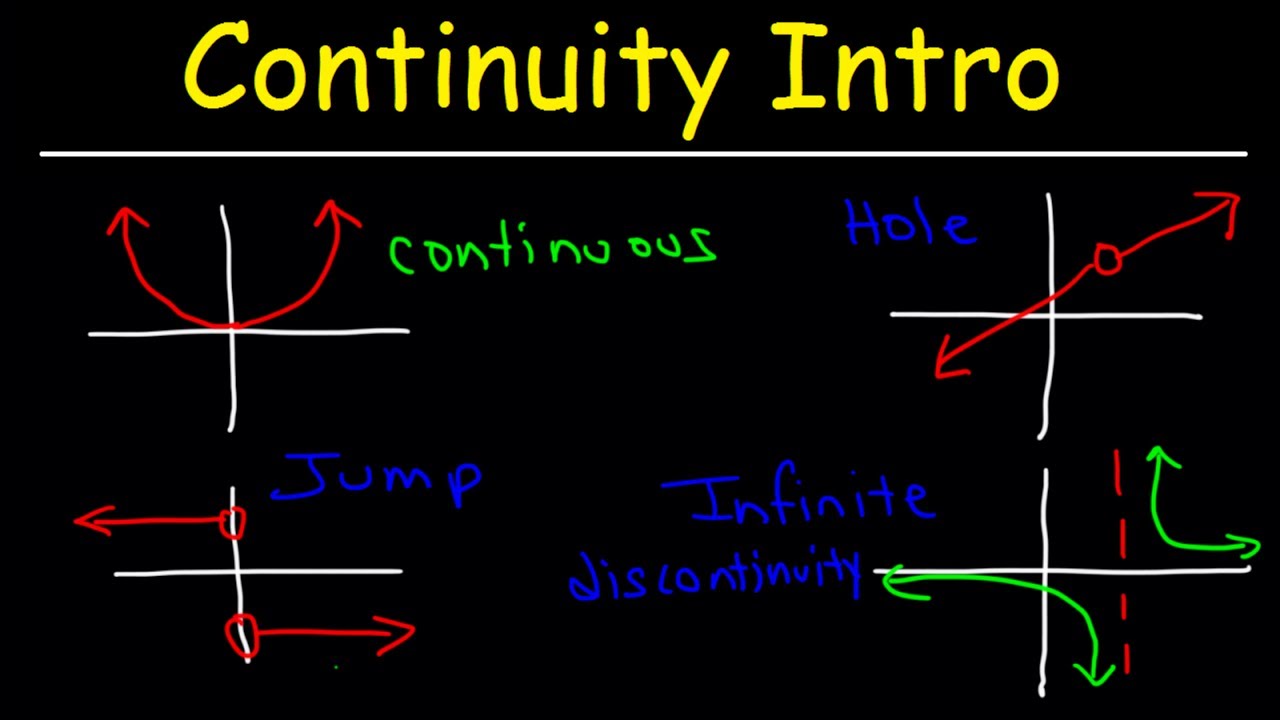
  • 🔄 Informal definition of continuity: a function is continuous if it can be drawn without lifting the pencil.
  • ✅ A function can be continuous or discontinuous on different intervals of its domain.
  • 📉 Example of a continuous function: one that can be drawn from start to end without lifting the pencil.
  • 🚫 Example of a discontinuous function: one that requires lifting the pencil due to an asymptote or break.
  • 🔍 Types of discontinuities: holes (removable), vertical asymptotes (non-removable), and skips/jumps (non-removable).
  • 🔗 Factors canceling in a function's fraction indicate a hole (removable discontinuity).
  • 🚧 Non-canceling factors in a function's fraction indicate a vertical asymptote (non-removable discontinuity).
  • 🔄 In trigonometric functions like tan(x), discontinuities occur where the cosine is zero.
  • 📉 Polynomial functions without denominators are generally continuous and well-behaved.
Q & A

  • What is the informal definition of continuity as described in the script?

    -The informal definition of continuity is the ability to draw a function without lifting up your pencil, indicating that the function is either continuous or discontinuous based on this criterion.

  • What are the three types of discontinuities mentioned in the script?

    -The three types of discontinuities mentioned are: a hole in the function, a vertical asymptote, and a skip or jump in the graph.

  • What is a removable discontinuity and how is it different from a non-removable discontinuity?

    -A removable discontinuity is a hole in the function that can be 'filled in' to make the function continuous, whereas a non-removable discontinuity, such as a vertical asymptote or a skip/jump, cannot be fixed by simply filling in a point.

  • How can you determine if a discontinuity is a hole or a vertical asymptote when factoring a function?

    -If a factor in the denominator cancels with a factor in the numerator, it results in a hole. If the factor in the denominator does not cancel with any factor in the numerator, it results in a vertical asymptote.

  • What is the significance of the denominator in determining discontinuities in a function?

    -The denominator is significant because a discontinuity occurs whenever the denominator equals zero, as division by zero is undefined, which can lead to a hole, vertical asymptote, or other types of discontinuities.

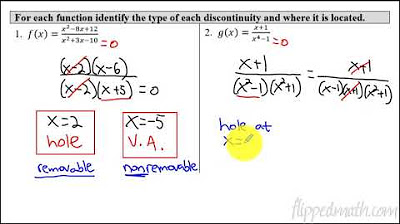
  • Why is the function x^2 - 8x + 12 / (x^2 + 3x - 10) discontinuous at x = -5 and x = 2?

    -The function is discontinuous at x = -5 and x = 2 because these values make the denominator equal to zero, resulting in a vertical asymptote at x = -5 and a hole at x = 2 due to the cancellation of the (x - 2) factor in the numerator.

  • How does the script suggest factoring the function g(x) = (x + 1) / (x^4 - 1) to identify discontinuities?

    -The script suggests using the difference of squares formula to factor x^4 - 1 into (x^2 + 1)(x^2 - 1), and further factor x^2 - 1 into (x + 1)(x - 1), which helps identify discontinuities at x = -1 and x = 1.

  • What trigonometric identity is used in the script to rewrite the function h(x) = tan(2x) and why?

    -The identity tan(x) = sin(x) / cos(x) is used to rewrite h(x) as sin(2x) / cos(2x) to identify the points where the cosine function equals zero, which would indicate discontinuities in the tangent function.

  • Why are polynomial functions without denominators considered 'well-behaved' in terms of continuity?

    -Polynomial functions without denominators are considered 'well-behaved' because they do not have discontinuities such as holes or vertical asymptotes, and they represent smooth curves without breaks.

  • What is the significance of the unit circle in identifying discontinuities in trigonometric functions like tan(x)?

    -The unit circle is significant because it helps identify the angles at which trigonometric functions like cosine are equal to zero, which in turn helps determine the vertical asymptotes in the graph of the tangent function.

  • What is the purpose of the practice problems and mastery check mentioned in the script?

    -The practice problems and mastery check are meant to reinforce the understanding of the concepts taught in the script, specifically the identification and categorization of different types of discontinuities in functions.

Outlines
00:00
📚 Introduction to Continuity and Discontinuities

Mr. Bortnick introduces the concept of continuity and discontinuities in calculus, focusing on the informal definition of continuity as the ability to draw a function without lifting the pencil. He distinguishes between continuous and discontinuous functions and hints at the mathematical definition to be covered in upcoming lessons. The instructor uses visual examples to illustrate continuous and discontinuous functions, including those with holes, vertical asymptotes, and jumps, categorizing them into removable and non-removable discontinuities.

05:04
🔍 Exploring Types of Discontinuities

The video delves deeper into the types of discontinuities, explaining vertical asymptotes as non-removable discontinuities that prevent drawing a function without lifting the pencil. The instructor provides a rule for identifying discontinuities by examining the factors in the numerator and denominator of a function. If factors cancel out, it results in a hole or removable discontinuity; otherwise, it indicates a vertical asymptote or non-removable discontinuity. The lesson includes an example of identifying discontinuities in a given function by factoring and analyzing the resulting equation.

10:05
📘 Factoring to Determine Discontinuities

Continuing the discussion on discontinuities, Mr. Bortnick emphasizes the importance of factoring to determine whether a discontinuity is a hole or a vertical asymptote. He provides a step-by-step guide on how to factor expressions and use the resulting factors to identify the type of discontinuity. The video includes examples of functions with discontinuities and demonstrates the process of factoring to find the points where the discontinuities occur.

15:06
📐 Trigonometric Functions and Discontinuities

The instructor introduces the concept of discontinuities in trigonometric functions, specifically focusing on the tangent function. By rewriting the tangent function in terms of sine and cosine, the video explains how to identify vertical asymptotes in trigonometric functions. The lesson includes an example of finding discontinuities in the tangent function by setting the denominator equal to zero and solving for the angle, which corresponds to the x-values where discontinuities occur.

📈 Polynomial Functions and Their Behavior

In the final part of the script, Mr. Bortnick discusses the behavior of polynomial functions without denominators, which are typically continuous and well-behaved. He explains that these functions do not have holes or vertical asymptotes and are characterized by their smooth, parabolic shapes. The instructor provides a brief overview of identifying continuous functions and encourages students to practice the concepts covered in the lesson and complete a mastery check.

Mindmap
Keywords
💡Continuity
Continuity in calculus refers to the property of a function that allows it to be drawn without lifting the pencil from the paper. It is a fundamental concept in understanding the behavior of functions. In the video, the instructor uses an informal definition of continuity to describe functions that can be drawn without interruption, contrasting them with discontinuous functions that require lifting the pencil, indicating breaks or gaps in the function's graph.
💡Discontinuities
Discontinuities are points on a function where the function is not defined or where the function does not have a limit. They represent breaks in the graph of a function. The video discusses different types of discontinuities, such as holes, vertical asymptotes, and jumps, which are essential for understanding the limitations of a function's domain and its behavior at certain points.
💡Removable Discontinuity
A removable discontinuity occurs at a point where the function is not defined, but the discontinuity can be 'removed' by redefining the function's value at that point. In the context of the video, the instructor explains that holes in a function's graph are examples of removable discontinuities because filling in the hole would result in a continuous function.
💡Non-Removable Discontinuity
Non-removable discontinuities are points where the function cannot be redefined to achieve continuity. Examples given in the video include vertical asymptotes and jumps, which cannot be 'filled in' to make the function continuous. These discontinuities are inherent to the function's definition and cannot be eliminated by simply reassigning a value.
💡Vertical Asymptote
A vertical asymptote is a line that the graph of a function approaches but never crosses, typically occurring at values where the function's denominator is zero (and the numerator is not zero). The video script uses vertical asymptotes to illustrate non-removable discontinuities, explaining that they create a barrier that prevents the function from being defined at certain points.
💡Factoring
Factoring is the process of breaking down a polynomial into a product of its factors. In the video, the instructor emphasizes the importance of factoring in identifying discontinuities, as it helps determine whether factors in the denominator cancel out with those in the numerator, which in turn indicates whether a discontinuity is a hole or a vertical asymptote.
💡Hole
A hole in the context of calculus is a type of removable discontinuity where the function is not defined at a particular point, but the surrounding function values are such that the point could be included without affecting the function's overall behavior. The video script provides examples of holes and explains how they can be identified through factoring and the cancellation of terms.
💡Polynomial Functions
Polynomial functions are algebraic expressions involving a sum of terms with variables raised to non-negative integer powers. The video mentions that polynomial functions without denominators are typically 'well-behaved,' meaning they are continuous and do not exhibit discontinuities such as holes or vertical asymptotes.
💡Tangent Function
The tangent function, represented as tan(x), is a trigonometric function defined as the ratio of the sine of an angle to the cosine of the same angle. In the video, the instructor discusses the tangent function in the context of identifying discontinuities, noting that the function becomes undefined when the cosine of the angle is zero, leading to vertical asymptotes.
💡Unit Circle
The unit circle is a circle with a radius of one, centered at the origin of a coordinate system. It is used in trigonometry to define and understand the sine and cosine functions. The video script refers to the unit circle when discussing the tangent function, explaining that the cosine function has zeros at certain angles, which correspond to vertical asymptotes in the graph of the tangent function.
Highlights

Introduction to the concept of continuity and informal definition: A function is continuous if it can be drawn without lifting the pencil.

Differentiation between continuous and discontinuous functions based on the ability to draw the function without interruption.

Exploration of discontinuities, including removable and non-removable types, with examples provided.

Removable discontinuities are characterized by holes in the graph, which can be 'filled in' to restore continuity.

Non-removable discontinuities include vertical asymptotes and skip/jumps, which cannot be corrected by filling in points.

Identification of discontinuities through factoring and analyzing the numerator and denominator of a function.

Rule for determining if a discontinuity is a hole or a vertical asymptote based on factor cancellation.

Application of the difference of squares factoring technique to identify discontinuities.

Use of trigonometric identities to rewrite and analyze functions for potential discontinuities.

Understanding that the cosine function equals zero at specific angles, leading to vertical asymptotes in tangent functions.

Identification of vertical asymptotes in the tangent function by setting the cosine of 2x to zero.

Explanation of why polynomial functions without denominators are considered 'well-behaved' and continuous.

Emphasis on the importance of factoring in determining the type and location of discontinuities.

Instruction on how to identify and categorize discontinuities in given functions through examples.

Note on the upcoming video providing unit circle tips and tricks for better understanding of trigonometric functions.

Conclusion of the lesson with a reminder to complete practice problems and a mastery check for the topic.

Transcripts

Browse More Related Video

Calculus AB/BC – 1.10 Exploring Types of Discontinuities

AP Calculus AB - 1.11 Defining Continuity at a Point

AP Calculus AB - 1.14 Connecting Infinite Limits and Vertical Asymptotes

Continuity!

Continuity

Continuity Basic Introduction, Point, Infinite, & Jump Discontinuity, Removable & Nonremovable

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusDiscontinuitiesContinuityAP LessonsMath EducationVertical AsymptoteRemovable DiscontinuityNon-Removable DiscontinuityTrigonometryWell-Behaved Functions