Continuity Basic Introduction, Point, Infinite, & Jump Discontinuity, Removable & Nonremovable
TLDRThe video script discusses the concept of continuity in functions, highlighting different types of discontinuities such as holes, jump, and infinite discontinuities. It explains that holes are removable while jumps and infinite discontinuities are not. The script provides methods for identifying points of discontinuity in graphs, especially focusing on rational functions and piecewise functions. It also demonstrates how to determine the constant values that make a function continuous at specific points, using examples and step-by-step problem-solving approaches.
Takeaways
- 📈 A graph is considered continuous if there are no jumps or breaks.
- 🚫 Discontinuities can be of three types: hole, jump, and infinite.
- 🕳️ Hole discontinuity is removable, unlike jump and infinite discontinuities.
- 🔍 To find points of discontinuity in a rational function, set the denominator to zero to find vertical asymptotes.
- 📏 Vertical asymptotes indicate infinite discontinuities where the function approaches positive or negative infinity.
- 🔄 cancelling factors in a function can lead to a hole discontinuity.
- 🔢 For piecewise functions, check if the y-values are the same at the points of potential discontinuity to determine continuity.
- 🌐 Linear, quadratic, and cubic functions are continuous, as are all polynomial functions.
- 🔄 To make a function continuous at a certain point, the y-values of the different parts of the function must be equal at that point.
- 🔢 Solving for constants in piecewise functions involves setting the different parts of the function equal to each other at points of discontinuity.
Q & A
What are the three types of discontinuities mentioned in the transcript?
-The three types of discontinuities mentioned are hole discontinuity, jump discontinuity, and infinite discontinuity.
What is a removable discontinuity?
-A removable discontinuity is a type of discontinuity that can be fixed by performing a limited operation, such as canceling out common factors. The hole discontinuity is an example of a removable discontinuity.
How can you identify a point of discontinuity in a rational function?
-You can identify a point of discontinuity in a rational function by setting the denominator equal to zero, which will give you the vertical asymptote, indicating an infinite discontinuity.
What is the difference between a jump discontinuity and an infinite discontinuity?
-A jump discontinuity occurs when the left and right sides of the graph do not connect, while an infinite discontinuity occurs when the function approaches positive or negative infinity as it approaches a vertical asymptote.
How can you determine if a discontinuity is removable or non-removable?
-A discontinuity is removable if it can be fixed by performing a limited operation like canceling out common factors. If it cannot be fixed, it is non-removable, such as jump and infinite discontinuities.
What happens when a function has a piecewise definition with different expressions for different intervals?
-A function with a piecewise definition can have points of discontinuity at the boundaries between the intervals. The continuity at these points can be determined by checking if the function's values are the same from both sides of the point.
How do you find the value of a constant to make a piecewise function continuous at a specific point?
-To find the value of a constant for a piecewise function to ensure continuity at a specific point, you set the expressions of the function from both sides equal to each other at that point and solve for the constant.
What are the characteristics of linear, quadratic, and cubic functions regarding continuity?
-Linear, quadratic, and cubic functions are all polynomial functions, which means they are continuous everywhere.
What is the role of absolute value functions in creating discontinuities?
-Absolute value functions can create jump discontinuities in a graph because they can result in the function having different values (positive or negative) depending on the sign of the variable.
How can you identify vertical asymptotes in a function?
-Vertical asymptotes can be identified by setting the denominator of a rational function equal to zero, as this will be the x-values where the function is undefined, indicating a point of infinite discontinuity.
What is the process to determine the points of discontinuity in a piecewise function?
-To determine the points of discontinuity in a piecewise function, you first identify the x-values where the function's definition changes. Then, you check if the function's values are the same immediately before and after these points to see if there is a jump or hole discontinuity.
Outlines
📊 Understanding Discontinuities in Functions
This paragraph discusses the concept of continuity in graphs, highlighting different types of discontinuities such as holes, jump discontinuities, and infinite discontinuities. It explains that holes are removable while jump and infinite discontinuities are not. The paragraph further provides methods for identifying points of discontinuity in a graph, using examples of rational functions and vertical asymptotes to illustrate infinite discontinuities. It also touches on removable discontinuities in the context of canceling factors and the presence of absolute value functions.
🔢 Analyzing Piecewise Functions for Discontinuities
The second paragraph delves into piecewise functions, explaining how to determine points of discontinuity. It mentions that linear, quadratic, and cubic functions are always continuous, while rational functions may have discontinuities when the denominator is zero. The paragraph uses a piecewise function as an example to demonstrate how to find discontinuities at specific x-values by comparing y-values on either side of the potential points of discontinuity. It also addresses the concept of making a function continuous at certain points by adjusting constants.
🧩 Solving for Constants in Piecewise Functions
This paragraph focuses on solving for constants in piecewise functions to ensure continuity at specific points. It presents a methodical approach to finding the values of constants that make the function continuous at given x-values. The paragraph provides a step-by-step explanation of the process, including setting equations equal to each other, substituting x-values, and solving for the constants. It uses multiple examples to illustrate the process, emphasizing the importance of matching y-values for continuity and highlighting the solutions for constants a and b in different scenarios.
Mindmap
Keywords
💡Continuity
💡Discontinuity
💡Vertical Asymptote
💡Rational Function
💡Hole Discontinuity
💡Jump Discontinuity
💡Infinite Discontinuity
💡Piecewise Function
💡Removable Discontinuity
💡Non-removable Discontinuity
💡Polynomial Function
Highlights
The graph discussed is continuous everywhere, with no jumps or breaks.
A point of discontinuity at x value c is introduced as a whole discontinuity.
Jump discontinuity is characterized by the left and right sides not connecting.
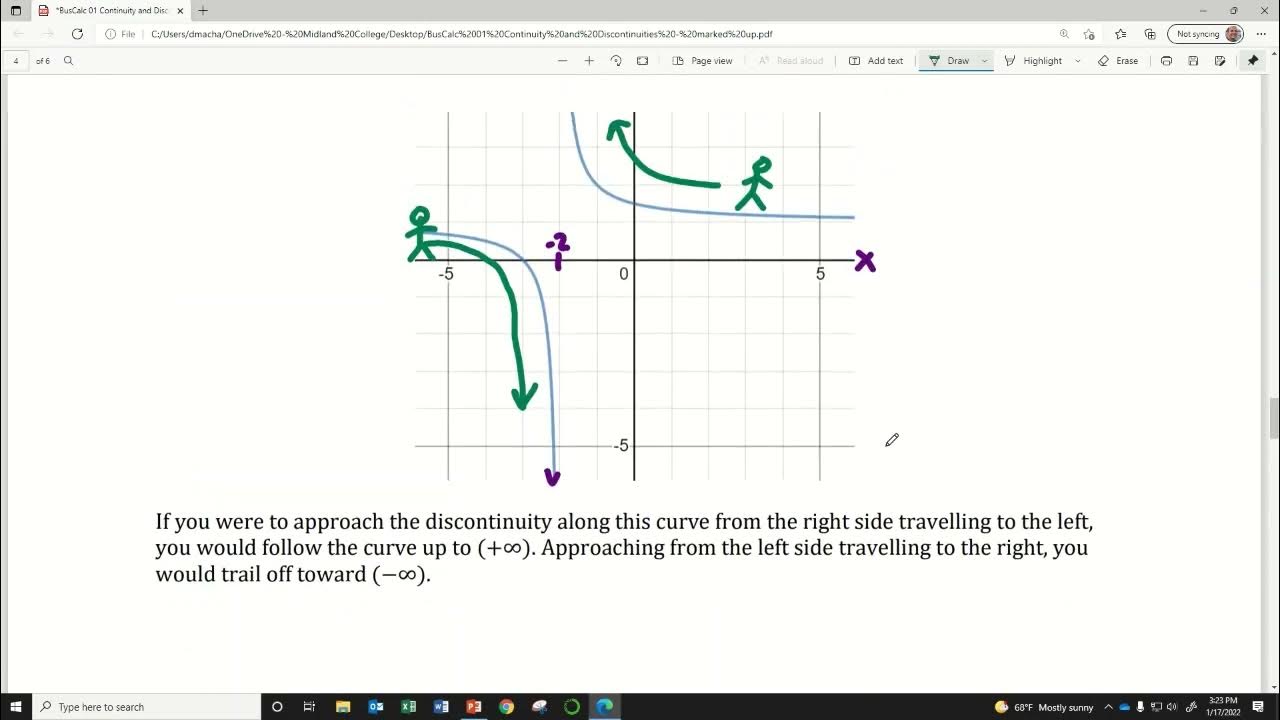
Infinite discontinuity occurs as one approaches a vertical asymptote, with the function approaching positive or negative infinity.
Hole discontinuity is removable, unlike jump and infinite discontinuities which are non-removable.
For rational functions, vertical asymptotes and thus points of discontinuity are found by setting the denominator equal to zero.
The function 1/x^2 has a vertical asymptote at x=0, marking a point of discontinuity due to being undefined.
For the function 5/(x+2), x cannot be -2, indicating a vertical asymptote and a point of discontinuity.
The function 3x + 2/(x + 2)(x - 5) has vertical asymptotes where x cannot equal 5, leading to infinite discontinuity.
The discontinuity type for f(x) = |x|/x is a jump discontinuity, occurring due to the absolute value function.
Jump discontinuities are non-removable and can also arise from piecewise functions.
Linear, quadratic, and cubic functions, as well as any polynomial functions, are always continuous.
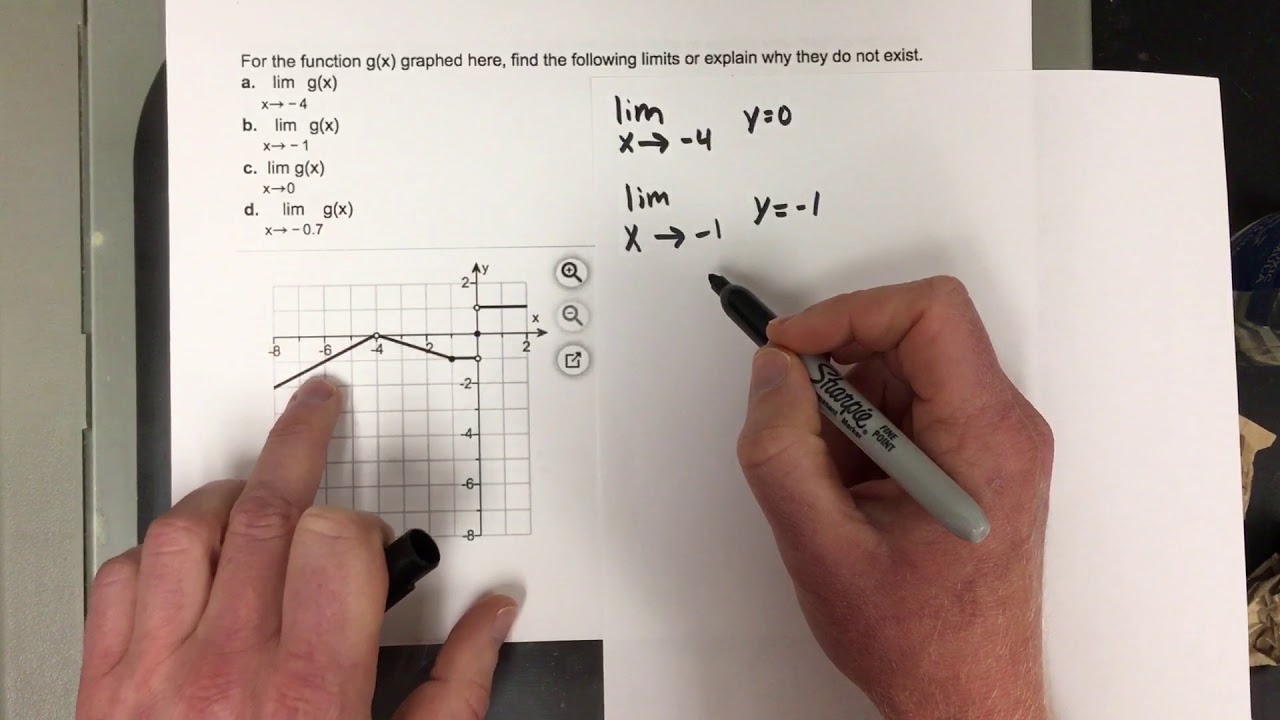
For the piecewise function given, discontinuity points are identified at x values of 1 and 2 by comparing y values.
At x=1, the function is discontinuous due to different y values in the two segments.
At x=2, the function is continuous because the y values from both segments are equal.
For the function f(x) = cx + 3 for x < 2 and 3x + c for x ≥ 2, c is found to be 3 for continuity at x=2.
In another example, for the function with different expressions for x < 3 and x ≥ 3, the constant a is found to be 2 for continuity.
For the function with expressions changing at x=1, x=4, and x≥4, the constants a and b are determined to be 2 and 3, respectively, for continuity across these points.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: