Integrating Exponential Functions

Chad Gilliland
2 Dec 201313:37
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script covers the integration of exponential functions involving 'e'. It begins with the fundamental derivatives of exponential functions and progresses to substitution techniques, demonstrating how to integrate various forms of 'e' to the power of a variable. The script provides several examples, including indefinite integrals and definite integrals over specific intervals, illustrating the process step by step. It also touches on solving differential equations and calculating the volume of water pumped into a tank, offering a comprehensive guide to integrating exponential functions.

Takeaways
  • 📈 The derivative of e^x is e^x.
  • 🧮 For e^u, the derivative is e^u * u'.
  • 🔄 The integral of e^u * du is e^u + C.
  • 🧑‍🏫 Example 1: Let u = 3x + 1. The integral of e^{3x+1} is (1/3) e^{3x+1} + C.
  • 📚 Example 2: Let u = -x^2. The integral of e^{-x^2} is -(5/2) e^{-x^2} + C.
  • 🧩 Example 3: Let u = x^{-1}. The integral of e^{x^{-1}} is -e^{x^{-1}} + C.
  • 📐 Example 4: Let u = cos x. The integral of e^{cos x} is -e^{cos x} + C.
  • 📊 Example 5: For a definite integral from 0 to 1, substitute the limits after finding the integral.
  • 🔍 Example 6: Use substitution u = cos(e^x) for complex integrals.
  • 🧮 For solving differential equations, integrate both sides and use initial conditions to find constants.
Q & A

  • What is the derivative of e to the power of x with respect to x?

    -The derivative of e to the power of x (e^x) with respect to x is e^x itself.

  • If the derivative of a function is e to the power of u times u prime, what is the integral of e to the power of u with respect to u?

    -The integral of e to the power of u (e^u) with respect to u is e^u plus a constant C.

  • In the context of the script, what is the substitution method used for integrating exponential functions?

    -The substitution method involves letting u be an expression involving x, finding du/dx, and then solving for dx in terms of du, which allows for the integral of e^u to be simplified and solved.

  • What is the integral of e to the power of (3x + 1) with respect to x?

    -The integral of e^(3x + 1) with respect to x is (1/3)e^(3x + 1) plus a constant C.

  • How is the integral of e to the power of (-x^2) simplified in the script?

    -The integral of e^(-x^2) is simplified by taking out the constant coefficient 5, resulting in -5/2 times the integral of e^(-x^2) times dx, which then becomes -5/2 * e^(-x^2) plus a constant C.

  • What is the integral of e to the power of (1/x) with respect to x?

    -The integral of e^(1/x) with respect to x is -e^(1/x) plus a constant C, after substituting u = 1/x and solving for dx in terms of du.

  • How is the integral of e to the power of cos(x) with respect to x approached in the script?

    -The integral of e^(cos(x)) with respect to x is approached by substituting u = cos(x), which gives du/dx = -sin(x), and then solving for dx in terms of du, resulting in the integral of -e^u * du, which simplifies to -e^(cos(x)) plus a constant C.

  • What is the process for solving a definite integral with a natural logarithm in the denominator, as shown in the script?

    -The process involves substituting the bottom of the fraction with u, finding du/dx, and then solving for dx in terms of du. The integral is then evaluated from the upper to the lower limit of integration, and the fundamental theorem of calculus is applied to find the difference between the evaluated limits.

  • How does the script handle the integration of the product of cosine and an exponential function?

    -The script suggests substituting u with the argument of the cosine function, finding du/dx, and then solving for dx in terms of du. The integral of the product becomes the integral of cosine(u) times du, which simplifies to the sine of u evaluated from the limits of integration.

  • What is the approach to solving a differential equation of the form dy = e^x - e^(-x) dx?

    -The approach involves separating variables and integrating both sides. The integral of dy is y, and the integral of the right-hand side involves recognizing the product of e^x and e^(-x) as e^(2x)/2, which is then integrated term by term.

  • How is the particular solution to a differential equation found in the script?

    -The particular solution is found by integrating the function to find the first derivative, then integrating again to find the original function, and finally applying the initial condition to determine the constant of integration.

  • What is the method for calculating the total amount of water pumped into a tank over a certain time period, as described in the script?

    -The method involves integrating the rate function R(t) from time 0 to the desired time period (in this case, 5 minutes). The result of this integration gives the total change in the amount of water in the tank.

Outlines
00:00
📘 Introduction to Integrating Exponential Functions

The speaker introduces the topic of integrating exponential functions involving the constant e. They recap the derivatives of e^x and e^u, emphasizing the formula for integration. They proceed with an example where u is set to 3x + 1, showing the step-by-step process to solve for the integral, ultimately arriving at the solution 1/3 e^(3x + 1) + C.

05:02
📝 Further Examples of Integration

The speaker continues with more examples of integrating exponential functions. In the first example, they set u to -x^2 and demonstrate the integration process, leading to -5/2 e^(-x^2) + C. Another example involves setting u to x^-1, and they detail the steps to integrate e^(x^-1) using substitution, resulting in -e^(x^-1) + C.

10:02
🔍 Complex Integrals and Definite Integrals

The speaker tackles more complex integrals, showing how to handle integrals involving fractions and trigonometric functions. They explain the process of letting u equal the exponent on e and provide detailed steps for substitution and integration. An example with definite integrals is given, demonstrating how to handle limits of integration and evaluate the result.

Mindmap
Keywords
💡Integration
Integration in calculus is the process of finding a function given its derivative, which is the opposite of differentiation. In the video, integration is the main theme, with the focus on integrating exponential functions involving 'e'. For example, the script mentions 'if I'm gonna DDX e to the u I'm going to get as my answer e to the U times du DX', illustrating the process of integrating an exponential function.
💡Exponential Functions
Exponential functions are mathematical functions where the variable is in the exponent. The script discusses how to integrate such functions, especially those with the base 'e'. The video uses the formula 'e to the U plus C' to represent the antiderivative of an exponential function, as seen in the example 'integral of e to the U D u'.
💡Derivative
A derivative in calculus represents the rate of change of a function. The script starts with the knowledge that 'the derivative of e to the X is e to the X', setting the foundation for understanding how to integrate exponential functions, as derivatives are the inverse process of integration.
💡U-Substitution
U-substitution is a technique used in calculus to simplify the integration of complex functions by substituting a new variable 'u' for a part of the original function. The script uses this method multiple times, such as when 'u equals 3x plus 1' to simplify the integral and find 'd u DX', which is a crucial step in the integration process.
💡Antiderivatives
Antiderivatives are functions that represent the reverse process of differentiation. The script mentions 'our answer, for the antiderivative is e to the U, plus C', indicating that the process of finding antiderivatives is central to the video's theme of integrating exponential functions.
💡Natural Logarithm
The natural logarithm is the logarithm to the base 'e' and is denoted as 'ln'. In the script, the natural logarithm is used in the context of definite integrals, such as 'the natural log of the absolute value of the bottom', to find the volume of water pumped into a tank over a time interval.
💡Definite Integrals
Definite integrals calculate the accumulated value of a function over a specified interval. The video script includes examples of definite integrals, like 'the integral from 2 to 1 plus e', to solve practical problems such as the total amount of water pumped into a tank within a certain time frame.
💡Differential Equations
Differential equations are equations that involve derivatives of a function. The script touches on solving differential equations, as seen in 'solve the differential equation', where the process involves integrating both sides to find the function that satisfies the given equation.
💡Initial Condition
An initial condition provides a starting point for solving differential equations. The script mentions 'satisfies the initial condition', which is crucial for finding a particular solution to a differential equation, such as determining the function 'f of X' that fits the given conditions.
💡Rate of Change
The rate of change is a measure of how quickly a quantity is changing. In the context of the script, 'the rate at which water is being pumped into a tank' is given by the function 'R of T', and the total change in the quantity of water over time is found by integrating this rate function.
Highlights

Introduction to integrating exponential functions with base 'e', emphasizing the derivative of e^x as e^x.

Explanation of the derivative of e^u as e^u * u', where u is a function of x.

Integration formula for e^u du, resulting in e^u + C.

Practice example with u = 3x + 1, illustrating the substitution method.

Solving for dx in terms of du/dx, simplifying the integral.

Second example with u = -x^2, showcasing the process of integrating with a quadratic function.

Integration of e^(-x^2) with a focus on the du/dx transformation.

Third example with u = x^(-1), demonstrating the integration of a function with a reciprocal exponent.

Integration of e^(cos(x)) with a focus on trigonometric substitution.

Solving a definite integral with a natural log transformation.

Evaluating the definite integral from 2 to 1 + e using properties of logarithms.

Integration of cos(u) with a focus on the argument substitution inside a cosine function.

Solving a differential equation by separating variables and integrating both sides.

Finding a particular solution to a differential equation with an initial condition.

Calculating the amount of water pumped into a tank using integration of a rate function.

Final example demonstrates the practical application of integration in calculating the volume of water in a tank over time.

Transcripts

Browse More Related Video

Integrating Exponential Functions By Substitution - Antiderivatives - Calculus

Business Calculus - Math 1329 - Section 5.1 - Indefinite Integration & Differential Equations

Antiderivatives and Indefinite Integrals

Indefinite Integral - Basic Integration Rules, Problems, Formulas, Trig Functions, Calculus

Calculus Chapter 3 Lecture 17 Indefinite Integrals

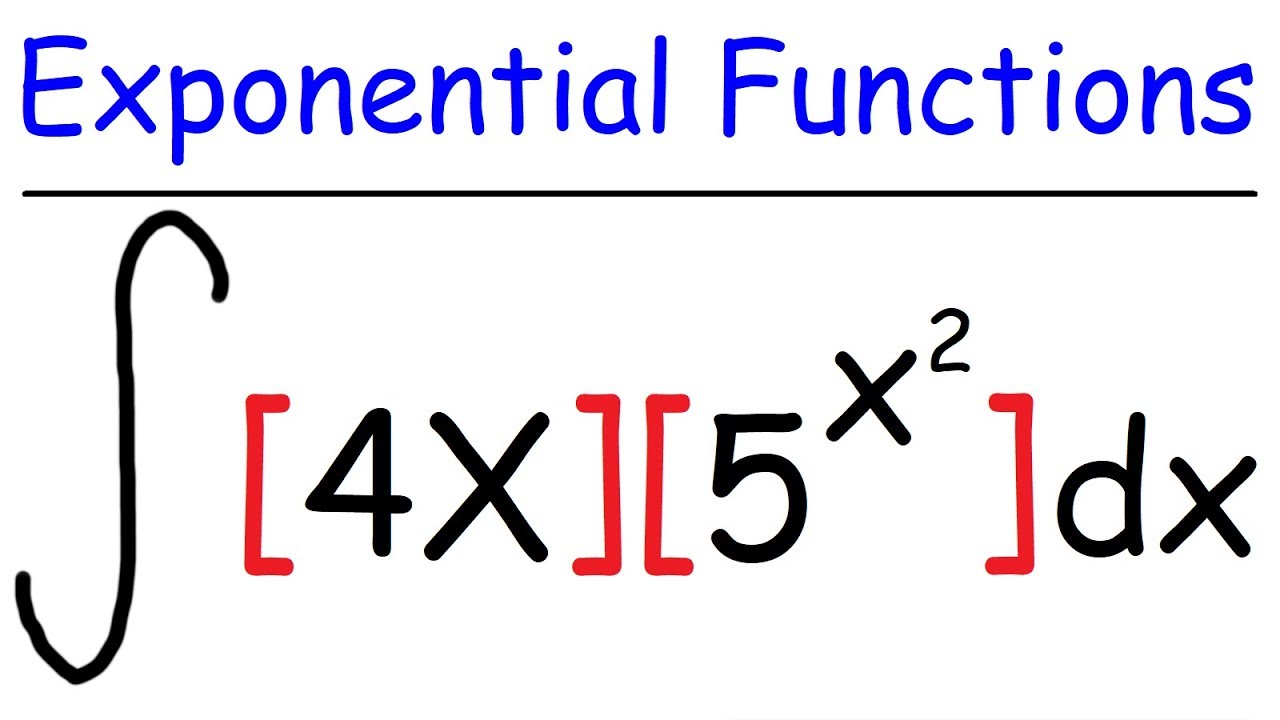
Indefinite Integral of Exponential Functions | Calculus

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Exponential FunctionsIntegration Techniquesu-SubstitutionMath TutorialDerivative RulesIntegral CalculusEducational ContentPractice ProblemsMathematical AnalysisLearning Resource