Finding function from power series by integrating | Series | AP Calculus BC | Khan Academy

Khan Academy
26 Dec 201605:46
EducationalLearning
32 Likes 10 Comments

TLDRIn this instructional video, the instructor explores the relationship between a given geometric series and its corresponding function. The series is identified as a derivative of a function, and the instructor guides viewers through the process of finding the original function by taking the antiderivative of the series. Using u-substitution, the integral is simplified, and the natural logarithm function is derived as the solution. The constant of integration is determined by substituting x=0, resulting in the function g(x) = ln(|1 - 2x|), applicable within the specified open interval.

Takeaways
  • 📚 The script discusses a mathematical problem involving a series and its corresponding function.
  • 🔍 The series given is identified as a geometric series with a first term of -2 and a common ratio of 2x.
  • 📉 The radius of convergence is mentioned as the range of x values for which the series converges.
  • 🔑 The task is to find the function that corresponds to a different series, which is related to the first series.
  • 💡 The series in question is recognized as the antiderivative of the first series, or vice versa, the first series is a derivative of the second.
  • 🧐 The derivative of -2x with respect to x is -2, and similarly, the derivative of -2x^2 is -4x, indicating a pattern.
  • 📝 The instructor introduces a function g(x) and suggests taking the indefinite integral of both sides of the equation to find g(x).
  • 📚 U-substitution is used to solve the integral, setting u = 1 - 2x, and du = -2dx.
  • 📈 The integral simplifies to the natural logarithm of the absolute value of u, which is then substituted back with the original expression to find g(x).
  • ✅ By substituting x = 0, the constant c in the natural logarithm function is determined to be zero.
  • 🎯 The final function g(x) is found to be the natural logarithm of the absolute value of (1 - 2x), subject to the open interval restriction.
Q & A

  • What is the given series in the script and how is it related to the function we need to find?

    -The given series is a geometric series with the first term as -2 and a common ratio of 2x. It is related to the function we need to find because the series provided in the question is the derivative of the function we are trying to determine.

  • What is the significance of the radius of convergence mentioned in the script?

    -The radius of convergence is the range of x values for which the series converges. It is important because it defines the interval within which the series and the function are valid.

  • Why is the first series a geometric series and how can it be summed up?

    -The first series is a geometric series because each term is obtained by multiplying the previous term by a common ratio, which is 2x in this case. It can be summed up using the formula for the sum of a geometric series: first term / (1 - common ratio).

  • What is the method used to find the function corresponding to the given series in the script?

    -The method used is integration. By taking the antiderivative of both sides of the equation, the function corresponding to the series is found.

  • How does the script suggest finding the function g(x) from the given series?

    -The script suggests that g(x) can be found by taking the indefinite integral of the given series, which is the antiderivative of the series.

  • What is the role of u-substitution in the integration process described in the script?

    -U-substitution is used to simplify the integral of the given series. By setting u equal to one minus two x, the integral becomes a simple natural logarithm function, which is easier to integrate.

  • What is the integral of 1/u and how is it related to the function g(x)?

    -The integral of 1/u with respect to u is the natural logarithm of the absolute value of u. In the context of the script, this integral is equal to g(x) after performing u-substitution and integrating.

  • How is the constant of integration, c, determined in the script?

    -The constant c is determined by substituting x = 0 into the integrated function and solving for c, which in this case turns out to be zero because g(0) is given as zero.

  • What is the final expression for g(x) found in the script?

    -The final expression for g(x) is the natural logarithm of the absolute value of (1 - 2x), which is found by integrating the given series.

  • Why is the absolute value used in the final expression for g(x)?

    -The absolute value is used to ensure that the function g(x) is well-defined for all x in the open interval, even though within the interval specified, the expression inside the logarithm is always positive.

  • What is the open interval mentioned in the script and why is it important?

    -The open interval mentioned is from -1/2 to 1/2. It is important because it defines the domain within which the series converges and the function g(x) is valid.

Outlines
00:00
📚 Understanding the Series and Its Corresponding Function

The instructor begins by presenting a series and its equivalence to a function within a specific interval. The series is identified as a geometric series with a first term of -2 and a common ratio of 2x. The sum of the series is derived using the formula for a geometric series, which is the first term divided by one minus the common ratio. This also establishes the radius of convergence for the series. The main task is to find the function corresponding to a given series, which is suspected to be the antiderivative of the initial series. The instructor suggests taking the indefinite integral of both sides of the equation to find the function, g(x), which is achieved through u-substitution and simplification to the natural logarithm of the absolute value of (1 - 2x), plus a constant c. By substituting x = 0, the constant c is determined to be zero, leading to the final function g(x).

05:02
🔍 Deriving the Function from the Series

This paragraph continues the exploration of the relationship between the series and its corresponding function. It emphasizes the recognition that the series given is the derivative of the function sought. The instructor explains the process of taking the antiderivative (indefinite integral) of both sides of the equation to find the function. The integral is simplified using u-substitution, leading to the natural logarithm of the absolute value of (1 - 2x). The restriction to the open interval ensures the argument of the logarithm is always positive, although the absolute value is kept for safety. The paragraph concludes with the successful derivation of the function, highlighting the key insight of recognizing the derivative relationship between the two series.

Mindmap
Keywords
💡Open Interval
An open interval is a set of real numbers that includes all the numbers between two given points except for the endpoints themselves. In the video, the open interval from negative 1/2 to 1/2 refers to all the x-values between these two numbers where the given mathematical series converges. This concept is essential for understanding the domain within which the series and functions are valid.
💡Geometric Series
A geometric series is a sequence of numbers where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio. In the script, the series is identified as a geometric series with a first term of negative two and a common ratio of 'two x', which is key to finding the sum of the series and understanding the convergence of the series.
💡Sum of a Series
The sum of a series refers to the result of adding all the terms of the series together. The video explains that the sum of the given geometric series is found by dividing the first term by one minus the common ratio, which is a fundamental concept in series analysis.
💡Radius of Convergence
The radius of convergence is a measure of the interval around a point where a power series converges. In the video, it is mentioned as the x values for which the series converges, which is crucial for determining the domain of validity for the series and the function derived from it.
💡Antiderivative
An antiderivative is a function that represents the reverse process of differentiation. In the context of the video, the second series is identified as the antiderivative of the first series, which helps in finding the function that corresponds to the series by integrating the first series.
💡Derivative
A derivative in calculus represents the rate at which a function changes with respect to one of its variables. The video mentions that the first series is a derivative of the second one, which is a key insight for relating the two series and finding the function that represents the second series.
💡Indefinite Integral
An indefinite integral is the process of finding the antiderivative of a function, often represented by the integral symbol and 'dx'. The video uses the concept of taking the indefinite integral of both sides of an equation to find the function corresponding to the given series.
💡U-Substitution
U-substitution is a method used in calculus to evaluate integrals by substituting a new variable u for an expression in the integral. In the script, u-substitution is used to simplify the integral of the function by setting u equal to 'one minus two x', which simplifies the integration process.
💡Natural Logarithm
The natural logarithm is the logarithm to the base 'e', where 'e' is an irrational constant approximately equal to 2.71828. In the video, the natural logarithm is the result of integrating 'du/u' and is part of the function that corresponds to the given series.
💡Constant of Integration
The constant of integration, often denoted by 'C', is added when finding an antiderivative because the derivative of a constant is zero, and thus, the antiderivative could have any constant value. In the video, it is determined that the constant of integration is zero by evaluating the function at x = 0.
💡Absolute Value
The absolute value of a number is its distance from zero on the number line, regardless of direction, and is denoted by two vertical lines around the number. In the video, the absolute value is used to ensure the natural logarithm function remains defined, as the logarithm of a negative number is not defined in the realm of real numbers.
Highlights

The expression for x in the interval (-1/2, 1/2) is given as a geometric series with the first term being -2 and common ratio 2x.

The sum of the geometric series is derived using the formula first term / (1 - common ratio).

The radius of convergence is discussed as the range of x values for which the series converges.

The task is to find the function corresponding to a given series, which is related to the first series.

The second series is identified as the antiderivative of the first series.

Derivatives of terms in the series are calculated to confirm the relationship between the two series.

The function g(x) is introduced to represent the antiderivative of the first series.

Integration is used to find g(x) by taking the indefinite integral of both sides of the equation.

U-substitution is applied with u = 1 - 2x to simplify the integral.

The integral is simplified to the natural logarithm of the absolute value of u.

The natural logarithm is expressed in terms of x after undoing the u-substitution.

The constant of integration c is determined by substituting x = 0 and solving for c.

It is found that c = 0, simplifying the expression for g(x).

The final function g(x) is given as the natural logarithm of the absolute value of (1 - 2x).

The importance of the open interval for the positivity of the function is noted.

The process concludes with the recognition that the series is the derivative of the found function.

The method of taking the antiderivative of both sides is highlighted as the key to solving the problem.

Transcripts

Browse More Related Video

Substitution Method for Definite Integrals **careful!**

my all-in-one calculus question

Integrating power series | Series | AP Calculus BC | Khan Academy

Integration of Taylor's Series | MIT 18.01SC Single Variable Calculus, Fall 2010

Taylor's Series of a Polynomial | MIT 18.01SC Single Variable Calculus, Fall 2010

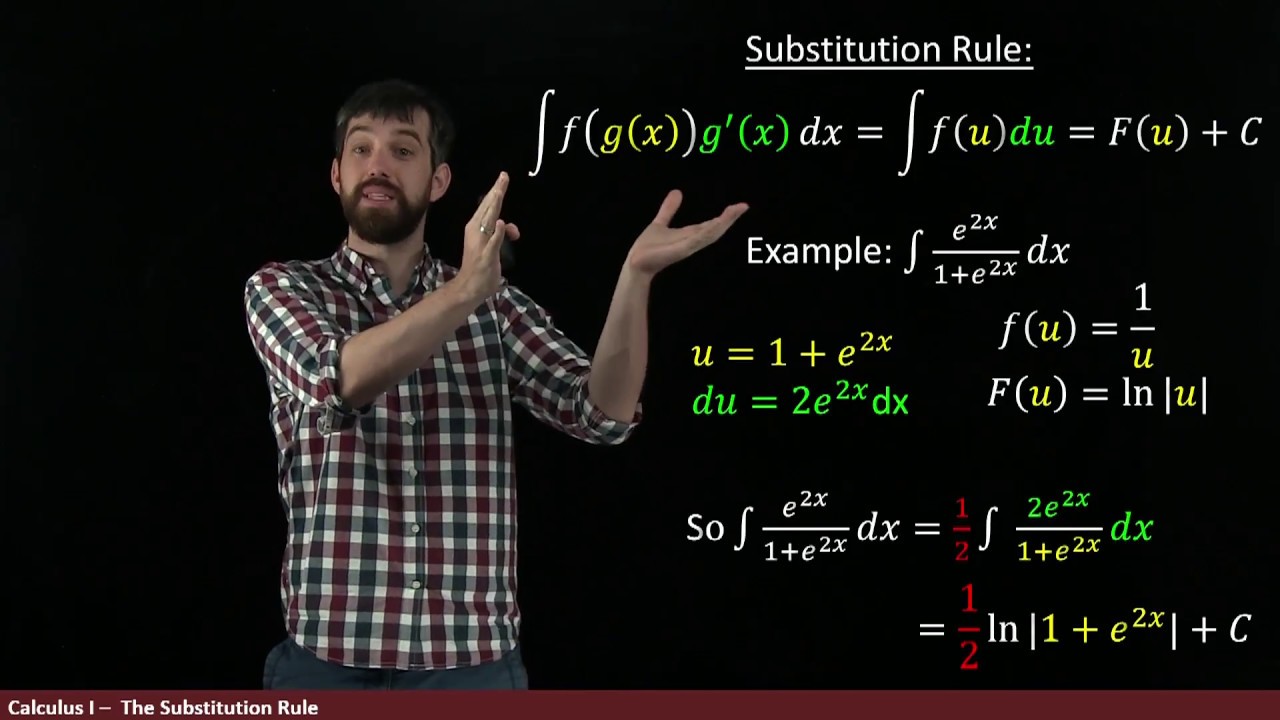
Adjusting the Constant in Integration by Substitution

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusGeometric SeriesAntiderivativesDerivativesIntegralsSeries AnalysisMathematicsEducational VideoInstructor GuideFunction Derivation