Taylor's Series of a Polynomial | MIT 18.01SC Single Variable Calculus, Fall 2010
TLDRIn this educational video, the professor guides students through the process of finding the Taylor series for a given polynomial function. Starting with the function f(x) = 3x^3 + 4x^2 - 2x + 1, the professor explains the general formula for the Taylor series and computes the necessary derivatives. Surprisingly, it is revealed that the Taylor series for this polynomial at x=0 is identical to the original function itself, highlighting the concept that Taylor series are increasingly accurate approximations of the function as the order increases.
Takeaways
- 📚 The video is focused on practicing the concept of Taylor series in mathematics.
- 🔍 The task is to find the Taylor series for the given polynomial function: \( f(x) = 3x^3 + 4x^2 - 2x + 1 \).
- ⏸ The professor suggests pausing the video to work on the problem before revealing the solution.
- 📝 The formula for the Taylor series is provided, emphasizing the sum of the nth derivative of the function at 0 over n factorial times \( x^n \).
- 📉 The process involves calculating the derivatives of the function and evaluating them at \( x = 0 \).
- 📊 A table is created to list the values of the derivatives at \( x = 0 \) up to the fourth derivative.
- 🔢 The zeroth derivative is the function itself evaluated at 0, which is 1.
- 📐 The first derivative evaluated at 0 is -2, and subsequent derivatives are calculated in a similar manner.
- 🔄 The nth derivative at 0 is 0 for \( n > 3 \), indicating the series will have only a finite number of terms.
- 🔑 The Taylor series for the given polynomial turns out to be the polynomial itself, highlighting a key property of Taylor series for polynomials at \( x = 0 \).
- 💡 The video concludes with the insight that the Taylor series of a polynomial at \( x = 0 \) is the polynomial itself, providing a deeper understanding of Taylor series approximations.
Q & A
What is the main topic of the video?
-The main topic of the video is to practice writing the Taylor series for a given polynomial function.
What is the given function for which the Taylor series is to be found?
-The given function is f(x) = 3x^3 + 4x^2 - 2x + 1.
What is the general formula for the Taylor series?
-The general formula for the Taylor series is f(x) = Σ (from n=0 to infinity) [f^n(0) / n!] * x^n, where f^n(0) is the nth derivative of f evaluated at x=0.
What is the zeroth derivative of the function f(x) in the context of the video?
-The zeroth derivative of the function f(x) is the function itself, which when evaluated at x=0 gives the value 1.
What is the first derivative of the given function?
-The first derivative of the function f(x) is 9x^2 + 8x - 2.
What is the value of the first derivative evaluated at x=0?
-The value of the first derivative evaluated at x=0 is -2.
What is the second derivative of the given function?
-The second derivative of the function f(x) is 18x + 8.
What is the value of the second derivative evaluated at x=0?
-The value of the second derivative evaluated at x=0 is 8.
What is the third derivative of the given function?
-The third derivative of the function f(x) is 18.
Why does the Taylor series of a polynomial function evaluated at x=0 result in the same polynomial?
-The Taylor series of a polynomial function evaluated at x=0 results in the same polynomial because the Taylor series represents an infinite sum of the function's derivatives evaluated at a point, and for polynomials, all derivatives beyond a certain order are zero, thus the series converges to the original polynomial.
What is the significance of the nth derivative being zero for n greater than 3 in the given function?
-The significance of the nth derivative being zero for n greater than 3 is that it indicates that all terms in the Taylor series beyond the third power of x will be zero, and thus the series will only include up to the cubic term.
Why does the order of terms in the Taylor series appear in reverse order compared to the original function?
-The order of terms in the Taylor series appears in reverse order compared to the original function because the Taylor series is constructed by starting from the zeroth derivative and incrementally adding higher derivatives, which naturally results in the powers of x increasing rather than decreasing.
What is the final result of the Taylor series for the given function?
-The final result of the Taylor series for the given function is the same as the original function: 3x^3 + 4x^2 - 2x + 1.
Why does the video emphasize that the Taylor series of a polynomial at x=0 is the polynomial itself?
-The video emphasizes this point to highlight a key property of Taylor series for polynomials at x=0, which is that they provide an exact representation of the polynomial, serving as a fundamental understanding for approximations and expansions in calculus.
Outlines
📚 Introduction to Taylor Series Practice
The professor begins the recitation by introducing the topic of Taylor series and encourages students to practice by finding the Taylor series for a given polynomial function, f(x) = 3x^3 + 4x^2 - 2x + 1. Students are prompted to pause the video to attempt the problem before the professor demonstrates the solution. The process involves recalling the general formula for the Taylor series and computing the necessary derivatives of the function at x = 0.
🔍 Deriving the Taylor Series for a Polynomial
The professor proceeds to derive the Taylor series for the given polynomial function by calculating its derivatives at x = 0 and evaluating them sequentially. The process includes creating a table to organize the derivatives and their evaluations. It is revealed that all derivatives higher than the third order are zero when evaluated at x = 0, leading to the conclusion that the Taylor series for this polynomial will only include up to the third power of x. The professor then plugs these values into the Taylor series formula, resulting in a series that mirrors the original polynomial function, illustrating the concept that the Taylor series at x = 0 for a polynomial is the polynomial itself.
Mindmap
Keywords
💡Taylor series
💡Polynomial
💡Derivative
💡Factorial
💡Approximation
💡Recitation
💡nth derivative
💡Zeroth derivative
💡Infinite sum
💡Evaluation
💡Coefficients
Highlights
Introduction to the task of finding the Taylor series for a given polynomial function.
Encouragement to pause the video for self-practice before revealing the solution.
Explanation of the general formula for the Taylor series.
The importance of calculating the nth derivative of the function at 0 for the Taylor series.
Demonstration of creating a table for the derivatives evaluated at 0.
Evaluation of the zeroth derivative of the function at 0 yielding 1.
Derivation and evaluation of the first derivative at 0 resulting in -2.
Calculation of the second derivative at 0, resulting in 8.
Identification of the third derivative at 0 as 18.
Observation that all derivatives higher than the third order evaluated at 0 are 0.
Plugging in the calculated values into the Taylor series formula.
Realization that the Taylor series for the given polynomial is the polynomial itself.
Discussion on the nature of Taylor series as an approximation that converges to the original function.
Insight that the Taylor series of a polynomial at 0 is the polynomial itself.
Reflection on the learning process and the value of understanding the Taylor series for polynomials.
Conclusion summarizing the main goal of the video and the outcome of the Taylor series exercise.
Transcripts
Browse More Related Video

2023 AP Calculus BC FRQ #6
Calculus Chapter 1 Lecture 3 Taylor Series
Integration of Taylor's Series | MIT 18.01SC Single Variable Calculus, Fall 2010

AP Calculus BC Lesson 10.14 Part 1

Worked example: coefficient in Taylor polynomial | Series | AP Calculus BC | Khan Academy
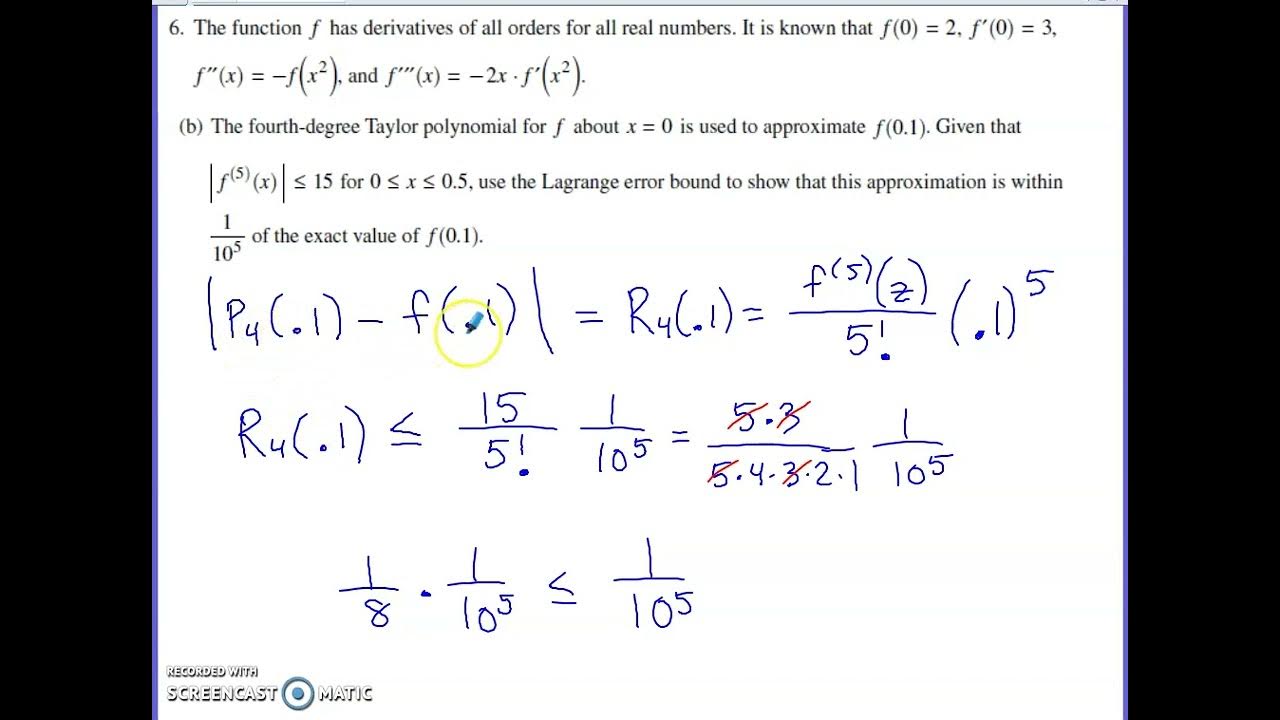
2023 AP Calculus BC Free Response Question #6
5.0 / 5 (0 votes)
Thanks for rating: