Integrating power series | Series | AP Calculus BC | Khan Academy

Khan Academy
16 Dec 201606:04
EducationalLearning
32 Likes 10 Comments

TLDRIn this instructional video, the presenter guides viewers through the process of calculating the definite integral of an infinite series function, f(x), from zero to one. The series is expressed as a sum of terms involving powers of x and constants. By leveraging the properties of integration, the presenter breaks down the problem into a series of integrals, which are then simplified using antiderivatives. The solution culminates in recognizing and summing an infinite geometric series, resulting in a final answer of 1/12. The video encourages viewers to engage with the material actively, promoting a deeper understanding of calculus concepts.

Takeaways
  • πŸ“š The problem involves calculating the definite integral of an infinite series function f(x) from 0 to 1.
  • πŸ” The function f(x) is represented as an infinite series with terms involving powers of x and 4.
  • πŸ“ The instructor encourages viewers to pause the video and attempt the problem on their own.
  • 🌟 The integral of a sum of terms can be expressed as the sum of the integrals of each term individually.
  • πŸ“ˆ The process involves taking the antiderivative of each term in the series and evaluating it from 0 to 1.
  • πŸ“š The antiderivative of the term \( (n+1) / (4^{n+1}) \cdot x^n \) is \( x^{n+1} / (n+1) \) with the original constant factor.
  • πŸ”‘ Simplification leads to an expression that can be evaluated at the bounds 0 and 1, resulting in a telescoping series.
  • πŸ“‰ The series simplifies to a geometric series after substituting x = 1 and subtracting the value at x = 0.
  • πŸ”’ The first term of the resulting geometric series is 1/16, and the common ratio is 1/4.
  • 🌠 The sum of the infinite geometric series converges to \( 1/16 \) divided by \( 3/4 \), which simplifies to 1/12.
  • ✨ The solution demonstrates the power of symbolic mathematics in simplifying complex problems into solvable forms.
Q & A

  • What is the function f(x) given in the script?

    -The function f(x) is an infinite series starting from n=1 to infinity, where each term is (n + 1) / (4^(n + 1)) multiplied by x to the power of n.

  • What is the task the instructor is trying to accomplish in the script?

    -The task is to find the definite integral of the function f(x) from 0 to 1.

  • Why is it suggested to pause the video during the explanation?

    -The instructor encourages students to pause the video to try solving the problem on their own or to keep working through it, which promotes active learning and understanding.

  • How does the instructor approach the problem of integrating an infinite series?

    -The instructor uses the property of integration that allows the definite integral of a sum to be the sum of definite integrals of the individual terms.

  • What mathematical property is used to simplify the integration of the series?

    -The property used is that the integral of a sum of functions is equal to the sum of the integrals of the functions.

  • How does the instructor rewrite the integral of the series?

    -The instructor rewrites the integral as the sum from n=1 to infinity of the definite integral of each term (n + 1) / (4^(n + 1)) * x^n dx.

  • What is the antiderivative of the term (n + 1) / (4^(n + 1)) * x^n?

    -The antiderivative is x^(n + 1) / (n + 1), with the original constant (n + 1) / (4^(n + 1)) remaining as a factor.

  • Why does the instructor simplify the antiderivative before evaluating the integral from 0 to 1?

    -Simplifying the antiderivative makes it easier to evaluate the integral by recognizing patterns and simplifying the expression before applying the limits of integration.

  • What does the instructor recognize in the simplified expression after taking the antiderivative?

    -The instructor recognizes an infinite geometric series with a common ratio of 1/4 after evaluating the antiderivative at the limits of integration.

  • How does the instructor find the sum of the infinite geometric series?

    -The instructor uses the formula for the sum of an infinite geometric series, which is the first term divided by one minus the common ratio, in this case, 1/16 divided by 3/4.

  • What is the final result of the definite integral of f(x) from 0 to 1?

    -The final result of the definite integral is 1/12.

Outlines
00:00
πŸ“š Calculating the Definite Integral of an Infinite Series

The instructor begins by presenting the problem of finding the definite integral of the function f(x) from 0 to 1, which is defined as an infinite series. The series is expressed as the sum from n=1 to infinity of (n+1) / (4^(n+1)) * x^n. The instructor encourages the audience to attempt the problem independently. The approach taken is to rewrite the problem as the sum of definite integrals of each term in the series, leveraging the property that the integral of a sum is the sum of the integrals. The instructor then proceeds to find the antiderivative of each term, simplifying the expression and eventually evaluating the integral from 0 to 1 for each term in the series.

05:02
πŸ” Simplifying the Series to an Infinite Geometric Series

Continuing from the previous explanation, the instructor simplifies the expression further by recognizing that the antiderivative results in a form that resembles an infinite geometric series. The first term of the series is identified as 1/16, and the common ratio is determined to be 1/4. Given that the absolute value of the common ratio is less than one, the series is guaranteed to converge. The instructor applies the formula for the sum of an infinite geometric series, which is the first term divided by one minus the common ratio, resulting in a sum of 1/12. The summary concludes by reflecting on the initial complexity of the problem and the elegance of the solution using symbolic mathematics to simplify and solve the integral of an infinite series.

Mindmap
Keywords
πŸ’‘Infinite Series
An infinite series is a sequence of numbers that continues indefinitely. In the context of the video, the function f(x) is defined as an infinite series where each term is of the form (n+1) / (4^(n+1)) * x^n, and the series is summed from n=1 to infinity. This concept is central to the video's theme of evaluating the definite integral of f(x) from 0 to 1.
πŸ’‘Definite Integral
A definite integral is a fundamental concept in calculus that represents the signed area under a curve between two points on the x-axis. In the video, the instructor is interested in finding the definite integral of the function f(x) from 0 to 1, which is the sum of the areas under the curve of f(x) over the given interval.
πŸ’‘Summation
Summation, often represented by the Greek letter sigma (Ξ£), is a mathematical operation that combines the elements of a set into a single value. In the script, the instructor uses summation to express the infinite series of the function f(x) and later to break down the definite integral of f(x) into a sum of definite integrals of its individual terms.
πŸ’‘Integration Properties
Integration properties are the rules that govern how integrals behave under various mathematical operations. The video script mentions these properties to justify the process of breaking down the integral of a sum into the sum of integrals, which is a key step in evaluating the integral of the given function.
πŸ’‘Antiderivatives
Antiderivatives, also known as indefinite integrals, are functions that can be differentiated to produce the original function. In the video, the instructor finds the antiderivative of each term in the series to evaluate the definite integral from 0 to 1, which is a crucial step in the process.
πŸ’‘Power Rule
The power rule is a basic principle in calculus that states the derivative of x^n (where n is a constant) is n*x^(n-1). In the script, the instructor reverses this rule to find the antiderivative of x^n, which is x^(n+1)/(n+1), and applies it to the terms of the series.
πŸ’‘Geometric Series
A geometric series is a series where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio. The video concludes with recognizing the sum of the definite integrals as a geometric series, which simplifies the calculation of the overall integral.
πŸ’‘Common Ratio
In a geometric series, the common ratio is the factor by which we multiply each term to get the next. In the video, the common ratio is identified as 1/4, which is crucial for determining the convergence of the series and calculating its sum.
πŸ’‘Convergence
Convergence refers to the property of a series where the sum of its terms approaches a finite value as the number of terms increases indefinitely. The video script discusses the convergence of the geometric series, which is guaranteed because the absolute value of the common ratio is less than one.
πŸ’‘Symbolic Mathematics
Symbolic mathematics involves the manipulation of mathematical symbols and the execution of operations according to rules that preserve the equality of expressions. The video script highlights the power of symbolic mathematics in evaluating the antiderivative of the series and simplifying the problem to a geometric series.
πŸ’‘First Term
In a series, the first term is the initial value that sets the pattern for the rest of the series. In the context of the video, the first term of the geometric series is 1/16, which is crucial for calculating the sum of the series using the formula for the sum of a geometric series.
Highlights

Introduction of an infinite series representation of the function f(x).

Encouragement for viewers to pause and attempt the problem independently.

Rewriting the series to prepare for integration.

Explanation of the property that allows the integral of a sum to be a sum of integrals.

Use of sigma notation to express the sum of integrals.

Evaluation of the integral by taking the antiderivative of each term.

Simplification of the antiderivative expression by canceling out terms.

Identification of the resulting expression as an infinite geometric series.

Determination of the first term and common ratio of the geometric series.

Application of the formula for the sum of an infinite geometric series.

Calculation of the sum of the series using the common ratio.

Final answer revealing the integral's value as 1/12.

Reflection on the initial daunting nature of the problem and the power of symbolic mathematics.

Emphasis on the ability to find the sum of an infinite series through integration.

Highlighting the importance of recognizing patterns in mathematical series.

Demonstration of the step-by-step process to solve complex integrals.

Transcripts

Browse More Related Video

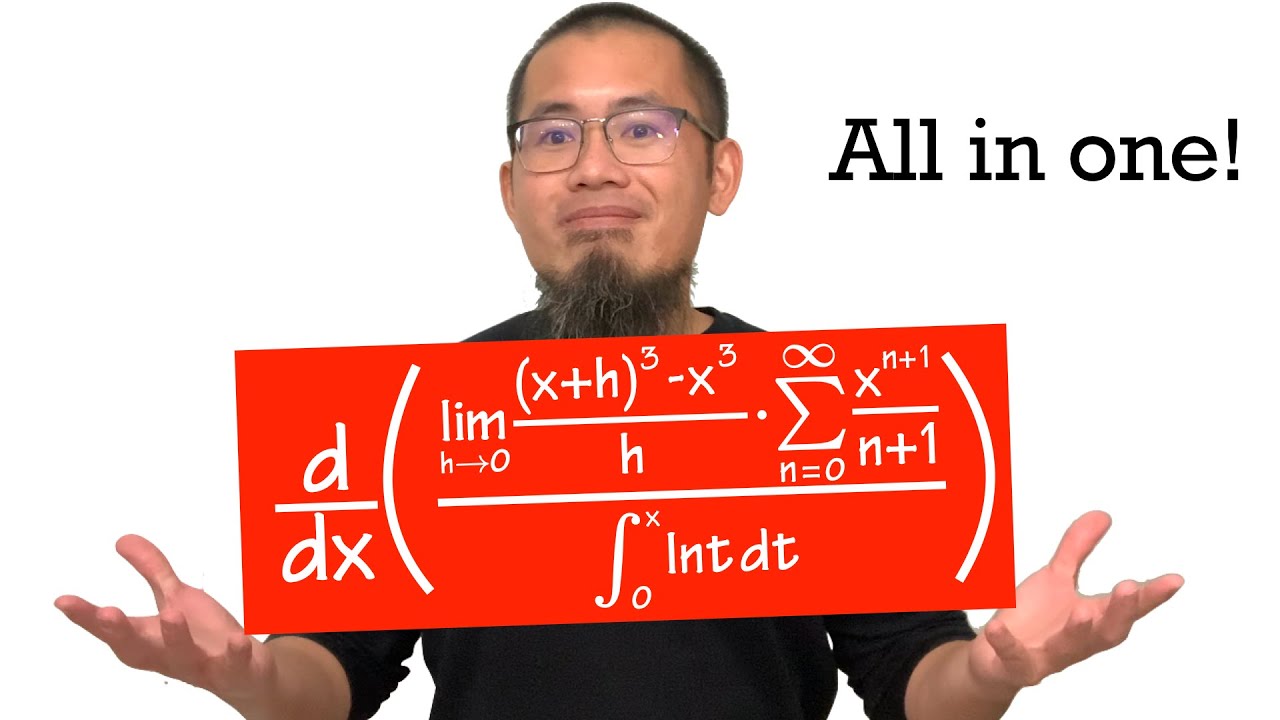
my all-in-one calculus question

Power series of ln(1+x_) | Series | AP Calculus BC | Khan Academy

Infinite Series Multiple Choice Practice for Calc BC (Part 8)

Worked example: cosine function from power series | Series | AP Calculus BC | Khan Academy

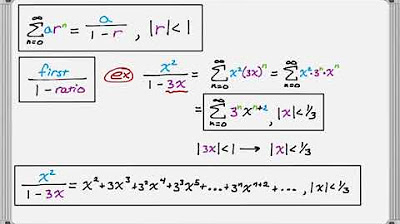
Geometric Series Summing to Functions

Finding function from power series by integrating | Series | AP Calculus BC | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Infinite SeriesDefinite IntegralMathematicsSummationIntegrationGeometric SeriesSeries ConvergenceAntiderivativesPower RuleSymbolic Math