Limits Involving Infinity

RH Mathematics

25 Aug 202133:24

EducationalLearning

32 Likes 10 Comments

TLDRThis video discusses mathematical concepts of limits involving infinity and asymptotes, focusing on graphs like y=1/x and y=arctan(x). It explains horizontal and vertical asymptotes, and how to determine them using limits. The video also covers growth rates of functions, including exponential, algebraic, and logarithmic, and provides examples to illustrate these concepts.

Takeaways

📈 The video discusses limits involving infinity and asymptotes, starting with the graph of y = 1/x to illustrate horizontal and vertical asymptotes.
🧭 It explains the concept of horizontal asymptotes using the limit as x approaches infinity of 1/x, which equals zero, and the vertical asymptote at x = 0.
📚 The script emphasizes the importance of using limits instead of asymptote language to describe the behavior of functions as x approaches infinity or negative infinity.
📉 The video provides a technical definition of limits, explaining how to get a function value close to a certain limit by taking sufficiently large x values.
📚 It introduces the taxonomy of growth rates, categorizing functions into exponential, algebraic, and logarithmic, with exponential growing the fastest and logarithmic the slowest.
🔢 The script explains how to determine limits involving infinity by comparing the growth rates of the numerator and denominator of a fraction.
📌 It highlights the 'number one rule of limits' in AP Calculus, which is to plug in the value first and see what happens, as it sometimes leads to the answer directly.
🤔 The video discusses the special case of limits involving natural logarithms and how they relate to the behavior of functions as x approaches infinity or negative infinity.
📐 It explains the concept of double horizontal asymptotes that occur with certain functions, such as those involving square roots or absolute values, and how to identify them.
📈 The script covers how to compute limits for functions with exponential terms in both the numerator and the denominator, and how the behavior changes with positive and negative infinity.
📘 Finally, the video challenges viewers with an example from the Taylor series unit, encouraging them to compute a complex limit involving e^x.

Q & A

What is the main topic discussed in the video?
-The main topic discussed in the video is limits involving infinity and asymptotes, particularly how to interpret and calculate them in the context of calculus.
What is the horizontal asymptote for the function y = 1/x?
-The horizontal asymptote for the function y = 1/x is y = 0, as the function approaches this value as x goes to both positive and negative infinity.
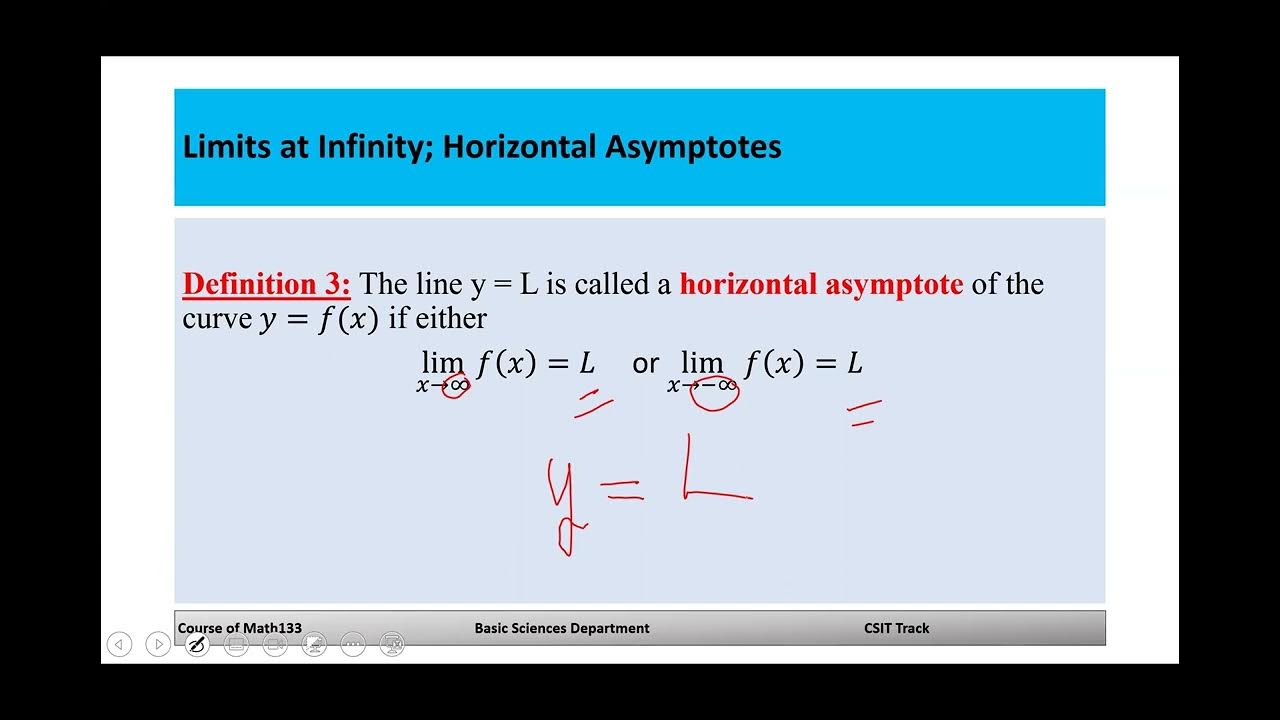
What is the relationship between a horizontal asymptote and the limit of a function as x approaches infinity?
-A horizontal asymptote corresponds to the limit of a function as x approaches infinity. If the limit exists, the y-value of the horizontal asymptote is the value that the limit approaches.
What is the vertical asymptote for the function y = 1/x?
-The vertical asymptote for the function y = 1/x is the line x = 0, as the function approaches infinity or negative infinity as x approaches zero from either side.
How does the limit of a function as x approaches zero from the positive side relate to a vertical asymptote?
-If the limit of a function as x approaches zero from the positive side is positive infinity, it suggests a vertical asymptote at x = 0, as the function values increase without bound as x gets closer to zero.
What is the definition of the limit as x approaches infinity of a function f(x)?
-The limit as x approaches infinity of a function f(x) is defined as the value that f(x) approaches as x gets arbitrarily large, regardless of the direction from which x approaches infinity.
What is the significance of the number e in calculus?
-The number e is significant in calculus as it is the base of the natural exponential function. It is approximately equal to 2.71828 and is the limit of (1 + 1/n)^n as n approaches infinity.
What is the limit as x approaches infinity for the function y = arctan(x)?
-The limit as x approaches infinity for the function y = arctan(x) is pi/2, indicating that the function approaches this value as x becomes very large.
What is the 'number one rule of limits' mentioned in the video?
-The 'number one rule of limits' mentioned in the video is to always try plugging in the value you are taking the limit of, especially when dealing with simple functions or when first learning about limits.
How does the video explain the concept of relative growth rates of different types of functions?
-The video explains the concept of relative growth rates by categorizing functions into exponential, algebraic, and logarithmic, and then discussing how these categories compare in terms of how quickly they grow as x increases.
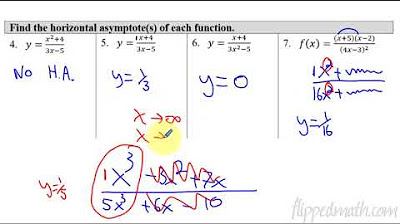
What is the significance of the ratio of the leading coefficients in determining horizontal asymptotes?
-The ratio of the leading coefficients is significant in determining horizontal asymptotes because it provides the y-value that the function approaches as x goes to infinity, especially when the highest powers of x in the numerator and denominator are the same.
What are the three scenarios that can cause a function to have double horizontal asymptotes?
-The three scenarios that can cause a function to have double horizontal asymptotes are: 1) a mix of exponential functions in the numerator and denominator with different bases, 2) a function like x divided by the square root of x squared, and 3) the inverse tangent function, which naturally has two horizontal asymptotes due to its behavior on the unit circle.
How does the video approach the concept of limits involving infinity for logarithmic functions?
-The video approaches the concept of limits involving infinity for logarithmic functions by stating that for large values of x, logarithmic functions grow very slowly and can be treated as constants when compared to growing polynomials or exponential functions.
What is the significance of the limit of a function as x approaches negative infinity?
-The limit of a function as x approaches negative infinity is significant because it helps determine the behavior of the function as x takes on very large negative values, which can be different from its behavior as x approaches positive infinity.
How can you determine if a function has a vertical asymptote?
-You can determine if a function has a vertical asymptote by checking if the function approaches infinity or negative infinity as x approaches a certain value, which typically indicates a division by zero or an undefined expression in the function.

Outlines

00:00

📈 Understanding Asymptotes and Limits

The video begins with an introduction to the concepts of asymptotes and limits, focusing on the graph of y = 1/x. The instructor explains the horizontal asymptote at y = 0 and the vertical asymptote at x = 0. The discussion then shifts to the mathematical definition of limits, particularly the limit as x approaches infinity. The instructor clarifies that the limit of 1/x as x approaches infinity is zero, which corresponds to the horizontal asymptote. Additionally, the limit as x approaches zero from the positive side of 1/x is discussed, suggesting it approaches positive infinity, aligning with the concept of a vertical asymptote.

05:01

📚 Exploring Limits and Growth Rates

This paragraph delves deeper into the technical definition of limits, particularly the limit as x approaches infinity. The instructor uses the example of y = arctan(x) to illustrate horizontal asymptotes at π/2 and -π/2. The concept of 'plugging in' values when dealing with limits is introduced, highlighting its simplicity and effectiveness in certain cases. The paragraph also introduces the taxonomy of growth rates, distinguishing between exponential, algebraic, and logarithmic functions, and their relative growth rates. The emphasis is on understanding how these functions behave as x approaches infinity or negative infinity.

10:01

🔍 Analyzing Algebraic Limits and Functions

The instructor discusses algebraic limits, emphasizing the importance of recognizing when to 'plug in' values and when additional steps are necessary. Examples are provided to illustrate how to handle limits involving exponential functions and polynomials. The concept of horizontal asymptotes is revisited, explaining how to determine them by comparing the highest powers in the numerator and denominator of a rational function. The paragraph concludes with a reminder that certain functions, like constants and trigonometric functions, do not grow and can be treated as such in limit calculations.

15:02

🌐 Discussing Exponential Functions and e

This section introduces the number e and its definition in terms of a limit, although the instructor notes that a deep understanding of e is not necessary unless one is interested in the proofs of exponential function properties. The focus then shifts to the natural logarithm, defined as the exponent that yields a given number when applied to e. Examples are given to illustrate how to calculate natural logarithms of various values. The paragraph concludes with a series of examples that demonstrate how to compute limits involving exponential functions and natural logarithms as x approaches infinity.

20:02

📉 Limits Involving Infinity and Asymptotes

The instructor explores the relationship between horizontal asymptotes and limits involving infinity. Examples are provided to show how to determine the limits of functions as x approaches both positive and negative infinity. The concept of double horizontal asymptotes is introduced, explaining how certain functions can have two different horizontal asymptotes depending on the direction from which infinity is approached. The paragraph concludes with a discussion on how to identify vertical asymptotes and their implications for limit calculations.

25:05

🔢 Solving Complex Limit Problems

This paragraph presents more complex limit problems, challenging the viewer to apply their understanding of asymptotes and limits. The instructor discusses how to handle functions with square roots and exponential terms, emphasizing the importance of recognizing patterns that lead to double horizontal asymptotes. The paragraph also covers how to determine the coefficients of a function given its horizontal and vertical asymptotes, providing a step-by-step approach to solving such problems.

30:05

🔬 Advanced Limit Calculations and Challenges

The final paragraph wraps up the video with a discussion on advanced limit calculations, particularly those involving Taylor series, which is a topic from the later part of the calculus course. The instructor challenges the viewer to compute a complex limit involving a Taylor series expansion, providing a brief moment for the viewer to attempt the problem before revealing the answer. The paragraph concludes with a summary of the key concepts covered in the video, including double horizontal asymptotes and the distinct behaviors of exponential functions as x approaches infinity and negative infinity.

Mindmap

Keywords

💡Infinity

In the context of the video, 'infinity' refers to the concept of an unbounded quantity that is larger than any finite number. It is central to discussions about limits, particularly when considering the behavior of functions as their input (x) approaches an infinitely large value. The script uses the term to describe horizontal asymptotes and limits, such as stating the limit as x approaches infinity of 1/x is zero.

💡Asymptote

An 'asymptote' is a line that a function approaches but never actually reaches as the input (x) approaches a certain value, typically infinity or negative infinity. The script discusses both horizontal and vertical asymptotes, using the graph of y=1/x to illustrate a horizontal asymptote at y=0 and a vertical asymptote at x=0.

💡Limit

A 'limit' is a fundamental concept in calculus that describes the value that a function approaches as the input (x) approaches a certain value. The video script explains limits in relation to infinity, such as the limit of 1/x as x approaches infinity being zero, which corresponds to a horizontal asymptote.

💡Horizontal Asymptote

A 'horizontal asymptote' is a horizontal line that a function approaches as its input (x) becomes very large or very small (towards positive or negative infinity). The script explains that the horizontal asymptote of y=1/x is y=0, indicating the function gets closer and closer to y=0 but never touches it as x increases.

💡Vertical Asymptote

A 'vertical asymptote' occurs at a value of x where the function is not defined (usually due to division by zero), and the function's values increase or decrease without bound as x approaches this value. The script identifies the vertical asymptote at x=0 for the function y=1/x.

💡Arctan (Arctangent)

The 'arctangent' function, denoted as 'arctan' or 'tan^(-1)', is the inverse function of the tangent. It is used in the script to illustrate a function with horizontal asymptotes at π/2 and -π/2 as x approaches positive and negative infinity, respectively.

💡Polynomial Functions

A 'polynomial function' is a function that involves only multiplication, addition, subtraction, and non-negative integer exponents of the variable. The script categorizes polynomials as algebraic functions and discusses their growth rates compared to exponential and logarithmic functions.

💡Exponential Functions

An 'exponential function' is a function where the variable is the exponent, such as 'e^x' or 'a^x' where 'a' is a constant. The script mentions that exponential functions grow faster than polynomial functions and provides the example of 10^x growing faster than 2^x.

💡Logarithmic Functions

A 'logarithmic function' is the inverse of an exponential function and involves the logarithm of a number to a given base. The script notes that logarithmic functions grow more slowly than polynomial functions and focuses on the natural logarithm (base e) in the context of AP Calculus.

💡Growth Rates

The 'growth rates' of functions refer to how quickly they increase or decrease as their input values change. The script discusses the taxonomy of growth rates, comparing the relative growth rates of exponential, algebraic, and logarithmic functions.

💡Leading Coefficients

In the context of polynomial functions, the 'leading coefficients' are the coefficients of the term with the highest power. The script explains that when determining horizontal asymptotes for rational functions, the ratio of the leading coefficients is used when the powers of x in the numerator and denominator are the same.

💡Natural Logarithm

The 'natural logarithm', often denoted as 'ln(x)', is the logarithm of a number with base 'e', where 'e' is an irrational constant approximately equal to 2.71828. The script defines the natural logarithm as the exponent to which 'e' must be raised to produce a given number.

💡Taylor Series

A 'Taylor series' is an infinite sum of terms that represents a function as a power series. The script briefly mentions the Taylor series towards the end, suggesting it as a more advanced topic for students interested in a deeper understanding of calculus.

Highlights

Introduction to limits involving infinity and asymptotes with a focus on the graph of y equals 1 over x.

Explanation of horizontal and vertical asymptotes using the graph of y equals 1 over x as an example.

Defining limits with technical definitions and their relation to asymptotes.

Illustration of how to approach limits and asymptotes using the function y equals arc tangent x.

The importance of plugging in values first when dealing with limits, especially in AP Calculus.

Taxonomy of growth rates comparing exponential, algebraic, and logarithmic functions.

Understanding limits involving infinity by comparing the growth rates of functions in fractions.

Technical definition of limits as x approaches infinity and how it relates to horizontal asymptotes.

Demonstration of multiplying by clever forms of 1 to simplify limits involving polynomials.

Identification of functions that do not grow, such as constants and trigonometric functions.

Introduction to the number e and its definition through a limit.

Explanation of natural logarithms and their relation to the special exponent on e.

Examples of computing limits as x approaches infinity and negative infinity for various functions.

Discussion on horizontal asymptotes and their connection to limits involving infinity.

Analysis of double horizontal asymptote scenarios and how to recognize them.

Solving for horizontal and vertical asymptotes using algebraic manipulation and limit properties.

Challenge problem involving the computation of a complex limit from the Taylor series unit.

Transcripts

Browse More Related Video

AP Calculus AB - 1.15 Connecting Limits at Infinity and Horizontal Asymptotes

Infinite limits and asymptotes | Limits and continuity | AP Calculus AB | Khan Academy

Math 133 Lecture 2 6

Calculus AB/BC – 1.15 Limits at Infinity and Horizontal Asymptotes

Monday Night Calculus: Limits at Infinity and Infinite Limits

Calculus AB Homework 1.4 Limits Involving Infinity

Related Tags

Calculus Limits Asymptotes Infinity Graphs Horizontal Asymptote Vertical Asymptote Algebraic Functions Exponential Growth Logarithmic Functions Educational Content