Multiplication and Division of Small Numbers

Professor Dave Explains
4 Aug 201706:19
EducationalLearning
32 Likes 10 Comments

TLDRThe video explains the basic arithmetic operations of addition, subtraction, multiplication, and division. It discusses how multiplication is an abbreviated form of repeated addition and how division does the opposite, breaking down numbers. Key concepts covered include: factors and products in multiplication, dividends, divisors, and quotients in division. It also explains commutative and associative properties and why they apply to multiplication but not division. The video advocates practicing mental math rather than over relying on technology, in order to build mental acuity and appreciate the patterns in numbers.

Takeaways
  • 😀 Multiplication is repeated addition, combining identical groups into a product
  • 😃 Division breaks down a larger number into smaller pieces based on a divisor
  • 🤓 Multiplication and division are inverse operations
  • 🧐 Multiplication is commutative and associative, division is not
  • 🤔 Memorizing multiplication tables builds mental math skills
  • 😕 Overreliance on technology weakens mental math abilities
  • 😡 Avoid overusing calculators for trivial math problems
  • 🤯 Practice mental math to appreciate patterns in numbers
  • 😎 Tips on mental math will be provided later
  • 👍 Comprehending the 4 basic operations takes practice
Q & A

  • How does multiplication relate to repeated addition?

    -Multiplication can be viewed as an abbreviated form of repeated addition. For example, 2 x 5 represents adding two, five times, which results in ten.

  • What are the two numbers that are multiplied called, and what is the result called?

    -The two numbers that are multiplied are called factors. The result is called the product.

  • How does division relate to multiplication?

    -Division is the inverse operation of multiplication. If multiplication combines groups into a larger number, division breaks down a larger number into smaller groups.

  • In division, what are the three key numbers called?

    -In division, the number being divided is called the dividend, the number dividing it is called the divisor, and the result is called the quotient.

  • Is multiplication commutative and associative - why/why not?

    -Yes, multiplication is commutative because 2 x 5 = 5 x 2. It is also associative, because the grouping of numbers being multiplied does not change the result.

  • Is division commutative and associative - why/why not?

    -No, division is not commutative nor associative. 10 / 5 / 2 is a different result than 10 / 2 / 5. The order matters in division.

  • What are some properties of the basic arithmetic operations?

    -Properties include: multiplication and addition are commutative and associative, subtraction and division are not. Subtraction/division are the inverse operations of addition/multiplication.

  • What are some benefits of memorizing multiplication tables?

    -Benefits include: faster at mental math, don't need to rely on technology like calculators, improves mental acuity and appreciation of number patterns.

  • What is the main message regarding use of technology for basic math?

    -The over-reliance on technology like phones for basic math is harmful - it reduces mental sharpness and misses the inherent patterns in numbers that mental math reveals.

  • What can be done going forward to improve mental math skills?

    -Practice basic arithmetic operations frequently. Memorize multiplication tables. Learn techniques for mental math calculations.

Outlines
00:00
😀 Understanding Multiplication and Division

This paragraph provides an introduction to multiplication and division operations. It explains how multiplication is an abbreviated form of repeated addition, with factors and a product. Division does the opposite, breaking down a larger number into smaller parts, with a dividend, divisor, and quotient. Key properties like commutativity and associativity are also discussed.

05:05
😃 Tips for Mental Math

This closing paragraph recommends practicing mental math instead of relying excessively on calculators. It promises future tips on mental math strategies. It then prompts readers to self-check their comprehension of the concepts covered.

Mindmap
Keywords
💡multiplication
Multiplication is combining multiple equal groups into a single number called a product. For example, 5 groups of 2 apples equals 10 total apples. Multiplication is related to repeated addition and is used as an abbreviated form when adding many equal groups.
💡division
Division is the inverse of multiplication, breaking down a larger number into equal groups or parts. For example, 15 apples divided into groups of 5 apples per basket results in 3 baskets total. Division solves problems involving partitioning or sharing amongst a number of groups.
💡factors
Factors are numbers that are multiplied together to get a product. For example, in the equation 2 x 5 = 10, 2 and 5 are factors that produce the product 10. Identifying the factor pairs of a number is key to understanding multiplication and division.
💡quotient
The quotient is the result of division. For example, when 15 is divided by 5, the quotient is 3, meaning there are 3 groups of size 5. The dividend is divided by the divisor to produce the quotient.
💡commutative
A commutative operation like multiplication can have its factors switched around but still produce the same result. For example, 2 x 5 = 5 x 2. Commutativity does not apply to subtraction and division.
💡associative
An associative operation like multiplication allows regrouping of factors without changing the product. For example, (2 x 3) x 4 = 2 x (3 x 4). Associativity does not apply to division.
💡arithmetic
Arithmetic refers to the basic numerical operations - addition, subtraction, multiplication, and division. Mastering arithmetic through practice builds mental acuity and pattern recognition with numbers.
💡properties
Properties refer to rules like commutativity and associativity that do or do not apply to operations like multiplication and division. Understanding properties provides insight into how numbers work together in different types of calculations.
💡memorize
Memorizing the multiplication tables through practice eliminates dependence on calculators for basic math. Instant recall of multiplication facts like 7 x 8 = 56 builds efficiencies for mental arithmetic.
💡patterns
There are inherent numerical patterns in the multiplication tables and other areas of arithmetic. Appreciating these patterns through practice without over-reliance on technology provides mental exercise and satisfaction.
Highlights

Multiplication can be viewed as an abbreviated form of repeated addition

Multiplication solves the problem of combining identical groups into a single larger number called the product

Division does the opposite of multiplication by breaking down a larger number into smaller ones

In multiplication, the numbers are called factors and the result is called the product

In division, the number being divided is the dividend, the number dividing is the divisor, and the result is the quotient

Multiplication is commutative - order of factors does not matter

Division is not commutative - order of division changes the result

Multiplication is associative - grouping of factors does not affect result

Division is not associative - order of successive divisions affects result

Memorizing multiplication tables improves mental math skills

Overreliance on technology weakens mental math abilities

Patterns are inherent in numbers

Addition, subtraction, multiplication and division are the four basic arithmetic operations

Understanding properties like commutativity and associativity comes from defining operations and observing

Practice makes math operations easier over time

Transcripts

Browse More Related Video

GED MATH 2024 Preparation Course - from the Absolute Beginning to Advanced Level

Math Videos: How To Learn Basic Arithmetic Fast - Online Tutorial Lessons

Mental Math Tricks - Addition, Subtraction, Multiplication & Division!

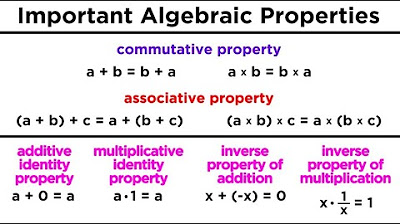
Basic Number Properties for Algebra

Math - Decimal Arithmetic

Terminology for the basic operations and writing algebraic expressions from words

Rate This

5.0 / 5 (0 votes)

Thanks for rating: