Infinite limits and asymptotes | Limits and continuity | AP Calculus AB | Khan Academy

Khan Academy
29 Jun 201704:13
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the mathematical concepts of vertical and horizontal asymptotes, using Desmos to visually demonstrate their relationship with limits. It explains how vertical asymptotes occur when a function approaches a non-zero value over zero, leading to an undefined limit, as seen when x approaches one in the function f(x) = 2/(x-1). In contrast, horizontal asymptotes represent limits that do exist, as the function value approaches a constant, even though the function may oscillate around it, such as y趋向于3 as x趋向于无穷大 in the provided example. The key distinction is that functions cannot cross vertical asymptotes but can cross horizontal ones while approaching them from either direction.

Takeaways
  • 📈 The video discusses the relationship between vertical and horizontal asymptotes in the context of limits using Desmos, an online graphing calculator.
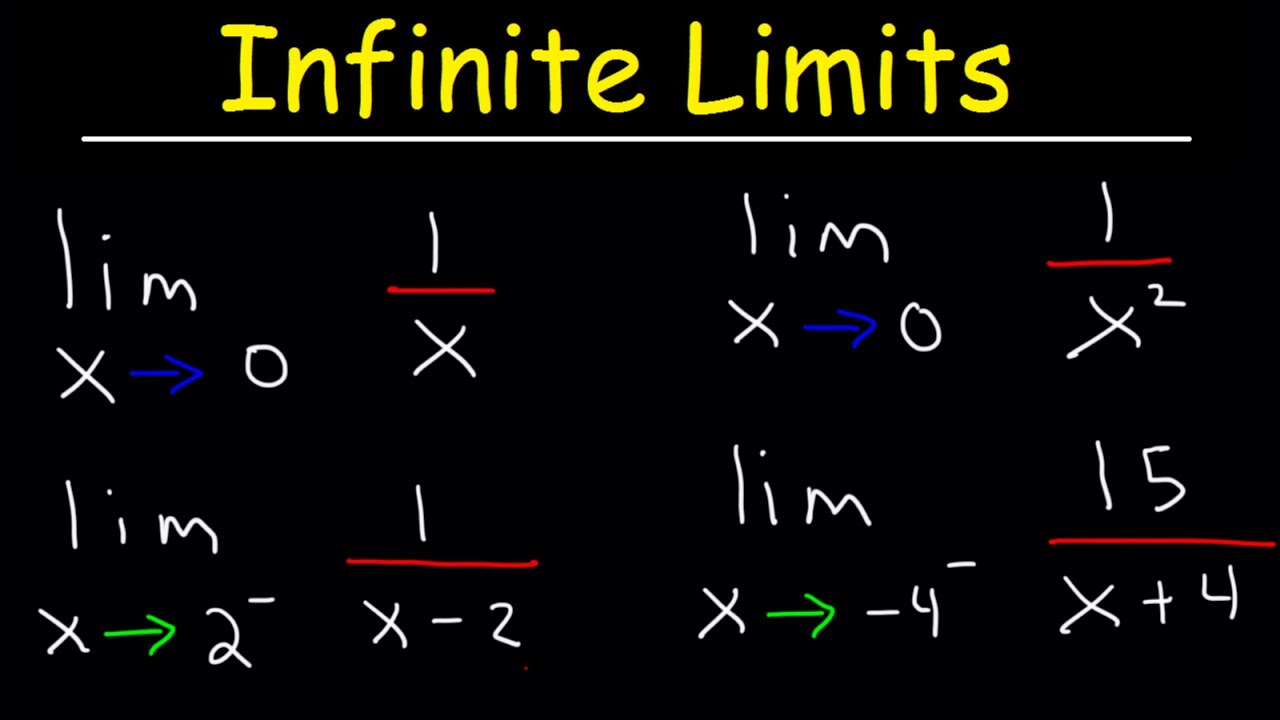
  • 🔍 The function 2/(x-1) is used to demonstrate the concept of a vertical asymptote at x=1, where the function approaches but never reaches the value of 2/0.
  • 💡 When x equals one, the function 2/(x-1) results in division by zero, indicating the presence of a vertical asymptote.
  • 👉 As x approaches one from the left, the function value tends towards negative infinity, showing that the limit is unbounded in the negative direction.
  • 👈 Similarly, as x approaches one from the right, the function value tends towards positive infinity, and the limit is unbounded in the positive direction.
  • ✋ The limit of 2/(x-1) as x approaches one does not exist, which is a characteristic of vertical asymptotes.
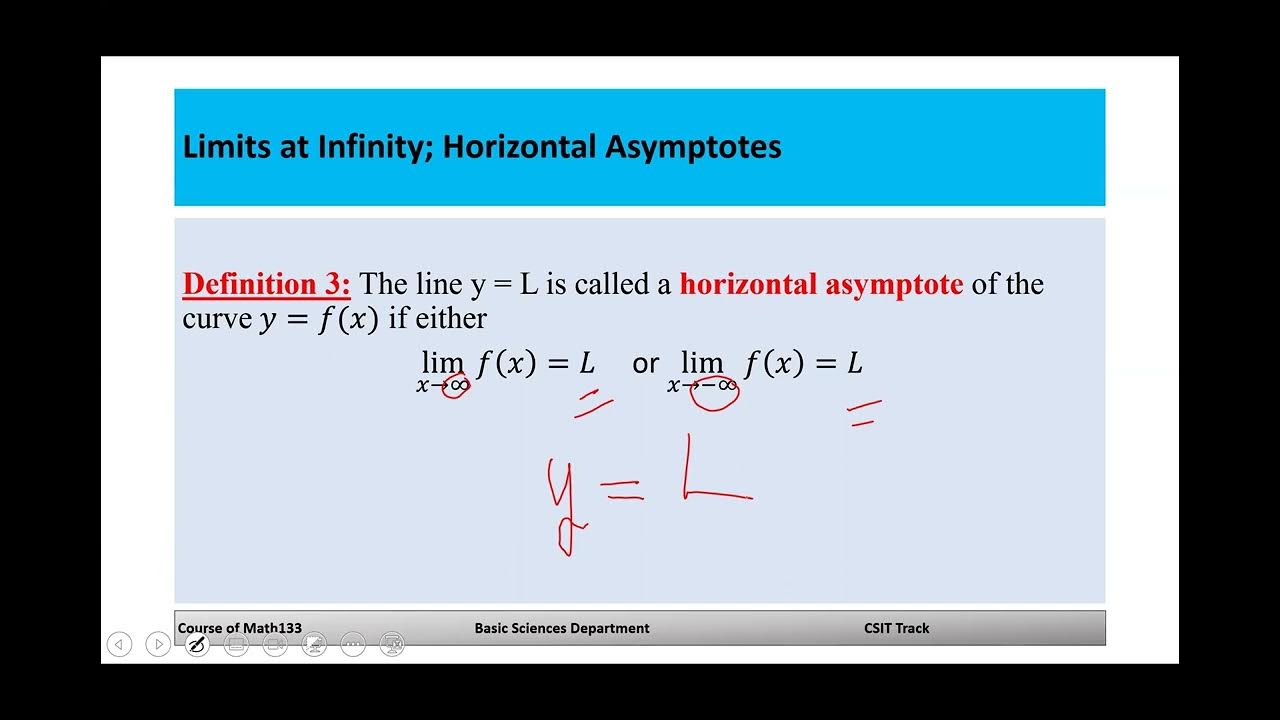
  • 🌟 The video introduces a horizontal asymptote by examining the behavior of a function as x approaches positive and negative infinity.
  • 📊 The function's value approaches three as x approaches infinity, indicating a horizontal asymptote at y=3.
  • 🔄 Even when a function oscillates around a horizontal asymptote, the limit can still exist, demonstrating a key difference from vertical asymptotes.
  • 🛑 Vertical asymptotes are lines that a function will not cross, whereas horizontal asymptotes can be crossed by the function as x values increase or decrease without bound.
Q & A

  • What is the relationship between vertical and horizontal asymptotes discussed in the video?

    -The video discusses how vertical and horizontal asymptotes relate to the concept of limits in calculus. Vertical asymptotes occur when a function approaches infinity or negative infinity as x approaches a certain value, while horizontal asymptotes represent values that a function approaches as x goes to positive or negative infinity.

  • What happens when x equals one in the function f(x) = 2/(x-1)?

    -When x equals one, the function f(x) = 2/(x-1) results in a division by zero, which is undefined. This indicates the presence of a vertical asymptote at x=1.

  • How does the behavior of f(x) = 2/(x-1) change as x approaches one from the left and right?

    -As x approaches one from the left, the function value becomes increasingly negative, suggesting an undefined limit in the negative direction. From the right, the function value becomes increasingly positive, indicating an undefined limit in the positive direction. This behavior is characteristic of a vertical asymptote.

  • What is the significance of the limit as x approaches one of f(x) = 2/(x-1)?

    -The limit as x approaches one of f(x) = 2/(x-1) does not exist. It is unbounded, with the function values going to negative infinity when approaching from the left and positive infinity when approaching from the right.

  • How does the video illustrate the concept of a horizontal asymptote?

    -The video uses a function that approaches a constant value of three as x goes to positive or negative infinity to illustrate a horizontal asymptote. It shows that even if the function oscillates around the horizontal asymptote, it continues to get closer to the asymptote's value as x increases.

  • Can a function with a horizontal asymptote cross the asymptote?

    -Yes, a function can cross a horizontal asymptote. The video demonstrates this by multiplying the function by the sine of x, causing it to oscillate around the horizontal asymptote.

  • What is the key difference between vertical and horizontal asymptotes?

    -The key difference is that a function cannot cross a vertical asymptote, whereas it can cross a horizontal asymptote while still approaching it from both positive and negative infinity.

  • How does the behavior of a function with a horizontal asymptote differ as x approaches infinity?

    -As x approaches infinity, the function with a horizontal asymptote gets closer and closer to the value of the asymptote, regardless of whether x is approaching from the positive or negative side.

  • What is the role of Desmos in the video?

    -Desmos is used as an online graphing calculator in the video to visually explore and demonstrate the relationships between vertical and horizontal asymptotes and the behavior of functions around these asymptotes.

  • How does the video explain the concept of limits in relation to asymptotes?

    -The video explains that limits provide a way to understand the behavior of functions near asymptotes. It shows how limits can be used to predict whether a function will approach a certain value (as with a horizontal asymptote) or become undefined (as with a vertical asymptote).

  • What is the significance of the sine function in the video's example of a function with a horizontal asymptote?

    -The sine function is used to demonstrate that even though a function can oscillate around a horizontal asymptote, it still approaches the asymptote's value as x increases indefinitely.

Outlines
00:00
📈 Exploring Asymptotes and Limits in Graphing

In this section, the instructor introduces the concept of using Desmos, an online graphing calculator, to investigate the relationship between vertical and horizontal asymptotes and their connection to limits. The focus is on the function f(x) = 2/(x-1), where the instructor demonstrates that at x=1, the function appears to have a vertical asymptote. This is deduced by observing that the function approaches negative infinity as x gets closer to 1 from the left, and positive infinity as x approaches 1 from the right, indicating an undefined limit. The instructor then contrasts this with a horizontal asymptote, using a different function that behaves such that its limit as x approaches infinity (or negative infinity) equals three. The key difference highlighted is that while vertical asymptotes are not crossed, horizontal asymptotes can be, yet the function continues to approach the asymptote's value as x increases indefinitely.

Mindmap
Keywords
💡Desmos
Desmos is an online graphing calculator used in the video to visually explore and graph mathematical functions. It is a tool that allows users to plot equations and observe their behavior, especially in relation to concepts like asymptotes and limits. In the video, Desmos is used to graph the function f(x) = 2/(x - 1) and to illustrate the vertical asymptote at x = 1.
💡Vertical Asymptote
A vertical asymptote is a line that the graph of a function approaches but never crosses as the variable approaches a certain value. In the context of the video, it is illustrated by the function f(x) = 2/(x - 1) where the graph approaches, but never intersects, the vertical line x = 1. The instructor explains that this is indicated by the function's undefined nature when x equals one, as substituting x with one results in a division by zero.
💡Limit
In mathematics, a limit is a fundamental concept that describes the behavior of a function as the input (or argument) approaches a particular value. The video discusses limits in relation to both vertical and horizontal asymptotes, explaining how the function's behavior changes as it approaches certain values. For instance, the limit of f(x) = 2/(x - 1) as x approaches 1 from the left is negative infinity, indicating that the function's output becomes increasingly negative without bound.
💡Horizontal Asymptote
A horizontal asymptote is a horizontal line that a graph of a function approaches as the variable approaches positive or negative infinity. In the video, the instructor creates a function that behaves such that its graph approaches the horizontal line y = 3 as x becomes very large or very small. This behavior signifies that regardless of how the function oscillates or fluctuates, its value tends towards three in the long run.
💡Infinity
Infinity is a concept representing an unbounded quantity, larger than any natural number. In the video, the term is used to describe the behavior of limits as the variable x approaches certain values. For example, the limit of the function f(x) = 2/(x - 1) as x approaches 1 from the left is negative infinity, indicating that the function's output grows without bound in the negative direction.
💡Undefined
In mathematics, an undefined value often arises when an operation is performed that has no meaningful result, such as division by zero. In the video, the instructor mentions that the limit of the function f(x) = 2/(x - 1) as x approaches 1 is undefined because substituting x with one results in division by zero, which has no finite or meaningful value.
💡Sine Function
The sine function is a trigonometric function that models periodic phenomena such as the motion of waves or the oscillation of a pendulum. In the video, the instructor multiplies the function that has a horizontal asymptote by the sine of x, resulting in a graph that oscillates around the horizontal asymptote. This demonstrates that even though the function crosses the horizontal asymptote, it still tends towards it as x becomes very large or very small.
💡Oscillation
Oscillation refers to the repetitive variation in size, form, or position of a quantity, often around an equilibrium or mean position. In the context of the video, the instructor illustrates how a function can oscillate around a horizontal asymptote by multiplying it with the sine function. This means that the function's value moves back and forth, crossing the horizontal asymptote but still approaching it in the limit as x approaches positive or negative infinity.
💡Graph
A graph is a visual representation of the relationship between variables, typically consisting of coordinates on a plane. In the video, graphs are used to visualize mathematical functions and their behavior in relation to asymptotes and limits. The instructor uses Desmos to graph functions and demonstrate how they interact with vertical and horizontal asymptotes.
💡Approach
In mathematics, to 'approach' a value means to get arbitrarily close to it without actually reaching it. The video discusses how functions approach certain values, particularly in the context of limits and asymptotes. For example, as x approaches 1 from the left in the function f(x) = 2/(x - 1), the function's value gets closer and closer to negative infinity, illustrating the concept of a vertical asymptote.
💡Trigonometry
Trigonometry is a branch of mathematics that deals with the relationships between the sides and angles of triangles, and by extension, the periodic behavior of functions like the sine function. In the video, the sine function is used to create a trigonometric function that oscillates around a horizontal asymptote, demonstrating the relationship between trigonometric functions and the behavior of mathematical graphs.
Highlights

Exploring the relationship between vertical and horizontal asymptotes in the context of limits.

Graphing the function f(x) = 2/(x-1) to observe the behavior at x=1.

Identifying a vertical asymptote at x=1 for the function 2/(x-1).

Discussing the limit of f(x) as x approaches 1 from the left, noting it is unbounded in the negative direction.

Explaining that the limit does not exist as x approaches 1 from the right, with the function approaching positive infinity.

Comparing vertical asymptotes to horizontal asymptotes and their relationship with limits.

Describing a function that approaches a horizontal asymptote at y=3 as x approaches infinity.

Observing the function's behavior as x approaches negative infinity, also approaching 3.

Noting that functions can cross a horizontal asymptote but not a vertical asymptote.

Demonstrating the oscillation around a horizontal asymptote by multiplying the function by the sine of x.

Highlighting that even when crossing, the limit of the function as x approaches infinity can still exist for a horizontal asymptote.

The key difference between horizontal and vertical asymptotes is that functions can cross horizontal but not vertical asymptotes.

The importance of understanding asymptotes for analyzing the behavior of functions, especially around singular points.

The practical application of understanding asymptotes in fields such as engineering and physics where limits and behavior of functions are crucial.

The use of Desmos as an online graphing calculator to visually explore mathematical concepts and their implications.

The educational value of visualizing mathematical functions and their asymptotic behavior for better comprehension.

Transcripts

Browse More Related Video

Infinite Limit vs Limits at Infinity of a Composite Function

Math 133 Lecture 2 6

Finding an Oblique Asymptote of a Rational Function (Precalculus - College Algebra 41)

How to graph a rational function using 6 steps

Infinite Limits and Vertical Asymptotes

How To Evaluate Limits From a Graph

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsAsymptotesLimitsDesmosGraphingVertical AsymptoteHorizontal AsymptoteInfinityFunction BehaviorEducational Content