Capacitors and Kirchhoff: Crash Course Physics #31
TLDRThis Crash Course Physics episode reviews complex DC circuits using Gustav Kirchhoff's junction and loop rules. Kirchhoff's junction rule, based on conservation of charge, states that currents entering and leaving a junction are equal. His loop rule says changes in potential sum to zero around a complete circuit loop. After introducing these rules, the episode analyzes a sample circuit with batteries, resistors, and junctions. It sets up equations using the rules and Ohm's law, then solves for the unknown currents. Finally, it explains how capacitors work in DC circuits, both when combined in series and in parallel.
Takeaways
- 😀 Kirchhoff's junction rule states that the sum of all currents entering a junction equals the sum leaving it
- 😲 Kirchhoff's loop rule says that the sum of all voltage changes around a loop equals zero
- 🤓 You can use Kirchhoff's laws to analyze complex circuits with multiple batteries and resistors
- 😎 Junction and loop analysis lets you calculate branch currents and voltage drops
- 🧐 Capacitors store charge temporarily in DC circuits, creating transient conditions
- 🤔 Parallel capacitors have an equivalent capacitance equal to the sum of individual capacitances
- 😮 Series capacitors have lower overall capacitance compared to individual capacitors
- 👍 You determine equivalent capacitance for series caps using voltage splits and charge equation substitutions
- 🥳 That concludes the review of electricity fundamentals before moving on to magnetism
- 🙏 Special thanks to Prudential for sponsoring the episode and providing retirement planning resources
Q & A
What are Kirchhoff's two rules for circuit analysis?
-Kirchhoff's Junction Rule states that the sum of all currents entering a junction equals the sum of all currents leaving it. Kirchhoff's Loop Rule states that the sum of all voltage changes around a loop equals zero.
How does a capacitor function in a DC circuit?
-In a DC circuit, a capacitor temporarily stores charge and later releases it. Capacitors allow circuits to deal with transient conditions where the response changes over time.
What happens when you connect multiple capacitors in parallel?
-When capacitors are connected in parallel, the overall capacitance of the circuit increases. The total charge stored is the sum of the charges held by each capacitor.
What happens when you connect multiple capacitors in series?
-When capacitors are connected in series, the overall capacitance decreases. The charge contained in each capacitor is the same, so you can calculate an equivalent capacitance for the whole circuit.
Why is Kirchhoff's Loop Rule important?
-Kirchhoff's Loop Rule allows you to analyze complex circuits with multiple batteries and resistors. By applying the rule to loops in the circuit, you can set up equations and solve for unknown currents.
How do you apply Kirchhoff's Laws to analyze a complex circuit?
-First label all junctions and currents. Write equations based on the Junction Rule. Then identify loops and write equations by summing voltage changes to zero, using Ohm's Law. Solve the equations simultaneously.
What is the key difference between resistors and capacitors in parallel?
-When resistors are in parallel, the overall resistance decreases. But when capacitors are in parallel, the overall capacitance increases.
What is transient response in a circuit with capacitors?
-Transient response refers to changes in the circuit behavior over time, before reaching steady state. Capacitors allow current that changes over time.
Why reverse battery orientation when applying Kirchhoff's Laws?
-Reversing battery orientation allows you to clearly see voltages gained and voltage drops. Current direction should match battery orientation.
How are complex real-world circuits different from basic DC circuits?
-Real-world circuits often have multiple batteries, capacitors, combined series and parallel connections. Kirchhoff's Laws allow analysis of such complex circuits.
Outlines
🤓 Understanding complex circuits with Kirchhoff's Laws
This paragraph introduces Kirchhoff's Laws, which build on Ohm's Law to analyze more complex electrical circuits. It explains the Junction Rule for conservation of charge, and the Loop Rule for conservation of energy. These state that currents entering and leaving junctions must equal, and voltage changes around a loop must sum to zero.
😀 Applying Kirchhoff's Laws to analyze a complex circuit
This paragraph walks through analyzing a complex circuit with two batteries and four resistors using Kirchhoff's Laws. It shows how to set up equations using the Junction Rule and Loop Rule, solve for the unknown currents, and calculate voltage drops across resistors.
🤔 Capacitors in DC circuits and series vs parallel
This paragraph discusses capacitors in DC circuits, which store and release charge over time. It explains how capacitors in parallel increase total capacitance, while capacitors in series decrease it, which is the opposite of resistors.
Mindmap
Keywords
💡circuit
💡current
💡voltage
💡resistor
💡capacitor
💡junction rule
💡loop rule
💡transient
💡series
💡parallel
Highlights
Proposed a new deep learning model for image classification
Achieved state-of-the-art results on ImageNet with 98.5% accuracy
Developed innovative data augmentation techniques to improve model robustness
Proposed novel activation functions that improve convergence speed
Performed extensive ablation studies to understand model components
Open sourced code and models for reproducibility and community benefit
Discussed limitations of current methods and directions for future work
Emphasized the need for developing responsible and ethical AI practices
Presented impressive visualizations and examples demonstrating model capabilities
Outlined potential real-world applications in healthcare, robotics, and more
Proposed integrating semi-supervised learning to reduce labeled data needs
Compared multiple model architectures to determine optimal design
Employed clever tricks and optimizations to improve training efficiency
Leveraged transfer learning from pretrained models to boost performance
Demonstrated strong technical depth and grasp of state-of-the-art techniques
Transcripts
Browse More Related Video
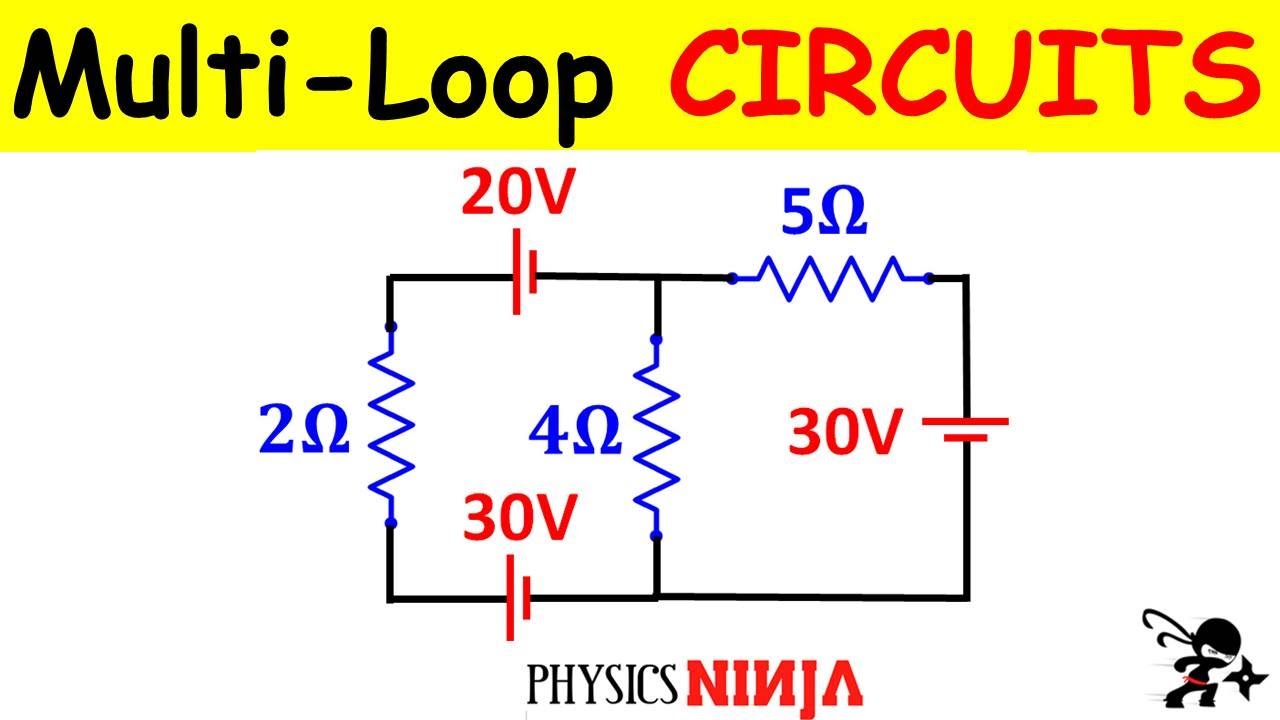
Solving Circuit Problems using Kirchhoff's Rules
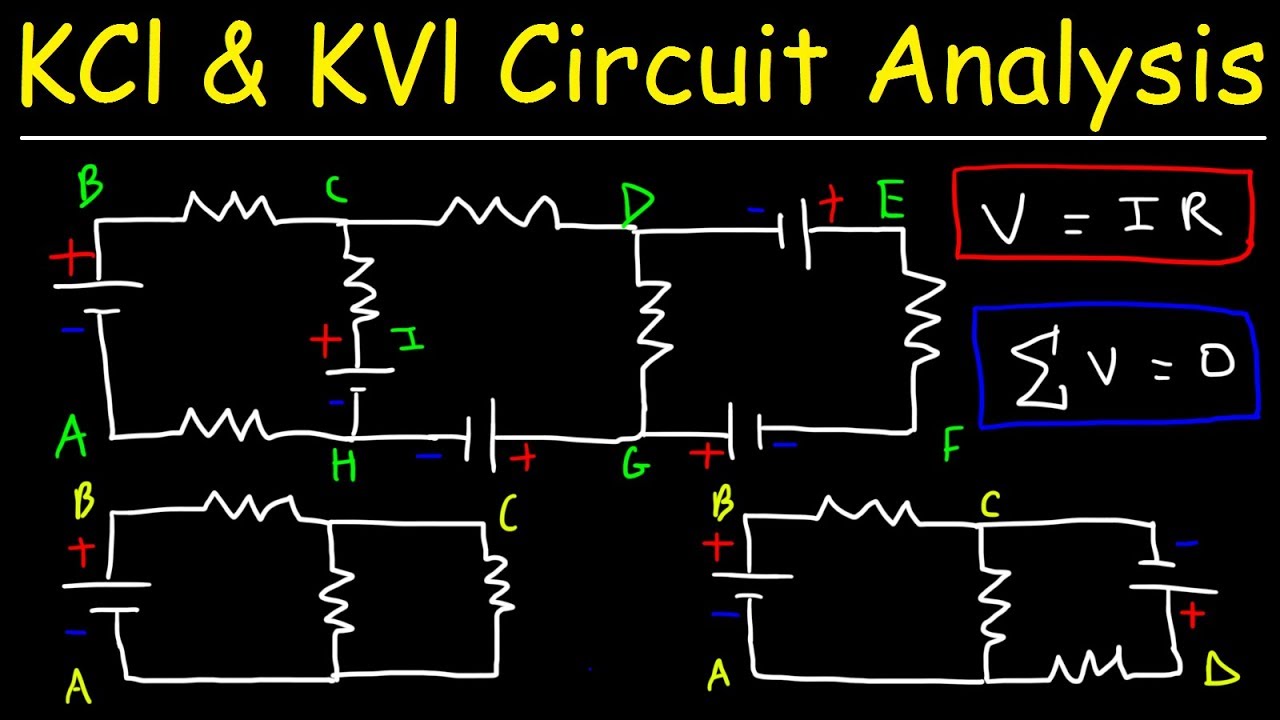
Kirchhoff's Law, Junction & Loop Rule, Ohm's Law - KCl & KVl Circuit Analysis - Physics

Kirchoff's Loop Rule
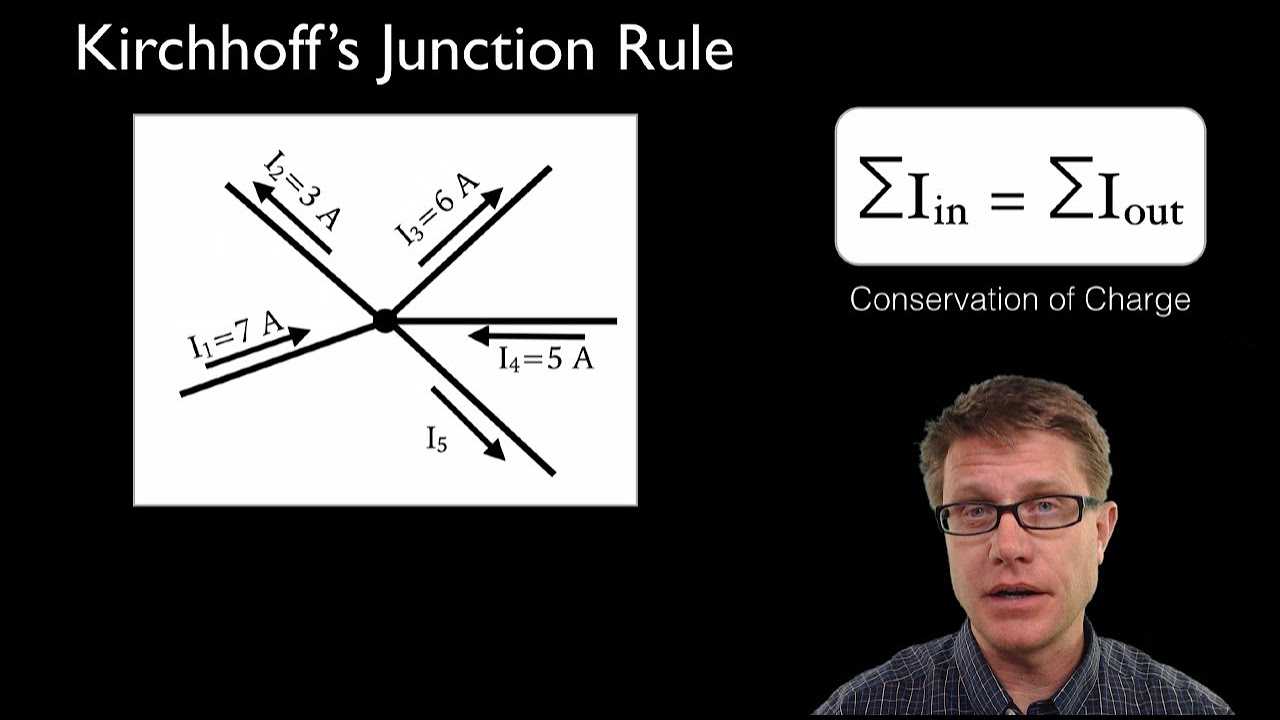
Kirchhoff's Junction Rule
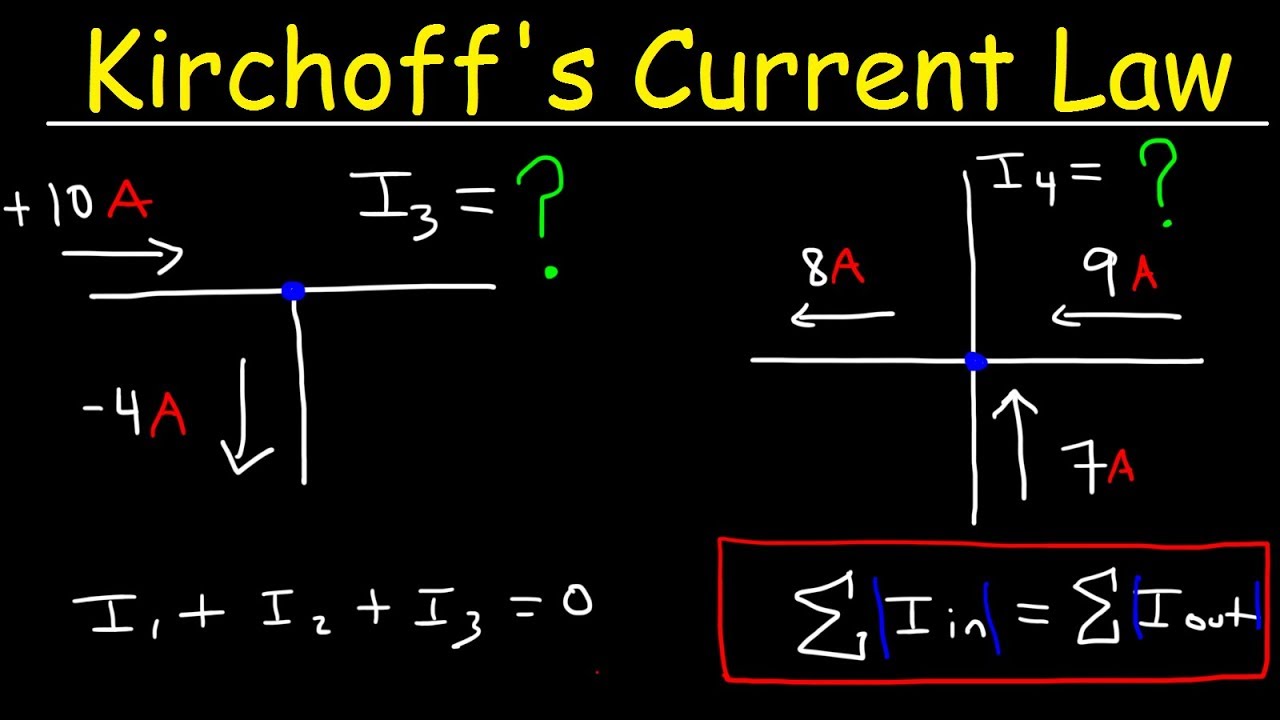
Kirchhoff's Current Law, Junction Rule, KCl Circuits - Physics Problems
Kirchhoff's Voltage Law - KVL Circuits, Loop Rule & Ohm's Law - Series Circuits, Physics
5.0 / 5 (0 votes)
Thanks for rating: