Calculus Polar Area Region Between Curves Example

turksvids

14 Dec 201303:30

EducationalLearning

32 Likes 10 Comments

TLDRThis video demonstrates how to find the area between two polar curves, specifically inside R = 3sin(θ) and outside R = 1 + sin(θ). The instructor explains the process by first determining the points where the curves intersect. Then, they find the area by integrating from π/6 to π/2, focusing on the larger region and subtracting the smaller region. The method is likened to finding volumes of revolution. The instructor emphasizes using a calculator for integration and notes the relevance of power-reducing formulas. The result is confirmed to be π.

Takeaways

📐 The video demonstrates how to find the area between two polar curves.
🎨 The speaker starts by drawing a picture to visualize the problem.
📊 The curves being analyzed are \( R = 3 \sin(\theta) \) and \( R = 1 + \sin(\theta) \).
🔄 The points where the curves intersect are found at \( \theta = \pi/6 \) and \( \theta = 5\pi/6 \).
📏 The speaker decides to find half of the region first and then double it to simplify calculations.
➕ The integral for the area is set up from \( \pi/6 \) to \( \pi/2 \).
➖ The area is calculated by integrating the outer curve and subtracting the inner curve.
🧮 The larger region is given by \( 3 \sin(\theta) \) and the smaller by \( 1 + \sin(\theta) \).
💻 The speaker prefers using a calculator for the final integration and obtains an area of \( \pi \).
📚 The video touches on using power-reducing formulas when calculating by hand.
✏️ The speaker notes that the problem could also be solved in one integral from \( \pi/6 \) to \( 5\pi/6 \) with the same result.

Q & A

What is the goal of the example in the video?
-The goal is to find the region between two polar curves: inside \( R = 3 \sin(\theta) \) and outside \( R = 1 + \sin(\theta) \).
Why is it important to find where the two curves are equal?
-Finding where the curves are equal helps identify the 'collision points,' which are critical in understanding the region's boundaries.
What are the values of \( \theta \) where the two curves intersect?
-The two curves intersect at \( \theta = \pi/6 \) and \( \theta = 5\pi/6 \).
How does the speaker approach solving the problem?
-The speaker draws a picture to visualize the region, identifies the intersection points, and decides to find the area of half the region first and then double it.
What is the integral setup used to find the area of the region?
-The integral setup is \( 2 \times \frac{1}{2} \int_{\pi/6}^{\pi/2} \left( (3 \sin(\theta))^2 - (1 + \sin(\theta))^2 \right) d\theta \).
Why does the speaker prefer to find half of the region's area and then double it?
-This method simplifies the calculation by eliminating the \( \frac{1}{2} \) factor in front of the area integral.
What analogy does the speaker use to explain finding the area between two polar curves?
-The speaker compares it to finding volumes of revolution with two curves, where you first square the terms and then subtract them.
What tools does the speaker use to solve the integral?
-The speaker uses a calculator to solve the integral, mentioning that doing it by hand would require using power-reducing formulas.
What is the final result of the integral calculation?
-The final result of the integral calculation is \( \pi \).
How does the speaker suggest an alternative approach to solving the problem?
-The speaker suggests that the problem could also be solved in one shot using \( \frac{1}{2} \int_{\pi/6}^{5\pi/6} \left( (3 \sin(\theta))^2 - (1 + \sin(\theta))^2 \right) d\theta \), yielding the same answer.

Outlines

00:00

🔍 Introduction to Finding Polar Areas

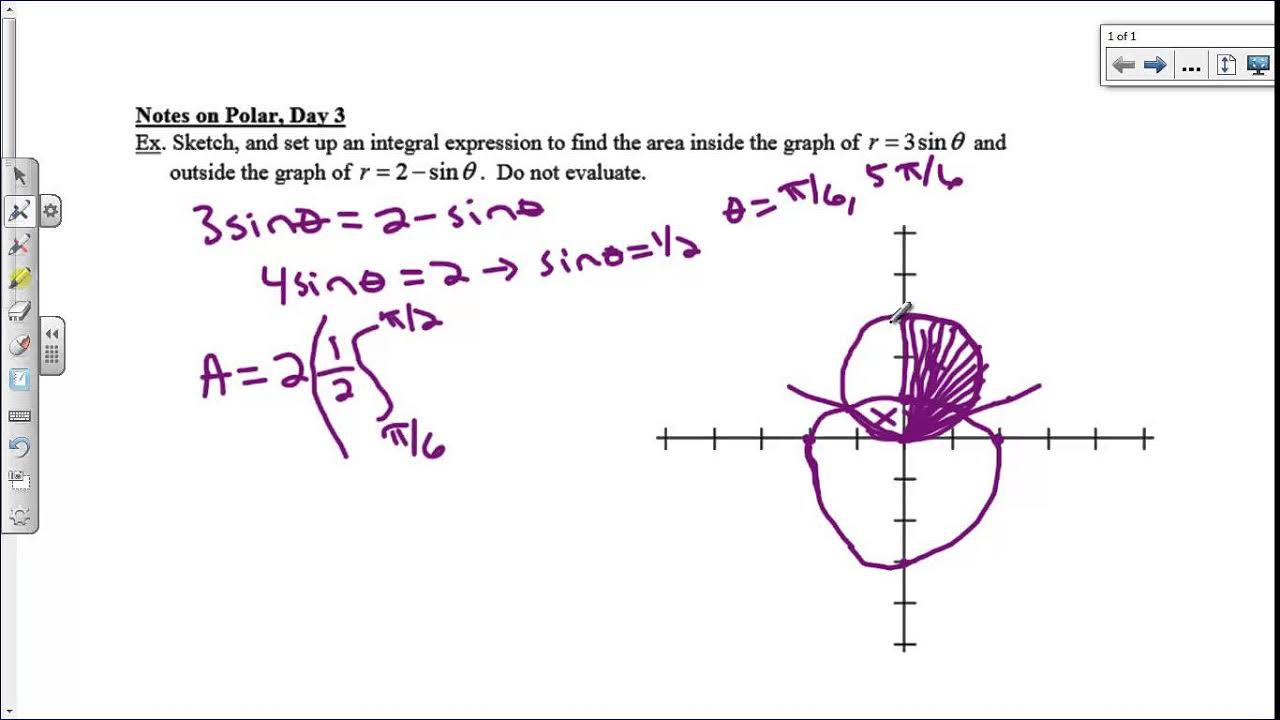
In this video, we're exploring the process of finding polar areas. Specifically, we will focus on the region between two polar curves: the area inside \( R = 3 \sin(\theta) \) and outside \( R = 1 + \sin(\theta) \). The aim is to solve this by visualizing the problem through a diagram, despite the diagram not being perfect.

🔢 Identifying Intersection Points

First, we need to determine where the two polar curves intersect. These points, where \( 3 \sin(\theta) \) equals \( 1 + \sin(\theta) \), are critical for understanding the problem. Solving for \(\theta\), we find the intersection points at \( \theta = \pi/6 \) and \( \theta = 5\pi/6 \).

📝 Visualizing and Simplifying the Region

To better understand the region, we consider radii at \( \theta = \pi/6 \) and \( \theta = \pi/2 \), shading the region between these bounds. By focusing on one half of the region and then doubling the result, we simplify the problem. This approach helps eliminate the constant 1/2 factor in the area integral.

📏 Setting Up the Integral

We set up the integral for the area from \( \theta = \pi/6 \) to \( \theta = \pi/2 \). The process involves drawing a radius from the origin to the outer curve \( 3 \sin(\theta) \) and subtracting the area up to the inner curve \( 1 + \sin(\theta) \). This is akin to calculating volumes of revolution where we subtract one curve from another.

🔄 Solving the Integral

To find the area, we set up the integral as twice the integral from \( \pi/6 \) to \( \pi/2 \) of \([ (3 \sin(\theta))^2 - (1 + \sin(\theta))^2 ] \, d\theta \). Using a calculator, the result is \(\pi\). If done by hand, power-reducing formulas for trigonometric functions, especially \(\cos(2\theta)\), would be essential.

✅ Alternative Method and Conclusion

An alternative method involves calculating the integral in one shot from \( \pi/6 \) to \( 5\pi/6 \) using the same integrand. This would yield the same result. The video concludes with a recap of the process and encouragement to the viewer. The final answer, whether using one or two steps, confirms the area as \(\pi\).

Mindmap

Keywords

💡Polar Curves

Polar curves are graphs of functions expressed in polar coordinates, where each point is determined by a distance from the origin and an angle. In the video, the problem involves finding the region between two polar curves: R = 3 sin(θ) and R = 1 + sin(θ).

💡Area in Polar Coordinates

Calculating the area in polar coordinates involves integrating the square of the radius function over the given range of angles. The video demonstrates finding the area inside one polar curve and outside another using integration techniques specific to polar coordinates.

💡Intersection Points

Intersection points are where two curves meet, having the same radius at the same angle. Identifying these points is crucial for setting the bounds of integration. The video finds the intersection points of the given polar curves at θ = π/6 and θ = 5π/6.

💡Integration Bounds

Integration bounds in this context are the angles between which the area is calculated. For the problem in the video, the bounds are from θ = π/6 to θ = π/2. These bounds define the region between the intersection points and where the calculation stops.

💡Radius

In polar coordinates, the radius is the distance from the origin to a point on the curve. The video involves drawing radii to understand the region between the curves and using the radius values in the integral to find the area.

💡Subtracting Areas

Subtracting areas involves finding the difference between the areas enclosed by two curves. The video subtracts the area inside the smaller polar curve from the area inside the larger polar curve to find the desired region.

💡Doubling the Area

Doubling the area is a technique used to simplify the integration process. By calculating the area of half the region and then doubling it, the video avoids dealing with the factor of 1/2 in the polar area formula.

💡Power-Reducing Formulas

Power-reducing formulas are trigonometric identities used to simplify integrals involving trigonometric functions. The video mentions using these formulas, particularly for cosine of 2θ, which are important for hand calculations in polar coordinates.

💡Volume of Revolution

The volume of revolution is a method in calculus for finding the volume of a solid formed by rotating a curve around an axis. The video draws an analogy between this method and finding areas between polar curves, highlighting the similar process of squaring functions and subtracting results.

💡Calculator

A calculator is used to perform complex integrals and arithmetic operations quickly and accurately. In the video, the speaker uses a calculator to find the final area value, emphasizing the practicality of computational tools in solving intricate mathematical problems.

Highlights

Introduction to finding polar areas between two curves.

Example problem: finding the area inside r = 3sin(θ) and outside r = 1 + sin(θ).

Importance of understanding where the curves intersect.

Intersection points identified at θ = π/6 and θ = 5π/6.

Visual representation helps in solving the problem.

Strategy: find half the region and then double it to simplify calculations.

Setup of the integral for the area calculation from θ = π/6 to θ = π/2.

Use of radius from the origin to the outer curve (3sin(θ)).

Calculation involves subtracting the smaller area (1 + sin(θ)) from the larger area.

Comparison to finding volumes of revolution with two curves.

Integral setup: 2 * (1/2 * integral from π/6 to π/2 of (3sin(θ))^2 - (1 + sin(θ))^2).

Emphasis on using a calculator for solving the integral.

Result of the integral calculation: π.

Mention of power reducing formulas for manual calculations.

Alternative method: single integral from π/6 to 5π/6.

Final answer verification and closing remarks.

Transcripts

Browse More Related Video

Notes on Polar, Day 3

Area Between the Graphs of Sine and Cosine | MIT 18.01SC Single Variable Calculus, Fall 2010

AP Precalculus Practice Exam Question 15

Arc Length of a Circle Formula - Sector Area, Examples, Radians, In Terms of Pi, Trigonometry

Area Between Two Curves with Multiple Regions: y = x^3-3x^2 and y = x-3

Calculus Polar Area Rose Curve Example