AP Precalculus Practice Exam Question 15

NumWorks
23 May 202303:52
EducationalLearning
32 Likes 10 Comments

TLDRThe transcript explains how to convert a complex number from rectangular coordinates to polar coordinates. The complex number (3, 3) is first represented in rectangular form as 3 + 3i. Using formulas, r is found as 3√2 and θ as π/4. The final expression in polar form is 3√2 (cos(π/4) + i sin(π/4)). The explanation includes steps for calculating r and θ, emphasizing the quadrant where the coordinates lie.

Takeaways
  • 📚 The script discusses converting a complex number from rectangular to polar form.
  • 📐 The complex number given is in the complex plane with coordinates (3, 3).
  • 🔍 Rectangular form of a complex number is represented as x + yi.
  • 📈 To find the polar form, formulas for r and theta are used: r^2 = x^2 + y^2 and tan(theta) = y/x.
  • 🔢 Given x = 3 and y = 3, the calculation for r^2 is 3^2 + 3^2, resulting in r = √18, which simplifies to 3√2.
  • 📉 The tangent of theta is calculated as 3/3, which equals 1, indicating theta could be π/4 or 3π/4.
  • 📍 Considering the complex number's position in the first quadrant, theta is π/4.
  • 🌐 The polar form of the complex number is expressed as r(cos(theta) + i*sin(theta)).
  • 📝 The final polar form of the given complex number is 3√2(cos(π/4) + i*sin(π/4)).
  • 🔑 The script emphasizes the importance of understanding the relationship between rectangular and polar coordinates for complex numbers.
  • 📌 The process involves both algebraic manipulation and understanding of trigonometric functions in the context of complex numbers.
Q & A

  • What is the standard format for representing a complex number in the complex plane?

    -The standard format for representing a complex number in the complex plane is in rectangular form, which is 'x + yi', where 'x' is the real part and 'y' is the imaginary part.

  • What are the polar coordinates of a complex number?

    -The polar coordinates of a complex number are represented by 'r' and 'θ', where 'r' is the magnitude (or modulus) of the complex number and 'θ' is the angle (or argument) with respect to the positive real axis.

  • How can you find the magnitude 'r' of a complex number given its rectangular coordinates?

    -You can find the magnitude 'r' of a complex number by using the formula r = sqrt(x^2 + y^2), where 'x' and 'y' are the rectangular coordinates.

  • What is the formula for finding the angle 'θ' of a complex number from its rectangular coordinates?

    -The angle 'θ' can be found using the formula tan(θ) = y/x, where 'x' and 'y' are the rectangular coordinates. The actual angle 'θ' must be determined considering the quadrant in which the complex number lies.

  • What is the magnitude 'r' of the complex number with rectangular coordinates (3, 3)?

    -The magnitude 'r' of the complex number with rectangular coordinates (3, 3) is 3√2, which is calculated as sqrt(3^2 + 3^2) = sqrt(18).

  • What are the possible values of 'θ' for a complex number with a tangent of 1?

    -The possible values of 'θ' for a complex number with a tangent of 1 are π/4 and 3π/4 radians, which correspond to angles in the first and third quadrants, respectively.

  • Why are only the angles π/4 and 3π/4 considered for the complex number with coordinates (3, 3)?

    -Only π/4 is considered for the complex number with coordinates (3, 3) because it lies in the first quadrant where both 'x' and 'y' are positive, and the tangent of π/4 is 1.

  • How can you express a complex number in polar form using 'r' and 'θ'?

    -A complex number can be expressed in polar form as r(cos(θ) + i*sin(θ)), where 'r' is the magnitude and 'θ' is the angle.

  • What are the formulas to convert from polar to rectangular coordinates for a complex number?

    -The formulas to convert from polar to rectangular coordinates are x = r*cos(θ) and y = r*sin(θ), where 'r' is the magnitude and 'θ' is the angle.

  • What is the polar form of the complex number with rectangular coordinates (3, 3)?

    -The polar form of the complex number with rectangular coordinates (3, 3) is 3√2(cos(π/4) + i*sin(π/4)).

  • How can the angle 'θ' be determined more precisely if the complex number is on the boundary of the quadrants?

    -If the complex number is on the boundary of the quadrants, the angle 'θ' can be determined more precisely by considering the signs of 'x' and 'y' and using the atan2 function, which takes into account the quadrant.

Outlines
00:00
📚 Conversion of Rectangular to Polar Form of a Complex Number

This paragraph explains the process of converting a complex number from its rectangular form to polar form. The complex number in question has rectangular coordinates (3, 3). The explanation begins with the basic representation of a complex number in rectangular form, \( x + yi \), and the goal of expressing it in polar form, \( r(\cos\theta + i\sin\theta) \). The formulas for finding \( r \) and \( \theta \) are introduced: \( r^2 = x^2 + y^2 \) and \( \tan(\theta) = \frac{y}{x} \). Using the given coordinates, \( r \) is calculated as \( \sqrt{18} \), which simplifies to \( 3\sqrt{2} \). For \( \theta \), since both x and y are positive and equal, the tangent of \( \theta \) is 1, indicating \( \theta = \frac{\pi}{4} \) in the first quadrant. The paragraph concludes with the polar form of the complex number, \( 3\sqrt{2}\cos(\frac{\pi}{4}) + i3\sqrt{2}\sin(\frac{\pi}{4}) \).

Mindmap
Keywords
💡Complex Number
A complex number is a number that can be expressed in the form of a + bi, where 'a' and 'b' are real numbers and 'i' is the imaginary unit with the property that i^2 = -1. In the context of the video, complex numbers are discussed in relation to their representation in the complex plane, which is a geometric way to visualize and work with these numbers.
💡Complex Plane
The complex plane, also known as the Argand plane, is a two-dimensional coordinate system that represents complex numbers with a horizontal axis for the real part and a vertical axis for the imaginary part. The video script uses the complex plane to illustrate the location of a complex number with rectangular coordinates (3, 3).
💡Rectangular Coordinates
Rectangular coordinates refer to the representation of a point in a two-dimensional space using an ordered pair (x, y), where 'x' is the horizontal distance and 'y' is the vertical distance from the origin. In the script, the complex number is initially given in rectangular form as (3 + 3i), with 3 being both the real and imaginary components.
💡Polar Coordinates
Polar coordinates are a two-dimensional coordinate system in which each point is determined by a distance from a reference point, called the origin, and an angle from a reference direction, typically the positive horizontal axis. The video explains how to convert a complex number from rectangular to polar form, which involves finding the radius 'r' and angle 'θ'.
💡Radius (r)
In polar coordinates, the radius 'r' is the distance from the origin to the point representing the complex number. The script calculates the radius by taking the square root of the sum of the squares of the real and imaginary parts, resulting in √(3^2 + 3^2) = 3√2 for the given complex number.
💡Theta (θ)
Theta, often denoted by the symbol 'θ', is the angle in polar coordinates that represents the direction of a point from the origin. The script uses the tangent function to find θ, which is the arctangent of the imaginary part divided by the real part, resulting in π/4 for the given complex number.
💡Tangent
The tangent of an angle in a right-angled triangle is the ratio of the length of the opposite side to the length of the adjacent side. In the context of the script, the tangent of theta is used to find the angle θ for the complex number, where tan(θ) = y/x, which simplifies to 1 for the given (3, 3) coordinates.
💡First Quadrant
The first quadrant of the Cartesian coordinate system is the region where both the x (real part) and y (imaginary part) are positive. The script mentions that the complex number's coordinates (3, 3) are in the first quadrant, which simplifies the determination of the angle θ to π/4, as it is the only angle in the first quadrant where the tangent is 1.
💡Cosine
Cosine is a trigonometric function that relates the angle of a right-angled triangle to the ratio of the adjacent side over the hypotenuse. In the script, cosine is used to express the real part of the complex number in polar form as r cos(θ), where r is the radius and θ is the angle.
💡Sine
Sine is another trigonometric function that relates the angle of a right-angled triangle to the ratio of the opposite side over the hypotenuse. The script uses sine to express the imaginary part of the complex number in polar form as r sin(θ), with the same radius and angle as in the cosine expression.
Highlights

A complex number can be represented as a point in the complex plane.

Complex numbers are typically expressed in rectangular form as x + iy.

The goal is to convert the complex number to polar form using r and theta.

Formulas for converting rectangular to polar form: r^2 = x^2 + y^2 and tan(theta) = y/x.

The given complex number has rectangular coordinates (3, 3).

Calculating r: r^2 = 3^2 + 3^2 = 18, so r = √18 = 3√2.

Finding theta: tan(theta) = 3/3 = 1, which corresponds to angles π/4 and 3π/4.

Since the point is in the first quadrant, theta = π/4 is the relevant angle.

Expressing the complex number in polar form: r(cos(theta) + i*sin(theta)).

Using the formulas x = r*cos(theta) and y = r*sin(theta).

The polar form of the given complex number is 3√2(cos(π/4) + i*sin(π/4)).

The process demonstrates the conversion of a complex number from rectangular to polar form.

Understanding the relationship between x, y coordinates and r, theta in polar form.

Applying trigonometric identities to find the polar form of a complex number.

The importance of considering the quadrant when determining the angle theta.

The final expression of the complex number in polar form is a concise representation.

Transcripts

Browse More Related Video

Polar coordinates 2 | Parametric equations and polar coordinates | Precalculus | Khan Academy

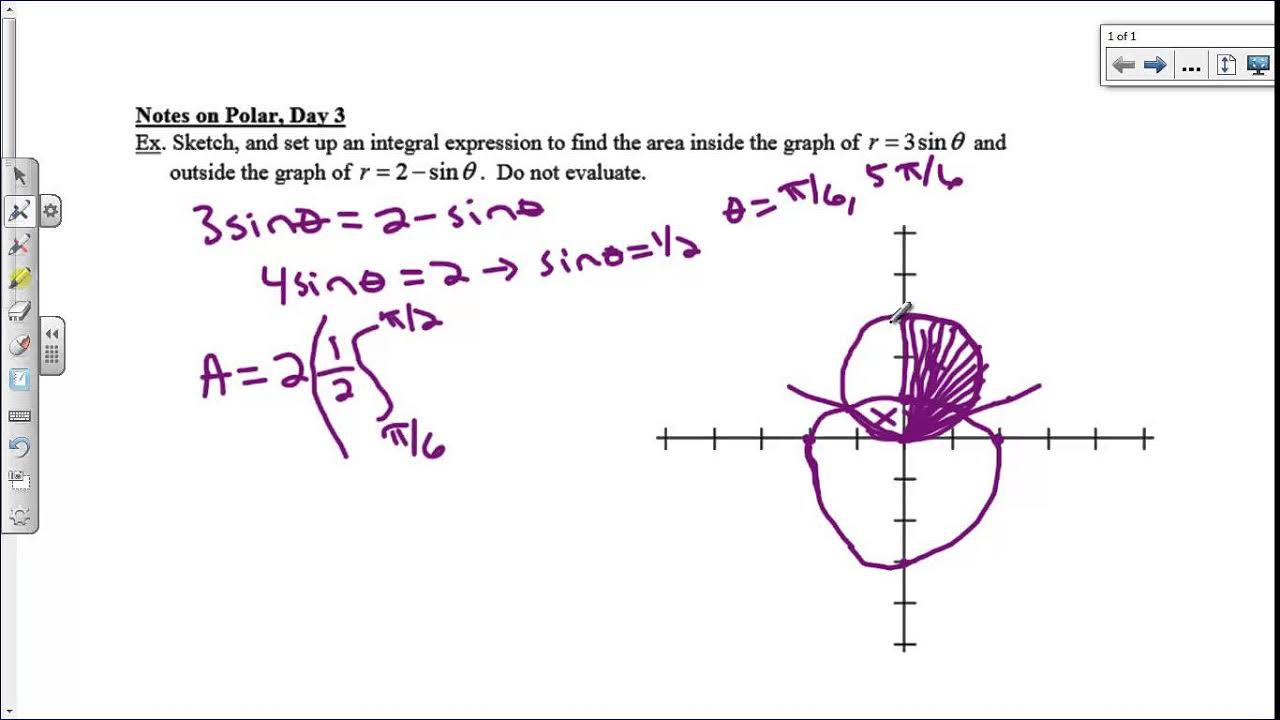
Notes on Polar, Day 3

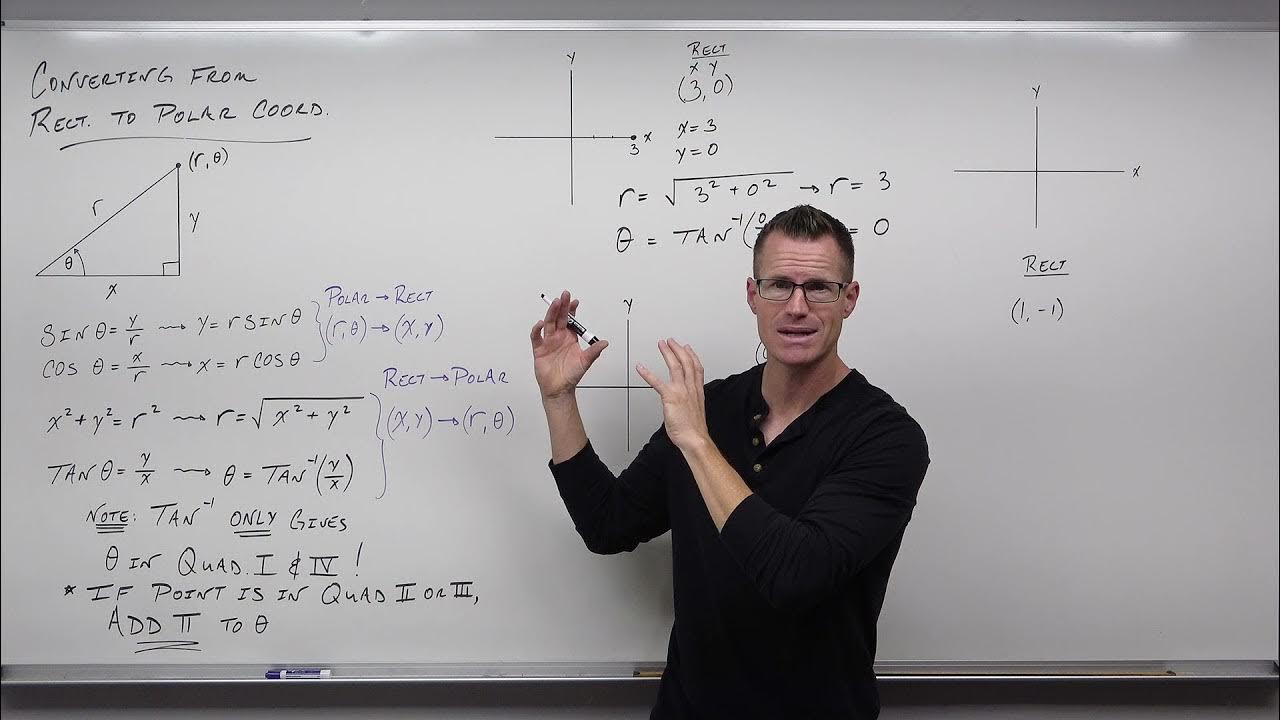
How to Convert From Rectangular Coordinates to Polar Coordinates (Precalculus - Trigonometry 38)

How to Convert From Rectangular Equations to Polar Equations (Precalculus - Trigonometry 39)

Polar coordinates 3 | Parametric equations and polar coordinates | Precalculus | Khan Academy

AP Precalculus Practice Exam Question 26

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Complex NumbersPolar CoordinatesRectangular FormMathematicsTrigonometryConversion GuideEducational ContentFirst QuadrantTangent FunctionTheta CalculationR Value