Area Between the Graphs of Sine and Cosine | MIT 18.01SC Single Variable Calculus, Fall 2010

MIT OpenCourseWare
7 Jan 201103:59
EducationalLearning
32 Likes 10 Comments

TLDRIn this recitation, the professor explores the concept of finding areas between curves, specifically focusing on the region between y=sin(x) and y=cos(x). The area of interest is between their first two consecutive intersection points at π/4 and 5π/4. Utilizing the symmetry of the functions, the professor demonstrates the integration process from π/4 to 5π/4 of (sin(x) - cos(x)), resulting in an intriguing area calculation that equals 2 times the square root of 2, illustrating the beauty of integration in understanding geometric shapes.

Takeaways
  • 📚 The lecture is about applications of integration, specifically finding the area between curves.
  • 📈 The region of interest is between the curves y = sin(x) and y = cos(x), which intersect at specific points.
  • 🔍 The focus is on the area between two consecutive intersection points, specifically at x = π/4 and x = 5π/4.
  • 🌀 The curves sin(x) and cos(x) are symmetrical and wrap around each other, creating a region of interest.
  • 📐 The integration bounds are set from π/4 to 5π/4 due to the symmetry and ease of calculation.
  • 📝 The method involves viewing the region as a series of rectangles and integrating the height of these rectangles to find the total area.
  • 📉 The upper curve is y = sin(x) and the lower curve is y = cos(x), with the height of each rectangle being sin(x) - cos(x).
  • ∫ The integral to find the area is ∫(sin(x) - cos(x)) dx from π/4 to 5π/4.
  • 🔢 The integral involves finding the antiderivative of sin(x) - cos(x), which simplifies to -cos(x) - sin(x).
  • 📌 The final calculation involves plugging in the bounds of integration and simplifying the result.
  • 🎯 The computed area of the region is found to be 2 times the square root of 2, assuming no calculation errors.
Q & A

  • What is the main topic of the recitation session?

    -The main topic of the recitation session is the application of integration, specifically finding the areas between curves.

  • What are the two functions mentioned in the script that form the region of interest?

    -The two functions mentioned are y = sine(x) and y = cosine(x).

  • Why does the professor describe the region as 'kind of cute'?

    -The professor finds the region cute because the two curves, sine and cosine, cross each other repeatedly and wrap around each other, forming an interesting pattern.

  • What are the two consecutive points where the sine and cosine functions cross each other that the professor chooses for the region of integration?

    -The professor chooses the points at pi/4 and 5*pi/4 where the sine and cosine functions cross each other.

  • Why does the professor mention that the area is the same regardless of the chosen intersection points?

    -The area is the same due to the symmetry of the sine and cosine functions, which means the shape and size of the region between any two consecutive intersection points will be identical.

  • How does the professor suggest visualizing the region for integration?

    -The professor suggests visualizing the region as being cut into a lot of little rectangles and integrating the height of those rectangles to get the total area.

  • What is the height of each rectangle in the professor's visualization?

    -The height of each rectangle is the difference between the upper curve (sine x) and the lower curve (cosine x), which is sine x - cosine x.

  • What is the integral set up by the professor to find the area of the region?

    -The integral set up by the professor is the integral from pi/4 to 5*pi/4 of (sine x - cosine x) dx.

  • How does the professor simplify the integral to find the area?

    -The professor simplifies the integral by recognizing that the derivative of sine is cosine and the derivative of cosine is -sine, leading to the integral of -sine x from pi/4 to 5*pi/4.

  • What is the final result the professor obtains for the area of the region?

    -The final result obtained by the professor for the area of the region is 2 times the square root of 2.

  • What would happen if the professor had set up the integral with cosine minus sine instead of sine minus cosine?

    -If the integral had been set up with cosine minus sine, the result would be the negative of the area, indicating the importance of the order of functions in the integral for determining the sign of the area.

Outlines
00:00
📚 Introduction to Calculating Area Between Curves

The professor begins a recitation session focusing on the application of integration, specifically the calculation of areas between curves. A particular region of interest is introduced, which is bounded by the curves y=sin(x) and y=cos(x). The professor finds the region 'cute' due to the curves' interwoven nature and the fact that they cross at consecutive points, specifically at pi/4 and 5pi/4. The task is to compute the area between these two points where the curves intersect.

📐 Setting Up the Integration for the Area Calculation

The professor continues the session by explaining the setup for the integration process. The region of integration is identified as the area between the two curves from pi/4 to 5pi/4, leveraging the symmetry of the functions. The integration interval is established, and the approach involves viewing the area as a sum of infinitesimally small rectangles. The height of these rectangles is determined by the difference in the y-values of the two functions, sin(x) - cos(x), and the width is represented by dx. The area calculation is thus the integral of this height over the specified interval.

🔍 Detailed Integration Process for the Curves

The professor elaborates on the integration process, emphasizing the importance of correctly identifying the upper and lower functions for the integral. The upper function is y=sin(x) and the lower is y=cos(x), with the integral being the difference between these two functions over the interval from pi/4 to 5pi/4. The integral is set up to find the area by summing the heights of the rectangles formed by the difference in y-values. The professor then proceeds to calculate the integral, which involves finding the antiderivative of the function and evaluating it at the bounds of the interval.

📉 Evaluating the Integral and Finding the Area

The professor completes the calculation by evaluating the integral at the bounds of the integration interval. The antiderivative of sin(x) is -cos(x), and for cos(x), it is sin(x). The professor substitutes the values of the functions at the endpoints of the interval and simplifies the expression to find the area. The result is simplified to 2 times the square root of 2, which is the area enclosed by the two curves between the specified points. The professor concludes the session by ensuring the correctness of the result and inviting students to verify the calculations themselves.

Mindmap
Keywords
💡Integration
Integration is a fundamental concept in calculus that involves finding the area under a curve or the sum of a series of values. In the context of the video, integration is used to calculate the area between two curves, specifically sine and cosine functions, which is the main theme of the lesson.
💡Area between curves
The area between curves is a geometric concept where the space enclosed by two intersecting curves is measured. In the script, the professor is interested in finding the area between the curves y = sine(x) and y = cosine(x), which is a central objective of the recitation.
💡Consecutive points
Consecutive points refer to two points that are immediately next to each other, often used in mathematics to define intervals or to identify points of interest. In the video, the professor identifies consecutive points where the sine and cosine functions intersect, which are π/4 and 5π/4.
💡Sine function
The sine function is a trigonometric function that relates the ratio of the opposite side to the hypotenuse in a right-angled triangle. In the script, the sine function is one of the two curves whose area between them is being calculated.
💡Cosine function
The cosine function, like the sine function, is a trigonometric function that describes the ratio of the adjacent side to the hypotenuse in a right-angled triangle. It is the second curve in the area calculation discussed in the video.
💡Symmetry
Symmetry in mathematics refers to the property of an object or function where it can be divided into two equal halves that mirror each other. The professor mentions the symmetry of the sine and cosine functions, which implies that the area calculation will be the same regardless of the chosen intersection points due to their symmetrical nature.
💡Rectangles
In the context of the video, rectangles are used as an approximation method for calculating the area under a curve. The professor suggests viewing the region as being cut into many small rectangles, where the height of each rectangle is given by the difference in the y-values of the two curves at a given x.
💡Height of rectangles
The height of rectangles in the script refers to the vertical distance between the sine and cosine curves at a particular x-value. This height is crucial for calculating the area of each rectangle, which is then summed up through integration.
💡Integral
An integral is a mathematical operation that represents the process of integration. The professor uses the integral to sum up the areas of the small rectangles, which is the method to find the total area between the sine and cosine curves over the specified interval.
💡Derivative
The derivative is a concept in calculus that represents the rate of change of a function with respect to one of its variables. In the script, the professor mentions the derivatives of sine and cosine functions to set up the integral for calculating the area.
💡Square root of 2
The square root of 2 is a mathematical constant that appears in various geometric and algebraic contexts. In the video, the professor concludes that the area between the sine and cosine curves over the specified interval is equal to 2 times the square root of 2, which is the final result of the integration.
Highlights

Introduction to the topic of applications of integration, specifically finding areas between curves.

Presentation of a cute region between y=sin(x) and y=cos(x) where the curves cross each other repeatedly.

Interest in computing the area between the two consecutive crossing points at pi/4 and 5pi/4.

Explanation of the symmetry in the area between any two consecutive intersection points due to the symmetry of the sine and cosine functions.

Selection of the integration bounds as pi/4 and 5pi/4 for simplicity.

Visualization of the region of integration and the bounds on x.

Methodology of approximating the area by integrating the height of small rectangles formed between the curves.

Identification of the upper curve as y=sin(x) and the lower curve as y=cos(x) for calculating the height of rectangles.

Expression of the area as the integral from pi/4 to 5pi/4 of (sin(x) - cos(x)) dx.

Clarification that reversing the order of subtraction results in the negative of the area.

Integration of the function sin(x) - cos(x) between the specified bounds.

Derivation of the integral as -sin(x) evaluated from pi/4 to 5pi/4.

Application of the fundamental theorem of calculus to evaluate the definite integral.

Substitution of the bounds into the integral to find the area.

Final calculation resulting in an area equal to 2 times the square root of 2.

Conclusion of the recitation with a correct and engaging summary of the process.

Transcripts

Browse More Related Video

Area Between y=x^3 and y=3x-2 | MIT 18.01SC Single Variable Calculus, Fall 2010

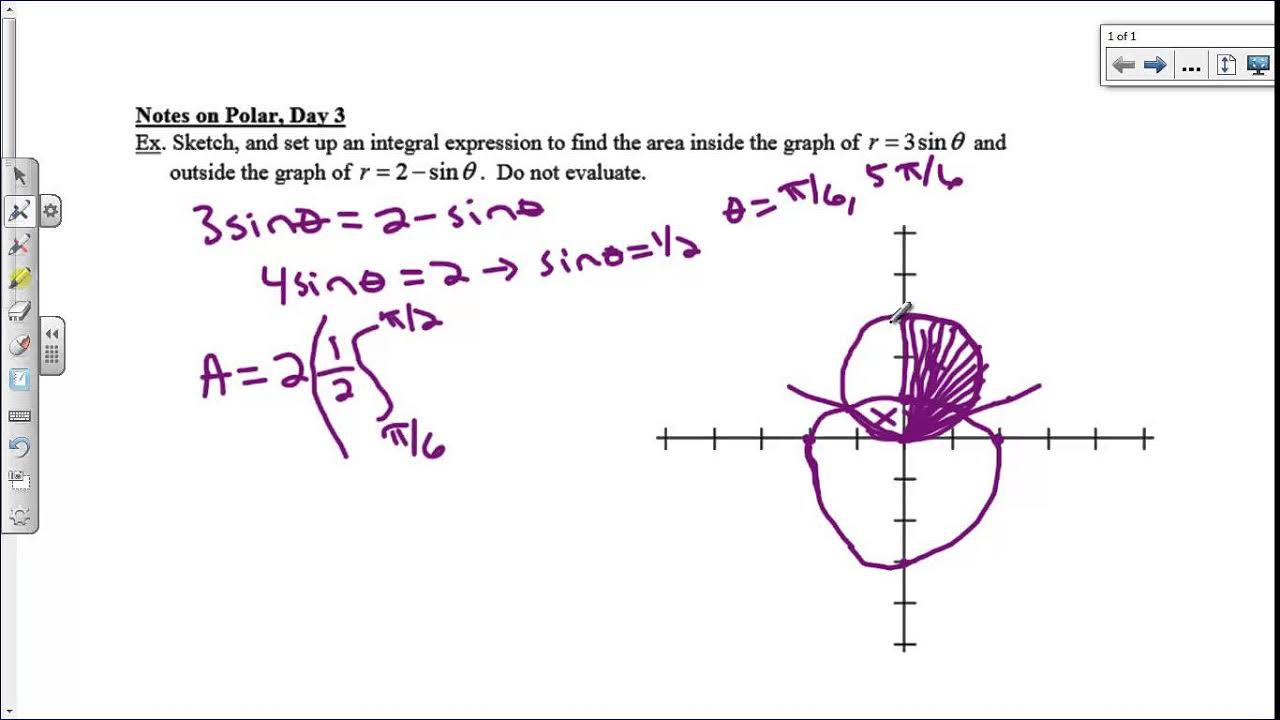
Notes on Polar, Day 3

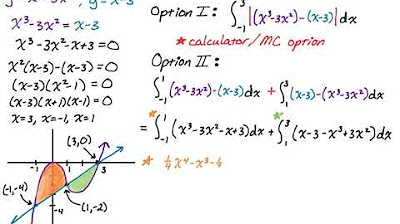
Area Between Two Curves with Multiple Regions: y = x^3-3x^2 and y = x-3

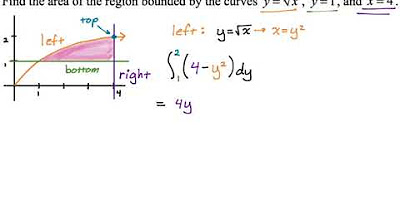
Area Between Curves: Integrating with Respect to y (Example 3)

Area Between Curves: y = cos(pi*x), y = 4x^2 -1

Area Between Curves: Integrating with Respect to y (Example 2)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
IntegrationMathematicsRecitationArea CalculationTrigonometric FunctionsCurve AnalysisEducational ContentSine FunctionCosine FunctionCalculus