Calculus Chapter 4 Lecture 33 Complex Volumes
TLDRIn this calculus lecture, Professor Greist explores the computation of complex volumes, focusing on solids of revolution—objects created by rotating 2D shapes around an axis. He demonstrates two methods for decomposing these solids into simpler volume elements: slicing orthogonally or parallel to the axis of revolution. Through examples, including a doughnut-like solid and a ball with a cylindrical hole, the professor illustrates how to calculate volumes using integral calculus, emphasizing the importance of choosing the right approach and understanding the limits of integration. The lecture concludes with an example of a vase formed by a specific curve, highlighting the versatility of calculus in solving geometric problems.
Takeaways
- 📏 Calculating volumes of complex solids can be challenging, but some can be solved using single-variable calculus by rotating 2D shapes around an axis.
- 🔄 Volumes of revolution involve rotating a shape around an axis, creating a 3D volume.
- 🍩 Two methods to compute such volumes include using disc-like elements orthogonal to the axis or cylindrical elements parallel to the axis.
- 📐 For a disc rotated about an axis to form a torus, the volume can be calculated using the formula 2π²R*a², where R is the distance from the center of the disc to the axis and a is the radius of the disc.
- 🔍 A change of coordinates can simplify complex integrals, such as those involving u-substitution.
- 🔄 The formula for the volume of a rotated shape often boils down to the area of the shape’s cross-section multiplied by the path traced by the shape’s center.
- 🔧 For a solid with a hole, like a bead, the volume can be computed using cylindrical volume elements with careful attention to integration limits.
- 🎯 Using annular volume elements, volumes can be computed by considering the difference between the areas of outer and inner discs.
- 🔢 In some cases, different methods of slicing (orthogonal vs. parallel) lead to the same integral and result, illustrating the consistency of the methods.
- 🔄 No single formula can solve all volume problems; it's crucial to think in terms of the geometry of the situation and the appropriate slicing method.
Q & A
What is the main topic of Professor Greist's lecture 33?
-The main topic of the lecture is computing volumes of solids, specifically focusing on volumes of revolution, which are solids obtained by rotating a two-dimensional shape around an axis.
Why is calculating the volume of general solids complex in multivariable calculus?
-Calculating the volume of general solids is complex because most solids have intricate shapes that do not lend themselves to simple mathematical descriptions or easy integration methods.
What are the two principal ways to decompose a volume of revolution into simple volume elements?
-The two principal ways are by taking slices orthogonal to the axis of revolution, leading to disc-like volume elements, and by slicing parallel to the axis of revolution, resulting in cylindrical volume elements.
What is the significance of the axis of revolution in the context of volumes of revolution?
-The axis of revolution is the line around which the two-dimensional shape is rotated to create the three-dimensional volume. It is crucial for determining the orientation and the type of volume elements used in the integration process.
How does the lecture describe the solid formed by rotating a disk about an axis?
-The lecture describes this solid as 'doughnut-like' and involves setting up a coordinate system with the y-axis as the axis of revolution and the disk having a radius 'a' and being located a distance 'R' away from the axis.
What is the formula for the volume element when decomposing the doughnut-like solid by slices parallel to the axis of revolution?
-The volume element is cylindrical, with the formula being 2πX * (2√(a^2 - (X - R)^2)) * dX, where X is the distance from the axis of rotation.
Why does the lecture suggest changing coordinates to 'u = X - R' when integrating?
-Changing coordinates to 'u = X - R' centers the coordinates at the origin of the disk, simplifying the integration by making the limits of integration symmetric and easier to handle.
What is the final volume of the doughnut-like solid obtained by integrating the cylindrical volume element?
-The final volume is 2π^2 * R * a^2, which is derived after performing the integration and simplifying the expression.
How does the lecture explain the volume of a ball with a cylindrical hole drilled through it?
-The lecture explains it by considering cylindrical volume elements with varying radius X and integrating the expression 4π * X * √(R^2 - X^2) * dX from X = a to X = R.
What is the significance of the limits of integration in the volume calculation of the ball with a cylindrical hole?
-The limits of integration, from 'a' to 'R', represent the range of the radius of the cylindrical volume elements, from the edge of the hole to the surface of the ball.
How does the lecture approach the volume calculation of a vase formed by rotating a specific curve about the y-axis?
-The lecture uses orthogonal slices to yield disks as volume elements, with the volume element being π * (x(y))^2 * dy, where x(y) = √(sin(y) + y) / π, integrated over y from 0 to 2π.
What is the final volume of the vase formed by rotating the given curve about the y-axis?
-The final volume is 2π^2, which is obtained after integrating the volume element and simplifying the resulting expression.
What advice does the lecture give for solving homework problems on volumes of revolution?
-The lecture advises to always consider how to decompose the region, whether by slicing parallel to or orthogonal to the axis of revolution, as this will determine the associated volume element used in the computation.
Outlines
📚 Introduction to Calculus of Revolution Volumes
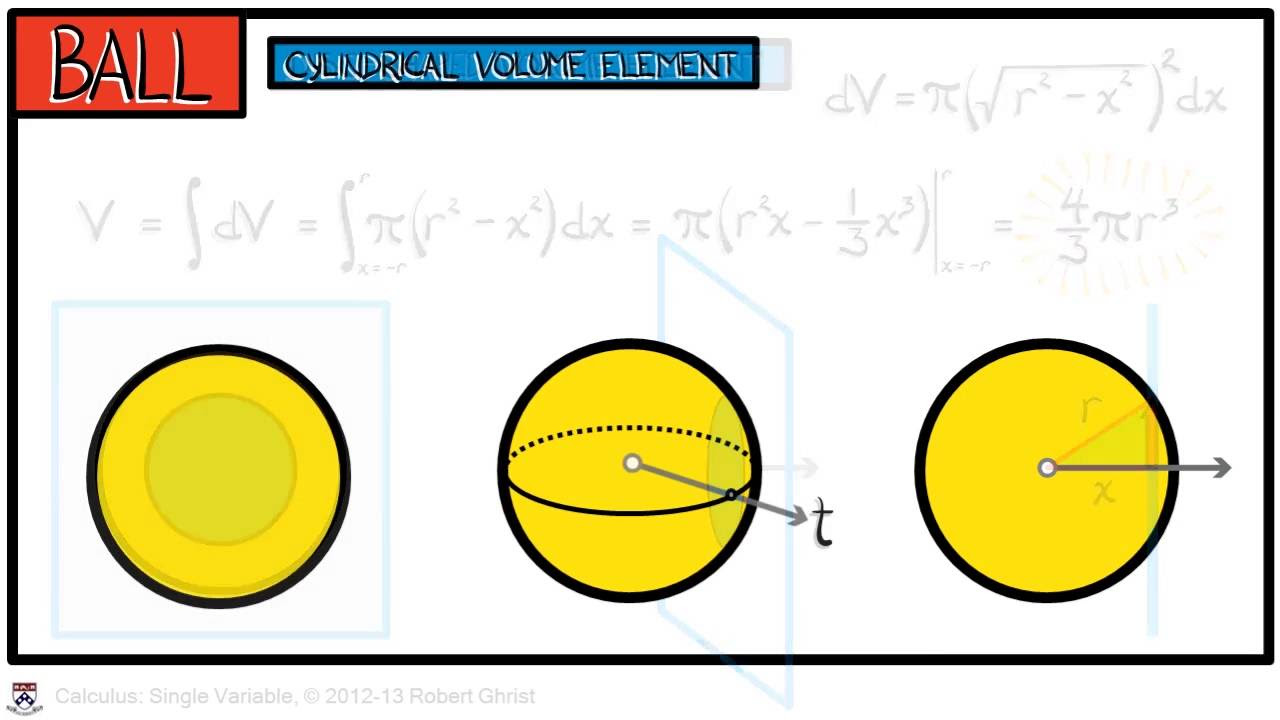
Professor Greist begins lecture 33 with an introduction to the complexities of computing complex volumes in multivariable calculus. He explains that while most solids are too complex to calculate, there is a subset, known as volumes of revolution, that can be computed using single variable calculus. These are solids formed by rotating a two-dimensional shape around an axis, creating a three-dimensional volume. The lecture focuses on two principal methods for decomposing such objects into simpler volume elements: slicing orthogonally to the axis of revolution, leading to disc-like elements, and slicing parallel to the axis, resulting in cylindrical elements. The professor sets up an example involving a doughnut-shaped solid, formed by rotating a disk of radius 'a' around an axis at a distance 'R' from the disk's center, and demonstrates how to compute its volume using cylindrical volume elements.
🔍 Detailed Analysis of Volumes of Revolution
The script continues with a deeper dive into the computation of volumes of revolution, using the example of a doughnut-shaped solid. The professor explains how to set up the volume element as a cylinder and integrate it to find the volume. He introduces a change of coordinates to simplify the integral and demonstrates how to compute the volume by integrating the area of the cross-sectional disk times the circumference traced by the center of the disk. The lecture then contrasts this method with an orthogonal slicing approach, which yields an annular volume element, and shows that both methods lead to the same result. The professor also explores the volume of a ball with a cylindrical hole, using both parallel and orthogonal slicing methods, and demonstrates the integral calculations for each, emphasizing the importance of understanding the limits of integration.
🎨 Advanced Examples and Volume Computation Techniques
In the final paragraph, the script presents more advanced examples of volume computation, including a vase formed by rotating a specific curve about the y-axis. The professor uses orthogonal slicing to derive the volume element as disks of radius 'X' and integrates this to obtain the volume. He also discusses the importance of choosing the correct volume element based on whether the slicing is parallel or orthogonal to the axis of revolution. The lecture concludes with a reminder that there is no one-size-fits-all formula for volume computation and that understanding the geometry of the problem is crucial. The professor hints at the next lesson, which will explore higher dimensions and the application of calculus to understand the geometry beyond the third dimension.
Mindmap
Keywords
💡Calculus
💡Volume of Revolution
💡Orthogonal Slices
💡Cylindrical Volume Element
💡Disk
💡Integration
💡Change of Coordinates
💡Annular Region
💡Right Triangle
💡Odd Function
💡Vase
Highlights
Introduction to Lecture 33 on complex volumes, emphasizing the challenges in computing volumes in multivariable calculus.
Explanation of the limited types of objects for which volume computation is feasible using single variable calculus, specifically solids of revolution.
Description of two principal methods for decomposing solids of revolution into volume elements: slicing orthogonally and parallel to the axis of revolution.
Example of computing the volume of a solid formed by rotating a disk, described as doughnut-like, using cylindrical volume elements.
Calculation of the volume element for a cylindrical slice, involving the height derived from a right triangle and the circumference.
Integration of the volume element to find the total volume of the doughnut-like solid, with a change of coordinates for simplification.
Observation that the integral of an odd function over a symmetric domain results in zero, simplifying the computation.
Final volume calculation resulting in 2 pi squared R a squared, highlighting a pattern in volume computation.
Alternative method for computing the volume using an orthogonal slice, leading to an annular volume element.
Determination of the radii for the annular volume element by constructing right triangles in the cross-sectional disk.
Simplification of the volume element expression and computation of the volume as an integral from negative a to a.
Reinforcement of the volume computation result using a different volume element, yielding the same final answer.
Introduction of a new example: computing the volume of a ball with a cylindrical hole, using a cylindrical volume element.
Integration of the volume element to find the volume of the ball with a hole, with careful attention to the limits of integration.
Use of a u-substitution to simplify the integral and arrive at the final volume formula for the ball with a hole.
Alternative computation using an orthogonal slice for the ball with a hole, resulting in the same volume formula.
Final example involving the volume of a vase formed by a specific curve rotated about the y-axis, with a simple integral for the volume.
Conclusion highlighting the importance of choosing the right method for decomposing a region when computing volumes of revolution.
Anticipation of the next lesson, which will explore higher dimensions and the geometry of the fourth dimension and beyond.
Transcripts
Browse More Related Video
Calculus Chapter 4 Lecture 32 Volumes

Calculus Chapter 4 Lecture 36 Surface Area

AP Calculus AB: Lesson 7.4: Disk and Washer Method

Lecture 20: Several Variables Volumes Galore

Calculus 1 Lecture 5.2: Volume of Solids By Disks and Washers Method

Unit IV: Lec 3 | MIT Calculus Revisited: Single Variable Calculus
5.0 / 5 (0 votes)
Thanks for rating: