Parametric Equations of Lines in 3D (Calculus 3)

Houston Math Prep
31 Jan 202116:12
EducationalLearning
32 Likes 10 Comments

TLDRThis video from Houston Math Prep teaches viewers how to create parametric equations for lines in 3D space. It begins with a refresher on 2D parametric equations and their relation to linear equations. The tutorial then delves into 3D parametric equations, explaining how to derive them using constant terms and linear 't' terms to represent a line's trajectory through space. Examples illustrate finding equations for lines passing through two points, adjusting the 't' parameter for different time spans, and defining line segments with specific 't' domains. The video aims to simplify the process of understanding and constructing parametric equations for 3D lines.

Takeaways
  • 📚 Parametric equations are a method to represent lines in 2D and 3D space using a separate equation for each coordinate that depends on a parameter, often denoted as 't'.
  • 📈 In 3D space, parametric equations of a line include constant terms and linear 't' terms, with the constant terms representing a point through which the line passes.
  • 🧭 The coefficients of the 't' terms in the parametric equations define a vector that indicates the direction of the line in 3D space.
  • 📍 To find parametric equations for a line through points P and Q, use the coordinates of P as the constants and the vector from P to Q for the 't' terms.
  • 🔍 The point P can be found when 't' equals zero in the parametric equations, and the line's orientation can be determined by the direction of the vector.
  • ⏱ The parameter 't' can represent time or any other scalar that scales the position along the line, and its domain can define a line segment rather than the entire line.
  • 🔄 Negative values of 't' can represent positions on the line in the opposite direction from the positive 't' values, extending the concept of the line's trajectory.
  • 🔢 Adjusting the coefficients for 't' in the parametric equations allows for changing the speed at which the line is traversed, such as taking three seconds to travel between points instead of one.
  • 📉 The process of finding parametric equations involves identifying constant terms from a given point and determining the direction vector from the difference between two points.
  • 📝 Specifying a domain for the parameter 't' is crucial for defining a line segment, where 't' is restricted to a certain interval, like between 0 and 1 or 0 and 3.
  • 🛤️ The concept of parametric equations is essential for understanding trajectories and motion in 3D space, with applications in various fields such as physics and engineering.
Q & A

  • What is the purpose of parametric equations in 3D space?

    -Parametric equations in 3D space are used to represent linear trajectories, including an additional z component, which helps define the direction and orientation of a line through space.

  • How are parametric equations different from the standard form of a linear equation in 2D space?

    -Parametric equations in 2D space use separate equations for the x and y coordinates, defined by a formula that depends on another parameter, usually represented by 't', which can represent time or any other variable.

  • What is the significance of the parameter 't' in parametric equations?

    -The parameter 't' in parametric equations is significant as it allows for the representation of the line's trajectory in a certain direction or orientation, and it can represent time or any other variable that changes along the line.

  • How can you find the parametric equations for a line that passes through two points in 3D space?

    -To find the parametric equations for a line passing through two points in 3D space, you first identify the constant terms (the coordinates of one of the points), then determine the direction vector by subtracting the coordinates of the first point from the second, and use these to form the parametric equations with 't' as the parameter.

  • What does the constant term in each parametric equation represent?

    -The constant term in each parametric equation represents a specific point in space that the line goes through, often referred to as the initial point or x₀, y₀, z₀.

  • How do the coefficients of 't' in the parametric equations relate to the direction of the line in 3D space?

    -The coefficients of 't' in the parametric equations represent a vector that points in the same direction as the line in 3D space, indicating the orientation of the line.

  • What is the difference between a line and a line segment in the context of parametric equations?

    -A line in the context of parametric equations can be represented by allowing 't' to be any real number, indicating an infinite line. A line segment, however, specifies a domain for 't', limiting its values to a particular interval, such as between 0 and 1, representing a finite piece of the line.

  • How can you adjust the parametric equations to represent a line segment with a specific duration or distance?

    -You can adjust the parametric equations to represent a line segment with a specific duration or distance by changing the domain of 't' to match the desired time interval and by adjusting the coefficients of 't' to ensure the line segment fits within that interval.

  • What does it mean when the value of 't' is set to zero in the parametric equations?

    -When the value of 't' is set to zero in the parametric equations, it represents the initial point of the line or line segment, which is the point where the line starts or the point through which the line passes when 't' equals zero.

  • How can you determine the value of 't' when you are at a specific point on the line?

    -You can determine the value of 't' for a specific point on the line by plugging the coordinates of that point into the parametric equations and solving for 't', which will give you the parameter value corresponding to that point along the line.

Outlines
00:00
📚 Introduction to Parametric Equations in 3D Space

This paragraph introduces the concept of parametric equations for lines in 3D space, drawing parallels with the familiar 2D linear equation y = mx + b. It explains how parametric equations are used to represent trajectories, with x, y, and z coordinates defined as functions of a parameter, typically denoted as 't', which represents time. The paragraph emphasizes the importance of a point and a direction vector to define a line in space, and demonstrates how to derive parametric equations for a line passing through two given points, P and Q, by identifying constants and the direction vector.

05:03
🔍 Deriving Parametric Equations for a Line Segment

Building upon the introduction, this paragraph delves into the process of finding parametric equations for a specific line segment between two points, P and Q. It illustrates how to calculate the direction vector from point P to Q and how to use this vector to establish the coefficients for 't' in the parametric equations. The paragraph also discusses the concept of orientation and the significance of 't' values in defining positions on the line at different times. It concludes with the idea of limiting the parameter 't' to a specific interval to represent a line segment rather than an entire line.

10:03
📉 Adjusting Time and Trajectory in Parametric Equations

This paragraph explores the idea of adjusting the time it takes to travel from one point to another on a line segment by modifying the coefficients of 't' in the parametric equations. It presents an example where the trajectory from point P to Q is altered to take three seconds instead of one, showing how to calculate new coefficients for 't' that reflect this change in time. The explanation includes a step-by-step process of plugging in different values for 't' to find the appropriate coefficients, ensuring that the line segment is traversed at the desired pace.

15:04
🕒 Comparing Trajectories with Different Time Intervals

The final paragraph compares two different trajectories between the same points, P and Q, but with different time intervals. It highlights how the constant terms in the parametric equations remain the same, while the coefficients for 't' are adjusted according to the time taken to travel the segment. The paragraph demonstrates that by changing the coefficients, one can control the speed at which the line segment is traversed. It concludes with a summary of the key points covered in the video, reinforcing the understanding of parametric equations for lines in 3D space.

Mindmap
Keywords
💡Parametric Equations
Parametric equations are a set of equations that express the coordinates of a point on a curve or a line in terms of a single variable, known as the parameter. In the context of the video, these equations are used to describe the position of points along a line in 3D space as a function of the parameter 't', which often represents time. The script explains that parametric equations allow for the representation of a line's trajectory and orientation.
💡3D Space
3D space refers to a three-dimensional coordinate system where any point can be defined by three coordinates, typically x, y, and z. The video script discusses how to represent lines in this three-dimensional space using parametric equations, adding a z-component to the familiar 2D concepts.
💡Linear Trajectories
Linear trajectories describe the path that a point moves along a straight line. The script uses this concept to explain how parametric equations can represent the movement of a point along a line in 3D space over time, with the parameter 't' indicating the position of the point at different times.
💡Slope Intercept Form
The slope intercept form of a linear equation is represented as y = mx + b, where 'm' is the slope of the line and 'b' is the y-intercept. The video script mentions this form as a common way to write the equation for a line in 2D space, which can be rearranged to solve for y, illustrating the concept of representing lines in different forms.
💡Vector
A vector is a mathematical object that has both magnitude and direction. In the script, the vector is used to represent the direction of a line in 3D space. The coefficients of the 't' terms in the parametric equations are derived from the vector that points in the direction of the line, such as the vector from point p to point q.
💡Line Segment
A line segment is a part of a line that is bounded by two distinct end points. The video script explains how to find parametric equations for a line segment by specifying a domain for the parameter 't', limiting the values of 't' to a certain interval, such as from 0 to 1, to represent a piece of the line between two points.
💡Trajectory
Trajectory refers to the path that an object follows through space as a function of time. The script discusses representing trajectories as lines in 3D space using parametric equations, emphasizing the aspect of time and the direction of movement along the line.
💡Orientation
Orientation in the context of the video refers to the direction in which a line or trajectory is moving. The script explains how the parametric equations can indicate the orientation of a line, such as moving forward in time, by the increasing values of the parameter 't'.
💡Domain
The domain of a function refers to the set of all possible input values (in this case, the parameter 't') for which the function is defined. The script mentions specifying the domain for 't' to indicate the range of values that 't' can take, which is essential for defining a line segment rather than an entire line.
💡Coefficients
Coefficients are numerical factors that multiply the variables in an algebraic expression. In the script, the coefficients for the 't' terms in the parametric equations determine the direction and rate of change along the line in 3D space. The coefficients are derived from the vector that represents the direction of the line.
Highlights

Introduction to parametric equations for lines in 3D space, emphasizing their utility in representing trajectories.

Explanation of how parametric equations differ from the standard form of a linear equation in 2D space.

The role of the parameter 't' in parametric equations, often assumed to represent time.

Illustration of how constant terms in parametric equations represent a point through which the line passes.

Importance of direction in defining a line in space, conveyed through the linear 't' terms.

Demonstration of deriving parametric equations for a line through points P and Q in 3D space.

Method of finding the direction vector by subtracting coordinates of points P and Q.

Construction of parametric equations using constants and the direction vector's coefficients.

Identification of the point where t equals zero, revealing the starting point of the trajectory.

Calculation of the time value at point Q to understand the orientation of the trajectory.

Concept of adjusting the parameter 't' to represent different segments of a line or the entire line.

Introduction of the domain for the parameter 't' to define a line segment between two points.

Example of finding parametric equations for a line segment with a specified domain for 't'.

Explanation of how negative values of 't' can represent movement away from point P.

Application of the method to find parametric equations for different line segments with various points.

Adjustment of the parameter 't' to represent a trajectory taking a different amount of time.

Strategy for adjusting the coefficients of 't' to change the speed of the trajectory.

Final summary of the process for deriving parametric equations for lines in 3D space.

Transcripts

Browse More Related Video

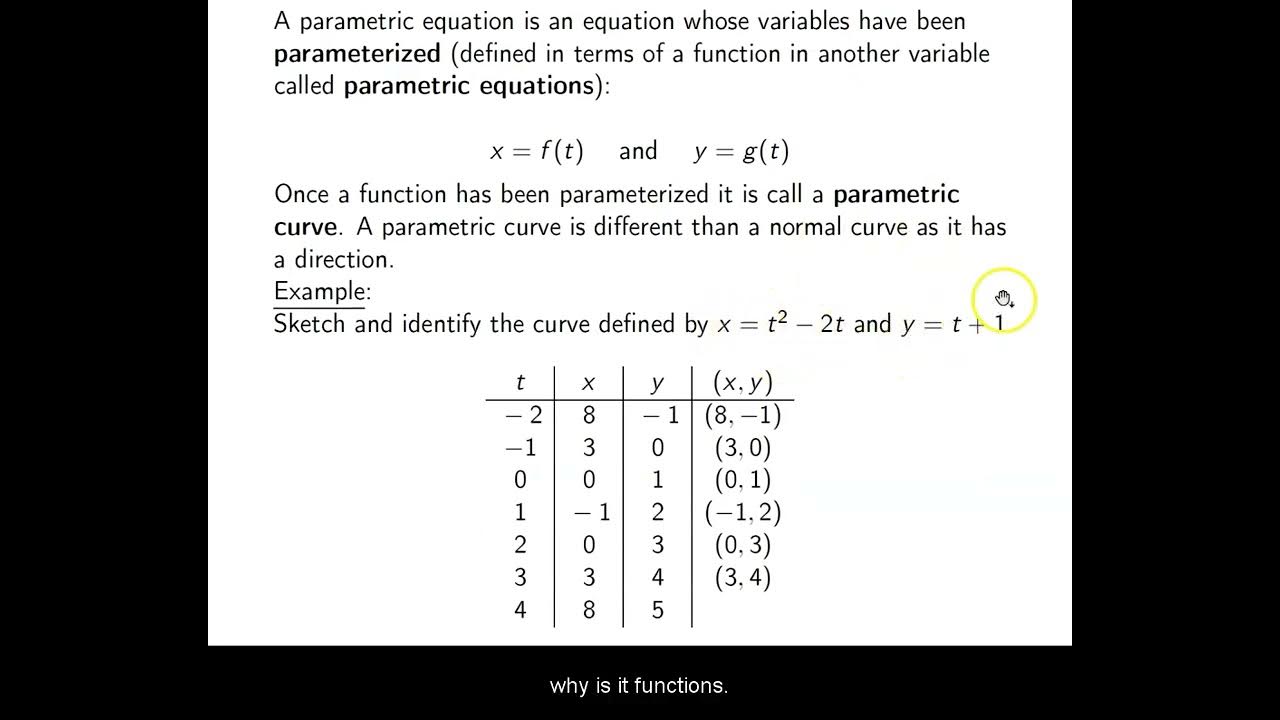
Ch. 8.4 Plane Curves and Parametric Equations

AP Calculus BC Lesson 9.1

Calculus 3: Equations of Lines and Planes (Video #5) | Math with Professor V

Parametric equations 3 | Parametric equations and polar coordinates | Precalculus | Khan Academy

Parametric equations 2 | Parametric equations and polar coordinates | Precalculus | Khan Academy

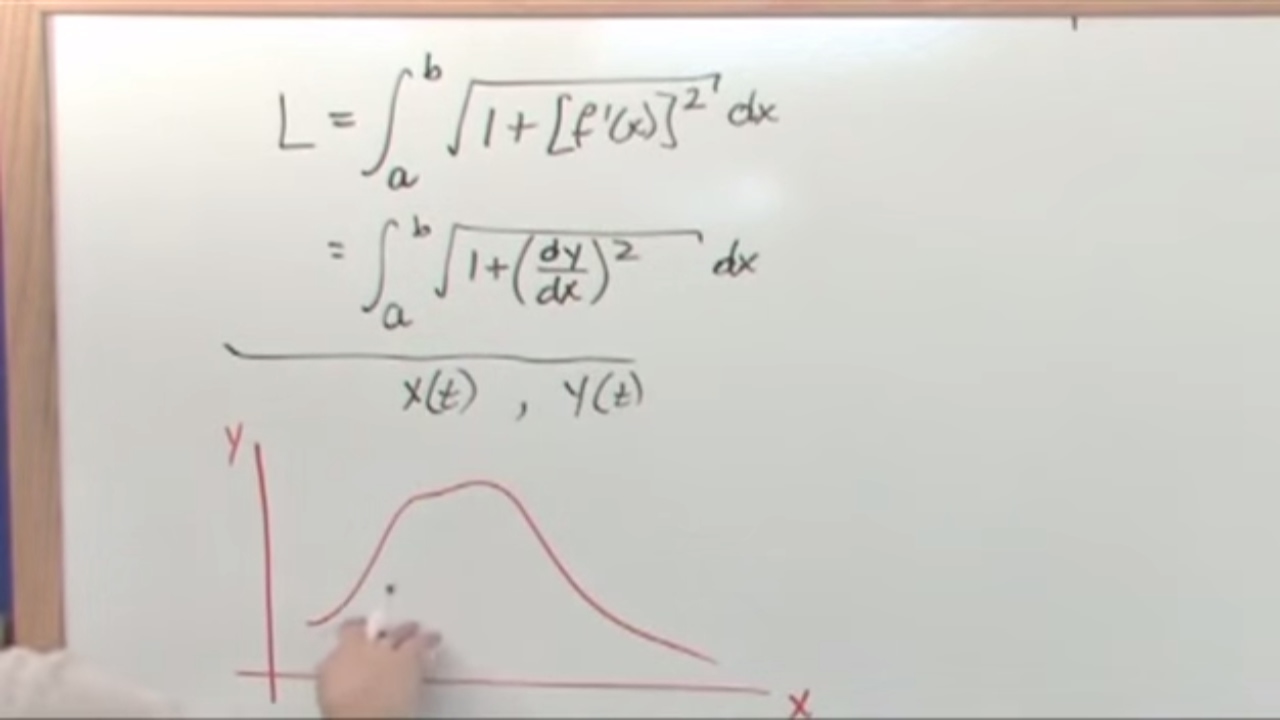
Lesson 11 - Arc Length In Parametric Equations (Calculus 2 Tutor)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Parametric Equations3D SpaceLinear TrajectoryMathematics TutorialVector AnalysisSlope InterceptTrajectory OrientationDirection VectorLine SegmentTime Parameter