Mean, median, mode of Ungrouped Data

Oninab (Educational) Resources
22 Jul 201916:14
EducationalLearning
32 Likes 10 Comments

TLDRThis tutorial video covers the calculation of mean, median, mode, and range for ungrouped data. It demonstrates the process with three problems, including a set of numbers, student test scores, and athletes' heights. The video guides viewers through each step, from arranging data to finding the central values and range, and concludes with creating a frequency table for the athletes' heights to determine the mean, median, and mode. The tutorial is an informative resource for understanding basic statistical measures.

Takeaways
  • 📚 The tutorial covers the calculation of mean, median, mode, and range for ungrouped data.
  • 🔢 To find the mean, sum all numbers in the dataset and divide by the count of numbers.
  • 📈 For the median, arrange numbers in ascending order and identify the middle number; if even count, average the two middle numbers.
  • 📊 The mode is the number that appears most frequently in the dataset.
  • 📉 The range of a dataset is the difference between the highest and lowest values.
  • 👉 The first problem involves calculating statistics for the numbers 35, 3, 72, 5, and 3, with the mean being 4, median 3, mode 3, and range 5.
  • 🏫 The second problem deals with student test scores, calculating the mean as 5.8, range as 6, median as 5.5, and mode as 5.
  • 🤸‍♂️ The third problem requires creating a frequency table for the heights of 15 athletes, with heights ranging from 126 cm to 131 cm.
  • 📐 The mean height is calculated using the sum of the product of each height and its frequency, divided by the total frequency count, resulting in a mean of 128.3 cm.
  • 🔑 To find the median height, the frequency table is used to identify the middle athlete's height, which is 129 cm for 15 athletes.
  • 🏅 The mode, the most common height, is also 129 cm, as it appears with the highest frequency of 4 times.
  • 📋 The tutorial concludes with a reminder to subscribe to the YouTube channel for more educational content.
Q & A

  • What is the main topic of the tutorial?

    -The main topic of the tutorial is statistics for ungrouped data, focusing on calculating mean, median, mode, and range.

  • What is the formula to calculate the mean of a set of numbers?

    -The mean is calculated by adding all the numbers in the set and then dividing by the count of the numbers.

  • How is the median of a set of numbers determined?

    -The median is determined by arranging the numbers in ascending order and finding the middle number. If there is an even count of numbers, the median is the average of the two middle numbers.

  • What is the mode in the context of a set of numbers?

    -The mode is the number that appears most frequently in the set.

  • How do you calculate the range of a set of numbers?

    -The range is calculated by subtracting the smallest number in the set from the largest number.

  • What is the mean of the first set of numbers provided in the tutorial (35, 3, 72, 5, and 3)?

    -The mean of the first set of numbers is 4.

  • What is the median of the first set of numbers in the tutorial?

    -The median of the first set of numbers is 3.

  • What is the mode of the first set of numbers in the tutorial?

    -The mode of the first set of numbers is 3, as it appears the most frequently.

  • What is the range of the first set of numbers in the tutorial?

    -The range of the first set of numbers is 5 (7 - 2).

  • How many students' scores are considered in the second problem of the tutorial?

    -There are 10 students' scores considered in the second problem.

  • What is the mean score of the students in the second problem of the tutorial?

    -The mean score of the students is 5.8.

  • What is the median score of the students in the second problem of the tutorial?

    -The median score is 5.5, which is the average of the 5th and 6th scores when arranged in ascending order.

  • What is the modal score of the students in the second problem of the tutorial?

    -The modal score is 5, as it appears the highest number of times.

  • What is the range of the students' scores in the second problem of the tutorial?

    -The range of the students' scores is 6 (9 - 3).

  • What is the mean height of the athletes in the third problem of the tutorial?

    -The mean height of the athletes is 128.3 cm.

  • What is the median height of the athletes in the third problem of the tutorial?

    -The median height is 129 cm, which corresponds to the height of the 8th athlete when arranged in ascending order.

  • What is the mode height of the athletes in the third problem of the tutorial?

    -The mode height is 129 cm, as it has the highest frequency of occurrence among the athletes' heights.

Outlines
00:00
📊 Statistics Tutorial: Mean, Median, Mode, and Range of Ungrouped Data

This paragraph introduces a statistics tutorial focusing on ungrouped data analysis. The speaker explains how to calculate the mean, median, mode, and range using a set of numbers as an example. The mean is found by summing all numbers and dividing by the count, resulting in 4 for the given set. The median is determined by arranging the numbers in ascending order and identifying the middle value, which is 3. The mode, the number that appears most frequently, is also 3. Lastly, the range is calculated by subtracting the smallest number from the largest, yielding 5. The tutorial continues with additional problems involving student test scores and a frequency table for athlete heights.

05:05
📐 Calculating Mean and Range of Student Test Scores

The second paragraph delves into calculating the mean and range of student test scores. The mean is calculated as 5.8 by summing all scores and dividing by the total number of students, which is 10. The range is found by subtracting the lowest score from the highest, resulting in 6. The speaker then explains how to find the median score by arranging the scores in ascending order. Since there are an even number of scores, the median is the average of the two middle scores, calculated to be 5.5. The mode, the most frequently occurring score, is identified as 5, which appears three times, more than any other score.

10:06
🏆 Creating a Frequency Table and Calculating Mean, Median, and Mode for Athlete Heights

In the third paragraph, the speaker discusses creating a frequency table for the heights of 15 athletes, measured in centimeters. The table lists each unique height and tallies the number of times it appears. The mean height is calculated by summing the product of each height and its frequency, then dividing by the total number of athletes, resulting in 128.3 cm. To find the median, the speaker notes that since there is an odd number of athletes, the middle value is the median, which corresponds to a height of 129 cm. The mode, the most common height, is also 129 cm, as it has the highest frequency of 4.

15:10
📚 Conclusion of the Statistics Tutorial with a Call to Action

The final paragraph concludes the tutorial by summarizing the calculations of mean, median, and mode for the athlete heights and reiterating the importance of creating a frequency table for ungrouped data. The speaker encourages viewers to subscribe to the YouTube channel for more educational content and thanks them for watching the video.

Mindmap
Keywords
💡Statistics
Statistics is the discipline that concerns the collection, analysis, interpretation, presentation, and organization of data. In the video, statistics is the overarching theme, with a focus on calculating various measures for ungrouped data, demonstrating its fundamental role in understanding and summarizing datasets.
💡Ungrouped Data
Ungrouped data refers to a set of numbers or observations that have not been organized into categories or groups. The video script provides examples of calculating statistical measures for such data, emphasizing the need for initial data arrangement before analysis.
💡Mean
The mean, often referred to as the average, is calculated by summing all the values in a dataset and then dividing by the number of values. The script illustrates how to calculate the mean for different sets of ungrouped data, showing its importance in finding the central tendency.
💡Median
The median is the middle value of a dataset when the numbers are arranged in ascending or descending order. The video explains the process of finding the median, highlighting its significance as a measure of central tendency, especially when data may not be symmetrically distributed.
💡Mode
The mode is the value that appears most frequently in a dataset. The script demonstrates the identification of the mode in different datasets, showing how it can be used to identify the most common or typical value within a set of ungrouped data.
💡Range
The range is the difference between the highest and lowest values in a dataset, providing a measure of dispersion or spread. The video script uses the range to illustrate the variability of the data points in the given examples.
💡Frequency Table
A frequency table is a tool used to organize data into a table that displays the frequency of each unique value. In the script, creating a frequency table for the heights of athletes is a step towards calculating the mean, median, and mode, showing its utility in handling ungrouped data.
💡Central Tendency
Central tendency refers to the typical or central value of a dataset, which can be measured by the mean, median, and mode. The video script discusses these measures as ways to represent the center of the data, which is crucial for summarizing and understanding data distributions.
💡Dispersion
Dispersion refers to the spread of data points around the central tendency. The range, as discussed in the script, is a measure of dispersion, indicating how much the data varies from the smallest to the largest value.
💡Data Analysis
Data analysis involves examining and interpreting data to draw conclusions. The video script is a tutorial on data analysis, specifically focusing on statistical measures for ungrouped data, to demonstrate the process of analyzing and understanding datasets.
💡Tutorial
A tutorial is a set of instructions or an educational program that provides information or teaches a skill. The script is a transcript of a tutorial video, aiming to educate viewers on how to calculate statistical measures for ungrouped data, making it an instructional resource.
Highlights

Introduction to the tutorial on statistics for ungrouped data.

Explanation of how to calculate the mean of a set of numbers.

Demonstration of calculating the median by arranging numbers in ascending order.

Identification of the mode as the most frequently occurring number in a set.

Calculation of the range by subtracting the lowest number from the highest.

Problem-solving approach applied to a set of student scores.

Method to find the mean of student scores by summing and dividing by the count.

Determination of the range of scores by identifying the highest and lowest values.

Arranging scores in ascending order to find the median score.

Calculation of the median score as the average of the two middle scores for an even set.

Identification of the modal score as the score that appears most frequently.

Introduction to the third problem involving the height of 15 athletes.

Explanation of creating a frequency table for ungrouped data.

Process of calculating the mean height using the summation of frequency times height.

Determination of the median height by locating the middle athlete in an ordered list.

Identification of the mode as the height with the highest frequency.

Conclusion of the tutorial with a summary of the mean, median, and mode calculations.

Call to action to subscribe to the YouTube channel for more educational content.

Transcripts

Browse More Related Video

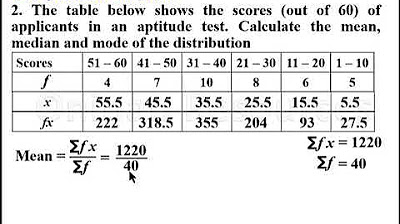
Mean, Median and Mode of Grouped Data(Lesson 2)

Measures of Central Tendency (Grouped Data) | Basic Statistics

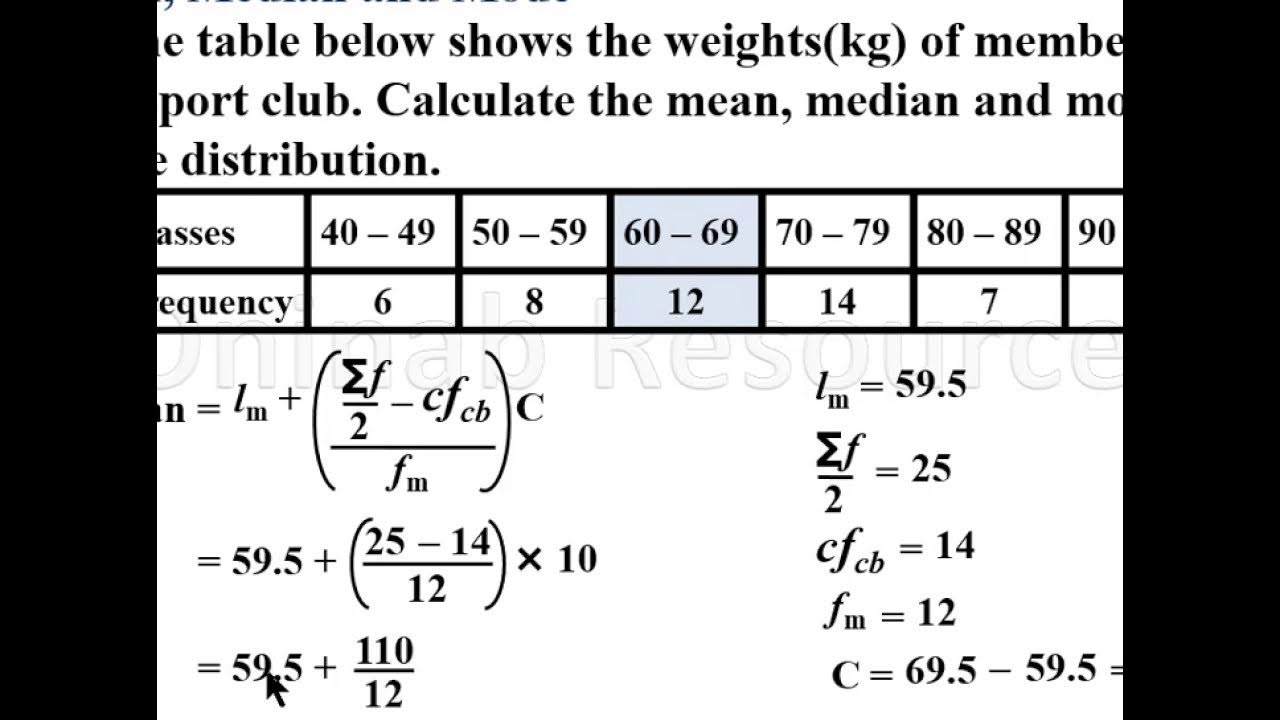
Mean, median and mode of grouped Data(Lesson 1)

Mode, Median, Mean, Range, and Standard Deviation (1.3)

Mean, Median and Mode - Measures of Central Tendency

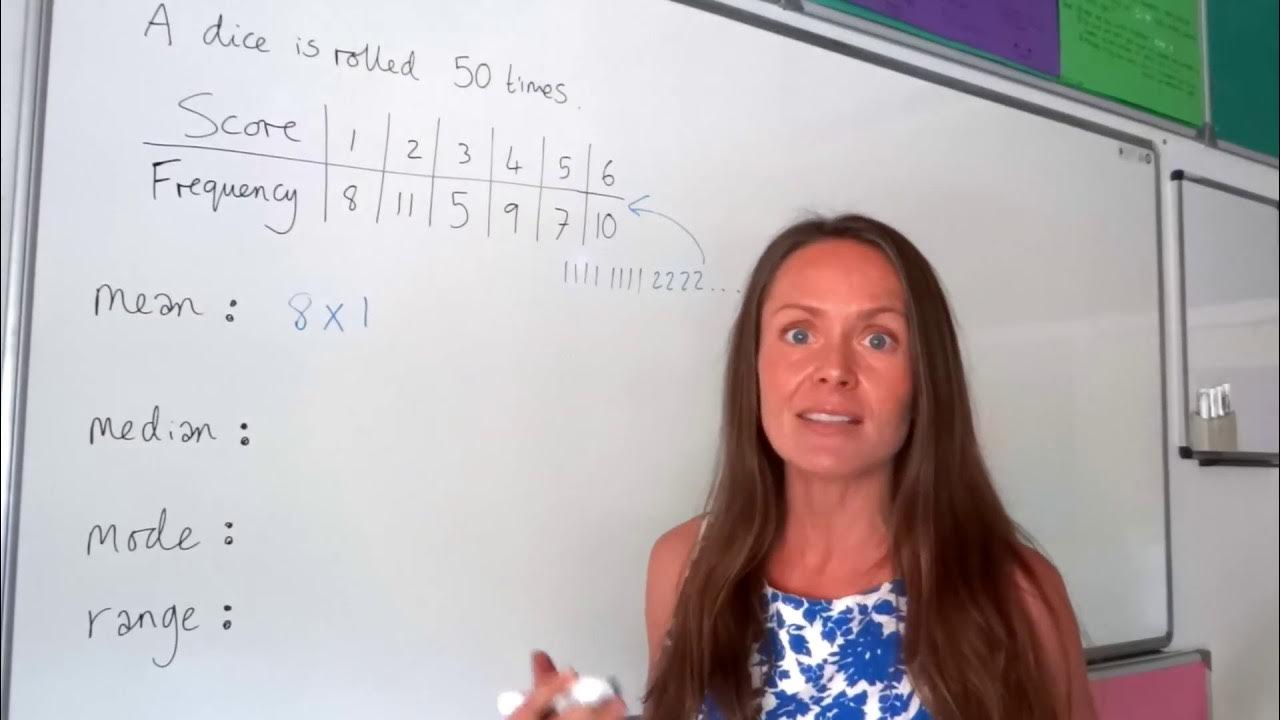
The Maths Prof: Frequency Tables (Mean, Median, Mode & Range)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Statistics TutorialMean CalculationMedian FinderMode AnalysisRange MeasureData AnalysisEducational ContentMathematics GuideTutorial SeriesUngrouped DataStatistical Methods