Mean, median and mode of grouped Data(Lesson 1)

Oninab (Educational) Resources
22 Jul 201912:35
EducationalLearning
32 Likes 10 Comments

TLDRIn this instructional video, the presenter guides viewers through the process of calculating the mean, median, and mode for grouped data, using a table of weights from a sports club as an example. The video explains how to reorganize data into a vertical form, calculate the mid-value of class intervals, and determine the FX column. It then demonstrates the formulas for mean, median, and mode, including how to identify the median class and calculate the lower class boundary. The presenter concludes with the final values for mean, median, and mode, providing a clear and concise tutorial on statistical analysis of grouped data.

Takeaways
  • ๐Ÿ“Š The video script is a tutorial on calculating the mean, median, and mode for grouped data.
  • ๐Ÿ“ˆ The example used is the weight distribution of members in a sports club, presented in a table.
  • ๐Ÿ”„ The table is reorganized from a horizontal to a vertical form to facilitate the calculations.
  • ๐Ÿ“ The formula for calculating the mean involves summing the product of frequency (f) and mid-value (x) of each class interval, then dividing by the total frequency.
  • ๐Ÿงฎ The mean weight is calculated to be 67.9 kg using the formula and the reorganized data.
  • ๐Ÿ”ข To find the median, the formula involves identifying the median class, which is the class containing the middle values in an ordered dataset.
  • ๐Ÿ‹๏ธโ€โ™‚๏ธ The median class is determined to be 60-69 kg, and the lower boundary of this class is calculated to be 59.5 kg.
  • ๐Ÿ“Š The median is calculated using the lower boundary of the median class, cumulative frequencies, and the frequency of the median class, resulting in a median weight of 68.67 kg.
  • ๐Ÿ”‘ The mode is found by identifying the class with the highest frequency, which is considered the modal class.
  • ๐Ÿ“‰ The mode is calculated using the lower boundary of the modal class, differences in frequencies of adjacent classes, and the class size, yielding a mode of 71.72 kg.
  • ๐Ÿ“š The video concludes with instructions to subscribe to the channel for more educational content.
Q & A

  • What statistical measures are discussed in the video?

    -The video discusses how to calculate the mean, median, and mode of grouped data.

  • What is the first step in calculating the mean for grouped data?

    -The first step is to reorganize the data from a horizontal form to a vertical form and create a column for the mid-value (x) of each class interval.

  • How is the mid-value (x) of a class interval calculated?

    -The mid-value (x) is calculated by finding the average of the upper and lower limits of each class interval.

  • What does the term 'FX' represent in the context of calculating the mean?

    -FX represents the product of frequency (f) and the mid-value (x) of the class interval.

  • How is the mean of the distribution calculated from grouped data?

    -The mean is calculated by summing up all the FX values and then dividing by the total number of observations.

  • What formula is used to find the median in grouped data?

    -The median is found using the formula Lm + ((ฮฃf/2) - Cf) / f, where Lm is the lower class boundary of the median class, ฮฃf is the sum of frequencies, and Cf is the cumulative frequency of the class before the median class.

  • How do you determine the median class in a set of grouped data?

    -The median class is determined by identifying the class that contains the middle value(s) of the dataset, which in this case is the class containing the 25th and 26th members.

  • What is the purpose of calculating the cumulative frequency?

    -The cumulative frequency is used to determine the total number of observations up to a certain class, which is necessary for finding the median and mode.

  • How is the mode of a distribution calculated from grouped data?

    -The mode is calculated using the formula Lm + (ฮ”1/(ฮ”1 + ฮ”2)) * C, where Lm is the lower class boundary of the modal class, ฮ”1 and ฮ”2 are the differences in frequencies between the modal class and adjacent classes, and C is the class width.

  • What is the significance of the class width in calculating the mode?

    -The class width (C) is used to adjust the lower class boundary of the modal class to find the exact mode value.

  • How does the video conclude?

    -The video concludes with the final calculated values for the mean, median, and mode of the given data set and a reminder to subscribe to the channel.

Outlines
00:00
๐Ÿ“Š Calculating Mean, Median, and Mode for Grouped Data

This paragraph introduces the process of calculating the mean, median, and mode for grouped data using an example of a sports club's members' weights. The speaker explains the need to reorganize the data from a horizontal to a vertical form, calculate the mid-value (X) for each class interval, and then determine the frequency times the mid-value (FX). The mean is calculated by summing FX and dividing by the total frequency. The median is found by identifying the median class, calculating the lower class boundary, and applying the median formula. Detailed steps include finding the cumulative frequency, lower class boundary, and frequency of the median class to derive the median value.

05:02
๐Ÿ”ข Detailed Steps for Median Calculation in Grouped Data

The second paragraph delves deeper into calculating the median for grouped data. It explains how to determine the median class by locating the middle members of the dataset and how to find the lower class boundary of the median class. The speaker provides the formula for the median and guides through the process of calculating the cumulative frequency, the frequency of the median class, and the class size. The formula is then applied with these values to find the median, which represents the middle value of the dataset.

10:03
๐Ÿ“ˆ Determining the Mode of Grouped Data

The final paragraph focuses on calculating the mode of the grouped data. It begins by identifying the modal class as the one with the highest frequency. The speaker outlines the steps to find the lower class boundary of the modal class, calculate the differences between the frequencies of adjacent classes (Delta 1 and Delta 2), and determine the class size. These values are then used in the mode formula to calculate the mode, which is the value that appears most frequently in the dataset. The paragraph concludes with the final mode calculation and a reminder to subscribe to the channel.

Mindmap
Keywords
๐Ÿ’กStatistics
Statistics refers to the branch of mathematics that deals with the collection, analysis, interpretation, presentation, and organization of data. In the context of the video, statistics is the subject matter being taught, specifically focusing on the calculation of mean, median, and mode for grouped data. The script demonstrates how to apply statistical methods to analyze the weight distribution of sports club members.
๐Ÿ’กUngrouped Data
Ungrouped data is a set of data points that have not been organized into categories or groups. It is the raw form of data collected without any specific arrangement. The video mentions ungrouped data in contrast to grouped data, which is the main focus of the lesson, where data is organized into specific ranges or intervals for easier analysis.
๐Ÿ’กGrouped Data
Grouped data is a form of data organization where individual data points are grouped into specific intervals or categories. This method is used to simplify the analysis of large data sets. The video script provides a step-by-step guide on how to calculate the mean, median, and mode for grouped data, using the weight of sports club members as an example.
๐Ÿ’กMean
The mean, often referred to as the average, is a measure of central tendency in statistics. It is calculated by summing all the values in a data set and then dividing by the number of values. In the video, the mean is calculated for the weight distribution of the sports club members, using the formula 'summation of FX over summation of f', where FX represents the product of frequency and the mid-value of the class intervals.
๐Ÿ’กMedian
The median is another measure of central tendency, which is the middle value of a data set when it is ordered from least to greatest. If there is an even number of observations, the median is the average of the two middle numbers. The script explains how to calculate the median for grouped data by identifying the median class and using the formula involving the lower class boundary, cumulative frequency, and class size.
๐Ÿ’กMode
The mode is the value that appears most frequently in a data set and is used to identify the most common occurrence. It is particularly useful when dealing with non-numeric data or when trying to identify popular items. In the video, the mode is calculated for the grouped data by identifying the class with the highest frequency and applying a specific formula that takes into account the lower class boundary, differences in frequencies, and class size.
๐Ÿ’กClass Interval
A class interval, also known as a bin, is a range of values into which data is divided in grouped data. It helps in summarizing and simplifying the data for analysis. The script uses class intervals such as '40 to 49', '50 to 59', etc., to organize the weight data of the sports club members and to calculate the mean, median, and mode.
๐Ÿ’กFrequency
Frequency refers to the number of times each value or group of values occurs in a data set. It is used to determine how often data points fall into each class interval. In the video, frequencies are given as '6', '4', '12', '14', '7', and '3', corresponding to the number of sports club members within each weight class interval.
๐Ÿ’กMid-value
The mid-value, or class midpoint, is the average of the upper and lower boundaries of a class interval. It represents the central value of the interval and is used in the calculation of the mean for grouped data. The script demonstrates the calculation of mid-values for each class interval, such as (40+49)/2 = 44.5, to find the central point for each weight range.
๐Ÿ’กCumulative Frequency
Cumulative frequency is the total number of observations below a particular value in an ordered data set. It is used to identify the point at which a certain number of data points fall within a range. In the video, cumulative frequency is calculated by adding the frequencies of all classes before the median class to determine the position of the median.
Highlights

Introduction to calculating mean, median, and mode of grouped data.

Reorganizing a horizontal table into a vertical form for easier calculations.

Explanation of the formula for calculating the mean using summation of FX over summation of f.

Creation of a new column for x, representing the mid-value of class intervals.

Calculation of the mid-value by averaging the upper and lower limits of each class interval.

Determination of FX as the product of frequency and the mid-value of the class interval.

Summation of frequencies to ensure the total equals the number of members in the dataset.

Calculation of the mean using the total FX sum divided by the total number of frequencies.

Introduction to the formula for calculating the median in grouped data.

Identification of the median class based on the total number of members.

Explanation of how to find the lower class boundary of the median class.

Calculation of cumulative frequency to determine the position of the median.

Determination of the median using the class width and cumulative frequencies.

Introduction to the formula for calculating the mode in grouped data.

Identification of the modal class as the class with the highest frequency.

Explanation of how to calculate the lower class boundary of the modal class.

Calculation of the differences between frequencies to find Delta 1 and Delta 2.

Determination of the class size or width for the modal class.

Final calculation of the mode using the provided formula and class parameters.

Conclusion summarizing the mean, median, and mode of the grouped data distribution.

Transcripts

Browse More Related Video

Statistics of Grouped Data: Mean, Median and Mode

Measures of Central Tendency (Grouped Data) | Basic Statistics

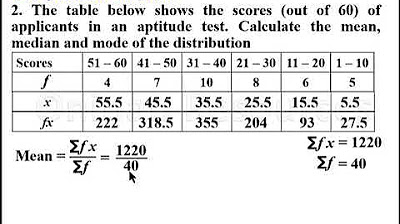
Mean, Median and Mode of Grouped Data(Lesson 2)

Measure of Central Tendency of Grouped Data for beginners

Statistics - Mean, Median & Mode for a grouped frequency data

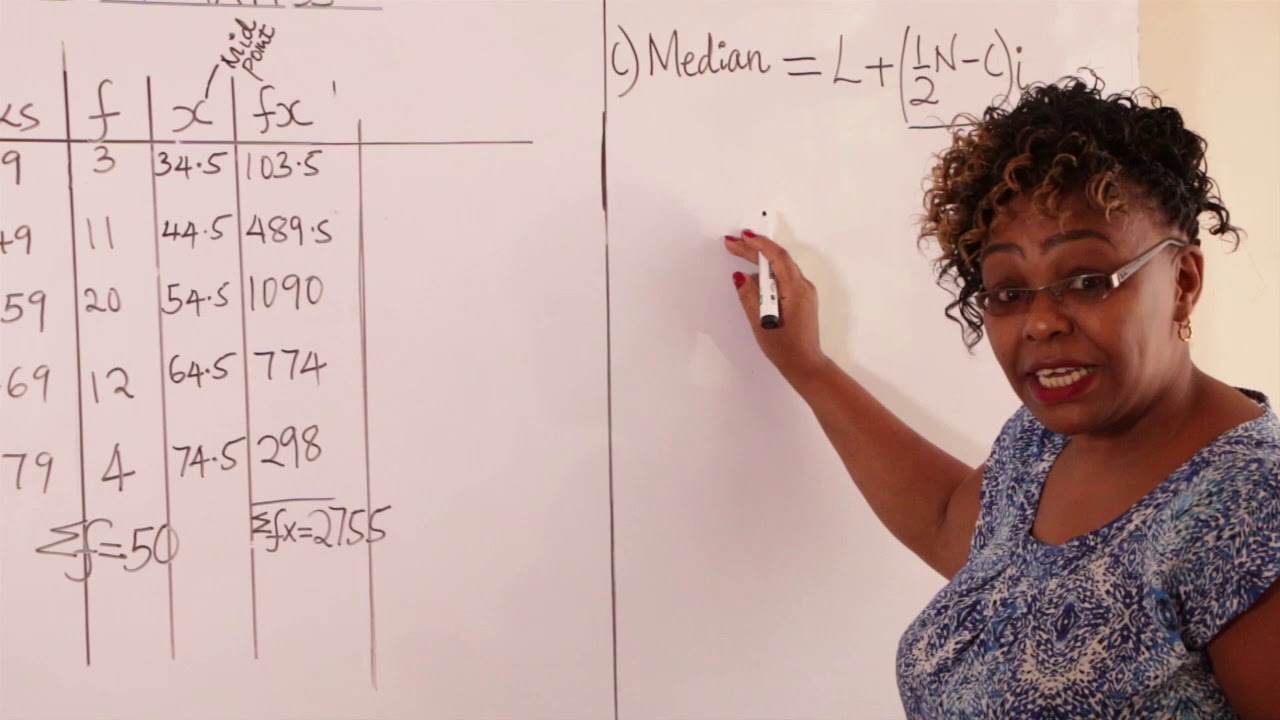
FORM 2 MATHS STATISTICS I MEAN,MODE & MEDIAN

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
StatisticsMean CalculationMedian FormulaMode MethodGrouped DataData AnalysisTutorialWeight DistributionMathematicsEducational Content