Calculus AB Homework 4.3: L'Hospital's Rule
TLDRThis video tutorial guides viewers through solving 12 calculus homework problems using L'Hôpital's Rule for limits involving indeterminate forms. It covers various scenarios, including limits as X approaches infinity, and demonstrates how to apply the rule iteratively. The script also explains how to identify dominating functions and their implications for limit calculations, providing a comprehensive understanding of the topic.
Takeaways
- 📚 The video script covers a series of calculus problems involving L'Hôpital's Rule for evaluating limits, particularly as X approaches infinity.
- 🔍 The script explains the concept of indeterminate forms, such as 0/0 or ∞/∞, which are common scenarios where L'Hôpital's Rule can be applied.
- 📈 It demonstrates how to apply L'Hôpital's Rule by taking derivatives of the numerator and denominator until a determinate limit can be found.
- 📘 The video script also discusses graphical interpretations of functions, helping to visualize the behavior of functions as X approaches infinity.
- 🤔 It highlights the importance of recognizing when to apply L'Hôpital's Rule, especially when dealing with limits that result in indeterminate forms.
- 📝 The script provides a step-by-step approach to solving each problem, including substitution and the iterative application of L'Hôpital's Rule.
- 📉 The concept of vertical and horizontal asymptotes is discussed, along with how to find them and their significance in the graph of a function.
- 📊 The video script includes examples of functions with removable discontinuities and how to identify them, such as factors that cancel out in the numerator and denominator.
- 🧩 It explains the use of algebraic manipulation to transform indeterminate forms into a format where L'Hôpital's Rule can be applied.
- 📚 The script touches on the concept of function domination, where one function grows faster than another as X approaches infinity.
- 🔑 The final takeaway is a summary of the growth rates of various functions, such as exponential functions, polynomials, and logarithmic functions, and how they relate to each other in terms of limit evaluation.
Q & A
What is the main topic of the video?
-The main topic of the video is the application of L'Hôpital's Rule to solve limits, specifically focusing on problems 14 through 25.
What is L'Hôpital's Rule used for?
-L'Hôpital's Rule is used for finding limits of the form 0/0 or ∞/∞ by taking the derivatives of the numerator and the denominator until a determinate limit is found.
In the context of problem 14, why is the limit of X cubed over e to the 3x considered indeterminate when X approaches infinity?
-The limit is indeterminate because both the numerator (X cubed) and the denominator (e to the 3x) approach infinity, resulting in an 'infinity over infinity' form.
What is the graphical interpretation of X approaching infinity for the function X cubed?
-Graphically, as X approaches infinity, the function X cubed grows without bound, and the Y values of its graph also approach infinity.
How does the video demonstrate the limit of the natural log of X over X squared as X approaches infinity?
-The video uses L'Hôpital's Rule by taking the derivative of the numerator (1/X) and the denominator (2X), and then evaluates the limit, which approaches 0.
What is the shortcut rule mentioned in problem 16 for limits involving polynomials in both the numerator and the denominator?
-The shortcut rule states that if the degrees of the numerator and denominator polynomials are the same, the limit is the ratio of the leading coefficients.
In problem 17, why does the limit of x squared plus 4 over 8 to the power X approach 0 as X approaches infinity?
-The limit approaches 0 because the exponential function in the denominator (8 to the power X) grows faster than the polynomial in the numerator (x squared plus 4), making the overall fraction approach 0.
What is the condition for a function G to dominate a function F as X approaches infinity?
-A function G dominates function F if the limit of F as X approaches infinity is infinity, the limit of G as X approaches infinity is also infinity, and the limit of F(X)/G(X) as X approaches infinity equals 0.
How does the video explain the limit of x times the tangent of 7 over X as X approaches infinity?
-The video rewrites the expression algebraically to create a 0/0 indeterminate form, applies L'Hôpital's Rule, and evaluates the limit to find that it approaches 7.
What is the purpose of rewriting the expression in problem 21 involving arctan(3x) - π/2 over arctan(7x) - π/2?
-The purpose of rewriting is to transform the indeterminate form 0/0 into a form where L'Hôpital's Rule can be applied by making the substitution of X approach infinity more straightforward.
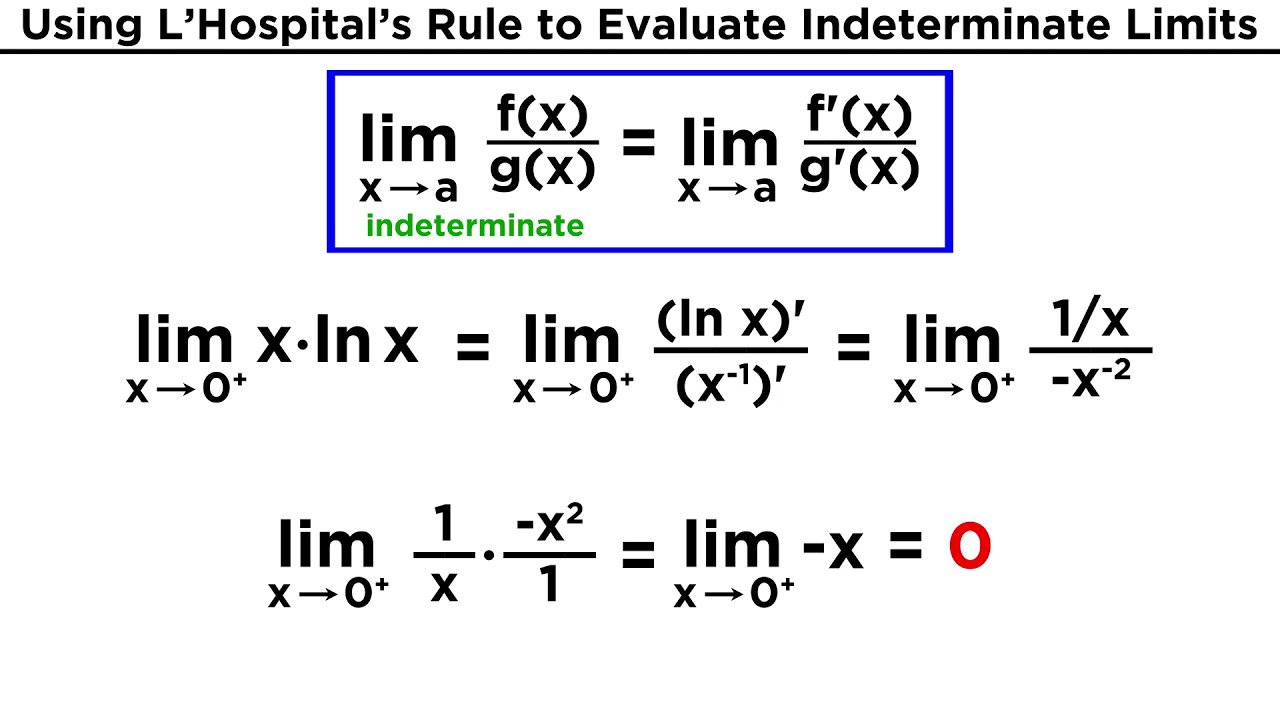
How does the video determine the limit of H(X) as X approaches 0 from the right for the function H(X) = 2x * ln(X)?
-The video uses L'Hôpital's Rule by taking the derivative of the numerator (2) and the denominator (-1/X^2), evaluates the limit, and finds that it approaches 0.
Outlines
📚 L'Hôpital's Rule Application in Limits
This paragraph introduces the application of L'Hôpital's Rule to solve limits as X approaches infinity, specifically for the function f(x) = x^3 / e^(3x). The approach involves recognizing the indeterminate form of infinity over infinity, applying the rule by taking derivatives of the numerator and denominator until a determinate limit is found, which in this case results in 0 after multiple applications of the rule.
🔍 Analyzing Limits with L'Hôpital's Rule and Polynomial Comparison
The second paragraph discusses the process of finding limits as X approaches infinity for different types of functions, including polynomials and exponentials. It explains how to use L'Hôpital's Rule to resolve indeterminate forms like infinity over infinity and also introduces a shortcut for when the numerator and denominator are polynomials of the same degree, leading to the ratio of leading coefficients as the limit.
📉 Limits Involving Growth Rates of Functions
This paragraph explores the concept of limits involving exponential and polynomial functions, emphasizing how exponential functions outgrow polynomials as X approaches infinity. It demonstrates the use of L'Hôpital's Rule in cases where the numerator and denominator grow without bound, resulting in the limit approaching 0 due to the faster growth of the exponential function.
🤔 Indeterminate Forms and Algebraic Manipulation
The fourth paragraph delves into solving limits with indeterminate forms that are not immediately solvable by L'Hôpital's Rule. It discusses algebraic manipulations to rewrite expressions in a form that allows the application of the rule, such as transforming X to 1/X to simplify the limit process and achieve determinate results.
📘 Applying L'Hôpital's Rule to Trigonometric and Inverse Trigonometric Functions
This paragraph examines the use of L'Hôpital's Rule in limits involving trigonometric and inverse trigonometric functions, particularly when the limit results in an indeterminate form like 0/0. It illustrates the process of applying the rule by taking derivatives of the numerator and denominator and simplifying the expressions algebraically to find the limit.
📐 Sketching Graphs and Determining Limits with Given Derivatives
The sixth paragraph presents a problem involving the sketching of possible graphs of functions F and G near a specific point and determining the limit of their ratio as X approaches that point. It uses given values and derivatives at the point to infer the behavior of the functions and apply L'Hôpital's Rule to find the limit, which turns out to be negative 2.
📈 Finding Asymptotes and Discontinuities in Rational Functions
This paragraph focuses on identifying vertical and horizontal asymptotes and discontinuities in a rational function defined by specific constants. It explains the process of finding asymptotes by setting the denominator to zero and determining the limit of the function as X approaches infinity and negative infinity, resulting in horizontal asymptotes. It also discusses the presence of removable discontinuities and how to represent them graphically.
📉 Analyzing the Limit of a Function with an Indeterminate Form
The seventh paragraph examines a function with an indeterminate form of 0^0 and explores the limit of its natural logarithm as X approaches zero from the right. It uses L'Hôpital's Rule to find the limit of the composed function and then applies logarithmic properties to determine the original function's limit, concluding that it approaches 1.
🌱 Understanding Function Dominance and Growth Rates
The final paragraph discusses the concept of function dominance, where one function grows faster than another as X approaches infinity. It provides examples of functions and explains why certain functions, like exponential and power functions, dominate others, such as logarithmic functions, based on their growth rates. This understanding can simplify the process of finding limits without the need for L'Hôpital's Rule when the dominance is clear.
Mindmap
Keywords
💡L'Hôpital's Rule
💡Indeterminate Form
💡Derivative
💡Limit
💡Infinity
💡Polynomial
💡Exponential Function
💡Natural Logarithm
💡Tangent
💡Arctan
💡Dominance in Functions
Highlights
Introduction to using L'Hôpital's Rule for solving limits of the form 0/0 or ∞/∞.
Explanation of the limit as X approaches infinity for the function X^3 / e^(3X) using substitution and L'Hôpital's Rule.
Graphical interpretation of limits involving infinity and the application of L'Hôpital's Rule.
Demonstration of repeated application of L'Hôpital's Rule for the indeterminate form ∞/∞.
Approach to limits involving natural logarithms and polynomials, showcasing L'Hôpital's Rule.
Simplification of limits by algebraic manipulation to fit L'Hôpital's Rule application criteria.
Comparison of polynomial and exponential growth rates to determine limits without calculus.
Use of L'Hôpital's Rule to find the limit of a function with a polynomial in the numerator and an exponential in the denominator.
Analysis of limits involving the product rule and chain rule for derivatives.
Strategy for rewriting complex limits algebraically to meet the conditions for L'Hôpital's Rule.
Application of L'Hôpital's Rule to trigonometric functions resulting in indeterminate forms.
Graphical and algebraic reasoning for limits involving exponential functions and polynomials.
Determination of vertical and horizontal asymptotes for rational functions using L'Hôpital's Rule and other methods.
Sketching possible graphs of functions with given derivatives and function values at specific points.
Explanation of why certain functions dominate others in terms of growth rate for large values of X.
Summary of the conditions under which L'Hôpital's Rule can be applied and its limitations.
Final review of the application of L'Hôpital's Rule to various types of limits and functions.
Transcripts
Browse More Related Video

Using L'Hopital's Rule to show that exponentials dominate polynomials

Calculus AB Homework 1.3 Evaluating Limits
Understanding Limits and L'Hospital's Rule

Introduction to l'Hôpital's rule | Derivative applications | Differential Calculus | Khan Academy

Challenging Limits from Graphs

L'Hopital's Rule (Zero Times Infinity)
5.0 / 5 (0 votes)
Thanks for rating: