L'Hopital's Rule (Zero Times Infinity)

turksvids
1 Jun 201303:53
EducationalLearning
32 Likes 10 Comments

TLDRThis video explains how to handle the indeterminate forms of 0/0 and 0*∞ using L'Hôpital's Rule. It demonstrates two examples: one with a limit as x approaches π/4, involving trigonometric functions, and another with x going to infinity, simplifying the expression and applying the rule to find the limit.

Takeaways
  • 📚 The video discusses L'Hôpital's Rule, focusing on cases involving 0/0 or ∞/∞ indeterminate forms.
  • 📉 The first example involves the limit as x approaches π/4 of (1 - tan(x)) * sec(2x), which results in a 0 * ∞ form.
  • 🔄 To handle the 0 * ∞ form, the video suggests inverting one of the terms, choosing the secant of 2x to invert.
  • 🔢 After inverting, the problem simplifies to 0/0, which is still an indeterminate form, but now manageable with L'Hôpital's Rule.
  • 🔑 The video demonstrates algebraic simplification to express 1/secant(2x) as cosine(2x), making the limit easier to evaluate.
  • 🧭 Applying L'Hôpital's Rule, the derivative of the top function (secant of x) and the derivative of the bottom function (-2 sin of 2x) are used.
  • 📈 Substituting π/4 into the derivatives gives a result of -2, leading to the conclusion that the limit is 1.
  • 🌐 The second example involves the limit as x approaches ∞ of x * sin(π/x), which results in an ∞ * 0 form.
  • 🔄 The video suggests inverting x to transform the ∞ * 0 form into an indeterminate 0/0 form, suitable for L'Hôpital's Rule.
  • 🔑 The derivatives used are the derivative of the top function (cosine of π/x * π/x^2) and the derivative of the bottom function (-1/x^2).
  • 📉 Simplifying the derivatives and substituting as x approaches ∞ results in a limit of π, as cosine(0) is 1.
  • 🚀 The video concludes with two examples of applying L'Hôpital's Rule to handle 0/0 and ∞/0 indeterminate forms, providing a clear guide for solving similar problems.
Q & A

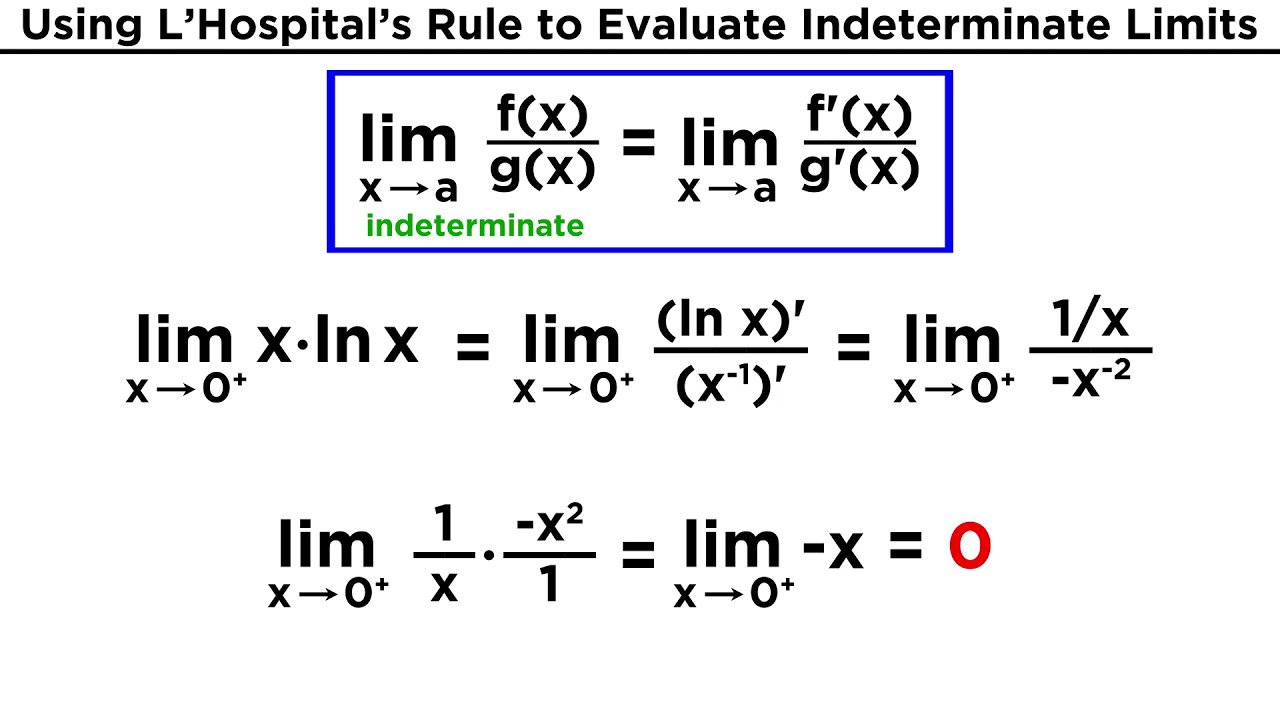
  • What is L'Hôpital's Rule used for?

    -L'Hôpital's Rule is used for evaluating limits that involve indeterminate forms, such as 0/0 or ∞/∞, by taking the derivative of the numerator and denominator and then re-evaluating the limit.

  • What is the first example limit given in the script?

    -The first example limit is as x approaches π/4 of (1 - tan(x)) * sec(2x).

  • Why does the script mention the tangent of π/4 equals 1?

    -The tangent of π/4 equals 1 because it's a known value from trigonometry, and it simplifies the expression to 0 in the numerator when x approaches π/4.

  • What happens when secant of 2x approaches infinity?

    -When secant of 2x approaches infinity, it means that the cosine of 2x, which is the reciprocal of secant, approaches zero.

  • Why is it necessary to invert one of the terms in the first example?

    -Inverting one of the terms is necessary to change the indeterminate form of 0 * ∞ into 0/0, which allows the application of L'Hôpital's Rule.

  • What is the choice made for the term to invert in the first example?

    -The choice made in the first example is to invert sec(2x), turning it into 1/sec(2x), which simplifies to cos(2x).

  • What is the result of applying L'Hôpital's Rule to the first example?

    -After applying L'Hôpital's Rule and substituting π/4, the result of the first example limit is 1.

  • What is the second example limit given in the script?

    -The second example limit is as x approaches infinity of x * sin(π/x).

  • Why is the term 'infinity times zero' considered an indeterminate form?

    -The term 'infinity times zero' is considered an indeterminate form because it could potentially evaluate to any number, depending on how the infinity and zero are approached.

  • What does the script suggest to do with the second example to handle the indeterminate form?

    -The script suggests inverting x to change the indeterminate form from ∞ * 0 to 0/0, which then allows the use of L'Hôpital's Rule.

  • What is the final result of the second example limit after applying L'Hôpital's Rule?

    -After applying L'Hôpital's Rule to the second example, the final result of the limit as x approaches infinity is π.

Outlines
00:00
📚 Introduction to L'Hôpital's Rule with 0/∞ Indeterminate Form

This paragraph introduces the application of L'Hôpital's Rule in scenarios where the limit results in an indeterminate form of 0/∞. The example given involves the limit as x approaches π/4 of (1 - tan(x)) * sec(2x). The presenter explains the initial setup, where the tangent of π/4 equals 1, leading to a 0 in the numerator, and the secant of 2 * π/4 approaches infinity, resulting in an indeterminate form of 0 * ∞. The presenter then decides to invert the secant function to apply L'Hôpital's Rule, simplifying the expression to 1/sec(2x), which is equivalent to cos(2x). The application of the rule leads to the calculation of the derivative of the numerator and the denominator, resulting in a limit value of 1.

🚀 Applying L'Hôpital's Rule to ∞/0 Indeterminate Form

In this second example, the presenter discusses the limit as x approaches infinity of sin(π/x). The limit results in an indeterminate form of ∞ * 0. To address this, the presenter chooses to invert x, transforming the expression into sin(π/x) over 1/x. This leads to another indeterminate form of 0/0, which is suitable for applying L'Hôpital's Rule. The derivatives of the numerator and denominator are calculated, with the chain rule simplifying the expression, leaving the cosine of π/x. Evaluating this as x approaches infinity, where π/x approaches 0, results in the limit being π, as cosine of 0 is 1.

Mindmap
Keywords
💡L'Hôpital's Rule
L'Hôpital's Rule is a mathematical procedure used to evaluate limits that involve indeterminate forms, such as 0/0 or ∞/∞. It states that if the limit of the ratio of the derivatives of two functions exists, then the limit of the original functions' ratio also exists and is equal to this derivative ratio. In the video, L'Hôpital's Rule is used to solve two examples where the original functions approach indeterminate forms, demonstrating its application in calculus.
💡Indeterminate Form
An indeterminate form occurs when the limit of a function results in a form that does not provide a clear answer, such as 0/0 or ∞/∞. The video script discusses how L'Hôpital's Rule can be applied to resolve these forms, which is central to the theme of evaluating limits in calculus.
💡Limit
A limit in calculus is the value that a function or sequence approaches as the input or index approaches some value. The script provides examples of limits, such as when x approaches π/4 or as x approaches infinity, to illustrate the process of applying L'Hôpital's Rule.
💡Tangent
The tangent function in trigonometry is defined as the ratio of the length of the opposite side to the length of the adjacent side in a right-angled triangle. In the script, the tangent of π/4 is mentioned as being equal to 1, which is a key point in evaluating the first example limit.
💡Secant
Secant is the reciprocal of the cosine function in trigonometry. It is used in the script to illustrate a function that approaches infinity as its argument approaches π/2, which is a crucial step in evaluating the first limit example.
💡Derivative
A derivative in calculus represents the rate at which a function changes with respect to its variable. The script mentions taking the derivative of the numerator and the denominator when applying L'Hôpital's Rule, which is essential for finding the limit of the original function.
💡Cosine
The cosine function is a fundamental trigonometric function that relates the angle of a right-angled triangle to the ratio of the adjacent side over the hypotenuse. In the script, the cosine of 2x is used after simplifying the secant function, which is part of the process to apply L'Hôpital's Rule.
💡Sine
The sine function, like cosine, is a trigonometric function that relates to the ratio of the opposite side to the hypotenuse in a right-angled triangle. The script refers to the sine function when taking the derivative of the denominator in the application of L'Hôpital's Rule.
💡Invert
In the context of the script, to invert a function means to replace it with its reciprocal. This is a strategy used to simplify the expression before applying L'Hôpital's Rule, as seen when the secant of 2x is inverted to cosine of 2x.
💡Chain Rule
The chain rule is a fundamental principle in calculus for finding the derivative of a composite function. The script mentions that the chain rule simplifies the process when applying L'Hôpital's Rule, as it often cancels out the denominator when differentiating.
💡Evaluation
Evaluation in the context of the script refers to the process of substituting specific values into the function to find the limit. The script describes the evaluation of the limit after applying L'Hôpital's Rule, resulting in the final answer for each example.
Highlights

Introduction to L'Hôpital's Rule for the indeterminate forms 0/0, ∞/∞, or 0*∞.

Example of applying L'Hôpital's Rule to the limit as x approaches π/4 of (1 - tan(x)) * secant(2x).

Tangent of π/4 equals 1, leading to a 0 in the numerator.

Secant of 2 * π/4 approaches ∞ as x approaches π/2, due to cosine approaching 0.

Inversion strategy for dealing with 0 * ∞, choosing to invert secant(2x).

Algebraic simplification to 1 / secant(2x), which is equivalent to cosine(2x).

Application of L'Hôpital's Rule to the new form, resulting in a derivative of the top and bottom functions.

Substitution of π/4 into the simplified expression, yielding a limit value of 1.

Second example with x approaching ∞ of x * sin(π/x), another indeterminate form of ∞ * 0.

Inversion strategy for ∞ * 0, choosing to invert x.

Limit simplification to 0/0 after inversion, setting up for L'Hôpital's Rule.

Derivatives of the top and bottom functions after applying L'Hôpital's Rule.

Simplification using the chain rule, which cancels out the denominator.

Evaluation of the limit as x approaches ∞, resulting in a final value of π.

Explanation of the chain rule's role in simplifying trigonometric limits.

Conclusion summarizing the two examples and the successful application of L'Hôpital's Rule.

Transcripts

Browse More Related Video

l'Hospital Practice | MIT 18.01SC Single Variable Calculus, Fall 2010

Introduction to l'Hôpital's rule | Derivative applications | Differential Calculus | Khan Academy

Understanding Limits and L'Hospital's Rule

L'hopital's rule

Calculus AB Homework 4.3: L'Hospital's Rule

Challenging Limits from Graphs

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
L'Hôpital's RuleCalculusIndeterminate FormsLimit CalculationTrigonometric LimitsDerivative ApplicationMathematics EducationProblem SolvingEducational ContentMath Tutorial