2.2 - Problem #8 (Calc)
TLDRThis video script discusses problem number eight from section 2.2, which involves evaluating the limit of a function as h approaches zero, a common calculus concept. The function given is f(x) = x^2, and the script guides through the process of finding the slope of the tangent line by simplifying the expression f(x + h) - f(x)/h. The cancellation of terms and factorization lead to the conclusion that the slope at x = -3 is -6, demonstrating the derivative's application in determining the tangent's slope at a specific point.
Takeaways
- 📚 The problem discusses a common calculus concept: the limit of the form \(\lim_{h \to 0} \frac{f(x+h) - f(x)}{h}\), which is used to find the slope of the tangent line to a curve at a given point.
- 🔍 The specific function given in the problem is \(f(x) = x^2\), and the goal is to evaluate the limit as \(h\) approaches 0 for a given \(x\) value.
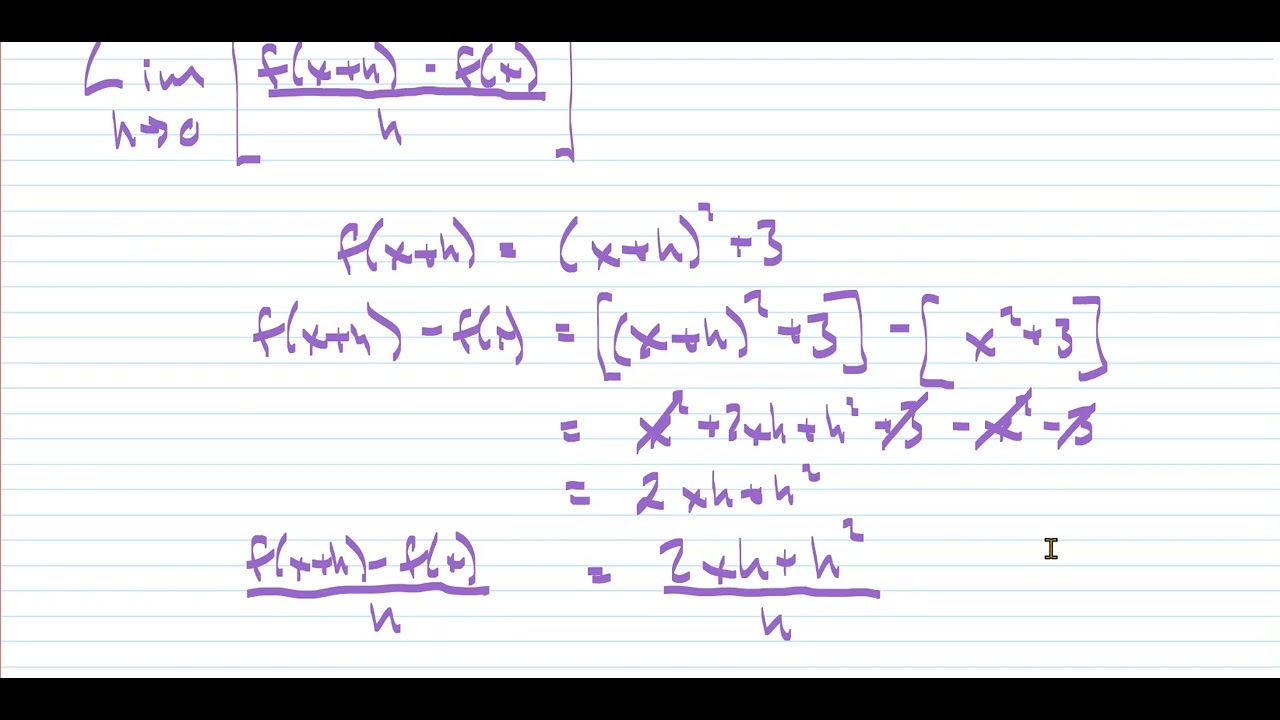
- 📘 The process begins by substituting \(x + h\) into the function, resulting in \((x + h)^2\).
- 🧩 The expansion of \((x + h)^2\) is done, yielding \(x^2 + 2xh + h^2\).
- ✂️ The next step involves subtracting \(x^2\) from the expanded form, simplifying the expression to \(2xh + h^2\).
- 🔄 The script then factors out an \(h\) from the terms \(2xh + h^2\), preparing for the limit operation.
- 📉 As \(h\) approaches 0, the terms involving \(h\) in the numerator cancel out, leaving \(2x\) as the result of the limit.
- 📌 The value of \(x\) is specified as \(-3\), and substituting this into the simplified limit gives \(2 \times (-3)\), which is \(-6\).
- 📐 The final takeaway is that the slope of the tangent line to the function \(f(x) = x^2\) at \(x = -3\) is \(-6\).
- 📝 The process is a clear demonstration of how to find the derivative of a function at a specific point, which is a fundamental concept in calculus.
Q & A
What is the main concept being discussed in the script?
-The script discusses the concept of finding the slope of a tangent line to a curve at a given point using the limit of a function as h approaches zero.
What is the specific limit form being evaluated in the script?
-The limit form being evaluated is \( \lim_{h \to 0} \frac{f(x + h) - f(x)}{h} \), which represents the derivative of the function f at point x.
What function is being used to demonstrate the concept in the script?
-The function used in the script is \( f(x) = x^2 \).
What is the value of x for which the limit is being evaluated?
-The limit is being evaluated for \( x = -3 \).
How does the script simplify the expression for the limit?
-The script simplifies the expression by substituting \( f(x) \) with \( x^2 \), expanding \( (x + h)^2 \), and then canceling out the \( x^2 \) terms.
What is the result of the simplified expression before taking the limit?
-The simplified expression before taking the limit is \( \frac{2xh + h^2}{h} \).
What happens when the limit is taken as h approaches zero?
-As h approaches zero, the terms \( 2xh \) and \( h^2 \) in the numerator become negligible compared to \( h \) in the denominator, leaving \( 2x \) as the result of the limit.
What is the final value of the limit after plugging in x = -3?
-After plugging in \( x = -3 \), the final value of the limit is \( 2 \times (-3) = -6 \).
What does the final value of the limit represent in the context of the function?
-The final value of the limit represents the slope of the tangent line to the function \( f(x) = x^2 \) at the point where \( x = -3 \).
Why is the limit form important in calculus?
-The limit form is important in calculus because it is the foundation for understanding derivatives, which describe the rate of change of a function and the slopes of tangent lines at any point on the function's graph.
Can the process described in the script be applied to any differentiable function?
-Yes, the process described in the script can be applied to any differentiable function to find the slope of its tangent line at a specific point.
Outlines
📚 Calculus Limit Problem Explanation
This paragraph introduces a common calculus problem involving the limit of a function as h approaches zero. The main objective is to find the slope of the tangent line to the function at a given point x. The problem is presented in the context of the limit of the form \( \lim_{h \to 0} \frac{f(x+h) - f(x)}{h} \). The function provided is f(x) = x^2, and the process involves substituting x with (x+h), expanding the squared term, and simplifying the expression to isolate the limit. The cancellation of terms and factoring out of h leads to the discovery that the limit is 2x, which represents the slope of the tangent line.
Mindmap
Keywords
💡Limit
💡Function
💡Slope
💡Tangent Slope
💡Difference Quotient
💡Distribute
💡Factor
💡Approach Zero
💡Cancel
💡Evaluate
💡Instantaneous Rate of Change
Highlights
Problem 8 for section 2.2 involves evaluating a limit that finds the tangent slope.
The limit is of the form \( \lim_{h \to 0} \frac{f(x + h) - f(x)}{h} \), which is common in calculus.
The function given is \( f(x) = x^2 \).
The process starts by substituting \( x + h \) into the function, resulting in \( (x + h)^2 \).
Expanding \( (x + h)^2 \) gives \( x^2 + 2xh + h^2 \).
The next step is to subtract \( x^2 \) from the expanded form.
The expression simplifies to \( \frac{2xh + h^2}{h} \) after cancellation.
Factoring out an \( h \) from the numerator simplifies the expression further.
The limit as \( h \) approaches 0 is then considered.
The \( h \) terms cancel out, leaving \( 2x \).
The value of \( x \) is given as -3, which is substituted into the expression.
The slope of the tangent line is found to be 2 at \( x = -3 \).
The final calculation results in a slope of -6 for the function at \( x = -3 \).
The process demonstrates the calculation of the derivative as a limit.
The problem illustrates the concept of the derivative as the slope of the tangent line.
The solution provides a clear example of applying calculus to find the slope at a specific point.
The transcript explains the steps in a logical and methodical manner.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: