Lecture 06: Derivatives the Esay Way Symbol Pushing
TLDRThis calculus lecture focuses on the algebraic computation of derivatives, essential for modeling physical and conceptual situations. It starts with basic functions, like linear and quadratic, and demonstrates how their derivatives can be algebraically derived. The lecture then generalizes this process to polynomials, introducing rules for derivatives of sums, products, and quotients of functions. It also covers the derivatives of trigonometric functions, such as sine and cosine, and introduces exponential functions, particularly e^x, whose derivative is the function itself. The goal is to provide a systematic method for finding derivatives of various functions, which can be applied to practical problems in different fields.
Takeaways
- 📝 The derivative is the slope of the tangent line to the graph of a function.
- 📊 Calculus helps deal with specific functions modeling physical and conceptual situations.
- 📈 Understanding derivatives graphically involves analyzing the car's movement on a road.
- 🔢 Calculating derivatives algebraically is essential for functions in various fields like physics, economics, and geometry.
- ⚙️ Derivatives can be computed for functions involving basic arithmetic operations and trigonometric functions.
- 🔍 The process of finding the derivative involves evaluating the limit as delta x approaches zero.
- 🧮 The power rule for differentiation states that the derivative of x^n is n * x^(n-1).
- ➕ The derivative of the sum of two functions is the sum of their derivatives.
- 🔢 The product rule and quotient rule explain how to find derivatives of products and quotients of functions.
- 📉 The derivative of the sine function is the cosine function, and the derivative of the cosine function is the negative sine function.
- 📈 The exponential function e^x has the unique property that its derivative is equal to itself.
- 🔧 Calculus provides a mechanical way to derive algebraic expressions that describe relationships in various fields.
Q & A
What is the relationship between the derivative and the slope of a tangent line?
-The derivative of a function at a given point is equal to the slope of the tangent line to the graph of the function at that point.
Why is the derivative important in practical applications?
-The derivative is important in practical applications because it helps in modeling physical and conceptual situations in fields such as physics, economics, and geometry, allowing us to compute rates of change, velocities, and slopes algebraically.
How does the derivative of a constant function look graphically?
-Graphically, the derivative of a constant function is a horizontal line, indicating that the slope is zero at every point, as the constant function does not change.
What is the derivative of the function f(x) = x^2, and how does it relate to the graph of the function?
-The derivative of the function f(x) = x^2 is f'(x) = 2x. This means that the slope of the tangent line to the graph of f(x) = x^2 at any point x is 2x, which can be seen graphically as a straight line with a slope that increases as x increases.
How does the process of taking the derivative of a function algebraically involve?
-The process involves using the limit definition of the derivative, which is the limit of [f(x + Δx) - f(x)] / Δx as Δx approaches 0. This process often simplifies to applying rules of differentiation such as the power rule, product rule, and chain rule.
What is the general rule for the derivative of a function of the form f(x) = x^n?
-The general rule for the derivative of a function of the form f(x) = x^n is f'(x) = n * x^(n-1), where n is a constant representing the exponent.
How does the derivative change when a constant is multiplied by a function?
-When a constant is multiplied by a function, the derivative of the new function is the constant multiplied by the derivative of the original function. This is because the constant factor scales the rate of change by the same amount.
What is the relationship between the sum of two functions and their derivatives?
-The derivative of the sum of two functions is equal to the sum of their derivatives. That is, if h(x) = f(x) + g(x), then h'(x) = f'(x) + g'(x).
What is the derivative of a function that is the product of two other functions?
-The derivative of a function that is the product of two other functions is given by the product rule: (f(x) * g(x))' = f'(x) * g(x) + f(x) * g'(x), which is not simply the product of the two derivatives.
What are the derivatives of the basic trigonometric functions sine and cosine?
-The derivative of the sine function is the cosine function, and the derivative of the cosine function is the negative of the sine function. That is, (sin(θ))' = cos(θ) and (cos(θ))' = -sin(θ).
What is special about the exponential function e^x?
-The exponential function e^x has the unique property that its derivative is equal to itself. That is, the derivative of e^x with respect to x is e^x.
How do the number of oscillations of a polynomial function relate to its derivative?
-The number of oscillations of a polynomial function decreases by one when taking its derivative. This can be observed both graphically and algebraically.
Outlines
📚 Introduction to Derivatives in Calculus
The video begins with an introduction to the concept of derivatives in calculus, emphasizing their importance in various fields such as physics, economics, and geometry. The instructor explains that derivatives are used to find the slope of the tangent line to a graph at any point, which can be understood both graphically and through physical examples like a car moving on a road. The focus of this lecture is to teach how to compute derivatives algebraically for functions involving basic arithmetic operations and trigonometric functions. The instructor also mentions that understanding derivatives algebraically is crucial for students as it allows them to find the velocity of a car from its position function without resorting to laborious limit computations or graphical estimations.
🔍 Deriving Derivatives Algebraically for Basic Functions
This paragraph delves into the algebraic process of deriving derivatives for basic functions. The instructor uses the example of the function f(x) = x^2, explaining how to compute its derivative by evaluating the limit of the difference quotient as delta x approaches zero. The process involves expanding the function at x plus delta x, simplifying the expression, and taking the limit to find that the derivative is 2x. The instructor also discusses how multiplying the function by a constant affects the derivative, demonstrating that the derivative of a constant times x squared is 2 times the constant times x. The concept is further expanded to include functions of the form x to a power, showing that the derivative of x cubed is 3x squared, and establishing a general rule for the derivative of x to the power of n, which is n times x to the power of n-1.
📈 Understanding Derivatives of Sums and Constant Multiples
The instructor explains how to find the derivatives of more complex functions that are constructed from simpler ones. For the sum of two functions, the derivative is the sum of the derivatives of the individual functions. Similarly, when a function is multiplied by a constant, the derivative of the new function is the constant times the derivative of the original function. The instructor provides an example with the function 5x^3 + 2x, showing how to apply the rules for derivatives of sums and constant multiples to find the derivative, which is 15x^2 + 2. The paragraph also touches on the fact that adding a constant to a function does not change its derivative, as the constant's derivative is zero.
📚 Product and Quotient Rules for Derivatives
This section introduces the product and quotient rules for finding derivatives of functions that are products or quotients of two other functions. Contrary to a common misconception, the derivative of the product of two functions is not simply the product of their derivatives. Instead, the product rule states that the derivative of the product of f(x) and g(x) is f'(x)g(x) + f(x)g'(x). Similarly, the quotient rule is more complex than one might initially assume, stating that the derivative of f(x)/g(x) is [g(x)f'(x) - f(x)g'(x)] / [g(x)]^2. The instructor emphasizes the importance of these rules for accurately computing derivatives in more advanced scenarios.
📉 Derivatives of Polynomial and Trigonometric Functions
The instructor discusses the process of taking derivatives of polynomial functions, which are sums of terms involving x raised to various powers. Using the function f(x) = x^3 - 6x^2 + 9x + 1 as an example, the derivative is computed to be 3x^2 - 12x + 9. The video also explores the relationship between the shape of the original function's graph and its derivative, noting that the number of oscillations in the derivative's graph is one less than the original polynomial. Trigonometric functions are introduced, with the sine and cosine functions defined in terms of a unit circle. The instructor explains that the derivative of sine is cosine, and the derivative of cosine is negative sine, highlighting the oscillating nature of these functions and their derivatives.
🌐 Exponential Functions and Their Unique Derivatives
The final paragraph of the script introduces exponential functions, focusing on the unique property of the exponential function e^x, whose derivative is equal to itself. The instructor explains that this function can be visualized by starting with a 45-degree line and continually steepening the slope as the function's value increases. The constant e, approximately equal to 2.71828, is highlighted as the base of the natural exponential function, and it is shown that the derivative of any exponential function a^x (where 'a' is a constant) is simply 'a' times the original function a^x. This leads to a summary of the derivatives covered in the lecture, including powers of x, sine, cosine, and e^x, providing a foundation for understanding how to mechanically compute derivatives for various types of algebraic expressions.
Mindmap
Keywords
💡Derivative
💡Tangent Line
💡Algebraic Expression
💡Limit
💡Slope
💡Position Function
💡Velocity
💡Trigonometric Functions
💡Exponential Functions
💡Product Rule
💡Quotient Rule
Highlights
Derivatives are the slope of the tangent line to a graph of a function.
Practical power of calculus involves computing derivatives algebraically for functions used in physics, economics, and geometry.
Derivatives can be obtained mechanically from algebraic expressions involving basic arithmetic operations and trigonometric functions.
For a position function p(t) = c*t, the derivative at each point is the constant c, representing the slope of the line.
The derivative of f(x) = x^2 is 2x, computed by evaluating the limit of (f(x + Δx) - f(x)) / Δx as Δx approaches 0.
Graphical representation shows the derivative of a function aligns with the slope of the tangent line at any point on the graph.
The derivative of a function multiplied by a constant is the constant times the derivative of the function.
The derivative of x^n (where n is a constant) is n*x^(n-1), following a general pattern for derivatives of functions to the power.
The sum of two functions' derivative is the sum of their individual derivatives.
Adding a constant to a function does not change its derivative, as the slope of the tangent line remains the same.
The product rule for derivatives states that the derivative of a product of two functions is not simply the product of their derivatives.
The derivative of a polynomial function can be found using the sum and product rules, reducing the oscillations by one.
Trigonometric functions sine and cosine are defined in relation to the unit circle and their derivatives are related to each other.
The derivative of sine is cosine, and the derivative of cosine is -sine, with graphical representations showing their oscillations.
Exponential functions have the unique property that their derivatives are equal to themselves, exemplified by e^x.
The derivative of any exponential function a^x (where 'a' is a constant) is the function itself multiplied by the constant 'a'.
A table of derivatives is provided to understand the mechanical process of finding derivatives for various types of functions.
Transcripts
Browse More Related Video

Calculus Chapter 1 Lecture 4 Computing Taylor Series
Derivatives of Trigonometric Functions - Product Rule Quotient & Chain Rule - Calculus Tutorial

Evaluating Integrals With Trigonometric Functions
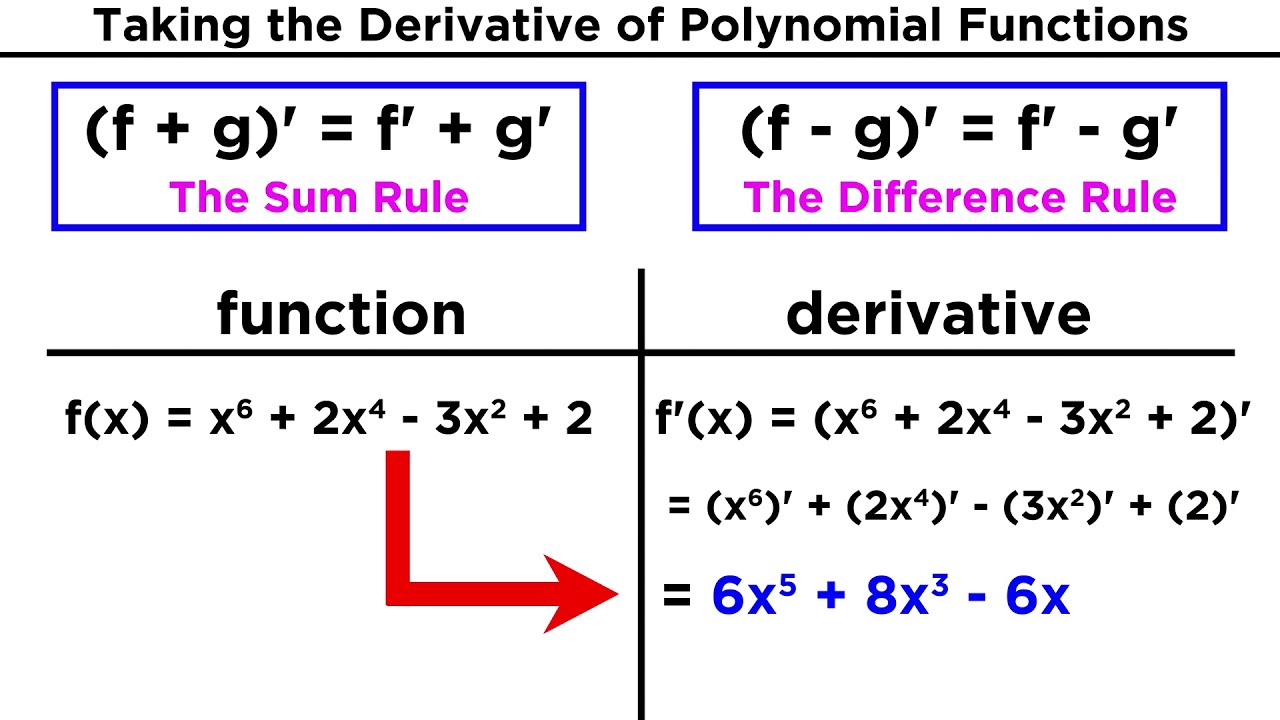
Derivatives of Polynomial Functions: Power Rule, Product Rule, and Quotient Rule

Math1325 Lecture 11 1 Derivatives of Logarithm
Calculus 1 Lecture 2.5: Finding Derivatives of Trigonometric Functions
5.0 / 5 (0 votes)
Thanks for rating: