Polar coordinates 3 | Parametric equations and polar coordinates | Precalculus | Khan Academy

Khan Academy
2 Feb 200907:37
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers a detailed tutorial on converting Cartesian functions to polar coordinates and vice versa. It begins with the conversion of the equation 3y - 7x = 10 into polar form, utilizing trigonometric identities and algebraic manipulation. The script then demonstrates converting y = 2x - 3 into polar coordinates, followed by examples of converting polar equations like r = 4sin(θ) and r = sin(θ) + cos(θ) back into Cartesian form. The process involves substituting r and θ with their respective expressions in terms of x and y, and simplifying the equations. The script concludes with a discussion on the graphical representation of these equations and the intuition behind the relationships between r and θ.

Takeaways
  • 📝 Converting Cartesian functions to polar coordinates involves substituting x and y with r and theta.
  • 🔍 For the equation 3y - 7x = 10, substitute y = r sin(theta) and x = r cos(theta) to get r in terms of theta.
  • 🔧 After substitution, factor out r to simplify the equation and solve for r.
  • 💡 The function r in terms of theta can be written as r = 10 / (3 sin(theta) - 7 cos(theta)).
  • 🔄 For the equation y = 2x - 3, use the same substitution method and factor out r.
  • 📐 After factoring and simplifying, the equation r = -3 / (sin(theta) - 2 cos(theta)) is obtained.
  • 🔄 To convert from polar to Cartesian coordinates, use the given polar function r = 4 sin(theta) and replace sin(theta) and cos(theta) with y/r and x/r respectively.
  • 🔗 Multiply through by r to eliminate the denominator and use r^2 = x^2 + y^2 to convert to Cartesian coordinates.
  • 🔢 For the polar function r = sin(theta) + cos(theta), substitute sin(theta) = y/r and cos(theta) = x/r, then simplify.
  • 📊 Converting polar to Cartesian coordinates often involves algebra and trigonometry to express the relationship in terms of x and y.
Q & A

  • What is the purpose of converting Cartesian functions to polar coordinates?

    -The purpose of converting Cartesian functions to polar coordinates is to express the relationship between variables in a different coordinate system that might be more suitable for certain problems, especially those involving circular or radial symmetry.

  • What is the Cartesian equation given in the script to be converted to polar coordinates?

    -The Cartesian equation given is 3y - 7x = 10.

  • How are y and x represented in terms of polar coordinates?

    -In polar coordinates, y is represented as r * sin(theta) and x is represented as r * cos(theta), where r is the radius and theta is the angle from the positive x-axis.

  • What is the result of substituting y and x with their polar coordinate equivalents in the equation 3y - 7x = 10?

    -After substitution, the equation becomes 3(r * sin(theta)) - 7(r * cos(theta)) = 10.

  • What is the simplified form of the equation after factoring out r?

    -After factoring out r, the equation simplifies to r * (3 * sin(theta) - 7 * cos(theta)) = 10.

  • What is the final form of the equation in polar coordinates after dividing by the expression (3 * sin(theta) - 7 * cos(theta))?

    -The final form is r = 10 / (3 * sin(theta) - 7 * cos(theta)).

  • How is the function r(theta) related to the polar coordinates conversion?

    -The function r(theta) represents the radius as a function of the angle theta, which is a way to express the relationship between r and theta in polar coordinates.

  • What is the Cartesian equation y = 2x - 3 converted to in terms of polar coordinates?

    -After conversion, the equation becomes r * sin(theta) = 2 * (r * cos(theta)) - 3, which simplifies to r * (sin(theta) - 2 * cos(theta)) = -3.

  • What is the polar function r = 4 * sin(theta) converted to in Cartesian coordinates?

    -The polar function r = 4 * sin(theta) converts to the Cartesian equation x^2 + y^2 - 4y = 0.

  • How does the script describe the process of converting polar coordinates to Cartesian coordinates?

    -The script describes the process by using the relationships y/r = sin(theta) and x/r = cos(theta) to substitute and simplify the polar equations into Cartesian form.

  • What is the significance of the polar function r = a^2?

    -The polar function r = a^2 represents a circle with a constant radius of 'a' in polar coordinates, which, when converted to Cartesian coordinates, becomes the equation x^2 + y^2 = a^4.

  • How does the script suggest visualizing the relationships between r and theta?

    -The script suggests using a graphing calculator set to polar coordinates to visualize the relationships and to see how the radius changes as theta varies.

Outlines
00:00
📐 Converting Cartesian to Polar Coordinates

This paragraph explains the process of converting a Cartesian function to polar coordinates. It begins with the equation 3y - 7x = 10 and substitutes y with r * sin(θ) and x with r * cos(θ), leading to an expression in terms of r and θ. The paragraph demonstrates algebraic manipulation to isolate r, resulting in r = (10 * sin(θ) - 7 * cos(θ)) / 3. It also covers another example, y = 2x - 3, and shows the conversion to polar form, ending with r = -3 / (sin(θ) - 2 * cos(θ)). The focus is on using trigonometric identities and algebra to perform the conversions.

05:01
🔄 Converting Polar to Cartesian Coordinates

The second paragraph delves into converting polar functions to Cartesian coordinates. It starts with the polar function r = 4 * sin(θ) and uses the relationships y = r * sin(θ) and x = r * cos(θ) to convert it into Cartesian form, resulting in x^2 + y^2 = 4y. The paragraph also discusses the conversion of r = sin(θ) + cos(θ) to Cartesian coordinates, which leads to x^2 + y^2 = y + x. Lastly, it touches on the concept of a circle in polar coordinates, given by r = a^2, and its Cartesian equivalent, x^2 + y^2 = a^4. The summary emphasizes the algebraic steps and the use of the toolkit for conversions between the two coordinate systems.

Mindmap
Keywords
💡Cartesian coordinates
Cartesian coordinates refer to a mathematical coordinate system that specifies each point uniquely in a plane by a set of numerical coordinates, which are based on the distance from two defined lines that meet at a right angle, known as the x-axis and y-axis. In the video, the theme revolves around converting equations from Cartesian to polar coordinates and vice versa, using Cartesian coordinates as the starting point for these transformations.
💡Polar coordinates
Polar coordinates are a two-dimensional coordinate system in which each point on a plane is determined by a distance from a reference point and an angle from a reference direction. The video focuses on the conversion between polar and Cartesian coordinates, illustrating how equations can be transformed to represent the same geometric figures in different coordinate systems.
💡Conversion
Conversion in the context of the video refers to the process of transforming equations from one coordinate system to another. It is a key process demonstrated in the video, showing how to change equations given in Cartesian coordinates to their polar counterparts and the other way around.
💡r sine of theta
The term 'r sine of theta' is used in the video to represent the y-component of a point in polar coordinates, where 'r' is the radius or distance from the origin and 'theta' is the angle measured from the positive x-axis. It is a fundamental relationship used in converting between Cartesian and polar coordinates, as seen when the instructor substitutes 'y' with 'r sine of theta' during the conversion process.
💡r cosine of theta
Similar to 'r sine of theta', 'r cosine of theta' represents the x-component of a point in polar coordinates. The video uses this term to illustrate the conversion process, showing how 'x' can be replaced with 'r cosine of theta' when transforming equations from Cartesian to polar coordinates.
💡SOHCAHTOA
SOHCAHTOA is a mnemonic used to remember the trigonometric ratios for sine, cosine, and tangent in a right-angled triangle. In the video, it is mentioned as the basis for the relationships between x, y, and r, theta in polar coordinates, providing a simple memory aid for the conversion formulas.
💡Algebra
Algebra is a branch of mathematics that uses symbols and rules to manipulate, solve, and analyze mathematical expressions. The video emphasizes the use of algebraic manipulation to convert equations between Cartesian and polar coordinate systems, showcasing the algebraic steps needed to perform these conversions.
💡Trigonometry
Trigonometry is a branch of mathematics that deals with the relationships between the sides and angles of triangles, particularly right-angled triangles. In the video, trigonometry is essential for understanding how to substitute and manipulate expressions involving sine and cosine when converting between coordinate systems.
💡Implicit equation
An implicit equation is a form of equation where the variable does not appear explicitly isolated on one side. In the video, the instructor arrives at an implicit equation 'x squared plus y squared is equal to 4y' when converting a polar equation to Cartesian coordinates, which still represents the geometric relationship without explicitly solving for one variable.
💡Graphing calculator
A graphing calculator is a specialized calculator that can be used to graph functions and equations. The video script mentions the use of a graphing calculator in polar coordinates to visualize the geometric shapes represented by the equations, suggesting a practical tool for understanding the conversions discussed.
💡Toolkit
In the context of the video, the 'toolkit' refers to the set of mathematical formulas and relationships used to convert between Cartesian and polar coordinates. The instructor 'cuts and pastes' this toolkit, emphasizing the importance of these formulas in performing the conversions demonstrated throughout the video.
Highlights

The process of converting Cartesian functions to polar coordinates is introduced.

Substitution of Cartesian coordinates (x, y) with polar coordinates (r, θ) using y = r * sin(θ) and x = r * cos(θ).

Simplification of the equation 3y - 7x = 10 into polar form results in r * (3sin(θ) - 7cos(θ)) = 10.

Factoring out r from the equation to express r as a function of θ.

Conversion of the equation y = 2x - 3 into polar coordinates by isolating r and θ variables.

Factoring out r from the equation r * (sin(θ) - 2cos(θ)) = -3 to find r in terms of θ.

The conversion of polar function r = 4 * sin(θ) into Cartesian coordinates by using algebraic manipulation.

Derivation of the Cartesian form x² + y² = 4y from the polar equation r = 4 * sin(θ).

Use of trigonometric identities and algebra to convert polar to Cartesian coordinates.

Introduction of the polar function r = sin(θ) + cos(θ) and its potential graphing on a polar coordinate system.

Substitution of sin(θ) = y/r and cos(θ) = x/r to convert r = sin(θ) + cos(θ) into Cartesian form.

Result of the conversion is the equation r² = y + x, which is then identified as x² + y².

Abstract polar equation r = a² is discussed, indicating a constant radius circle in Cartesian coordinates.

Conversion of r = a² into Cartesian coordinates by squaring both sides, resulting in x² + y² = a⁴.

Emphasis on the importance of algebra and trigonometry in converting between Cartesian and polar coordinates.

A promise to explore the graphs of these equations in future videos to provide more intuition.

The video concludes with a summary of the algebraic process for converting between coordinate systems.

Transcripts

Browse More Related Video

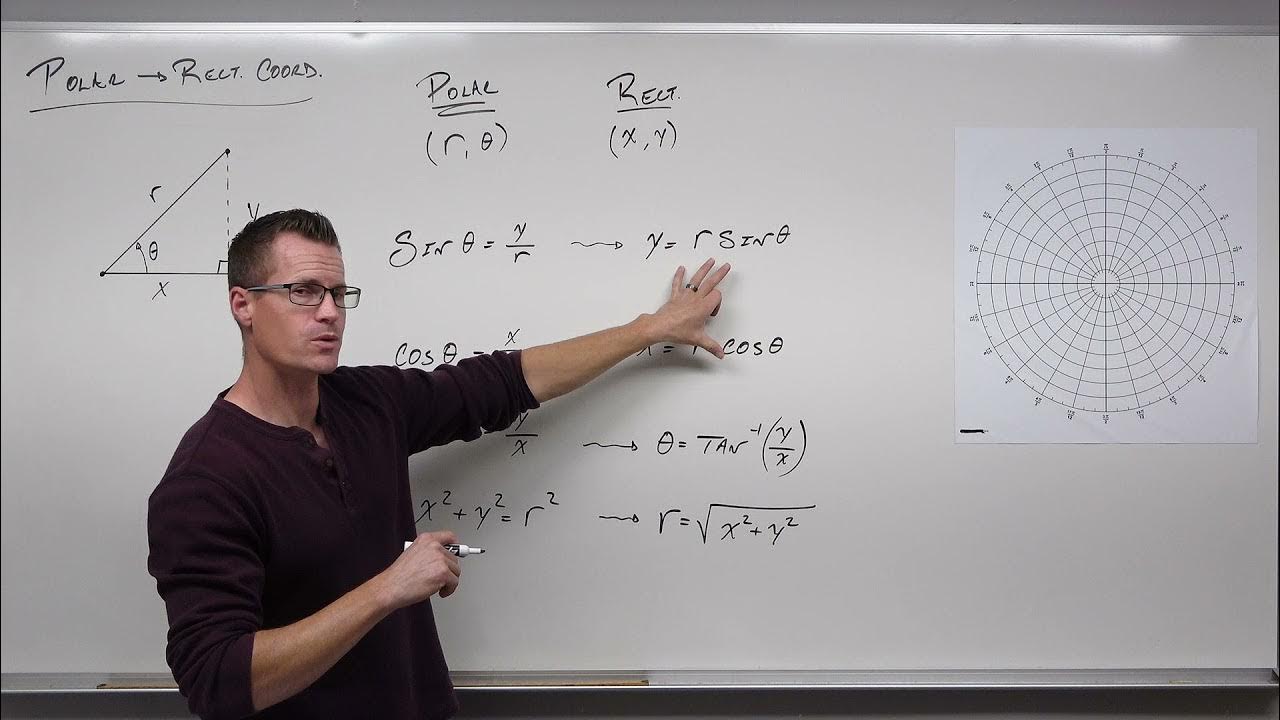
How to Convert From Polar Coordinates to Rectangular Coordinates (Precalculus - Trigonometry 37)

How to Convert From Rectangular Equations to Polar Equations (Precalculus - Trigonometry 39)

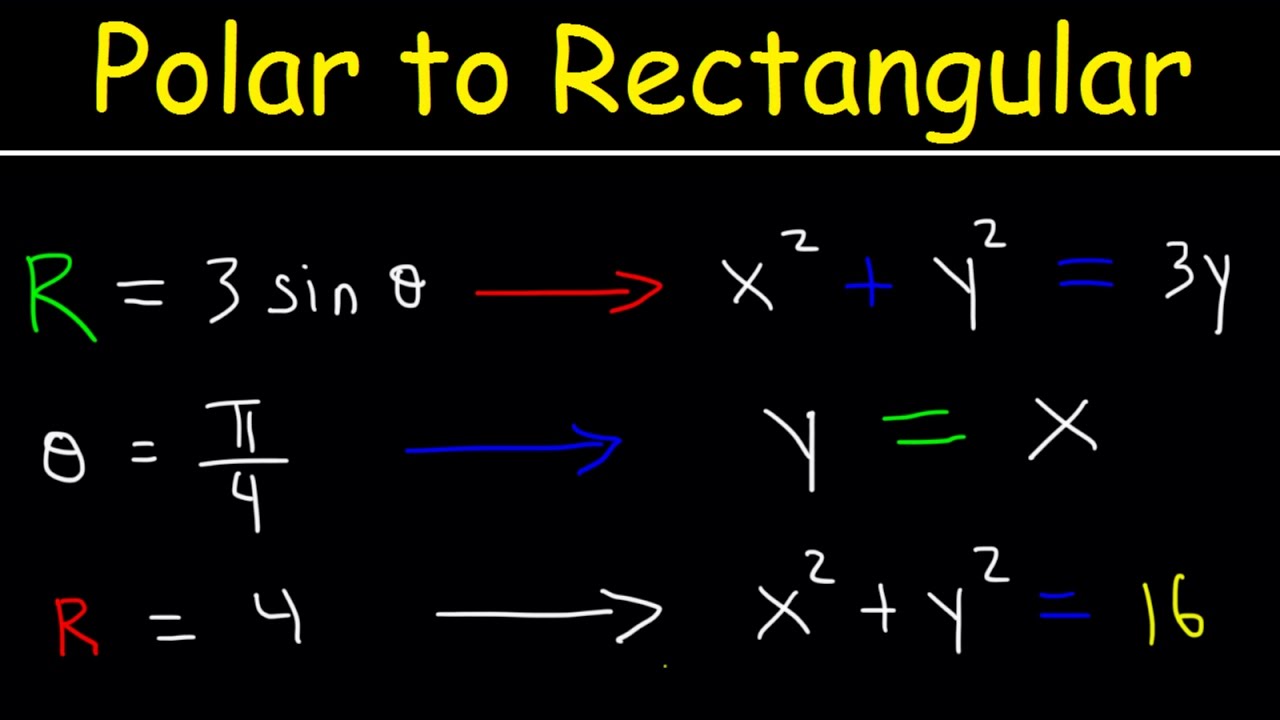
Polar Equations to Rectangular Equations, Precalculus, Examples and Practice Problems

Polar coordinates 2 | Parametric equations and polar coordinates | Precalculus | Khan Academy

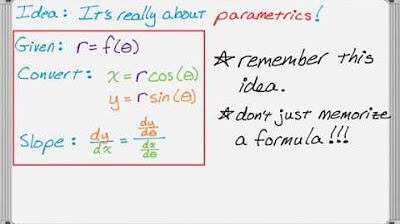
Finding Slope of a Polar Curve in Calculus

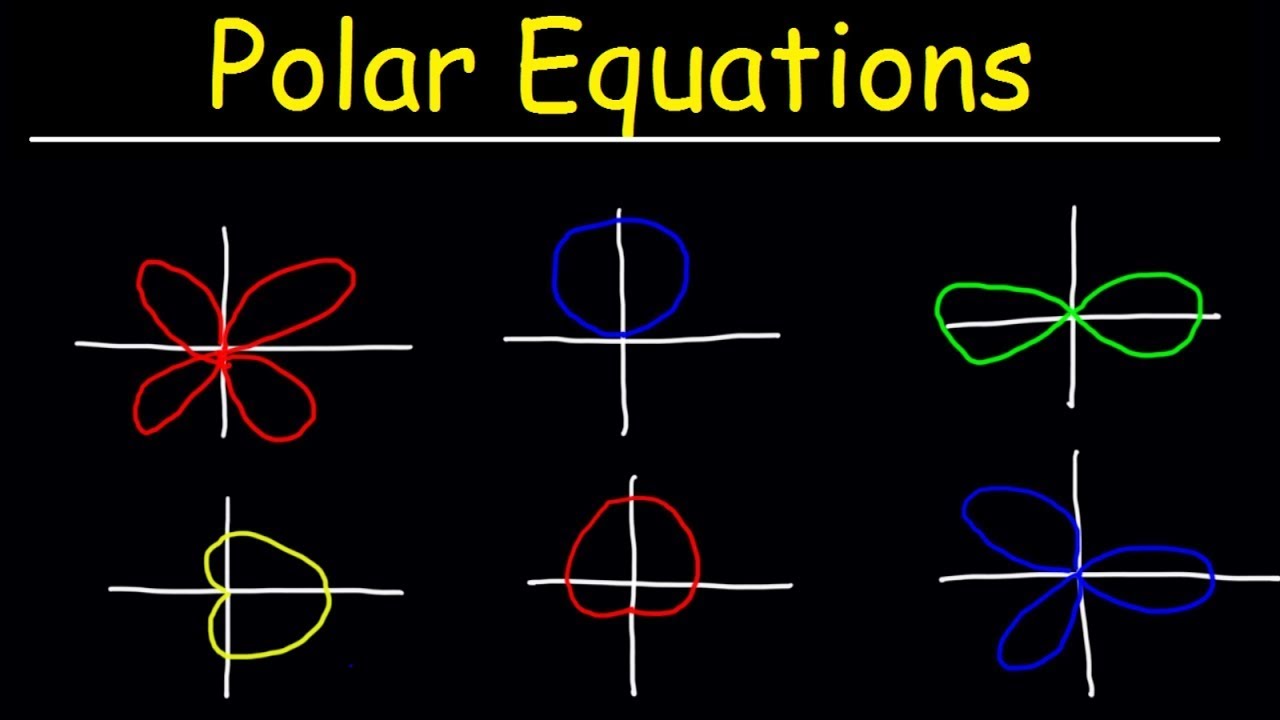
How To Graph Polar Equations

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Cartesian CoordinatesPolar CoordinatesMathematics TutorialTrigonometryAlgebraConversion ToolsEducational ContentGeometry LessonsGraphing CalculatorMathematical Concepts