Second Derivative of Implicit Function

turksvids
21 Sept 201303:24
EducationalLearning
32 Likes 10 Comments

TLDRThe video script outlines the process of finding the second derivative of an implicitly defined function, specifically a hyperbola represented by the equation x^2 - y^2 = 25. The presenter begins by taking the first derivative, applying the chain rule for the function y(x), and solving to find dy/dx = x/y. To find the second derivative, the derivative of dy/dx is taken, resulting in an expression involving x, y, and dy/dx. The presenter then applies algebraic manipulation and substitution to simplify the expression, factoring out a negative sign and substituting the original equation to obtain the final form of the second derivative as -25/y^3. The summary emphasizes the importance of completing the quotient rule and being on the lookout for opportunities to simplify expressions, particularly with conic sections, to provide a clear and concise explanation of the process.

Takeaways
  • 📚 Start by identifying the function to differentiate, which is x^2 - y^2 = 25, representing a hyperbola.
  • 🔑 Use the chain rule to differentiate y^2 since y is a function of x, resulting in 2y(dy/dx).
  • 🧭 After differentiating, set the expression equal to zero and solve for the first derivative dy/dx = x/y.
  • 🔍 To find the second derivative, differentiate dy/dx with respect to x, considering it as an implicit differentiation.
  • ⚖️ Apply the quotient rule for differentiation, which involves differentiating the numerator and denominator separately.
  • 🔁 Substitute the previously found first derivative (dy/dx = x/y) into the expression for the second derivative.
  • 📐 Perform algebraic manipulation to simplify the expression, resulting in y^2 - x^2 / y^3.
  • 📉 Factor out a negative sign to get -(x^2 - y^2) / y^3, which simplifies the expression.
  • 🔗 Recognize that x^2 - y^2 equals 25 from the original equation and use this to further simplify the second derivative to -25/y^3.
  • 📝 Keep an eye out for opportunities to simplify expressions, especially with conic sections and implicit differentiations.
  • 🎓 The process demonstrates the importance of completing the quotient rule and not stopping midway through the differentiation process.
Q & A

  • What is the equation of the function being discussed in the transcript?

    -The equation of the function is x^2 - y^2 = 25, which represents a hyperbola.

  • What is the first derivative of the function with respect to x?

    -The first derivative, using the chain rule for y as a function of x, is dy/dx = x/y.

  • How does the chain rule apply when finding the derivative of y^2 with respect to x?

    -The chain rule is applied by recognizing that y is a function of x, so the derivative of y^2 with respect to x is 2y(dy/dx).

  • What is the issue encountered when trying to find the second derivative?

    -The issue is that the first derivative is also implicitly defined, containing both x and y, which makes it difficult to express the second derivative solely in terms of x and y.

  • What is the quotient rule used for in this context?

    -The quotient rule is used to find the derivative of the first derivative (dy/dx) with respect to x, as it involves a fraction where the numerator and denominator are both functions of x.

  • How is the second derivative simplified in terms of the original equation?

    -The second derivative is simplified by factoring out a negative sign and using the original equation x^2 - y^2 = 25 to rewrite the expression as -25/y^3.

  • What is the common mistake that people make when taking derivatives, as mentioned in the transcript?

    -A common mistake is to stop in the middle of applying the quotient rule, not completing the differentiation process.

  • Why is it important to complete the quotient rule when taking derivatives?

    -Completing the quotient rule is important to accurately find the derivative of a quotient of two functions, ensuring that the derivative is correctly expressed.

  • What is the final expression for the second derivative of the given function?

    -The final expression for the second derivative is -25/y^3, after simplification using the original equation.

  • Why is it beneficial to look for ways to simplify implicit second derivatives?

    -Simplifying implicit second derivatives can make the expression easier to work with and understand, especially when dealing with conic sections or other complex equations.

  • What is a common challenge when dealing with second derivatives of implicitly defined functions?

    -A common challenge is expressing the second derivative in terms of only x and y, without the derivative notation (dy/dx), which requires careful application of algebraic manipulation and substitution.

  • How does the process of finding the second derivative in the transcript help in understanding the behavior of the function?

    -Finding the second derivative provides insight into the concavity and inflection points of the function, which are important for understanding the function's shape and behavior, especially in the context of conic sections.

Outlines
00:00
📚 Finding the Second Derivative of an Implicitly Defined Function

The video script begins with the goal of finding the second derivative of a hyperbolic function implicitly defined by x^2 - y^2 = 25. The first step involves taking the derivative of the equation, which leads to 2x - 2y(dy/dx) = 0. The chain rule is applied to account for y as a function of x, resulting in the first derivative dy/dx = x/y. The process then moves on to finding the second derivative, which involves differentiating dy/dx, applying the quotient rule, and simplifying the expression. The second derivative is found to be (y^2 - x^2) / y^3, which is then factored and simplified further to -25/y^3, using the original equation x^2 - y^2 = 25. The video emphasizes the importance of completing the quotient rule and provides a simplified form of the second derivative, highlighting a common simplification technique when dealing with conic sections and implicit differentiation.

Mindmap
Keywords
💡Implicitly defined function
An implicitly defined function is a relationship between variables that is not explicitly given in the form y = f(x) or x = g(y). In the video, the function x^2 - y^2 = 25 is an example of an implicitly defined function, representing a hyperbola. The process of finding derivatives of such functions often involves applying the chain rule when differentiating with respect to one of the variables.
💡Derivative
A derivative in calculus represents the rate at which a quantity changes with respect to another quantity. In the context of the video, the first and second derivatives of the function x^2 - y^2 = 25 are being calculated to understand how the function changes with respect to x. The first derivative dy/dx is found using the chain rule, and the second derivative involves further differentiation.
💡Chain rule
The chain rule is a fundamental theorem in calculus for finding the derivative of a composite function. It states that the derivative of a function composed of two functions is the product of the derivative of the outer function and the derivative of the inner function. In the video, the chain rule is used to find the first derivative of y^2 with respect to x, since y is a function of x.
💡Hyperbola
A hyperbola is a type of conic section defined by the set of all points where the difference of the distances to two fixed points (foci) is constant. The video's function x^2 - y^2 = 25 is a standard equation for a hyperbola, which is the geometric shape being analyzed throughout the script.
💡Quotient rule
The quotient rule is a method in calculus for finding the derivative of a function that is the quotient of two other functions. It states that the derivative of u/v is (vu' - uv') / v^2. In the video, the quotient rule is applied when differentiating the first derivative dy/dx with respect to x to find the second derivative.
💡Second derivative
The second derivative of a function is the derivative of the first derivative. It provides information about the concavity of a function and can indicate points of inflection. In the video, the second derivative is sought to understand the behavior of the hyperbola more deeply, and it is found by differentiating the expression for dy/dx.
💡Simplifying expressions
Simplifying expressions involves using algebraic techniques to make a mathematical expression more straightforward or easier to understand. In the context of the video, the presenter simplifies the expression for the second derivative by factoring and using the given equation x^2 - y^2 = 25 to rewrite the final expression as -25/y^3.
💡Conic sections
Conic sections are curves obtained by intersecting a cone with a plane. They include circles, ellipses, parabolas, and hyperbolas. The video focuses on the hyperbola, which is a type of conic section, and discusses the process of finding derivatives of its equation.
💡Constant
In calculus, a constant is a term that does not contain any variables and thus does not change during differentiation. In the video, the constant 25 from the equation x^2 - y^2 = 25 is noted when differentiating and simplifying the expression for the second derivative.
💡Algebraic manipulation
Algebraic manipulation refers to the process of rearranging and reorganizing terms in a mathematical expression to simplify it or to make it easier to solve. In the video, algebraic manipulation is used to combine terms and find a common denominator when working with the expression for the second derivative.
💡Substitution
Substitution is a mathematical technique where one expression is replaced with another to simplify a problem or to make further calculations possible. In the video, substitution is used to replace dy/dx with x/y in the expression for the second derivative, which helps to express the derivative solely in terms of x and y.
Highlights

The second derivative of an implicitly defined function is being found using the example of a hyperbola x^2 - y^2 = 25.

The first derivative dy/dx is obtained by applying the chain rule for y as a function of x, resulting in dy/dx = x/y.

The second derivative involves taking the derivative of the first derivative, which also requires the use of the quotient rule.

The quotient rule is applied correctly without stopping mid-rule, which is a common mistake when differentiating.

Substituting the expression for dy/dx into the second derivative equation simplifies the expression.

Algebraic manipulation leads to a common denominator, resulting in the numerator y^2 - x^2/y.

Factoring out a negative sign from the numerator simplifies the expression further.

The original equation x^2 - y^2 = 25 is used to rewrite the second derivative as -25/y^3.

The process demonstrates the importance of completing the quotient rule and not stopping mid-rule when differentiating.

Substitution of known expressions is a key step in simplifying the second derivative.

Algebraic techniques like finding a common denominator are crucial for simplifying complex derivative expressions.

Factoring can greatly simplify the final expression of the second derivative.

Using the original equation to substitute and simplify the derivative is a clever technique.

The process emphasizes the need for careful and methodical application of differentiation rules.

The example of a hyperbola is used to illustrate the process of finding second derivatives of implicitly defined functions.

The video provides a step-by-step guide to finding second derivatives, which is helpful for learners.

The presenter warns about common pitfalls when differentiating, such as stopping the quotient rule mid-way.

The final simplified form of the second derivative is -25/y^3, showcasing the power of algebraic simplification.

Transcripts

Browse More Related Video

Second derivatives (implicit equations): find expression | AP Calculus AB | Khan Academy

Implicit Differentiation for Calculus - More Examples, #1

Implicit Differentiation for Calculus - More Examples #2

How to use implicit differentiation on an equation for dy dx

Trig Implicit Differentiation Example

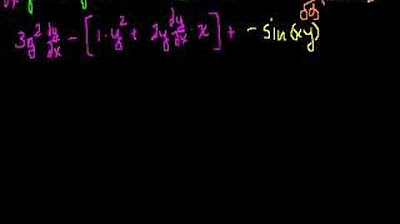
Implicit Differentiation (part 2)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Derivative CalculationImplicit FunctionHyperbola MathChain RuleQuotient RuleMathematical TutorialSecond DerivativeFunction AnalysisAlgebraic SimplificationMath EducationGeometry Concepts