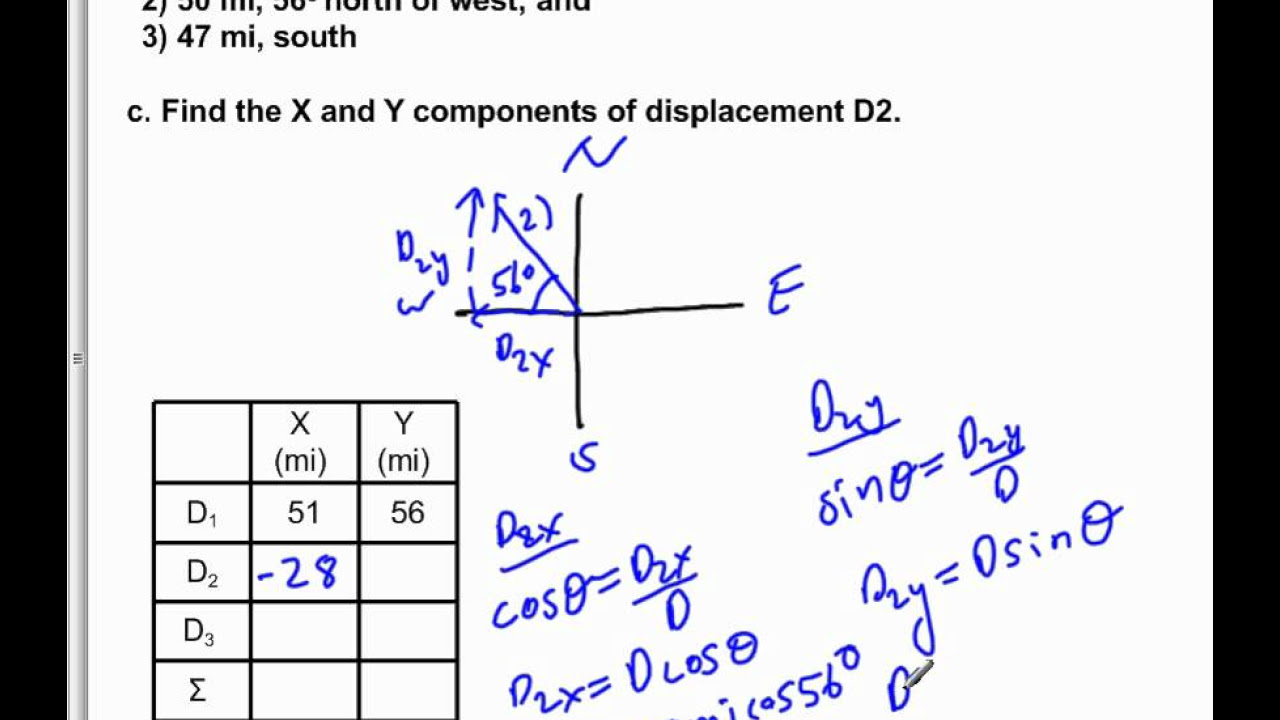
Adding and Subtracting Vectors
TLDRThe video script offers an insightful guide on vector manipulation, focusing on the fundamental concepts of vector addition, subtraction, and scalar multiplication. It emphasizes the importance of maintaining a vector's magnitude and direction during these operations. The process of vector addition is likened to walking in one direction and then another, resulting in a resultant vector that represents the net displacement. Subtraction is portrayed as reversing one vector's direction, leading to a resultant vector that reflects the difference in direction and magnitude. Scalar multiplication is explained as simply multiplying the vector's components by a scalar value, resulting in a vector that is scaled in length but retains the same direction. The script concludes with a practical demonstration of these operations using algebraic expressions and graphical representations, providing a clear and engaging explanation suitable for viewers new to vector mathematics.
Takeaways
- 📐 **Vector Addition**: When adding vectors, you align them so that the end of one vector is at the start of the other to form a resultant vector.
- 🔄 **Vector Direction**: The direction of a vector is crucial; it should not change during addition or subtraction.
- 📏 **Vector Magnitude**: The length of a vector remains constant during vector operations; only the direction changes when vectors are added or subtracted.
- ↔️ **Vector Subtraction**: Subtracting a vector is equivalent to adding a vector in the opposite direction, represented as negative.
- 🔢 **Algebraic Operations**: Vectors are added or subtracted algebraically by combining their respective x and y components.
- 📈 **Scalar Multiplication**: Multiplying a vector by a scalar involves multiplying both the x and y components by that scalar, resulting in a vector that is longer or shorter but in the same direction.
- 🚶 **Geometric Interpretation**: You can visualize vector addition as walking in one direction and then another, effectively taking a shortcut.
- 🔀 **Vector Translation**: Vectors can be moved (translated) without altering their magnitude or direction, only their position changes.
- 🔴 **Resultant Vector**: The final vector obtained from adding or subtracting vectors is called the resultant vector.
- 🛤️ **Vector Path**: The path created by adding vectors can be thought of as a path taken by moving in the direction of one vector and then the other.
- 🔵 **Component-wise Operation**: Vector operations are performed component-wise, meaning each corresponding component (x, y) of the vectors is operated on separately.
Q & A
What is a vector?
-A vector is a mathematical object that has both magnitude and direction. It is typically represented by an arrow with a specific length and direction in a coordinate system.
How do you add two vectors together?
-To add two vectors, you place one vector so that the end of the first vector coincides with the start of the second vector. The resultant vector starts at the beginning of the first vector and ends at the end of the second vector.
What is the geometric interpretation of subtracting a vector?
-Subtracting a vector geometrically means taking the original vector and reversing its direction, creating what is known as the negative of the vector. The resultant vector starts from the end of the negative vector and ends at the start of the original vector.
How do you represent vector addition algebraically?
-Algebraically, vector addition is represented by adding the corresponding components of the vectors. If vector A has components (x1, y1) and vector B has components (x2, y2), their sum is (x1 + x2, y1 + y2).
What is the scalar multiplication of a vector?
-Scalar multiplication involves multiplying both the x and y components of a vector by a scalar value. If the vector has components (x, y) and the scalar is 'k', the result is a new vector with components (kx, ky).
Why is it important not to change the length or the angle of a vector when adding or subtracting?
-Changing the length or the angle of a vector would alter its magnitude and direction, resulting in a different vector. The operations of addition and subtraction are meant to combine or separate vectors while preserving their original properties.
How does the direction of a vector affect the resultant vector when adding two vectors?
-The direction of each vector affects the overall direction of the resultant vector. When adding vectors, the resultant vector's direction is determined by the orientation of the individual vectors and their respective angles.
What happens to the resultant vector when you add vectors that are in opposite directions?
-When adding vectors in opposite directions, the resultant vector's direction will be determined by the vector with the greater magnitude. The resultant vector will point in the direction of the larger vector and its magnitude will be the difference in magnitudes of the two vectors.
How do you algebraically subtract one vector from another?
-To algebraically subtract one vector from another, you subtract the corresponding components of the second vector from the first vector. If vector U has components (x1, y1) and vector V has components (x2, y2), the result of U - V is (x1 - x2, y1 - y2).
What is the effect of scalar multiplication on the direction of a vector?
-Scalar multiplication does not change the direction of a vector. It only affects the magnitude (length) of the vector. If the scalar is positive, the direction remains the same; if the scalar is negative, the direction is reversed.
Can you add vectors that are not in the same plane?
-Vectors can be added only if they are in the same plane or space. If vectors are not in the same plane, they must be expressed in a common coordinate system before they can be added or subtracted.
Outlines
🔄 Vector Addition and Subtraction Basics
The script introduces the concept of vector addition by describing how two vectors, A and B, can be added by aligning them end-to-end without altering their magnitude or direction, forming a resultant vector A + B. It further explains vector subtraction, where the second vector is reversed (negative B) before being added to the first vector, creating a resultant vector A - B. This section of the script uses straightforward visual explanations to clarify these operations, emphasizing the importance of maintaining the original characteristics of vectors during these processes.
📐 Algebraic Operations on Vectors
This segment covers the algebraic methods for adding, subtracting, and scalar multiplication of vectors. It demonstrates these operations by calculating the components of vectors U and V in a plane. The vector addition U + V results in new coordinates by simply adding the respective components. For subtraction U - V, it subtracts the components accordingly. Scalar multiplication is also introduced, where a vector's components are multiplied by a scalar, extending the vector's magnitude while keeping its direction consistent. The script provides clear examples and explains these concepts using an XY coordinate system to visualize the operations.
Mindmap
Keywords
💡Vector
💡Addition of Vectors
💡Subtraction of Vectors
💡Magnitude and Direction
💡Scalar Multiplication
💡Components
💡Resultant Vector
💡Algebraic Operations
💡Directional Movement
💡Vector Representation
💡Distributive Property
Highlights
Vectors can be added together by aligning them head-to-tail without changing their length or direction.
When adding vectors, place one vector so that where it ends, the other begins.
The resultant vector of adding two vectors together represents the combined effect of their directions and magnitudes.
To subtract a vector, reverse its direction by 180°, maintaining its original length.
The resultant vector of vector subtraction shows the effect of one vector overcoming the other.
Vector operations can be visualized geometrically or algebraically.
Algebraic operations on vectors involve adding or subtracting their respective x and y components.
Scalar multiplication of a vector involves multiplying both the x and y components by the scalar value.
Scalar multiplication results in a vector that is longer or shorter than the original, but in the same direction.
Vectors can be shifted left, right, up, or down without altering their inherent properties.
Maintaining the angle and length of vectors is crucial during addition and subtraction to preserve their properties.
The concept of vector addition can be likened to navigating through different directions to reach a destination.
Visual representation of vector addition and subtraction can be achieved through simple geometric shifts.
Understanding vector operations is fundamental in fields that involve direction and magnitude, such as physics and engineering.
The distributive property is applied in scalar multiplication to find the new vector components.
Vector operations are a fundamental tool in linear algebra and have wide-ranging applications in mathematics and science.
The process of adding and subtracting vectors can be easily demonstrated with simple drawings and algebraic expressions.
Vectors are defined by both magnitude (length) and direction, making them a powerful tool for representing quantities in two dimensions.
The video provides a clear and concise explanation of vector operations, making it accessible for beginners.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: