Physics 15 Torque (22 of 25) More Examples: 4 V(max)=? Before Car Tips
TLDRThe video script explores the concept of torque in the context of a car driving at high speeds along a circular path. It explains that insufficient friction can cause a car to slip out, while excessive speed on a well-frictioned surface can lead to a vehicle flip, particularly for trucks with high centers of gravity. The video derives a formula to calculate the maximum velocity (V-max) a car can achieve before tipping over, which depends on the car's width (D), the radius of the turn (R), and the height of the center of mass (H). The formula is V = √(G * D * R / (2 * H)), where G is the acceleration due to gravity. The script also discusses how wider cars, larger turn radii, and lower centers of gravity can increase the maximum safe velocity and reduce the risk of tipping over, making it a practical application of torque in vehicle dynamics.
Takeaways
- 🚗 The concept of torque is used to determine the maximum velocity a car can drive on a circular path before tipping over.
- 🔄 At the moment a car is about to tip, the sum of all torques about a pivot point is zero, indicating equilibrium.
- ⚖️ The torque equation involves the centrifugal force causing a clockwise turn and the weight of the car causing a counterclockwise torque.
- 📐 The maximum velocity (v-max) is derived from the balance of forces acting on the car, considering the car's mass, velocity, radius of turn, and height of the center of mass.
- √ The formula for v-max is the square root of (G * D * R) / (2 * H), where G is the acceleration due to gravity, D is the width of the car, R is the radius of the curve, and H is the height to the center of mass.
- 🚦 Wider cars (greater distance between tires) can achieve higher maximum velocities before tipping over.
- 🛣️ Larger radius of the curve reduces the likelihood of a car tipping over, which is why it's preferred in highway design.
- 📏 A lower center of mass (height to the center of mass) results in a higher maximum velocity and decreased likelihood of the car tipping over.
- 🏎️ High-performance or racing cars often have a lower center of gravity to improve stability and handling at high speeds.
- 🚚 Trucks with high centers of gravity and heavy loads are more susceptible to tipping over at high speeds on circular paths.
- ⚓️ The stability of a vehicle can be improved by lowering the center of mass, which is why sports cars and racing vehicles are designed with low profiles.
Q & A
What happens if there's not enough friction between the tires and the road when a car is driving fast in a circular path?
-If there's not enough friction, the car will simply slip out of the road.
What is the potential outcome when there is plenty of friction and the car is driving too fast in a circular path?
-With plenty of friction, driving too fast can cause the car to flip over, especially if it's a truck with a high center of gravity.
What factors are considered in calculating the maximum velocity (V-max) a car can drive before tipping over?
-The factors considered are the velocity of the car (V), the radius of the turn (R), the mass of the car (M), and the height of the center of mass (H).
At the moment a car is about to tip over, what happens to the force acting on the car?
-At the moment the car is about to tip over, there is no weight pushing down on the car on the side of the tire closest to the pivot point.
What is the significance of the pivot point (point A) in the context of the car tipping over?
-The pivot point (point A) is where we assume the car will tip over. At this point, the sum of all torques about point A equals zero, which is an equilibrium moment.
What is the relationship between the centrifugal force and the torque that causes the car to flip over?
-The centrifugal force causes a negative torque, which, when not balanced by other forces, can cause the car to flip over.
How does the width of the car (D) factor into the equation for the maximum velocity?
-The width of the car (D) is used to calculate the perpendicular distance from the line of action of the force to the pivot point, which is then used in the formula to find the maximum velocity (V-max).
What is the formula for calculating the maximum velocity (V-max) a car can drive before tipping over?
-The formula is V = √(G * D * R / (2 * H)), where G is the acceleration due to gravity, D is the width of the car, R is the radius of the curve, and H is the height of the center of mass.
How does the width of the car affect the likelihood of it tipping over?
-The wider the car, the farther the tires are spaced apart, which increases the maximum velocity and makes it less likely for the car to tip over.
What is the effect of the radius of the curve on the car's stability while driving in a circular path?
-The larger the radius of the curve, the less likely the car will tip over. This is why freeway entrances and off-ramps are designed with a large radius to minimize the risk.
Why is it important to keep the center of mass of a vehicle low?
-A lower center of mass reduces the maximum velocity at which the vehicle can tip over, making it less likely to flip, especially in situations where the road is slick or icy.
How does the height of the center of mass (H) affect the maximum velocity (V-max) of the car?
-The greater the height of the center of mass (H), the lower the maximum velocity (V-max) the car can achieve before tipping over. Conversely, a higher center of gravity increases the likelihood of the car tipping over at lower velocities.
Outlines
🚗 Maximum Velocity and Car Stability
The first paragraph discusses the concept of torque in relation to a car driving at high speeds along a circular path. It explains that insufficient friction can cause the car to slip, while excessive speed with sufficient friction can lead to the car flipping over, particularly with heavy trucks. The key to preventing a flip is to calculate the maximum velocity (V-max) before the car tips over, using the car's velocity (V), the radius of the turn (R), the car's mass (M), and the height of the center of mass (H). The equilibrium moment is reached when the sum of all torques about a pivot point (A) equals zero. The forces involved are the centrifugal force pushing the car sideways and the weight of the car creating a counterclockwise torque. The formula derived for V-max is the square root of (G * D * R) / (2 * H), where G is the acceleration due to gravity, D is half the width of the car, and H is the height of the center of mass. The paragraph also explores how the width of the car, the radius of the curve, and the height of the center of mass affect the maximum velocity and stability.
🔍 Impact of Center of Gravity on Stability
The second paragraph emphasizes the importance of the car's center of gravity in relation to its stability and the likelihood of tipping over. It states that a higher center of gravity increases the chances of the car tipping over, as indicated by a decrease in V-max when H becomes larger. This highlights the practical application of torque in solving real-world problems, such as vehicle design and road safety, by understanding the factors that contribute to a vehicle's stability during high-speed maneuvers.
Mindmap
Keywords
💡Torque
💡Friction
💡Centripetal Force
💡Centrifugal Force
💡Velocity
💡Radius of the Turn
💡Mass of the Car
💡Height of the Center of Mass
💡Width of the Car
💡Equilibrium Moment
💡Pivot Point
Highlights
The concept of torque can be used to find the maximum velocity a car can drive before tipping over when taking a turn.
A car may slip out of the road or flip over depending on the friction between the tires and the road.
Driving too fast on a circular path can cause a car to flip, especially trucks with a high center of gravity.
At the moment a car is about to tip over, there is no weight pushing down on the outside wheel.
The sum of all torques about a pivot point equals zero at the moment of equilibrium when the car is about to tip.
The centrifugal force causes a negative torque while the car's weight causes a positive torque.
The equation for the maximum velocity (V-max) involves the car's mass, velocity, radius of the turn, width of the car, and height of the center of mass.
The maximum velocity a car can drive before tipping over is given by the formula V = sqrt(G * D * R / (2 * H))
Wider cars with tires farther apart have a higher maximum velocity and are less likely to tip over.
A larger radius of the curve reduces the likelihood of a car tipping over.
Freeway entrance and off-ramp curves are designed with a large radius to minimize the risk of cars tipping over or sliding off.
A lower center of mass increases the maximum velocity a car can achieve before tipping over.
A higher center of mass reduces the maximum velocity and increases the likelihood of the car tipping over.
The concept of torque is used to solve this practical problem involving the dynamics of a car taking a turn.
Understanding the relationship between torque, mass, velocity, and the car's dimensions can prevent accidents caused by driving too fast in turns.
This analysis has practical applications in vehicle design, road design, and driver education to improve safety.
Transcripts
Browse More Related Video

Physics 15 Torque (10 of 27) When Will A Car Tip Over?

AP Physics Workbook 3.F Horizontal Circles

Banked Curve Physics - Uniform Circular Motion
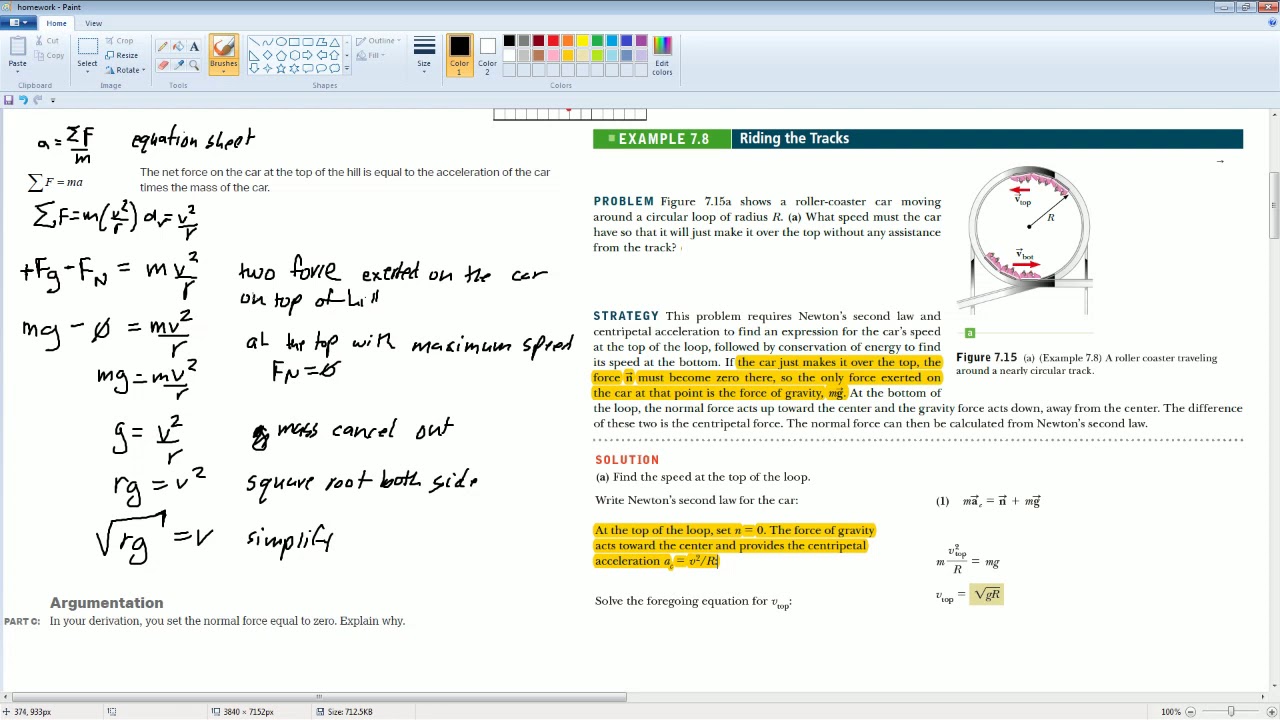
AP Physics Workbook 3.E Maximum speed over the top
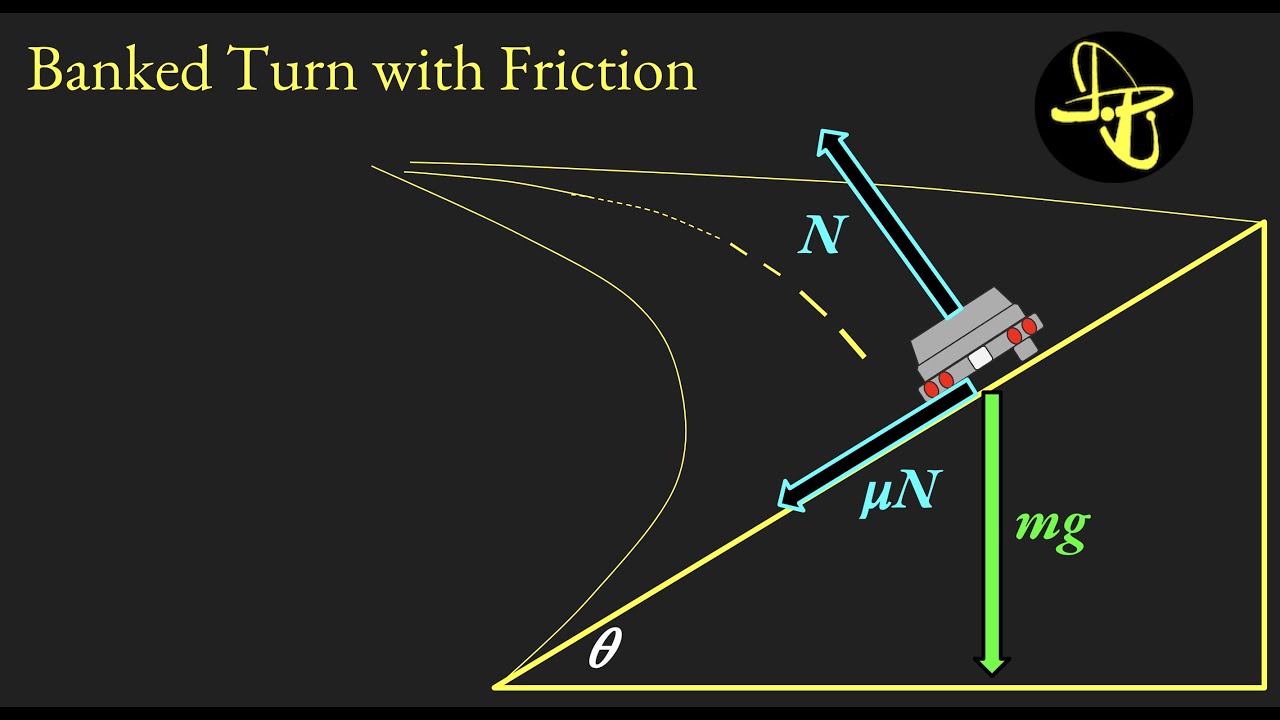
Banked Turn with Friction - Physics of Speed Limits on Banked Curves

banked curves and circular motion explained
5.0 / 5 (0 votes)
Thanks for rating: