Calculating Torque
TLDRIn this informative video, the presenter teaches viewers three distinct methods to calculate torque, a vector quantity that measures the rotational force applied to an object. The first method involves directly applying the formula for torque, which is the cross product of the force vector and the distance vector from the pivot. The second method breaks down the force vector into parallel and perpendicular components, focusing only on the perpendicular component that contributes to the torque. The third method simplifies the calculation by considering the sine of the angle between the force and the distance as the shortest distance from the pivot to the line of action of the force. The presenter uses a numerical example with a force of 20 Newtons, a distance of three meters, and an angle of 30 degrees to demonstrate each method, resulting in a torque of 30 Newton meters. The video concludes with a reminder to consider the direction of the torque, which is positive for counterclockwise rotation and negative for clockwise rotation, and encourages viewers to ask questions and engage with the content.
Takeaways
- 📐 **Definition of Torque**: Torque is a vector quantity defined as the cross product of the radius vector (R) and the force vector (F), often simplified to the magnitude of R times the magnitude of F times the sine of the angle (theta) between them.
- ⚙️ **Direction of Torque**: The direction of torque is determined by the force's tendency to rotate the system. Counterclockwise rotation is considered positive, while clockwise is negative, reminding us that torque is a vector quantity.
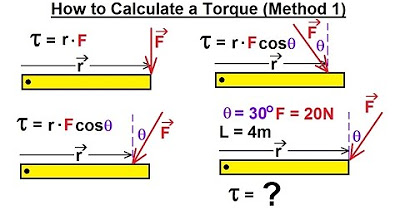
- 🔢 **Direct Calculation Method**: The first method calculates torque directly by applying the formula \( \text{Torque} = R \times F \times \sin(\theta) \), which gives the magnitude of the torque.
- 📏 **Component Analysis Method**: The second method involves breaking down the force vector into components parallel and perpendicular to the radius vector. Only the perpendicular component contributes to the torque.
- 🔄 **Perpendicular Component Importance**: The parallel component of force does not contribute to torque, as it only tends to stretch the object and does not cause rotation.
- 📐 **Line of Action Concept**: The third method involves considering the line of action of the force, where the sine of the angle (theta) is the closest distance from the pivot to this line of action.
- 🔢 **Simplified Torque Calculation**: In the third method, the torque can be calculated using the full magnitude of the force and the closest distance from the pivot to the line of action.
- 📈 **Numerical Example**: A numerical example is provided using a force of 20 Newtons, a distance to the pivot of 3 meters, and an angle of 30 degrees, resulting in a torque of 30 Newton meters.
- ⚖️ **Magnitude and Direction**: Torque calculation involves determining both the magnitude (using the formula) and the direction (based on the force's tendency to cause rotation, either clockwise or counterclockwise).
- 🧮 **Multiple Methods for Calculation**: Three different methods are presented for calculating torque, each providing the same result, demonstrating the versatility in approach to the problem.
- 📚 **Educational Content**: The script is educational, aiming to clarify the concept of torque for students in algebra-based physics or high school classes.
- ✅ **Confirmation of Understanding**: The script confirms understanding by showing that all three methods yield the same result, reinforcing the concept that torque is independent of the method of calculation.
Q & A
What is torque and how is it defined in terms of force and distance?
-Torque is a vector quantity that describes the rotational force acting on an object. It is defined as the cross product of the distance vector (R) from the pivot point and the force vector (F), which can be mathematically represented as τ = R x F. If only the magnitude is needed, it is calculated as the magnitude of R times the magnitude of F times the sine of the angle (θ) between the two vectors.
What is the significance of the angle θ in the calculation of torque?
-The angle θ is the angle between the force vector (F) and the distance vector (R). It is crucial in determining the magnitude of the torque because the sine of this angle is multiplied by the magnitudes of R and F. The torque's direction is affected by whether the force tends to rotate the object counterclockwise (positive torque) or clockwise (negative torque).
How does the direction of the force affect the sign of the torque?
-The direction of the force in relation to the rotation it induces determines the sign of the torque. If the force tends to cause counterclockwise rotation, it is considered a positive torque. Conversely, if the force causes clockwise rotation, it is a negative torque. This convention helps to remember that torque is a vector quantity with a direction.
What is the direct method for calculating torque as described in the script?
-The direct method involves applying the formula for torque directly using the given values. For a force F, distance R, and angle θ, the torque is calculated as τ = R * F * sin(θ). This method is straightforward and requires substituting the given values into the formula.
How can you calculate the torque using the components of the force vector?
-The torque can be calculated by breaking down the force vector (F) into two components: one parallel to the distance vector (R) and one perpendicular. The total torque is the sum of the torques due to these components. The parallel component does not contribute to the torque, while the perpendicular component does, and it is calculated as τ = R * (F * sin(θ)) * sin(θ), where the second θ is the angle between R and the perpendicular component of F.
What is the third method for calculating torque mentioned in the script?
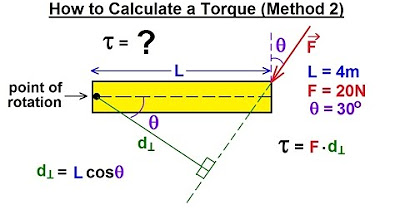
-The third method involves considering the line of action of the force and the closest distance from the pivot to this line. The torque is then calculated using the full magnitude of the force and this closest distance, which is essentially R * sin(θ), where θ is the angle between the force and the line of action. This method simplifies the calculation by directly using the sine of the angle as the distance from the pivot to the line of action.
Why is the parallel component of the force not considered when calculating torque?
-The parallel component of the force does not contribute to the rotation of the object; it only tends to stretch or compress the object along the direction of the force. Therefore, it does not produce any torque and is not considered in the calculation of torque.
How does the magnitude of the perpendicular component of the force affect the torque?
-The magnitude of the perpendicular component of the force, which is calculated as F * sin(θ), directly affects the torque. This component is the only part of the force that tends to rotate the object, and thus it is the primary contributor to the torque when the angle θ is not zero degrees.
What is the relationship between the line of action and the sine of the angle θ in the third method?
-In the third method, the sine of the angle θ is equivalent to the closest distance from the pivot to the line of action of the force. This relationship allows for a simplified calculation of torque using the formula τ = F * (closest distance from the pivot to the line of action).
How does the direction of the torque relate to the individual forces acting on the system?
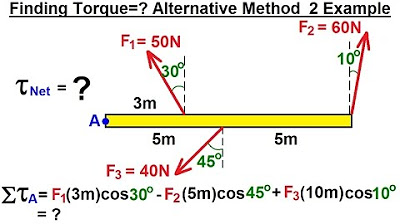
-The direction of the torque is determined by the direction in which each individual force tends to rotate the system. If a force tends to cause counterclockwise rotation, it is assigned a positive direction for torque. If it causes clockwise rotation, it is negative. The overall direction of the torque is the vector sum of the directions of the individual torques due to each force.
What is the practical significance of understanding the three methods for calculating torque?
-Understanding the three methods for calculating torque provides flexibility and insight into different scenarios where torque calculations are necessary. Each method can be more convenient depending on the available information and the specific system being analyzed, allowing for more efficient problem-solving in physics and engineering applications.
Outlines
📚 Understanding Torque Calculation Methods
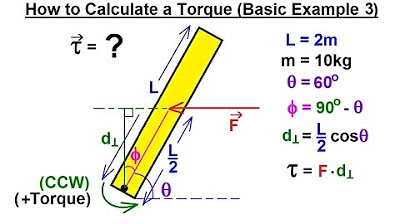
This paragraph introduces the concept of torque and its calculation methods. The speaker begins by defining torque as a vector quantity resulting from the cross product of force (F) and distance (R) from the pivot, including the sine of the angle (theta) between them. The direction of torque is determined by the force's tendency to rotate the system, with counterclockwise being positive and clockwise negative. Three methods for calculating torque are outlined: direct application of the formula, breaking down force into components (focusing on the perpendicular component), and using the closest distance from the pivot to the line of action of the force. A numerical example with a force of 20 Newtons, a distance of 3 meters, and an angle of 30 degrees is used to illustrate the methods.
📐 Calculating Torque Using Force Components
The second paragraph delves into the second method of calculating torque, which involves breaking down the force vector into parallel and perpendicular components relative to the vector R. The torque is the sum of the torques due to these components. It's emphasized that only the perpendicular component contributes to torque, as the parallel component does not cause rotation. Using the example from the first paragraph, the perpendicular component's torque is calculated, resulting in the same torque value as the direct method. This approach simplifies the calculation by focusing solely on the component of force that affects rotation.
🔍 Advanced Torque Calculation with the Line of Action
The third paragraph presents the third and more advanced method of calculating torque. It involves considering the line of action of the force and using the closest distance from the pivot to this line. The sine of the angle theta is replaced by this distance in the formula, allowing for the use of the full force magnitude in the calculation. The example provided is revisited, with the closest distance calculated as 1.5 meters, leading to the same torque value of 30 Newton meters as the previous methods. The direction of the torque is determined by the individual forces' tendencies to rotate the system.
Mindmap
Keywords
💡Torque
💡Pivot
💡Force
💡Radius Vector (R)
💡Cross Product
💡Magnitude
💡Angle Theta (θ)
💡Direction of Torque
💡Components of Force
💡Line of Action
💡Sine Function
Highlights
Torque is a vector quantity defined as the cross product of the position vector (R) and the force vector (F).
The magnitude of torque is calculated as the magnitude of R times the magnitude of F times the sine of the angle (theta) between the vectors.
The direction of torque is determined by the right-hand rule, with counterclockwise rotation being positive.
If multiple forces act on a system, the total torque is the vector sum of the torques due to each force.
A force can be broken down into parallel and perpendicular components relative to the position vector (R).
Only the perpendicular component of the force contributes to torque, while the parallel component does not cause rotation.
The torque due to a force can be calculated as the distance to the pivot times the perpendicular component of the force.
A numerical example is provided using a force of 20 Newtons, a distance to the pivot of 3 meters, and an angle of 30 degrees.
Method one for calculating torque involves directly applying the formula for torque.
Method two involves breaking down the force vector into components and calculating the torque due to each component.
Method three uses the concept of the line of action of the force, which is the hypotenuse of the triangle formed by the force vector and the position vector.
The closest distance from the pivot to the line of action of the force is used to calculate torque in method three.
Torque can be calculated using the full magnitude of the force and the closest distance from the pivot to the line of action.
The direction of torque is determined by the tendency of each individual force to rotate the object, either counterclockwise or clockwise.
The three methods provided offer different approaches to calculating torque, suitable for various algebra-based physics or high school classes.
The importance of understanding the vector nature of torque and its direction is emphasized for solving physics problems.
The video concludes with an invitation for viewers to ask questions, subscribe to the channel, and engage with the content.
Transcripts
Browse More Related Video
Physics 15 Torque Fundamentals (4 of 13) How to Calculate a Torque (Method 1)
Physics 15 Torque Fundamentals (5 of 13) How to Calculate a Torque (Method 2)
Physics 15 Torque Fundamentals (9 of 13) How to Calculate a Torque (Basic Example 3)
Physics 15 Torque (9 of 27) Alternative Method 1 & 2: Example Torque=?
Physics 15 Torque (7 of 27) Couple: Alternative Method 1
Physics 15 Torque Fundamentals (8 of 13) How to Calculate a Torque (Basic Example 2)
5.0 / 5 (0 votes)
Thanks for rating: