Physics 15 Torque (7 of 27) Couple: Alternative Method 1
TLDRIn this video, the presenter explores two methods for calculating torque on a beam. The traditional method involves finding the perpendicular distance (D) from the line of action of the force to the pivot point, which can be expressed in terms of the beam's length (L) and the angle (Theta) between the force and the beam. Torque is then calculated as the force (F) times L times the sine of Theta. An alternative method focuses on the vertical component of the force (Fy), which contributes to the torque, while the horizontal component (Fx) does not. The torque can also be described as Fy times L, and since Fy is F times the cosine of the angle (Fe) between the force and the vertical, the formula becomes F times L times the cosine of Fe. The presenter demonstrates that both methods yield the same result and shows that the choice between them depends on which is more straightforward to apply in a given situation. The video concludes with a promise of more examples in future videos to illustrate the calculation of torque with multiple forces.
Takeaways
- 🔍 **Torque Calculation Method**: Traditionally, torque is calculated as the force multiplied by the perpendicular distance (L) from the force's line of action to the pivot point.
- ⚙️ **Positive Torque Convention**: A force that would cause a counterclockwise motion on a beam is considered to produce a positive torque.
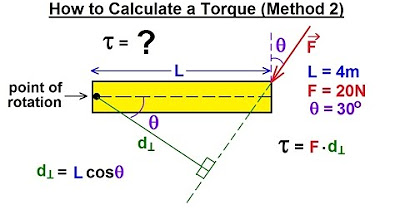
- 📐 **Right Triangle Application**: The perpendicular distance (D) can be found using trigonometry within a right-angled triangle formed by the beam's length (L) and the angle (Θ).
- 📈 **Trigonometric Expression**: Torque can be expressed as the force (F) times the length of the beam (L) times the sine of the angle (Θ), or F * L * sin(Θ).
- 🔄 **Component Contribution**: Only the vertical component of the force contributes to the torque, as the horizontal component's line of action passes through the pivot point.
- 📍 **Pivot Point Consideration**: The torque is the product of the vertical force component (F_y), which is F multiplied by the cosine of the angle (Fe), and the length of the beam (L).
- 🧮 **Cosine Function Utilization**: The torque can also be found by multiplying the force's magnitude by the cosine of the angle between the force and the vertical, then by the length of the beam (F * cos(Fe) * L).
- 🔗 **Angle Relationship**: The sine of the angle (Θ) is equivalent to the cosine of the complementary angle (Fe), hence sin(Θ) = cos(Fe).
- ✅ **Equivalence of Methods**: Both methods of calculating torque, using the perpendicular distance or the cosine of the angle, yield the same result.
- 🛠️ **Multiple Forces Consideration**: If there are multiple forces acting on a beam, each force can be individually multiplied by the cosine of the angle between its direction and the vertical to find their torque contributions.
- 📚 **Practical Application**: Depending on the situation, it may be more practical to use the cosine of the angle method rather than calculating the perpendicular distance directly.
Q & A
What is the traditional method of finding torque about a point?
-The traditional method involves calculating the torque as the product of the force acting on a beam, the distance from the force's point of application to the pivot point (L), and the sine of the angle (Theta) between the force and the perpendicular to the beam.
Why is the torque considered positive when the force causes a counterclockwise motion?
-The convention in physics is that a force causing a counterclockwise rotation results in a positive torque, while a force causing a clockwise rotation results in a negative torque.
What is the perpendicular distance (D) in terms of the length of the beam (L) and the angle (Theta)?
-The perpendicular distance (D) can be found using the right triangle formed by the force vector and the beam. It is equal to the length of the beam (L) times the sine of the angle (Theta), or D = L * sin(Theta).
How does one find the vertical component of the force (Fy) that contributes to the torque?
-The vertical component of the force (Fy) is found by taking the horizontal force (F) and multiplying it by the cosine of the angle (Fe) between the force and the vertical direction, so Fy = F * cos(Fe).
Why does the horizontal component of the force (Fx) not contribute to the torque?
-The horizontal component (Fx) does not contribute to the torque because its line of action passes through the pivot point, resulting in no moment arm and thus no torque being generated around that point.
What is an alternative method to calculate torque without finding the perpendicular distance D?
-An alternative method is to calculate the torque as the product of the force's vertical component (Fy), the cosine of the angle between the force and the vertical direction, and the length of the beam (L).
How is the sine of the angle (Theta) related to the cosine of the angle (Fe)?
-The sine of the angle (Theta) is equal to the cosine of the complementary angle (Fe), which means sin(Theta) = cos(90° - Theta) or sin(Theta) = cos(Fe), given that Theta + Fe = 90°.
What is the formula for torque using the cosine of the angle between the force and the beam?
-The formula for torque using the cosine of the angle is Torque = F * L * cos(Fe), where F is the magnitude of the force, L is the length of the beam, and Fe is the angle between the force and the vertical.
When is it more convenient to use the cosine of the angle method to find torque?
-It is more convenient to use the cosine of the angle method when finding the perpendicular distance (D) is difficult or when dealing with multiple forces where calculating the angle between each force and the vertical is simpler.
How can you generalize the torque calculation for multiple forces acting on a beam?
-For multiple forces, you can calculate the torque for each force separately by multiplying the force by the cosine of the angle between its direction and the vertical to the beam, and then summing all the individual torques to get the total torque.
Why is it important to consider the direction of the force when calculating torque?
-The direction of the force is crucial because torque is a vector quantity that depends on both the magnitude and the direction of the force relative to the pivot point. The direction determines whether the torque will cause a clockwise or counterclockwise rotation.
What is the significance of the right triangle formed by the force vector and the beam in torque calculations?
-The right triangle allows for the application of trigonometric functions to find the perpendicular distance (D) from the force's line of action to the pivot point, which is essential for calculating the torque using the traditional method.
Outlines
🔧 Exploring Torque Calculation Methods
This paragraph introduces two methods for calculating torque on a beam. The traditional method involves finding the perpendicular distance (d) from the force's line of action to the pivot point (point A) and using the formula torque = force * d. The alternative method focuses on the vertical component of the force (Fy), which contributes to the torque, and is calculated using the formula torque = Fy * L, where L is the length of the beam. The paragraph explains that the sine of the angle (theta) between the force and the beam can be used in the first method, while the cosine of the angle (Fe) between the force and the vertical is used in the second method. Both methods yield the same result, offering flexibility in torque calculation depending on the available information.
📐 Comparing Torque Calculation Techniques
The second paragraph emphasizes the practicality of choosing the appropriate method for calculating torque based on the ease of finding the required components. It suggests that sometimes calculating the perpendicular distance can be difficult, whereas using the force's vertical component and the cosine of the angle between the force and the vertical to the beam might be simpler. The paragraph assures that this alternative method will be further illustrated through additional examples in upcoming videos, highlighting the flexibility and application of torque calculation methods in different scenarios.
Mindmap
Keywords
💡Torque
💡Beam
💡Force
💡Point of Rotation
💡Perpendicular Distance
💡Right Triangle
💡Hypotenuse
💡Angle Theta
💡Component of Force
💡Cosine of the Angle
💡Counterclockwise Motion
Highlights
Exploring a different method of finding torque, which is actually the traditional method in disguise.
Torque is traditionally calculated as the force acting on a beam at a distance, multiplied by the perpendicular distance from the force's line of action to the point of rotation.
The perpendicular distance (D) can be found in terms of the beam's length (L) and the angle (Theta), using trigonometric relationships.
Torque can also be calculated using the vertical component of the force (Fy) and the length of the beam (L), disregarding the horizontal component (Fx) which does not contribute to torque.
The vertical component of the force (Fy) is found by multiplying the total force (F) by the cosine of the angle (Fe) between the force and the vertical.
An alternative method to find torque is by multiplying the force's magnitude, the cosine of the angle between the force and the vertical, and the length of the beam.
The relationship between the sine of the angle Theta and the cosine of the angle Fe is established, showing that they are equivalent for calculating torque.
Two expressions for torque are shown to be equivalent: one using the perpendicular distance D and the sine of the angle, and the other using the cosine of the angle Fe and the beam's length L.
When multiple forces are acting on a beam, each force can be individually multiplied by the cosine of the angle between its direction and the vertical to find the total torque.
The choice between using the perpendicular distance or the cosine of the angle depends on which is easier to calculate in a given scenario.
The video demonstrates the flexibility in calculating torque, offering two different but equally valid methods.
Understanding the trigonometric relationships between force components and angles is crucial for accurately calculating torque.
The video emphasizes the importance of considering the direction of force components and their impact on torque calculation.
Practical applications of torque calculation are shown through the analysis of forces acting on a beam.
The video concludes with a promise to present more examples in upcoming videos, reinforcing the practical understanding of torque calculation.
The convention of positive torque causing a counterclockwise motion of the beam is introduced and used throughout the video.
The video provides a clear explanation of why the horizontal component of force does not contribute to torque when the line of action passes through the pivot point.
The mathematical derivation of torque using trigonometric functions is detailed, offering a deeper understanding of the underlying principles.
Transcripts
Browse More Related Video
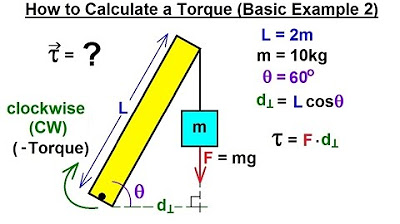
Physics 15 Torque Fundamentals (8 of 13) How to Calculate a Torque (Basic Example 2)
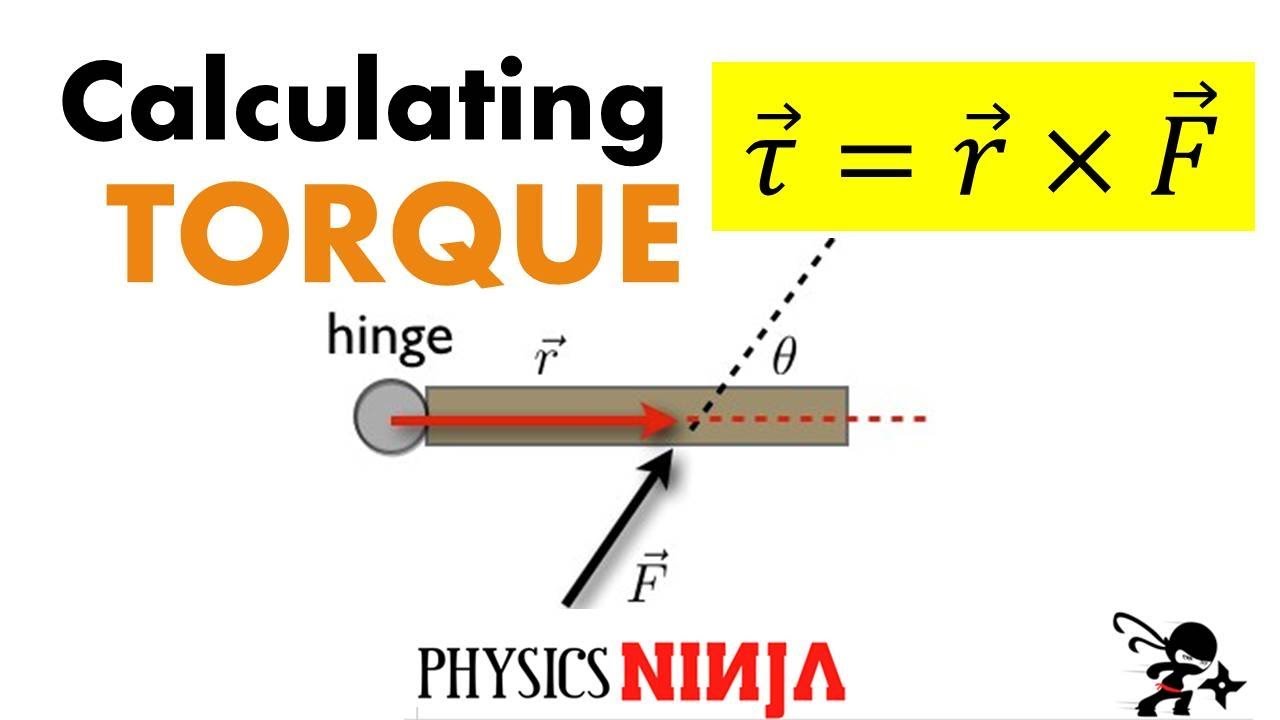
Calculating Torque
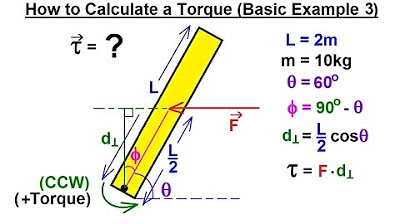
Physics 15 Torque Fundamentals (9 of 13) How to Calculate a Torque (Basic Example 3)
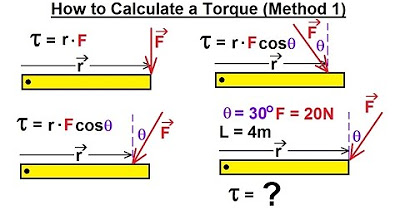
Physics 15 Torque Fundamentals (4 of 13) How to Calculate a Torque (Method 1)

Torque = Force Times Lever Arm | Physics with Professor Matt Anderson | M12-06
Physics 15 Torque Fundamentals (5 of 13) How to Calculate a Torque (Method 2)
5.0 / 5 (0 votes)
Thanks for rating: