General Relativity Lecture 9
TLDRThe transcript appears to be a detailed lecture on Einstein's field equations, a set of equations that describe the fundamental interaction of gravitation as a result of spacetime being curved by mass and energy. The lecturer emphasizes the complexity of these equations and their computational intensity, suggesting that solving them typically requires computational tools like Mathematica. The discussion covers the basic principles of general relativity, the comparison with Newtonian concepts, and the transition to the geometrical understanding of gravity in the context of general relativity. The lecturer also touches upon the Schwarzschild geometry, the Ricci tensor, and the significance of the energy-momentum tensor in the field equations. The script delves into the mathematical formulations, including the Christoffel symbols and the curvature tensor, leading up to the formulation of Einstein's field equations. It concludes with the implications of these equations in the absence of a source, such as in the case of gravitational waves or the Schwarzschild solution, and the potential inclusion of a cosmological constant.
Takeaways
- 📘 Einstein's field equations are complex and computationally intensive, often requiring computer assistance for solutions.
- 📚 General relativity simplifies the principles of gravity but introduces computational challenges compared to Newtonian physics.
- 🔗 Newton's concept of gravity involves a two-way interaction between masses and the gravitational field, which is also a feature in general relativity.
- 🌌 The Schwarzschild geometry, a solution to Einstein's equations, describes the spacetime geometry outside a spherical mass and is analogous to Newtonian gravity outside a massive object.
- ⚖️ The Ricci tensor, derived from the Riemann curvature tensor, is a key component in formulating Einstein's field equations, representing the curvature of spacetime.
- 🔍 The Einstein tensor, formed by combining the Ricci tensor and the curvature scalar, is used on the left-hand side of Einstein's field equations, linking geometry and the distribution of matter/energy.
- 🤔 The energy-momentum tensor is a symmetric tensor that describes the density and flow of energy and momentum in spacetime, which is used on the right-hand side of Einstein's equations.
- 📉 In the absence of sources (vacuum), the Ricci tensor components can be zero, leading to solutions like gravitational waves and the Schwarzschild metric, which describe non-trivial geometries.
- 🌟 The cosmological constant can be treated as part of the energy-momentum tensor or as a modification to the spacetime geometry, reflecting its role in the dynamics of the universe.
- ➿ The trace of the energy-momentum tensor, when zero, simplifies Einstein's field equations and is relevant for massless particles like photons or gravitons.
- 🧮 The Einstein field equations can be manipulated to express the curvature scalar in terms of the energy-momentum tensor, highlighting the interplay between geometry and matter/energy distribution.
Q & A
Why are Einstein's field equations considered computationally intensive?
-Einstein's field equations are computationally intensive because they involve a large number of symbols and derivatives, making them complex to solve and challenging to write down explicitly on a single piece of paper. The equations also become complicated quickly when trying to solve them in various circumstances.
What is the fundamental principle behind the 'two-way street' concept in the context of Newton's theory of gravity?
-The 'two-way street' concept in Newton's theory of gravity refers to the mutual interaction between the gravitational field and masses. Masses affect the gravitational field, and in turn, the gravitational field influences the motion of masses. This is a foundational aspect of how gravity operates in Newtonian physics.
How is the gravitational potential (Phi) related to the force experienced by a particle in Newtonian mechanics?
-In Newtonian mechanics, the force experienced by a particle due to gravity is given by the gradient of the gravitational potential (Phi). Specifically, the force on a particle is equal to the negative mass of the particle times the gradient of Phi. This relationship is derived from the equation where the gravitational force is written as minus M times the gradient of Phi.
What is the significance of the Poisson equation in the context of Newtonian gravity?
-The Poisson equation is significant in Newtonian gravity as it describes how the distribution of mass in space determines the gravitational potential. It is a scalar equation that relates the second partial derivatives of the gravitational potential to the mass density, which is a source of the gravitational field.
What is a key difference between the energy momentum tensor and the four-vector of charge density and current?
-The energy momentum tensor is a rank-2 tensor that includes components for energy density and momentum density, as well as their corresponding flows. It is a more complex object that encompasses both energy and momentum aspects of a system. In contrast, the four-vector of charge density and current describes the density and flow of electric charge, which is a simpler concept and involves only the charge density and the current associated with the charge.
Why is it important for physical laws to be expressed as tensor equations in the context of relativity?
-It is important for physical laws to be expressed as tensor equations in the context of relativity because tensors are objects that transform in a specific way under changes of coordinates, which allows these laws to be true in all frames of reference. This is crucial for the theory of relativity, which relies on the principle that the laws of physics should hold in all inertial frames.
What is the Schwarzschild geometry, and how is it related to Newtonian gravity?
-The Schwarzschild geometry is a solution to Einstein's field equations that describes the spacetime geometry outside a spherically symmetric, non-rotating mass. It is related to Newtonian gravity because it reduces to Newton's law of gravity in the weak-field limit, where the effects of general relativity are negligible, and the gravitational field is weak.
What is the role of the Ricci tensor in Einstein's field equations?
-The Ricci tensor, denoted as R_{μν}, is a part of the Einstein tensor G_{μν}, which is the left-hand side of Einstein's field equations. It is derived from the metric tensor and its first and second derivatives. The Ricci tensor, along with the curvature scalar, is used to formulate the geometric side of the equations, which are then set equal to the energy momentum tensor on the right-hand side.
Why is the Einstein tensor (G_{μν}) considered to be covariantly conserved?
-The Einstein tensor is considered to be covariantly conserved because its covariant derivative with respect to any index is zero, i.e., D_{μ}G^{μν} = 0. This property ensures that the tensor satisfies the requirement of local conservation of energy and momentum, which is a fundamental principle in physics.
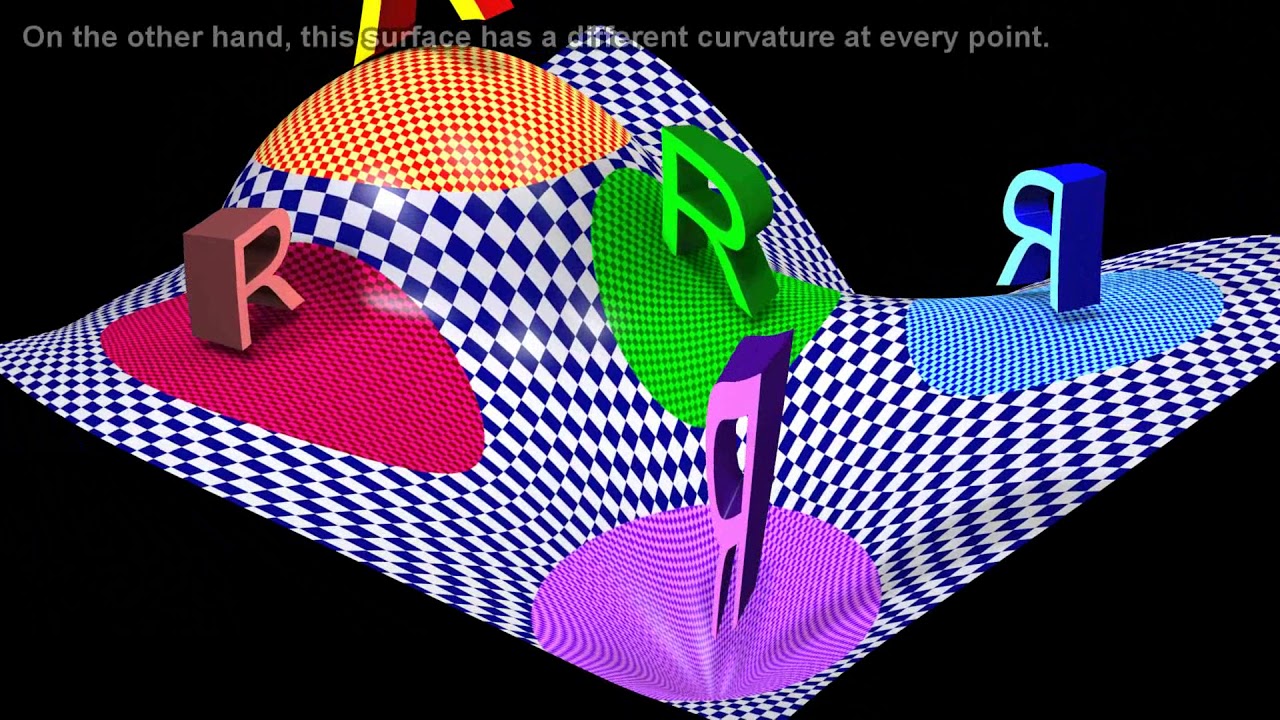
What is the physical interpretation of the Ricci scalar (R) in the context of general relativity?
-The Ricci scalar, denoted as R, is a single number that is derived from the Ricci tensor by contracting its indices. It represents the curvature of spacetime and can be thought of as a measure of the overall 'density' of the gravitational field. When the Ricci scalar is zero, it implies that the spacetime is locally flat, but this does not necessarily mean that the spacetime is globally flat or without curvature.
How do gravitational waves fit into the framework of Einstein's field equations?
-Gravitational waves are solutions to Einstein's field equations that describe ripples in spacetime caused by accelerating masses. They are represented by a Ricci tensor that is zero (R_{μν} = 0), indicating that the waves do not have a static source of energy and momentum. Gravitational waves carry energy and momentum as they propagate through spacetime, and their existence was a major prediction of general relativity.
Outlines
😀 Introduction to Einstein's Field Equations and Newtonian Concepts
The paragraph introduces the complexity of Einstein's field equations, contrasting them with the simplicity of the principles of general relativity. It discusses the computational challenges in solving these equations and the use of computer software like Mathematica. It also sets the stage for a discussion on Newton's version of these field equations, emphasizing the two-way relationship between masses and the gravitational field, and how this concept is expressed mathematically.
📚 Newton's Field Equations and their Relation to Einstein's Theory
This section delves into Newton's field equations, explaining how the gravitational force on a particle is represented and how the gravitational potential affects the movement of masses. It further explores Poisson's equation, which describes the gravitational field caused by mass distributions. The paragraph also touches on the solution to this equation for a spherically symmetric object, like a star or a planet, and how it relates to Newton's constant and mass density.
🌌 Transitioning from Newtonian to Relativistic Gravitation
The paragraph discusses the transition from Newtonian gravitation, which is action at a distance, to the field theory approach of general relativity. It highlights the Schwarzschild geometry as a solution to Einstein's equations, drawing parallels between the Newtonian potential and the time-time component of the metric. The concept of mass affecting geometry is introduced as a preliminary to understanding Einstein's field equations.
🚀 The Role of Energy Density in General Relativity
This part emphasizes the importance of energy density in the context of general relativity. It explains that energy density, as part of a tensor, replaces the mass density on the right-hand side of the field equations. The paragraph also discusses the equivalence of mass and energy, and how energy density is a more complex concept that includes more than just the energy density itself.
🔋 Conservation of Charge and the Four-Vector
The paragraph explores the concept of electric charge and its conservation, introducing the charge density and current. It explains how these quantities form a four-vector in the context of special relativity. The continuity equation for charge is derived, illustrating how charge conservation is a local phenomenon, requiring charge to flow through the boundaries of a region.
⚙️ Energy, Momentum, and their Conservation in Relativity
This section discusses the conservation of energy and momentum, highlighting the differences between these quantities and electric charge. It explains that energy and momentum are not invariant and form components of a four-vector, the energy-momentum tensor. The paragraph also touches on the concept of total energy and momentum not being invariant, unlike charge.
🌟 The Schwarzschild Metric and its Significance in Vacuum Solutions
The paragraph focuses on the Schwarzschild metric, which is a solution to Einstein's field equations that describes the geometry of spacetime outside a spherical mass. It is highlighted that the Schwarzschild metric is Ricci flat, meaning that the Ricci tensor Rmu-nu is zero everywhere outside the singularity, which is a significant property in the context of vacuum solutions.
📏 The Einstein Tensor and its Components
This section introduces the Einstein tensor, which is used on the left-hand side of Einstein's field equations. The paragraph explains the properties of the Einstein tensor, including its symmetry and how it is derived from the Ricci tensor and the curvature scalar. The discussion also includes the trace of the energy-momentum tensor and its implications for the field equations.
🤔 The Physical Meaning of the Ricci Scalar, Tensor, and Riemann Tensor
The paragraph explores the physical meaning behind the Ricci scalar, the Ricci tensor, and the Riemann tensor. It acknowledges the complexity of visualizing these tensors and their individual meanings, especially the Riemann tensor. However, it points out that the Ricci scalar can be zero even when the Riemann tensor is not, as in the case of gravitational waves, indicating that the Ricci scalar contains less information than the full curvature tensor.
🌌 The Impact of the Cosmological Constant on Einstein's Field Equations
This section discusses the cosmological constant, which can be thought of as part of the energy-momentum tensor or as a component of the geometry in Einstein's field equations. The paragraph explains that the cosmological constant can be moved to either side of the equation, affecting the interpretation of the equation in terms of geometry or energy-momentum, respectively.
📐 Historical Calculation of Mercury's Orbit Using Einstein's Theory
The final paragraph touches on the historical use of Einstein's theory to calculate the orbit of Mercury, which had been observed by astronomers. It is mentioned that Einstein likely used an approximation of the Schwarzschild solution to account for the small corrections to Newtonian mechanics and that the exact solution by Schwarzschild came shortly after, allowing for more precise calculations.
Mindmap
Keywords
💡Einstein's Field Equations
💡General Relativity
💡Curvature Tensor
💡Christoffel Symbols
💡Newtonian Mechanics
💡Schwarzschild Metric
💡Ricci Tensor
💡Energy-Momentum Tensor
💡Geodesic
💡Covariant Derivative
💡Cosmological Constant
Highlights
Einstein's field equations are computationally intensive and complex, often requiring computational tools like Mathematica to solve.
The principles of general relativity are simple, but applying them beyond basic concepts becomes complicated quickly.
Newton's concept of gravity is a two-way street where masses affect the gravitational field, and the field affects how masses move.
The gravitational force on a particle can be described as the gradient of the gravitational potential, which is a function of position.
Poisson's equation in Newtonian gravity relates the gravitational potential to the distribution of masses causing the field.
In the case of a spherically symmetric object, the gravitational potential outside the mass can be solved uniquely.
The Schwarzschild geometry is a solution to Einstein's equations, particularly relevant for massive objects like stars.
Einstein's field equations are more complex than Newton's, involving the energy-momentum tensor and the geometry of space-time.
The geodesic rule in general relativity states that particles move along space-time geodesics once the geometry is known.
Energy density in relativity is part of a tensor, which includes more than just energy density, representing a complex set of quantities.
The conservation of charge is a local idea, meaning charge cannot disappear or appear within a region without a current flowing through its boundaries.
The energy-momentum tensor is a rank-two tensor that combines the flow and density of energy and momentum, satisfying a continuity equation.
The Einstein tensor, formed from the Ricci tensor and the curvature scalar, is covariantly conserved and satisfies the continuity equation for energy momentum.
Einstein's field equations can be simplified in the vacuum case, where the energy-momentum tensor is zero, leading to Rμν = 0.
The Schwarzschild metric is an example of a solution to the vacuum Einstein equations, representing the space-time geometry outside a massive object.
Gravitational waves are solutions to Einstein's equations that represent ripples in space-time, propagating without the need for a source.
The cosmological constant can be thought of as part of the energy-momentum tensor or as a contribution to the geometry in Einstein's field equations.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: