Confidence Interval and Interpretation for the Percentage of Yellow Peas with StatCrunch

The Math Sorcerer
11 Sept 201806:31
EducationalLearning
32 Likes 10 Comments

TLDRThe transcript details a statistical experiment involving peas, where the goal is to construct a 95% confidence interval to estimate the percentage of yellow peas in a sample. The total number of observations (peas) is 592, with 168 being yellow. Using statistical software like StatCrunch, the process involves inputting the number of successes (yellow peas) and the total observations to calculate the confidence interval. The result is a range that, with 95% confidence, should contain the true population percentage of yellow peas. The calculated interval is between 24.7% and 32%, which includes the expected 25%, indicating no contradiction to the initial hypothesis. The explanation also emphasizes the practical significance of confidence intervals in estimating population proportions based on sample data.

Takeaways
  • 🌱 The genetic experiment involved peas, with the offspring consisting of 424 green and 168 yellow peas.
  • 📊 A 95% confidence interval was constructed to estimate the percentage of yellow peas, indicating a range of values within which the true percentage lies.
  • 🔢 To calculate the confidence interval, you need the total number of observations (n) and the number of successes (X), which in this case is the total number of yellow peas.
  • 🧮 The total number of observations (n) is the sum of green and yellow peas, which is 424 + 168 = 592 (not 590 as mentioned in the transcript).
  • ✅ The number of successes (X) is the total number of yellow peas, which is 168.
  • 📐 The confidence interval was calculated using statistical software, with the default confidence level set at 95%.
  • 📉 The lower and upper limits of the confidence interval were provided by the software, with the result rounded to three decimal places.
  • 💯 The 95% confidence level implies that if the experiment were repeated 100 times, 95 of those confidence intervals would contain the true percentage of yellow peas.
  • 🔑 The transcript incorrectly states the total number of observations as 590, but the correct calculation is 592.
  • 🚫 The initial expectation was that 25% of the peas would be yellow, which is within the calculated confidence interval, indicating no contradiction.
  • ✋ The manual interpretation of the confidence interval involves stating the confidence level and describing the population parameter, which is the percentage of yellow peas.
  • 📝 The confidence interval provides a range within which the true population percentage of yellow peas is believed to lie, with a 95% level of confidence.
Q & A

  • What is a confidence interval?

    -A confidence interval is a range of values that is likely to contain the true value of an unknown population parameter with a certain level of confidence. It is used to estimate the population parameter based on a sample.

  • What does a 95% confidence level mean in the context of the script?

    -A 95% confidence level implies that if the experiment were repeated 100 times with different samples, the calculated confidence interval would contain the true population percentage in 95 out of those 100 instances.

  • What are the two key components needed to construct a confidence interval for a percentage or proportion?

    -The two key components needed are: n, which is the total number of observations (in this case, the total number of peas), and X, which is the total number of successes (the number of yellow peas).

  • How do you calculate the total number of observations (n) in the given script?

    -The total number of observations (n) is calculated by adding the number of green peas to the number of yellow peas, which in the script is 424 (green peas) + 168 (yellow peas) = 592.

  • What is the total number of successes (X) in the context of the script?

    -In the context of the script, the total number of successes (X) is the number of yellow peas, which is 168.

  • How does the script use software to calculate the confidence interval?

    -The script uses software called StatCrunch to calculate the confidence interval. It inputs the total number of observations (n) and the number of successes (X), selects the confidence level (95%), and then computes the interval.

  • What does the lower and upper limit of the confidence interval represent?

    -The lower and upper limits of the confidence interval represent the range within which the true population percentage of yellow peas is expected to lie with a certain level of confidence (95% in this case).

  • How is the percentage of yellow peas in the sample related to the expected percentage in the script?

    -The script mentions that it was expected that 25% of the peas would be yellow. Since the confidence interval includes 25%, it suggests that the observed data does not contradict the expectation.

  • How would you interpret the confidence interval manually?

    -To interpret the confidence interval manually, you would state the confidence level (e.g., 95%) and then describe that the population percentage of yellow peas is between the lower and upper limits of the interval. This indicates that you can be 95% confident that the true percentage of yellow peas in the entire population falls within this range.

  • Why is it important to calculate a confidence interval even if the exact number of yellow peas in the sample is known?

    -Calculating a confidence interval is important because it provides an estimate of the population parameter (the percentage of yellow peas) that accounts for sampling variability. It gives a range that is likely to contain the true population percentage, which is useful for making inferences about the entire population based on sample data.

  • What is the significance of the confidence interval including the expected percentage of yellow peas?

    -If the confidence interval includes the expected percentage of yellow peas, it suggests that the observed sample data is consistent with the expectation. It means that the true population percentage could reasonably be equal to the expected value, given the confidence level.

  • How would you express the confidence interval in a written format?

    -The confidence interval should be expressed in a written format as a range with the lower limit first, followed by the upper limit, both enclosed in parentheses. For example, if the interval is from 24.7% to 32%, it would be written as (24.7%, 32%).

Outlines
00:00
🌱 Constructing a Confidence Interval for Peas Experiment

This paragraph outlines the process of creating a 95% confidence interval to estimate the percentage of yellow peas in a genetic experiment. The experiment involved peas, with 424 green and 168 yellow peas observed in one sample. The key to constructing this interval is understanding that it represents a range of values within which the true percentage of yellow peas lies, with a 95% level of confidence. The total number of observations (n) is the sum of green and yellow peas, which is 592 in this case. The total number of successes (X), which represents the yellow peas, is 168. Using statistical software like StatCrunch, one can input these values to obtain the lower and upper limits of the confidence interval. The interval is then written with three decimal places and is interpreted to mean that if the experiment were repeated many times, 95% of the resulting intervals would contain the true percentage of yellow peas. The interval does not contradict the expectation that 25% of the peas would be yellow, as this value falls within the calculated interval.

05:01
📊 Interpreting the Confidence Interval Manually

The second paragraph delves into how to interpret the confidence interval for the percentage of yellow peas manually. It emphasizes the importance of the confidence level, which in this case is 95%, and explains that this level implies that if the experiment were repeated 100 times, the true population percentage would fall within the calculated interval in 95 out of those instances. The paragraph also clarifies that the interval provides an estimate for the population percentage of yellow peas, which cannot be known with absolute certainty. By converting the decimal values of the interval into percentages (24.7% and 32%), the interpretation becomes more accessible. The key takeaway is that the confidence interval gives a range within which the true population percentage is likely to fall, based on the sample data and the chosen confidence level.

Mindmap
Keywords
💡Genetic Experiment
A genetic experiment is a scientific procedure that involves the manipulation and study of the genetic material of organisms, typically to understand how genes function and interact. In the video, a genetic experiment with peas is conducted to observe the inheritance of color traits, resulting in offspring with either green or yellow peas.
💡Peas
Peas are plants often used in genetic studies due to their simple genetic traits and ease of cultivation. In the script, peas serve as the subject of the genetic experiment, where the color of the peas (green or yellow) is the trait being studied.
💡Confidence Interval
A confidence interval is a range of values, derived from a statistical model, that is likely to contain the value of an unknown parameter. It is used to estimate the true value of a population parameter with a certain level of confidence. In the video, a 95% confidence interval is constructed to estimate the percentage of yellow peas in the population.
💡Proportion
Proportion refers to the ratio of one quantity to another, often expressed as a percentage. In the context of the video, the proportion of yellow peas is the focus, as it represents the success rate or the frequency of the yellow trait in the pea offspring.
💡Successes
In the context of statistical experiments, 'successes' refers to the number of times an event of interest occurs. In the video, the successes are the yellow peas, which represent the instances where the desired genetic trait (yellow color) is observed.
💡Observations
Observations are the data points collected during an experiment. In the script, the total number of observations is the sum of green and yellow peas, which together form the dataset used to calculate the confidence interval.
💡Population Percentage
The population percentage refers to the proportion of a particular characteristic within an entire population. In the video, the goal is to estimate the population percentage of yellow peas, which is the percentage of all yellow peas in the entire pea population.
💡Sample
A sample is a subset of a population that is used to represent the whole for the purpose of research or analysis. In the video, the peas that were used in the genetic experiment represent a sample from which inferences are made about the larger population of peas.
💡Hypothesis Test
A hypothesis test is a statistical method used to evaluate whether a hypothesis about a population is likely to be true or false. While not the main focus of the video, it is mentioned as a future step in the analysis of the genetic experiment data.
💡Percentage
Percentage is a way of expressing a proportion or a fraction as a part of 100. It is used in the video to express the proportion of yellow peas in the sample and to estimate the proportion in the entire population of peas.
💡Statistical Software
Statistical software, such as StatCrunch mentioned in the video, is used to perform complex statistical analyses, including the calculation of confidence intervals. In the context of the video, the software is used to compute the 95% confidence interval for the proportion of yellow peas.
💡Interpretation
Interpretation in statistics involves understanding and explaining the results of an analysis. The video script discusses how to interpret the confidence interval manually, emphasizing the meaning of the 95% confidence level in the context of the genetic experiment.
Highlights

A genetic experiment with peas resulted in a sample of offspring consisting of 424 green and 168 yellow peas.

The goal is to construct a 95% confidence interval to estimate the percentage of yellow peas.

95% confidence means that if the experiment is repeated 100 times, 95 of those results would be correct.

To construct a confidence interval, you need the total number of observations (n) and the total number of successes (X).

For this experiment, n is the total number of peas (424 green + 168 yellow), and X is the number of yellow peas (168).

Using StatCrunch software, input the values for X (successes) and n (total observations) to calculate the confidence interval.

The confidence interval for the percentage of yellow peas is calculated to be between 24.7% and 32.0%.

The expected percentage of yellow peas was 25%, which is included within the calculated confidence interval.

The confidence interval indicates that the true percentage of yellow peas could easily be 25%.

Manual interpretation of the confidence interval involves stating the confidence level and describing the population percentage.

The population percentage of yellow peas is said to be between 24.7% and 32.0% with 95% confidence.

The confidence interval provides a range of values for the population percentage based on a sample of the population.

The experiment's results are used to estimate the population percentage of yellow peas, which is otherwise impossible to find.

The confidence interval is useful for understanding the variability in experimental results and provides an estimate for the population parameter.

The process of constructing a confidence interval is applicable for proportions or percentages in statistical analysis.

StatCrunch is a tool that simplifies the calculation of confidence intervals for proportions or percentages.

The experiment's findings can be generalized to the population with a certain level of confidence, which is crucial in scientific research.

Understanding the concept of confidence intervals is fundamental to interpreting statistical results accurately.

Transcripts

Browse More Related Video

Confidence Interval for a population proportion | Solved Problems

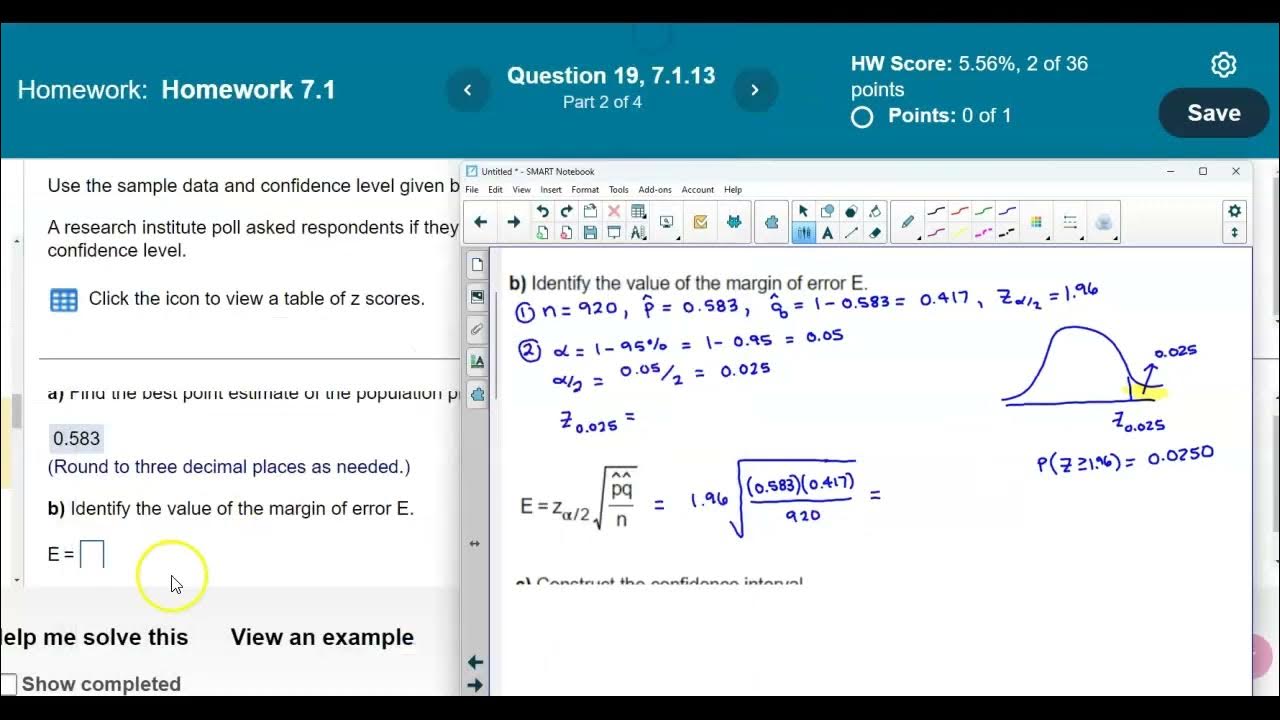
Math 14 7.1.13 Find the point estimate, margin of error & confidence interval of pop. proportion p.

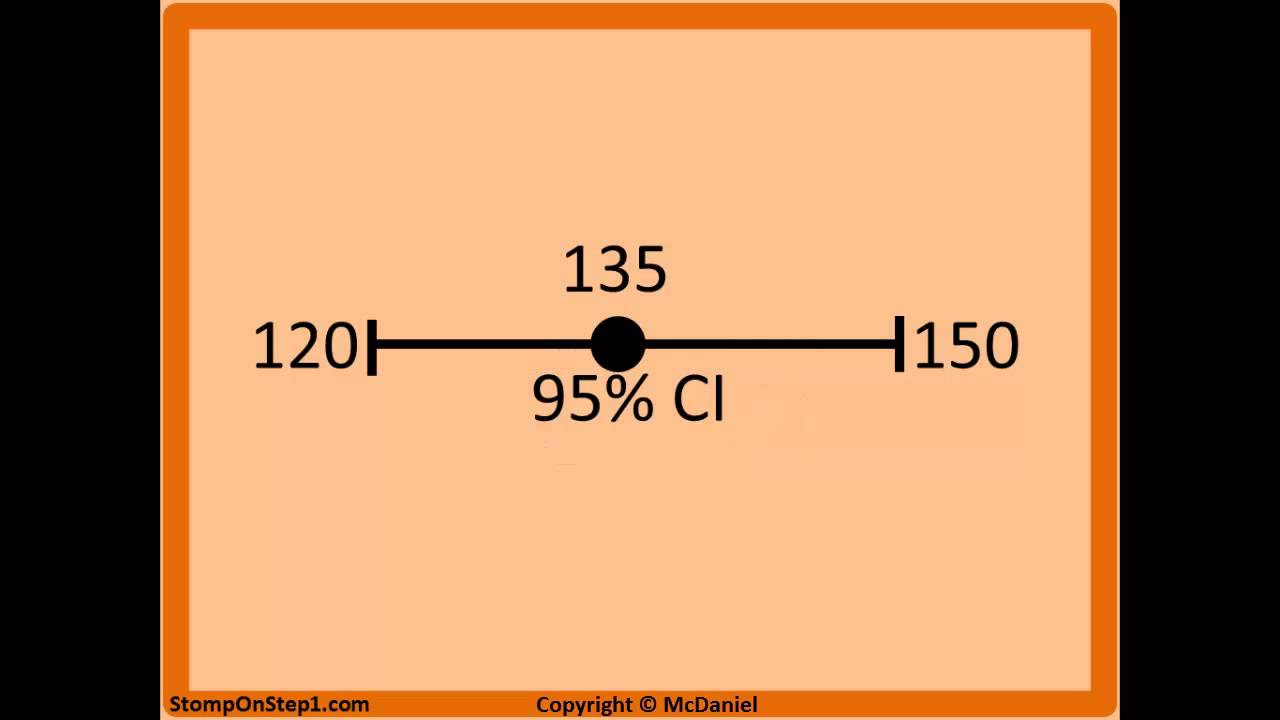
Confidence Interval Interpretation. 95% Confidence Interval 90% 99%

Confidence Intervals, Clearly Explained!!!

What is a Confidence Interval? | Puppet Master of Statistics

Confidence Interval [Simply explained]

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Genetic ExperimentPeas AnalysisConfidence IntervalStatistical MethodProportion EstimationYellow PeasGreen PeasStatistical SoftwarePopulation PercentageData InterpretationScience EducationResearch Methodology