Calculus -- The foundation of modern science
TLDRThe video script explores the significance of calculus in understanding the fundamental laws of the universe, which are expressed through mathematics. It explains how calculus reveals the beauty of nature and enables predictions in modern physics. The concepts of distance (Y) and time (X) are introduced, with the purple line illustrating their relationship. The script delves into the concept of speed, represented by green rectangles, and how the sum of their areas equals the distance traveled. It then transitions to scenarios involving variable speeds and negative speeds, represented by yellow rectangles, which can indicate movement in reverse. The fundamental theorem of calculus is introduced, which states that no matter the graph's complexity, the sum of rectangles equals the distance traveled. The script further explains derivatives as the rate of change, or slope, and integrals as the sum of all small rectangles' areas, representing the distance traveled over time. The integral of a function f(x) from zero to a specific value is shown to be equivalent to the total distance covered, encapsulating the essence of the fundamental theorem of calculus and its role as the language of the universe's laws.
Takeaways
- 📐 **Mathematics as the Language of the Universe**: The fundamental laws of the universe are expressed through mathematics, particularly calculus.
- 🎨 **Beauty of Nature Through Calculus**: Calculus is the tool that allows us to perceive the intrinsic beauty of natural phenomena.
- 🚀 **Foundation of Modern Physics**: Calculus is essential for making predictions in the field of modern physics.
- ⏱️ **Variables X and Y**: X represents time, while Y represents distance in space, illustrating the space-time relationship.
- 🔵 **Speed as Distance Over Time**: Speed is defined as the distance an object travels in one unit of time, visualized by green rectangles.
- 🟡 **Negative Speed and Backward Motion**: Yellow rectangles represent negative speed, indicating that an object is moving in the opposite direction.
- 🔶 **The Fundamental Theorem of Calculus**: Regardless of the graph's nature, the sum of the areas of rectangles (representing small increments of distance over time) equals the total distance traveled.
- 📉 **Derivative as Instantaneous Speed**: The derivative, represented as 'dy/dx', is the rate of change of distance with respect to time, or the slope of the tangent line to the curve at any point.
- 📈 **Integral as Accumulated Distance**: The integral of a function, denoted as ∫f(x)dx, represents the sum of all the infinitesimally small rectangles' areas under the curve, which corresponds to the total distance traveled.
- 🔺 **Graph Smoothness and Speed Changes**: A smooth graph indicates continuous speed changes, while sharp corners represent abrupt changes in speed.
- 🔴 **Calculus in Describing Universe's Laws**: Calculus provides the framework to formulate equations that describe the behavior of all objects in the universe.
Q & A
What is the significance of mathematics in understanding the fundamental laws of the universe?
-Mathematics, particularly calculus, is the language through which the fundamental laws of the universe communicate. It allows us to describe and predict natural phenomena with precision.
How does calculus contribute to the beauty of nature?
-Calculus helps us to understand and quantify the continuous changes in nature, such as the motion of objects, which in turn reveals the inherent beauty and complexity of natural processes.
What role does calculus play in modern physics?
-Calculus is essential for making predictions in modern physics, as it provides the mathematical tools to describe and analyze changes in quantities over time and space.
What variables are represented by Y and X in the context of the script?
-In the script, variable Y represents distance in space, while variable X represents moments in time.
What does the purple line in the script represent?
-The purple line represents the relationship between space and time, illustrating the distance in space for every moment in time.
How is speed defined in the context of the script?
-Speed is defined as the distance traveled in one unit of time and is represented by the areas of green rectangles in the script.
What is the significance of adding the areas of all the rectangles together?
-Adding the areas of all the rectangles together gives the total distance traveled by an object over a period of time, which is a fundamental concept in calculus.
How does a negative speed affect the calculation of distance traveled?
-A negative speed, represented by yellow rectangles, indicates that the object is moving backwards. The negative areas of these rectangles cancel out the positive areas of the green rectangles, resulting in the net distance traveled.
What does the Fundamental Theorem of Calculus state?
-The Fundamental Theorem of Calculus states that the integral of the speed (or the rate of change) over a time interval is equal to the total distance traveled during that interval.
What is the derivative of y with respect to x, and how is it represented in the script?
-The derivative of y with respect to x is the rate of change of y with respect to x, represented as 'dy/dx' in the script. It is the slope of the tangent line to the graph of y at a particular point and indicates the speed at that moment.
How is the concept of 'dx' used in calculus to describe small increments of time?
-'dx' represents a very small increment of time. In calculus, it is used to analyze the behavior of a function, such as speed, over an infinitesimally small interval, which allows for the calculation of derivatives and integrals.
What is the integral of f(x), and how does it relate to the distance traveled?
-The integral of f(x) is the sum of all the values of f(x) multiplied by 'dx' over an interval. It represents the area under the curve of the graph of f(x) and, in the context of the script, is equivalent to the total distance traveled over that interval.
Outlines
📐 Introduction to Calculus and Its Role in Understanding Nature
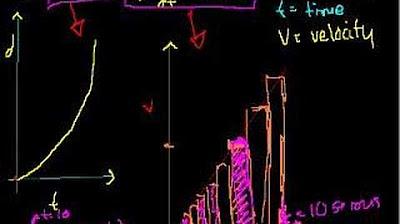
This paragraph introduces the fundamental laws of the universe and their mathematical language, with calculus as the key to understanding it. Calculus is highlighted as essential for appreciating the beauty of nature and enabling the predictions of modern physics. The variables Y and X are defined as representing distance in space and moments in time, respectively. The relationship between space and time is illustrated by a purple line, which shows the distance for every moment. The concept of speed as distance traveled in one unit of time is introduced, with speed represented by green rectangles. The paragraph concludes by illustrating how the sum of the areas of these rectangles equates to the total distance traveled.
🚀 Variable Speed and the Fundamental Theorem of Calculus
The second paragraph explores scenarios where objects change speed and move in reverse, represented by yellow rectangles indicating negative speed. The concept of adding the areas of rectangles to find the total distance traveled is reiterated, with the inclusion of negative areas to account for backward movement. The graph's transition from having sharp corners to a smooth curve is described, emphasizing how the Fundamental Theorem of Calculus applies to all types of graphs. The theorem is introduced as stating that the sum of rectangles equals the distance traveled. The concept of a small increment of time 'dx' and distance 'dy' is explained, with 'dy/dx' representing the speed and later re-labeled as the derivative of y with respect to x, symbolizing the slope of the line at any given moment.
🏞️ The Derivative and Its Relation to Slope and Speed
This paragraph delves into the concept of the derivative, 'dy/dx', and its relation to the slope of a line, which varies depending on whether the object is speeding up, moving at a constant speed, or moving backwards. The changes in slope are associated with abrupt changes in 'dy/dx', especially at sharp corners of the graph. When the speed changes smoothly, 'dy/dx' is renamed as a function f(x), which corresponds to the slope at every moment in time. The distance traveled during an infinitesimally small moment is described as the product of speed (f(x)) and time (dx), equating to f(x) multiplied by dx. This product also represents the area of the rectangle for that moment, linking the concept of distance to the geometric concept of area.
🌌 The Integral and Calculating Total Distance Traveled
The final paragraph explains the concept of the integral, which represents the sum of all values of f(x) multiplied by dx, symbolizing the total area under the graph. This area corresponds to the total distance traveled by the object. The integral is described with a specific focus on the area between X equals zero and X equals ten, denoted as the integral of f(x) from zero to ten. The paragraph reinforces the Fundamental Theorem of Calculus, which equates the integral of the speed graph to the distance traveled. This theorem is portrayed as the foundation for writing equations that describe the behavior of all objects in the universe, emphasizing that the universe's fundamental laws are written in the language of calculus.
Mindmap
Keywords
💡Calculus
💡Derivative
💡Integral
💡Fundamental Theorem of Calculus
💡Speed
💡Distance
💡Time
💡Graph
💡Rectangles
💡Slope
💡Negative Speed
💡Continuous Curve
Highlights
The fundamental laws that govern the universe speak to us in the language of mathematics.
Calculus is the key to understanding the mathematical language of the universe.
Calculus allows us to see the true beauty of nature through mathematical relationships.
Calculus makes the predictions of modern physics possible by quantifying change over time.
Variable Y represents distance in space, while variable X represents moments in time.
The purple line shows the relationship between space and time for every moment.
Speed is the distance traveled in one unit of time, represented by green rectangles.
Adding the areas of all the rectangles gives the total distance traveled.
When speed changes or is negative (yellow rectangles), the areas still sum to the net distance traveled.
The Fundamental Theorem of Calculus states that the sum of the rectangles always equals the distance traveled.
The derivative, dy/dx, represents the instantaneous speed as the slope of the line at any point.
A smooth curve (no sharp corners) means the speed changes smoothly over time.
The distance traveled during a small time increment is the speed (f(x)) multiplied by the time (dx).
The area of each rectangle equals the distance traveled during that time interval.
The integral of f(x) represents the sum of all the areas (positive and negative) under the curve.
The integral from a to b gives the total distance traveled between times a and b.
The Fundamental Theorem of Calculus links derivatives (rates of change) and integrals (accumulated change).
Calculus provides the foundation for writing equations that describe how all objects in the universe behave.
The language of calculus is the language in which the fundamental laws of the universe are written.
Transcripts
Browse More Related Video

Integration and the fundamental theorem of calculus | Chapter 8, Essence of calculus

What Is an Integral?
Definite integrals (part II)

Business Calculus - Math 1329 - Section 5.3 - The Definite Integral and Fundamental Thm of Calculus

Distance vs. Displacement & Speed vs. Velocity | Kinematics Explained

Math 1325 Lecture 13 2
5.0 / 5 (0 votes)
Thanks for rating: