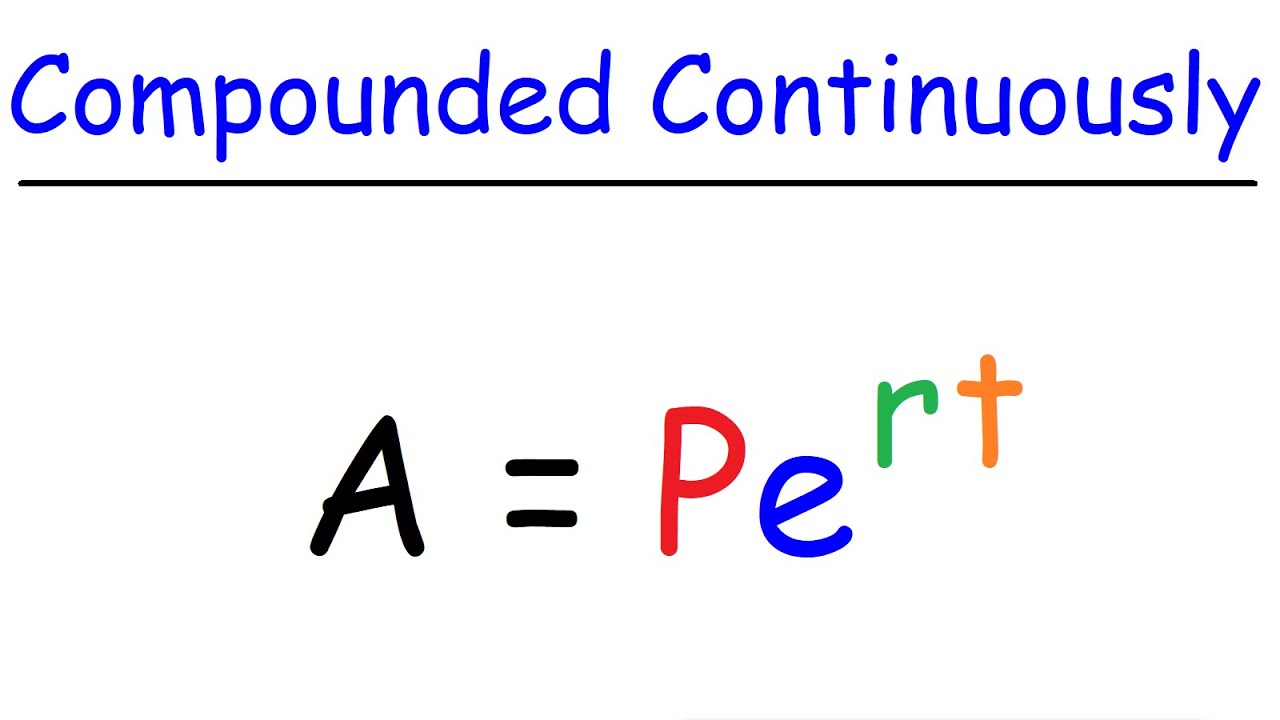
Continuous Money Flow: Future Value with increasing rate of growth
TLDRThe video script delves into the concept of continuous money flow, specifically focusing on the future value of an income stream. It introduces the formula for calculating the future value, which involves an integral of the revenue or income stream multiplied by e^(rt), where r is the interest rate and t is time. The script explains three forms of revenue or income: constant, linear, and percentage increase. Using an example of a bi-weekly paycheck with a 5% annual increase, the video demonstrates how to calculate the future value over a 20-year period with an annual interest rate of 4.45%. The process involves multiplying the revenue function by e^(0.05t) and integrating over time, simplifying the expression, and applying u-substitution to find the antiderivative. The final future value is approximately $541,124.39, illustrating the power of continuous compounding and investment growth.
Takeaways
- 📈 The future value of a continuous money flow is calculated using an integral formula that involves revenue or income multiplied by e^(rt) over a time period.
- 💼 Revenue is the income a business receives, while income is the money an individual earns or takes in.
- 📊 Revenue or income can be represented as a constant function, a linear function (with annual raises), or a continuously compounded percentage increase.
- 💵 A constant revenue function could be a fixed annual salary, such as $50,000 per year.
- 📈 A linear revenue function could represent an annual raise, like starting with $50,000 and increasing by $2,000 each year.
- 🔺 A percentage increase in revenue, such as a 5% raise, is modeled using continuous compounding, which is e^(0.05t).
- 🧮 The example in the script involves a bi-weekly paycheck of $350, which is compounded annually by 5%.
- 🚀 The revenue function for the example is calculated as $9,100 * e^(0.05t), representing the annualized income with a 5% increase.
- 📉 The interest rate used in the example is 4.45%, which is the rate at which the investment grows.
- ⌛ The integral is calculated over a period of 20 years to find the future value of the investment.
- 🧮 Using u-substitution, the future value is found by integrating e^(0.0945t) and evaluating from 0 to 20 years.
- 💰 The calculated future value of the investment is approximately $541,124.09, given the conditions in the example.
Q & A
What is the formula for the future value of a continuous money flow?
-The formula for the future value of a continuous money flow is the integral from 'a' to 'b' of the revenue or income stream, times e to the r*t, dt, where r is the revenue or income and t is the time.
What does 'rft' represent in the context of the formula?
-'Rft' stands for revenue or income, which refers to the money that a business takes in or an individual earns or receives.
What are the three forms that revenue or income can take in these problems?
-The three forms that revenue or income can take are: a constant function, a linear function, and a continuously compounded percentage increase.
If you earn a constant $50,000 a year, what would your revenue function be?
-If you earn a constant $50,000 a year, your revenue function would be a constant function with a value of $50,000.
How would you represent a linear increase in revenue, such as a $2,000 raise every year?
-A linear increase in revenue, like a $2,000 raise every year, would be represented by a linear function starting with $50,000 and increasing by $2,000 times t.
What is the formula for a continuously compounded percentage increase in revenue?
-The formula for a continuously compounded percentage increase in revenue is to take the initial amount, in this case $50,000, and multiply it by e raised to the power of the percentage increase times t, where the percentage increase is expressed as a decimal.
What is the revenue function for an individual receiving $350 every other week for an entire year, with a 5% increase per year?
-The revenue function would be 9100 (which is $350 times 26 bi-weekly paychecks) times e to the power of 0.05t, representing a 5% increase per year.
What is the annual interest rate used in the example provided in the script?
-The annual interest rate used in the example is 4.45 percent.
How is the integral in the future value formula simplified when multiplying e terms together?
-When multiplying e terms together, you use the exponent rule to add the exponents since the base is the same. This simplifies the integral to a single e term with the sum of the original exponents.
What is the final future value calculated in the script, and what does it represent?
-The final future value calculated is approximately $541,124.39. This represents the accumulated amount from investing $350 every other week for 20 years with a 5% annual increase and an interest rate of 4.45%.
How was the antiderivative obtained in the script?
-The antiderivative was obtained through u-substitution on the power of e, evaluating e to the 0.0945t from 0 to 20 years.
What is the significance of the future value calculation in financial planning?
-The future value calculation is significant in financial planning as it helps individuals and businesses understand the potential growth of their investments or savings over time, taking into account factors like regular contributions and compound interest.
Outlines
📈 Introduction to Continuous Money Flow and Future Value
This paragraph introduces the concept of continuous money flow and its future value calculation. The formula for future value is presented as an integral of a revenue or income stream over time, multiplied by e^(r*t). Revenue is defined as the money a business takes in, while income is the money an individual earns. The revenue or income can be constant, linear, or increase by a percentage. The example given involves a bi-weekly paycheck of $350, with a 5% annual increase, compounded continuously. The interest rate is 4.45%, and the future value is calculated over a period of 20 years.
🧮 Calculating the Future Value with a Compound Interest Rate
The second paragraph focuses on the calculation of future value using the formula derived from the first paragraph. It simplifies the integral by combining the exponents of 'e', resulting in an integral of 9100 * e^(.0945*t). The future value is then found by dividing 9100 by .0945 and evaluating the antiderivative from 0 to 20 years. The final result is an estimated future value of approximately $541,124.39, demonstrating the growth of an investment with a starting salary of $350 bi-weekly, increasing by 5% per year, and an interest rate of 4.45%.
Mindmap
Keywords
💡Continuous Money Flow
💡Future Value
💡Integral
💡Revenue or Income Stream
💡Constant Function
💡Linear Function
💡Percentage Increase
💡Continuously Compounded
💡Interest Rate
💡Bi-weekly Paycheck
💡Antiderivative
Highlights
The video provides an example of calculating the future value of a continuous money flow.
The formula for future value is the integral of the revenue or income stream times e^(r*t) dt.
Revenue or income can take one of three forms: constant, linear, or exponential growth.
A constant revenue function means a fixed annual income that does not change.
A linear revenue function increases by a fixed amount each year, like a $2,000 raise.
An exponential revenue function grows at a continuous compounded rate, like a 5% pay raise each year.
The example problem involves a bi-weekly paycheck of $350 that increases by 5% per year.
The revenue function is calculated as $9,100 * e^(0.05*t), where $9,100 is the annualized bi-weekly paycheck.
The interest rate in the problem is 4.45% per year.
The future value integral is from 0 to 20 years of the revenue function times e^(0.0445*t).
By adding the exponents, the integral simplifies to 9.45% * t * dt.
The antiderivative of the integral is (9,100 / 0.0945) * e^(0.0945*t).
Evaluating the antiderivative from 0 to 20 years gives the future value of approximately $541,124.
The example demonstrates how to calculate the future value of an investment with a growing contribution amount and interest rate.
The method involves finding the revenue function, calculating the integral, and evaluating the antiderivative.
The video provides a step-by-step explanation of the process, making it easy to follow.
It's a practical application of calculus in finance for calculating the future value of an investment.
The video is helpful for understanding the concept of continuous money flow and its future value calculation.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: