Finding Vertical Asymptotes of Rational Functions (Precalculus - College Algebra 38)
TLDRThis video script dives into the fascinating world of rational functions, which are essentially fractions with variables in both the numerator and the denominator. The host explains that these functions can lead to intriguing graphical representations and emphasizes the importance of understanding domain issues that arise from the denominator being zero. The video outlines how to identify and graph rational functions, focusing on the concepts of holes and vertical asymptotes. Holes occur when a factor in the denominator that causes it to equal zero can be canceled out by a corresponding factor in the numerator, while vertical asymptotes represent points where the function is undefined because the denominator cannot be zero. The script provides a step-by-step guide on factoring the numerator and denominator, setting factors equal to zero to find domain issues, and determining whether these issues result in holes or vertical asymptotes. The host also clarifies the difference between even and odd multiplicities of factors in the denominator and how they affect the appearance of vertical asymptotes. The summary concludes with the host's intention to continue discussing other types of asymptotes and further graphing techniques in subsequent content.
Takeaways
- 📐 **Rational Functions Defined**: Rational functions are fractions where the numerator and the denominator both contain variables. If the denominator has no variables, it's a polynomial.
- 🚫 **Domain Issues**: The presence of variables in the denominator of a rational function can lead to domain restrictions, specifically where the denominator equals zero.
- ⛔ **Denominator Equals Zero**: When the denominator of a rational function equals zero, it results in a discontinuity, which can manifest as either a hole or a vertical asymptote.
- 🕳 **Holes in Graphs**: A hole, or removable discontinuity, occurs when the factor causing the denominator to equal zero can be canceled by a factor in the numerator.
- 🚧 **Vertical Asymptotes**: A vertical asymptote is present when the factor causing the denominator to equal zero cannot be canceled by any factor in the numerator.
- 🔢 **Multiplicity Matters**: The multiplicity of the factor causing the denominator to equal zero determines the behavior of the vertical asymptote—odd multiplicity results in one behavior, while even multiplicity results in a symmetrical behavior.
- 🔍 **Identifying Discontinuities**: To identify discontinuities, factor the rational function, set each factor of the denominator equal to zero to find the domain issues, and then determine if they are holes or vertical asymptotes.
- 📈 **Graphing Rational Functions**: When graphing, rational functions can be sketched by finding x-intercepts, y-intercepts, vertical asymptotes, and possibly horizontal or oblique asymptotes.
- 🧮 **Numerator's Role**: The numerator of a rational function helps determine x-intercepts and provides information about whether a domain issue is a hole or a vertical asymptote.
- ➗ **Zero Denominator**: A rational function is undefined when the denominator equals zero, which is not allowed as it represents an attempt to divide by zero.
- ✍️ **Graphing Process**: The process of graphing a rational function involves factoring, defining the domain, identifying discontinuities, and then sketching the graph with the correct asymptotes and intercepts.
Q & A
What are rational functions?
-Rational functions are fraction functions where variables are present in both the numerator and the denominator. They can cause domain issues when the denominator equals zero.
What happens when the denominator of a rational function equals zero?
-When the denominator equals zero, it results in a discontinuity, which can either be a hole in the graph or a vertical asymptote, depending on whether the factor causing the zero can be canceled by a factor in the numerator.
What is a hole in the context of rational functions?
-A hole is a point on the graph of a rational function where the function is undefined because the denominator equals zero and the factor causing this can be canceled by a factor in the numerator.
What is a vertical asymptote and how does it differ from a hole?
-A vertical asymptote is a line that the graph of a rational function cannot cross. It occurs when the denominator equals zero and the factor causing this cannot be canceled by a factor in the numerator, unlike a hole where the factor can be canceled.
What is the significance of the multiplicity of a factor in a rational function?
-The multiplicity of a factor that causes the denominator to equal zero determines the behavior of the vertical asymptote. An even multiplicity results in a symmetrical asymptote, while an odd multiplicity results in an asymmetrical one.
How do you identify the domain of a rational function?
-The domain of a rational function is identified by setting each factor of the denominator equal to zero and noting the values of x that make the denominator zero. These values are not included in the domain.
What is an x-intercept and how is it related to the numerator of a rational function?
-An x-intercept is a point where the graph of a function crosses the x-axis. It is found by setting the numerator of the rational function equal to zero and solving for x.
What is the zero product property and how is it used in finding domain issues?
-The zero product property states that if a product of factors equals zero, then at least one of the factors must be zero. It is used to find domain issues by setting each factor of the denominator equal to zero to identify values of x that are not in the domain.
How do you determine the type of discontinuity (hole or vertical asymptote) at a given point?
-To determine the type of discontinuity, factor the rational function, set the denominator equal to zero to find potential domain issues, and then check if the factor causing the issue can be canceled by a factor in the numerator. If it can be canceled, it's a hole; if not, it's a vertical asymptote.
What is the role of the numerator in defining the graph of a rational function?
-The numerator is used to find x-intercepts and to determine whether a discontinuity is a hole or a vertical asymptote. It does not, however, provide specifics for the vertical asymptote beyond its existence.
Why is it important to define the domain of a rational function before identifying asymptotes or x-intercepts?
-Defining the domain first ensures that you identify all the values of x that are not allowed, which helps in accurately determining the discontinuities and preventing the cancellation of factors that should not be canceled, thus avoiding misconceptions about the function's behavior.
Outlines
😀 Introduction to Rational Functions and Their Excitement
The video begins with an introduction to rational functions, which are fractions with variables in both the numerator and the denominator. The presenter emphasizes the excitement around these functions due to the interesting graphs they produce. The importance of understanding domain issues related to the denominator, which can cause problems not seen with polynomials, is highlighted. The concept of domain is reviewed, and the idea that the denominator cannot be zero is reiterated. The video promises to teach techniques for graphing rational functions and to discuss the two types of graph issues that can arise: holes and vertical asymptotes.
📐 Identifying Domain Issues and Asymptotes in Rational Functions
The paragraph delves into the process of identifying domain issues in rational functions when the denominator equals zero, leading to discontinuities. It differentiates between holes, which are removable discontinuities, and vertical asymptotes, which act as a barrier the graph cannot cross. The presenter explains that while holes can be 'filled' by defining the function with an additional point, vertical asymptotes represent a more severe discontinuity. The role of the numerator in determining x-intercepts and the nature of the denominator (whether it results in a hole or a vertical asymptote) is also discussed. The paragraph concludes with a teaser for upcoming examples that will clarify these concepts.
🔍 Factoring and Identifying Domain Problems in Rational Functions
This section focuses on the method of factoring the numerator and denominator of a rational function to identify domain problems. It explains that factors leading to a zero denominator that can be canceled out indicate a hole, whereas factors that cannot be canceled signify a vertical asymptote. The presenter stresses the importance of never canceling out factors that would eliminate a domain problem, as this would mask the true nature of the discontinuity. The paragraph also introduces the concept of multiplicity in factors and how it affects the appearance of vertical asymptotes, with even multiplicity leading to symmetrical behavior and odd multiplicity leading to non-symmetrical behavior.
📈 Sketching Rational Functions: Finding Asymptotes and Intercepts
The paragraph outlines the steps for sketching rational functions, starting with factoring both the numerator and the denominator. It emphasizes setting each factor of the denominator equal to zero to find domain issues and then determining whether these issues result in holes or vertical asymptotes. The presenter also discusses how to find x-intercepts by setting the numerator equal to zero and how to identify y-intercepts. The process of sketching the graph using these identified points and asymptotes is briefly introduced, with a promise to cover it in more detail in subsequent parts of the video.
🚫 Understanding and Defining Discontinuities in Rational Functions
The focus of this paragraph is on understanding the nature of discontinuities in rational functions. It explains that when a factor from the denominator cannot be canceled with a factor from the numerator, it results in a vertical asymptote. The presenter clarifies that vertical asymptotes are graphical representations of domain issues and that they come in four varieties, depending on the multiplicity of the factor causing the zero denominator. The concept of even and odd multiplicity is further explored, showing how it affects the direction of the asymptote as the function approaches the point of discontinuity.
🔗 Factoring and Domain Definition in Graphing Rational Functions
This section provides a detailed explanation of the preliminary steps in graphing rational functions, specifically focusing on factoring and domain definition. It stresses the importance of factoring both the numerator and the denominator and then setting each factor of the denominator equal to zero to find the values for which the function is undefined. The paragraph also explains how to identify the type of discontinuity (hole or vertical asymptote) by attempting to cancel factors from the numerator and denominator. The concept of the zero product property is used to simplify the process of finding domain issues.
🕳️ Dealing with Holes in Rational Functions
The final paragraph discusses how to deal with holes in rational functions. It clarifies that while a hole appears as a point not included in the graph, it is still a point that the graph interacts with. The presenter explains the process of finding the coordinates of a hole by allowing the function to include the value that would normally cause a domain issue, thus revealing the value at which the hole occurs. The paragraph concludes with a reminder of the importance of defining the domain first and then interpreting the discontinuities graphically, ensuring that the graph accurately represents the behavior of the rational function.
Mindmap
Keywords
💡Rational Functions
💡Domain
💡Graph Issues
💡Hole
💡Vertical Asymptote
💡Discontinuity
💡X-Intercepts
💡Factoring
💡Multiplicity
💡Zero Product Property
💡Asymptote Types
Highlights
Rational functions are exciting mathematical entities that are fractions resulting in intriguing graphical representations.
Understanding rational functions involves recognizing them as fractions with variables in both the numerator and the denominator.
Domain issues arise in rational functions when the denominator equals zero, unlike polynomials which are fully continuous.
Two types of graph issues occur when the denominator equals zero: a hole in the graph or a vertical asymptote.
A hole is a point that the function graph misses, while a vertical asymptote is a 'force field' that the graph cannot cross.
The numerator of a rational function provides x-intercepts and helps determine whether the denominator issue is a hole or a vertical asymptote.
Factoring the rational function is the first step to identify potential domain issues and the type of discontinuity.
Removable discontinuities, or holes, occur when a factor causing the denominator to zero can be canceled with a numerator factor.
Vertical asymptotes happen when a factor causing the denominator to zero cannot be canceled and result in one of four graphical cases.
The multiplicity of the factor causing the denominator to zero affects the appearance of vertical asymptotes, with even multiplicity resulting in symmetrical asymptotes.
Identifying and graphing rational functions involves finding x-intercepts, y-intercepts, vertical asymptotes, and possibly horizontal or oblique asymptotes.
The process of graphing rational functions includes factoring, defining the domain, and identifying discontinuities such as holes or vertical asymptotes.
The concept of limits is important when dealing with holes in rational functions, as the limit exists even though the point itself is undefined.
When sketching graphs of rational functions, it's crucial to define the domain first and then identify the type of discontinuity at each point where the denominator is zero.
The numerator's factors can be used to determine if an x-intercept exists and to find the value of a hole if present.
After identifying holes and vertical asymptotes, the function can be simplified for easier graphing, but the original domain and discontinuities must be respected.
Graphing rational functions requires understanding the behavior of the graph around holes and vertical asymptotes, which can be complex but are essential for an accurate representation.
Transcripts
Browse More Related Video
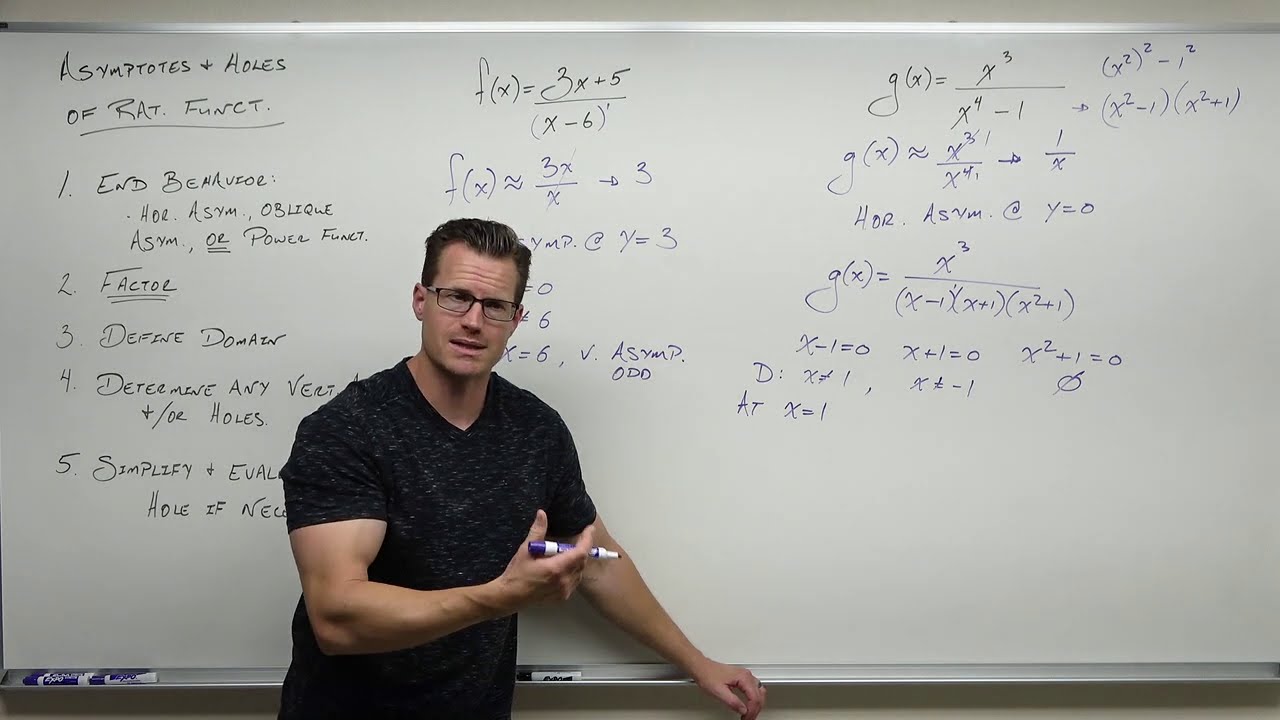
Finding Asymptotes and Holes of Rational Functions (Precalculus - College Algebra 43)

How to graph a rational function using 6 steps

Inequalities with Rational Functions (Precalculus - College Algebra 47)
Horizontal and Vertical Asymptotes - Slant / Oblique - Holes - Rational Function - Domain & Range
How To Find The Vertical Asymptote of a Function

Graphing Rational Functions (Precalculus - College Algebra 44)
5.0 / 5 (0 votes)
Thanks for rating: